4J3, Diffusion of a Chemical | MIT 18.01SC Single Variable Calculus, Fall 2010

MIT OpenCourseWare
7 Jan 201112:22
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the professor introduces a problem involving a shallow circular pool where a disinfecting chemical is released at the center and diffuses symmetrically. The concentration of the chemical at a distance 'r' from the center is given by a formula involving a constant 'k'. The challenge is to determine the total amount of chemical released. The professor suggests using shells or strips to approximate the volume and integrate to find the exact amount, hinting at the use of Riemann sums and the integral of the concentration function over the pool's volume.

Takeaways
  • ๐ŸŠโ€โ™‚๏ธ A shallow circular reflecting pool with depth D and radius R has a disinfecting chemical released at its center.
  • ๐Ÿงช The concentration of the chemical at a distance r from the center is given by k/(1 + r^2) grams per cubic meter.
  • ๐Ÿ“ To find the total amount of chemical released, we need to integrate the concentration over the entire pool.
  • ๐Ÿ“ The pool can be divided into cylindrical shells of thickness ฮ”r, each with an inner radius ri and outer radius ri+1.
  • ๐Ÿ”„ Each shell can be approximated as a flat prism when opened up, with length 2ฯ€ri and height D.
  • ๐Ÿงฎ The volume of each shell is approximately 2ฯ€ri * D * ฮ”r cubic meters.
  • ๐Ÿ“Š The amount of chemical in each shell is the volume of the shell multiplied by the concentration k/(1 + ri^2).
  • โž• Summing the amounts of chemical in all shells gives an approximation for the total amount of chemical in the pool.
  • ๐Ÿ” As the number of shells increases, the sum approaches the integral 2ฯ€Dk โˆซ (r/(1 + r^2)) dr from 0 to R.
  • ๐Ÿ’ก The integral can be solved using the substitution method, recognizing that the derivative of 1 + r^2 is 2r.
Q & A

  • What is the main problem discussed in the video?

    -The main problem is to determine the total amount of a disinfecting chemical that was released into a shallow circular reflecting pool given its concentration at a point r meters from the center.

  • What is the significance of the constant 'k' in the concentration formula?

    -The constant 'k' represents the concentration of the chemical at the center of the pool, in grams per cubic meter.

  • What is the hint provided by the professor for solving the problem?

    -The hint is to visualize the problem by drawing a picture, consider using shells or strips for approximation, and eventually let the approximation tend toward an integral.

  • What is the shape of the pool and its dimensions?

    -The pool is circular with a uniform depth 'D' and a radius 'R', both measured in meters.

  • How does the concentration of the chemical change with distance from the center?

    -The concentration of the chemical decreases as the distance from the center increases, following the formula k / (1 + r^2).

  • What mathematical concept is used to estimate the amount of chemical in the pool?

    -The mathematical concept of Riemann sums is used to estimate the amount of chemical, which converges to an integral as the number of shells tends to infinity.

  • How is the volume of each shell estimated?

    -The volume of each shell is estimated as the product of the circumference of the circle (2ฯ€r), the depth of the pool (D), and the thickness of the shell (ฮ”r).

  • What is the purpose of increasing the number of partitions (shells)?

    -Increasing the number of partitions allows the approximation to become more accurate, as it effectively narrows the width of each shell and leads to a more precise integral calculation.

  • What is the integral set up by the professor to find the exact amount of chemical released?

    -The integral is set up as the limit of the sum of chemical amounts in each shell as the number of shells goes to infinity, represented as โˆซ(2ฯ€Dk * r / (1 + r^2)) dr from 0 to R.

  • What strategy is hinted at for solving the integral?

    -The strategy hinted at involves recognizing that the derivative of 1 + r^2 is 2r, which may simplify the integral and help in solving for the total amount of chemical released.

  • How does the concentration formula relate to the volume of the shell in calculating the amount of chemical?

    -The amount of chemical in each shell is found by multiplying the volume of the shell by the concentration at its interior radius, which accounts for the distribution of the chemical throughout the pool.

Outlines
00:00
๐ŸŒ€ Introduction to the Chemical Diffusion Problem

The professor introduces a mathematical problem involving a shallow circular reflecting pool with uniform depth and radius, where a disinfecting chemical is released at the center. The concentration of the chemical at any point 'r' meters from the center is given by a formula involving a constant 'k'. The task is to determine the total amount of chemical released into the pool. The professor suggests visualizing the problem and using approximations with 'shells' to estimate the total amount, hinting at the transition from these approximations to an integral for a precise calculation.

05:02
๐Ÿ“ Estimating Chemical Release Using Shells

The professor proceeds to explain the process of estimating the amount of chemical released by considering the pool as consisting of concentric shells or strips. Each shell is assumed to have a uniform concentration of the chemical. The volume of each shell is calculated using the formula for the volume of a cylindrical segment, which is then multiplied by the concentration at the shell's inner radius to estimate the chemical amount in that shell. The process involves summing these amounts for all shells from the center of the pool to its radius, with the understanding that as the number of shells increases, the approximation becomes more accurate, ultimately leading to an integral.

10:03
๐Ÿงช Transitioning to an Integral for Exact Calculation

The professor concludes the explanation by transitioning from the approximation method to an exact calculation using an integral. The integral is set up to calculate the total amount of chemical released into the pool by summing the contributions from an infinite number of infinitesimal shells, as the number of shells approaches infinity. The integral is expressed in terms of the given concentration formula and the limits from 0 to the pool's radius 'R'. The professor also hints at a strategy for solving the integral by considering the derivative of the denominator in the concentration formula, which is '1 plus r squared', suggesting that the derivative might simplify the integration process.

Mindmap
Keywords
๐Ÿ’กShallow circular reflecting pool
A shallow circular reflecting pool is a flat, round body of water with a uniform depth. In the video, it serves as the medium in which a disinfecting chemical is released and diffuses symmetrically outward. The pool's uniform depth and radius are essential parameters for the diffusion problem discussed.
๐Ÿ’กDisinfecting chemical
The disinfecting chemical is the substance introduced at the center of the pool, which then diffuses outward. It is the focus of the problem, as the video aims to determine the total amount of this chemical released based on its concentration at various points in the pool.
๐Ÿ’กConcentration
Concentration in this context refers to the amount of a substance, in this case, the disinfecting chemical, per unit volume. The script describes the concentration at a point a little r meters from the center as 'k over 1 plus r squared', which is a mathematical expression representing how the concentration decreases with distance from the center.
๐Ÿ’กDiffusion
Diffusion is the process by which particles spread from an area of higher concentration to an area of lower concentration. In the video, the chemical diffuses symmetrically from the center of the pool, creating a gradient of concentration that decreases with distance from the center.
๐Ÿ’กConstant k
The constant 'k' is a coefficient in the concentration formula that represents a proportionality factor. It is used to calculate the concentration of the chemical at any point within the pool and is essential for determining the total amount of chemical released.
๐Ÿ’กRiemann sums
Riemann sums are a method for approximating the definite integral of a function by summing the products of function values and the width of intervals. In the video, Riemann sums are used as an approximation technique to estimate the total amount of chemical in the pool by summing over concentric shells.
๐Ÿ’กIntegral
An integral is a mathematical concept used to calculate the area under a curve, which in this video, represents the total amount of chemical in the pool. The script suggests taking the limit of Riemann sums as the number of intervals goes to infinity to find the exact integral, which gives the total chemical amount.
๐Ÿ’กVolume
Volume is the measure of the space occupied by an object. In the context of the video, the volume of each cylindrical shell is calculated to estimate the amount of chemical in that shell by multiplying the volume by the concentration of the chemical.
๐Ÿ’กRadii
Radii in the script refer to the different distances from the center of the pool at which the chemical concentration is considered. The video uses a series of radii to partition the pool into shells for the purpose of approximating the total amount of chemical released.
๐Ÿ’กDerivative
A derivative in calculus represents the rate at which a function changes with respect to its variable. The script hints at using the derivative of '1 plus r squared' to solve the integral for the total amount of chemical, as the derivative is related to the concentration formula.
๐Ÿ’กLimit
In mathematics, a limit is the value that a function or sequence approaches as the input or index approaches some value. The video discusses taking the limit as the number of partitions (n) goes to infinity, which turns the Riemann sum into an integral, allowing for the calculation of the exact total amount of chemical.
Highlights

Introduction to the problem: determining the amount of a disinfecting chemical released into a circular reflecting pool.

Description of the pool with uniform depth D and radius R, and the chemical's concentration given by k / (1 + r^2).

Hint provided: use a picture, approximations using shells, and the concept of integrals to solve the problem.

Drawing the pool and illustrating the concept of cylindrical shells for approximation.

Explanation of the method: taking a fixed radius and assuming constant diffusion for small strips (shells).

Introduction of Riemann sums and the transition to integrals for better approximations.

Setting up n partitions from r_0 = 0 to r_n = R to estimate the volume of the cylindrical shells.

Calculating the volume of each shell as 2ฯ€r_iDฮ”r.

Multiplying the volume by the concentration to find the amount of chemical in each shell.

Summing the amounts over all shells to get the total amount of chemical in the pool.

Converting the sum to an integral to find the exact amount of chemical.

Setting up the integral: โˆซ[0, R] (2ฯ€Dk r / (1 + r^2)) dr.

Hint for solving the integral: consider the derivative of (1 + r^2).

Summary of the process: from estimating using shells to finding the integral for the total chemical amount.

Conclusion: Exploiting the relationship between sums and integrals for an accurate calculation.

Transcripts

Browse More Related Video

Integrals, Population, and Radial Density Functions

2022 AP Calculus AB BC Free Response #4

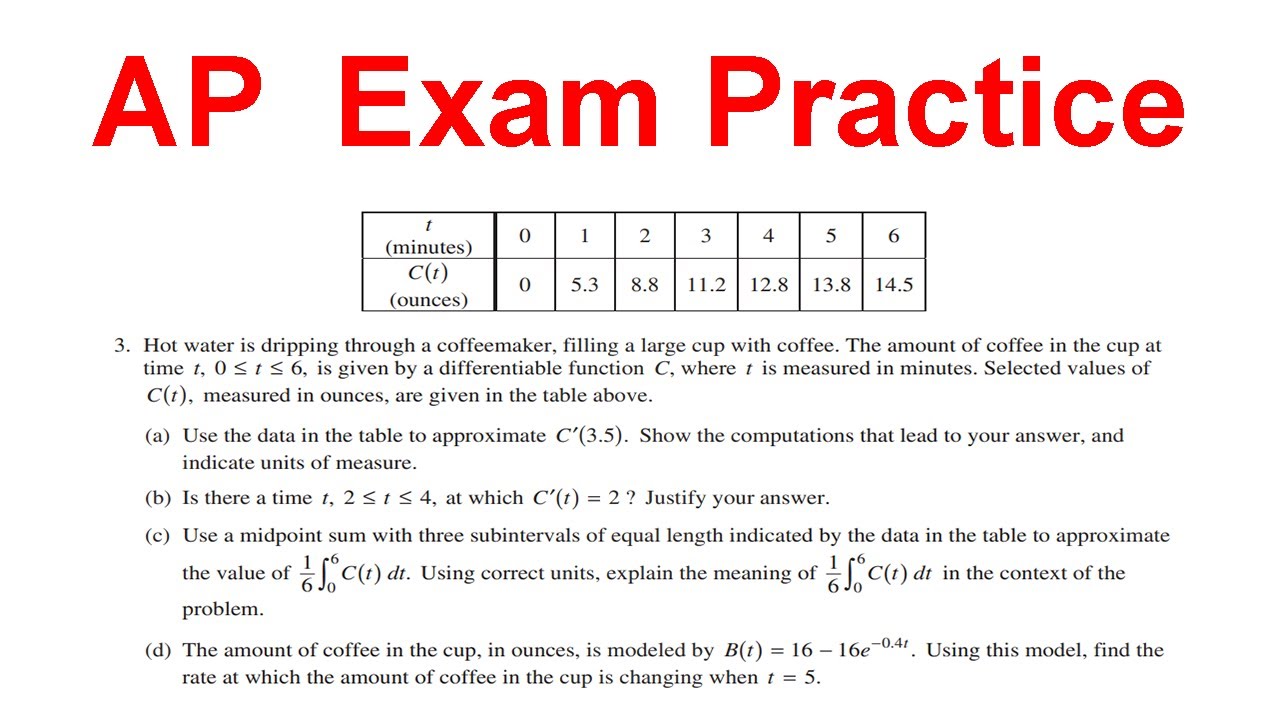
How to solve Table of Values Questions on the AP Calc AB Exam

2021 AP Calculus AB Free Response #1 Solutions (First Administration)

2023 AP Calculus AB & BC FRQ #1

2011 Calculus AB free response #5a | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Chemical DiffusionMathematical ModelingCircular PoolConcentration GradientIntegral CalculusEducational VideoProblem SolvingVolume EstimationRiemann SumsDiffusion Analysis