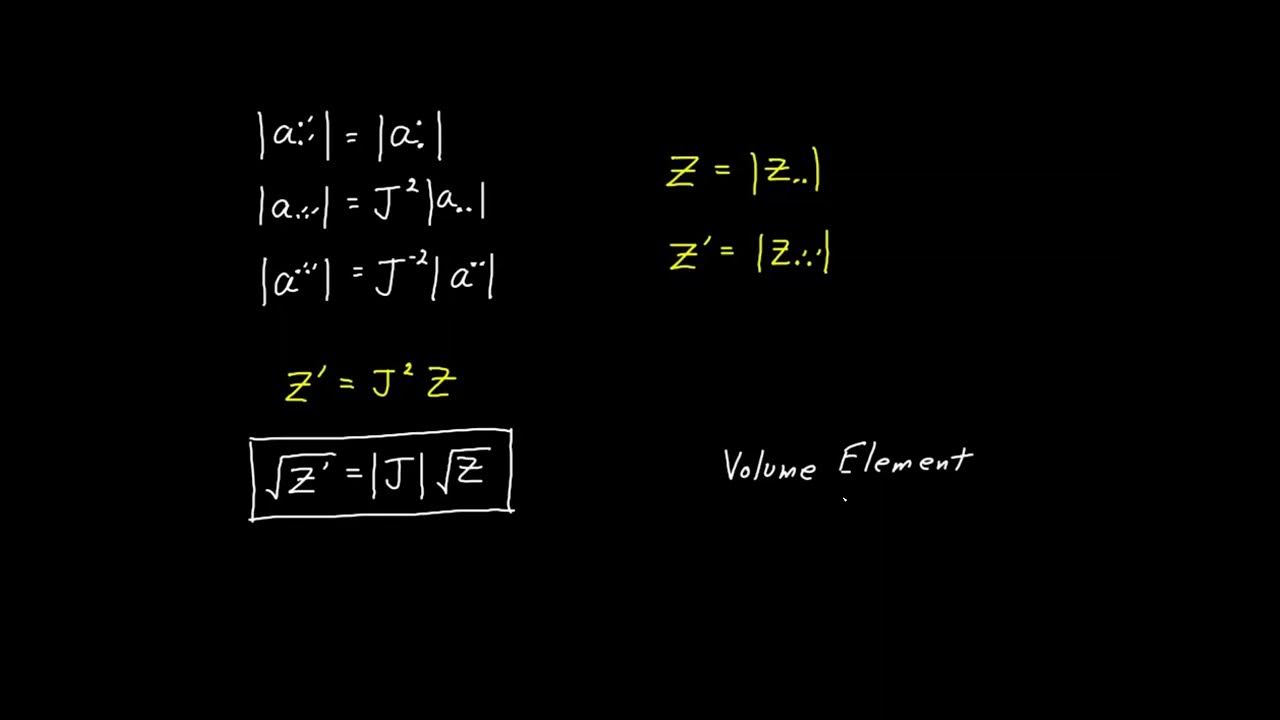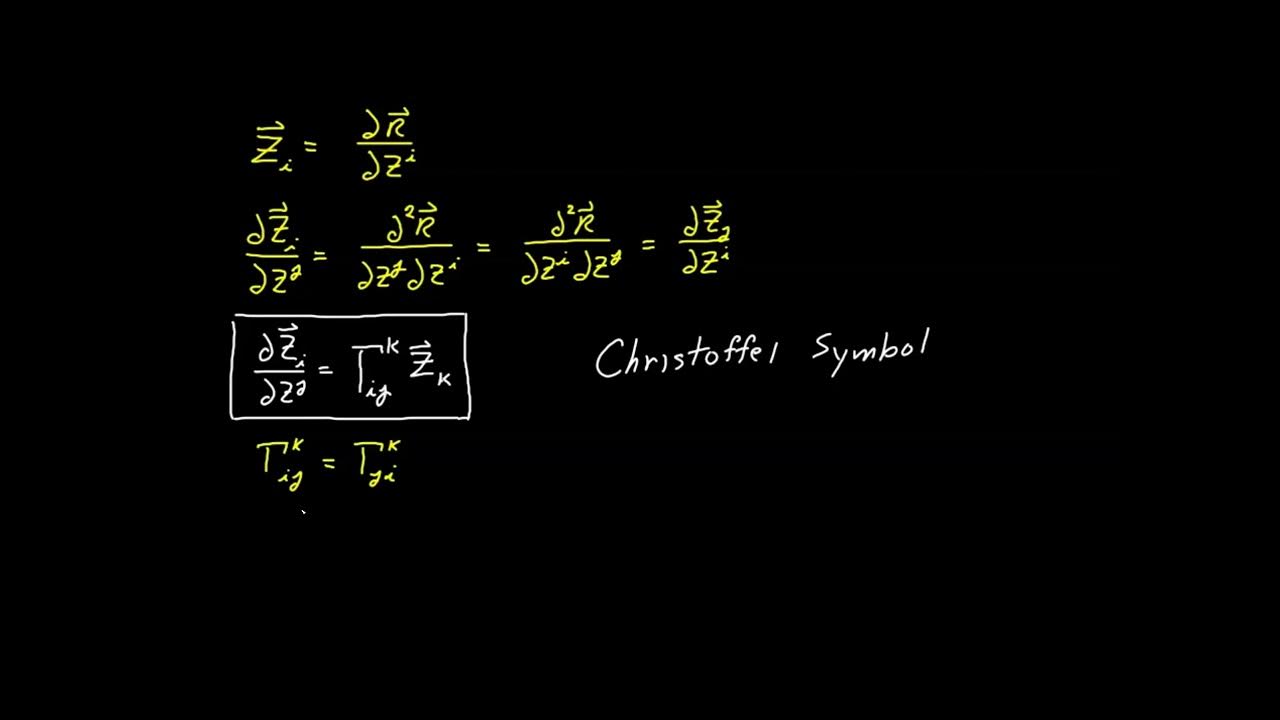Video 31 - Christoffel Symbol Examples
TLDRThis video, part 31 of a series on tensor calculus, focuses on calculating Christoffel symbols in various coordinate systems using a formula derived in a previous episode. The script explains that in Cartesian and affine coordinates, all Christoffel symbols are zero due to constant metric tensors. For plane polar and cylindrical polar coordinates, the non-zero symbols are calculated by identifying non-constant tensor components and their partial derivatives. The process is streamlined by recognizing symmetry and orthogonality. Spherical polar coordinates are also covered, with the script detailing the non-zero symbols resulting from partial derivatives of the metric tensor. The video concludes with a clear presentation of the Christoffel symbols for each coordinate system.
Takeaways
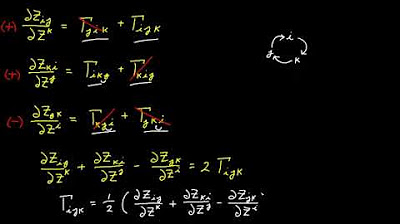
- 📚 The video discusses the evaluation of Christoffel symbols in various coordinate systems using a formula derived in a previous video.
- 🎯 In Cartesian coordinates, the covariant metric tensor is constant, resulting in all Christoffel symbols being zero.
- 🔍 For affine coordinates, similar to Cartesian, the metric tensor elements are constants, leading to zero Christoffel symbols.
- 🌀 Transitioning to polar coordinates, the script explains that only certain permutations of indices will yield non-zero Christoffel symbols due to the non-constant nature of specific tensor elements.
- 📉 The video highlights a technique to reduce computational work by identifying non-constant tensor elements and their dependencies, which dictate the non-zero Christoffel symbols.
- 📈 In plane polar coordinates, only the Christoffel symbols with indices (2,2,1) in some permutation are non-zero, simplifying calculations.
- 📊 The script demonstrates the calculation of non-zero Christoffel symbols for plane polar coordinates, showing specific results for γ₁₂², γ₂₁², and γ₂₂₁.
- 🌐 Moving to cylindrical polar coordinates, the results are almost identical to plane polar coordinates due to the similar nature of the metric tensor.
- 🌍 For spherical polar coordinates, the script identifies two non-constant elements in the metric tensor, leading to a more complex set of non-zero Christoffel symbols.
- 📘 The video concludes with the derived non-zero Christoffel symbols for both the first and second kind in spherical polar coordinates, providing a comprehensive understanding of their calculation.
Q & A
What is the main topic of this video?
-The main topic of this video is the evaluation of Christoffel symbols in various sample coordinate systems using tensor calculus.
Why are all the Christoffel symbols equal to zero in Cartesian coordinates?
-In Cartesian coordinates, the covariant metric tensor is made up of constants, which means all its partial derivatives are zero, leading to all Christoffel symbols being zero.
What is the situation with Christoffel symbols in affine coordinates?
-Similar to Cartesian coordinates, in affine coordinates, the covariant metric tensor elements are constants, resulting in all partial derivatives being zero and thus all Christoffel symbols being zero.
How many different possibilities are there for Christoffel symbols in two-dimensional plane polar coordinates?
-There are eight different possibilities for the Christoffel symbols in two-dimensional plane polar coordinates.
Why can we reduce the number of calculations for Christoffel symbols in plane polar coordinates?
-We can reduce the number of calculations because the right two indexes of the Christoffel symbols are symmetric, and the covariant metric tensor has only one non-constant component, which depends only on the variable r.
What is the only non-zero partial derivative in the covariant metric tensor for plane polar coordinates?
-The only non-zero partial derivative in the covariant metric tensor for plane polar coordinates is ∂z/∂r for the element z22, which is 2r.
Which Christoffel symbols in plane polar coordinates are non-zero?
-The non-zero Christoffel symbols in plane polar coordinates are those with indices being some permutation of 2, 2, and 1.
How are the Christoffel symbols of the second kind related to the first kind?
-The Christoffel symbols of the second kind are obtained by raising the first index of the first kind using the contravariant metric tensor elements.
What is the result for the Christoffel symbol γ^1_22 in plane polar coordinates?
-The result for the Christoffel symbol γ^1_22 in plane polar coordinates is -r.
How many non-zero Christoffel symbols of the first kind are there in spherical polar coordinates?
-There are six non-zero Christoffel symbols of the first kind in spherical polar coordinates.
Outlines
📚 Introduction to Christoffel Symbols in Tensor Calculus
This paragraph introduces the topic of Christoffel symbols in tensor calculus, focusing on their evaluation in various coordinate systems using a formula derived from the previous video. The speaker explains that in Cartesian coordinates, the covariant metric tensor is a constant identity matrix, resulting in all Christoffel symbols being zero. The same result applies to affine coordinates due to their constant elements. The paragraph sets the stage for a deeper exploration of Christoffel symbols in different coordinate systems.
🌐 Analyzing Christoffel Symbols in Plane Polar Coordinates
The speaker delves into the specifics of calculating Christoffel symbols in plane polar coordinates. They note that although there are eight theoretical possibilities due to the two-dimensional nature of the system, symmetry reduces the unique calculations to six. A key insight is that only one component of the covariant metric tensor varies with the radial coordinate 'r', leading to a significant simplification in the calculation process. The paragraph concludes with the identification of the only non-zero Christoffel symbols, which are permutations of the indices 2, 2, and 1.
📉 Calculation of Christoffel Symbols in Cylindrical Polar Coordinates
This section mirrors the process of calculating Christoffel symbols in cylindrical polar coordinates, which are similar to plane polar coordinates but in three dimensions. The speaker highlights that only one partial derivative of the covariant metric tensor is non-zero, significantly reducing the number of calculations required. The paragraph walks through the evaluation of the three non-zero Christoffel symbols, which are permutations of the indices 1, 2, and 2, and concludes with the corresponding second kind Christoffel symbols.
🌍 Deriving Christoffel Symbols for Spherical Polar Coordinates
The final paragraph addresses the derivation of Christoffel symbols in spherical polar coordinates. The speaker identifies two non-constant elements in the covariant metric tensor, leading to a limited set of non-zero partial derivatives. The paragraph systematically evaluates the possible non-zero Christoffel symbols, which are permutations of the indices 1, 2, and 3, and their corresponding second kind symbols. The process involves recognizing the symmetry in the indices and the orthogonal nature of the system, resulting in a concise set of results for both the first and second kind Christoffel symbols.
🔚 Conclusion on Christoffel Symbols Across Coordinate Systems
In conclusion, the speaker summarizes the process of deriving Christoffel symbols for various coordinate systems, emphasizing the methodical approach and the insights gained from understanding the properties of the covariant metric tensor in each system. The paragraph reinforces the importance of recognizing constant elements and symmetries to simplify calculations. The speaker expresses confidence that viewers should now be equipped to derive Christoffel symbols for any coordinate system.
Mindmap
Keywords
💡Tensor Calculus
💡Christoffel Symbols
💡Covariant Metric Tensor
💡Partial Derivatives
💡Cartesian Coordinates
💡Affine Coordinates
💡Polar Coordinates
💡Cylindrical Polar Coordinates
💡Spherical Polar Coordinates
💡Symmetry
💡Contravariant Metric Tensor
Highlights
Introduction to the process of evaluating the Christoffel symbols in various sample coordinate systems using a derived formula.
In Cartesian coordinates, the Christoffel symbols are all zero due to the covariant metric tensor being constant.
Affine coordinates also result in zero Christoffel symbols for the same reason as Cartesian coordinates.
In plane polar coordinates, the non-zero Christoffel symbols are limited due to the non-constant component of the metric tensor.
Efficient calculation of Christoffel symbols by identifying non-zero partial derivatives in the metric tensor.
Specific non-zero Christoffel symbols in plane polar coordinates are identified through permutations of indices.
Derivation of Christoffel symbols of the second kind by raising the first index using the contravariant metric tensor.
Orthogonal systems simplify the calculation of Christoffel symbols of the second kind due to diagonal elements.
Cylindrical polar coordinates exhibit similar results to plane polar coordinates in Christoffel symbols.
Spherical polar coordinates involve more non-zero partial derivatives, leading to more non-zero Christoffel symbols.
The calculation of Christoffel symbols in spherical polar coordinates is demonstrated with specific examples.
Christoffel symbols of the second kind in spherical polar coordinates are derived using the contravariant metric tensor.
The final results for Christoffel symbols in spherical polar coordinates are presented for both the first and second kinds.
The video concludes with a comprehensive understanding of deriving Christoffel symbols for any coordinate system.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: