Entropy Change For Melting Ice, Heating Water, Mixtures & Carnot Cycle of Heat Engines - Physics

The Organic Chemistry Tutor

5 Dec 201722:08

EducationalLearning

32 Likes 10 Comments

TLDRThis video script offers a comprehensive tutorial on calculating entropy changes in various physical processes. It covers the entropy change during the melting of ice, heating of water, and mixing of water at different temperatures, using formulas involving heat transfer, mass, specific heat capacity, and temperature. The script also explains the concept of entropy in the context of a Carnot engine, illustrating how to determine the temperature of a hot reservoir and the entropy change of the engine's cycle, reinforcing the principle that reversible cyclic processes in ideal heat engines result in zero net entropy change.

Takeaways

🔄 The entropy change is calculated as the heat transferred divided by the temperature, and it is a measure of disorder in a system.
❄️ For the melting of ice, the entropy change is found using the formula: mass of ice times the heat of fusion divided by the temperature in Kelvin.
🌡️ When dealing with a temperature change, an average temperature is used for the entropy calculation, as seen in heating water from 0°C to 100°C.
📉 The entropy change can be calculated using the formula involving the natural logarithm of the final temperature divided by the initial temperature for heating processes.
💧 In the mixing of water samples at different temperatures, the final temperature of the mixture is the average of the initial temperatures.
↕️ Entropy change for a substance is positive when the temperature increases and negative when it decreases, indicating an increase or decrease in disorder.
🔄 For an isothermal process, the entropy change is positive when heat is absorbed and negative when heat is released.
🔄 In a Carnot engine, the ratio of heat absorbed to heat released is equal to the ratio of the temperatures of the hot and cold reservoirs.
🔄 The net entropy change for a reversible cyclic process, such as the Carnot cycle, is zero, as the system returns to its initial state.
📈 The entropy change for an adiabatic process is zero since no heat is exchanged with the surroundings.
📚 The formula for entropy change during heating or cooling of a substance can be derived from the integral of dq/T, where dq is a small change in heat.

Q & A

What is the formula for calculating the entropy change for a process?
-The entropy change for a process is calculated using the formula ΔS = Q/T, where Q is the heat transferred and T is the temperature in Kelvin.
How is the entropy change for the melting of ice at 0 degrees Celsius calculated in the script?
-The entropy change for the melting of ice is calculated by using the formula ΔS = (mass × heat of fusion) / temperature. In the script, it's 15 grams of ice with a heat of fusion of 334 joules per gram at a temperature of 273.15 Kelvin, resulting in an entropy change of 18.34 joules per kelvin.
What is the role of the specific heat capacity in calculating the entropy change for heating water?
-The specific heat capacity is used in the formula Q = mcΔT to calculate the heat energy required to heat up water, where m is the mass, c is the specific heat capacity, and ΔT is the change in temperature.
Why is an average temperature used when calculating the entropy change for heating water from 0 to 100 degrees Celsius?
-An average temperature is used because the temperature is not constant during the heating process. The average temperature helps to account for the temperature change over the entire process.
What is the formula for calculating the entropy change using natural logs, and when is it applicable?
-The formula for calculating the entropy change using natural logs is ΔS = mc * ln(Tf/Ti), where Tf is the final temperature, Ti is the initial temperature, m is the mass, and c is the specific heat capacity. This formula is applicable when heating or cooling a substance and calculating the entropy change for a process with a temperature change.
How is the final temperature of a mixture calculated when two samples of water at different temperatures are mixed?
-The final temperature of a mixture is calculated by averaging the initial temperatures of the two samples. In the script, 3 kg of water at 70 degrees Celsius is mixed with 3 kg at 30 degrees Celsius, resulting in an average final temperature of 50 degrees Celsius.
What is the significance of the entropy change being positive or negative in the context of heating or cooling a substance?
-A positive entropy change indicates an increase in disorder, which occurs when a substance is heated and its temperature increases. Conversely, a negative entropy change indicates a decrease in disorder, which occurs when a substance is cooled and its temperature decreases.
How is the temperature of the hot reservoir in a Carnot engine determined in the script?
-The temperature of the hot reservoir in a Carnot engine is determined using the ratio of heat absorbed (Qh) to heat discarded (Qc), which is equal to the ratio of their respective temperatures (Th/Tc). In the script, with Qh = 12,000 joules and Qc = 6,000 joules at Tc = 300 Kelvin, Th is calculated to be 600 Kelvin.
What is the net entropy change for an ideal Carnot cycle, and why?
-The net entropy change for an ideal Carnot cycle is zero because it is a reversible cyclic process. The total entropy increase from the heat absorbed is balanced by the entropy decrease from the heat discarded.
How is the entropy change for the isothermal expansion and compression in a Carnot cycle calculated, and what is the result?
-The entropy change for isothermal processes is calculated using ΔS = Q/T. For the isothermal expansion, ΔSh = Qh/Th, and for the isothermal compression, ΔSc = Qc/Tc. In the script, both processes result in an entropy change of ±20 joules per kelvin, which cancel each other out, confirming the net entropy change for the Carnot cycle is zero.

Outlines

00:00

🧊 Entropy Change in Melting Ice

This paragraph discusses the calculation of entropy change when 15 grams of ice at zero degrees Celsius melts. Entropy, a measure of disorder, is calculated using the formula ΔS = Q/T, where Q is the heat transferred and T is the temperature in Kelvin. The heat transferred (Q) is found by multiplying the mass of the ice (15g) by the heat of fusion (334 J/g), resulting in 5015 J. The temperature is 273.15 K. The entropy change is thus 18.34 J/K. The concept of entropy units is also explained, emphasizing that they are in joules per kelvin.

05:01

🌡 Entropy Change During Heating of Water

The second paragraph explains how to calculate the entropy change when heating two kilograms of water from zero to 100 degrees Celsius. It introduces the concept of using an average temperature due to the temperature change. The heat energy required is calculated using the mass, specific heat capacity of water (4184 J/kg·°C), and the temperature change. The average temperature is found by averaging 273 K and 373 K, resulting in 323.15 K. The entropy change is calculated to be 2590 J/K using the formula ΔS = Q/T. An alternative, more precise method using the natural logarithm of the final and initial temperatures is also presented, yielding a result of 2612 J/K.

10:01

🔄 Entropy Change in Water Mixture

This paragraph explores the entropy change when mixing three kilograms of water at 70 degrees Celsius with another three kilograms at 30 degrees Celsius. It explains that the final temperature of the mixture is the average of the two initial temperatures. The entropy change for the hot water sample cooling down to 50 degrees Celsius is calculated using the formula ΔS = Q/T, with Q being the heat transferred and T the average temperature. The entropy change for the hot water is negative (-753.9 J/K), while for the cold water, it is positive (802 J/K) as its temperature increases. The total entropy change for the process is the sum of both, resulting in a net increase of 48.1 J/K.

15:03

🔧 Derivation of Entropy Change Formula

The fourth paragraph delves into the derivation of the formula for calculating entropy change when heating or cooling a substance. It starts with the integral form of entropy change, ΔS = ∫dQ/T, and substitutes dQ with mc dT, where mc is the product of mass and specific heat capacity. The integral of 1/T is then evaluated from the initial to the final temperature, resulting in the formula ΔS = mc (ln(T2) - ln(T1)). This formula is simplified to ΔS = mc ln(T2/T1), showing that the entropy change is positive when the temperature increases and negative when it decreases.

20:03

🔄 Carnot Engine Entropy Analysis

The final paragraph discusses the entropy change in a Carnot engine cycle, where 12,000 joules of heat energy are absorbed from a hot reservoir and 6,000 joules are discarded to a cold reservoir at 300 K. The temperature of the hot reservoir is calculated using the efficiency of the Carnot cycle. The entropy change for the Carnot cycle is analyzed by considering the isothermal expansion and compression steps, where entropy changes are calculated using ΔS = Q/T. For the isothermal expansion, the entropy change is +20 J/K, and for the isothermal compression, it is -20 J/K, resulting in a net entropy change of zero for the cycle, which is consistent with the second law of thermodynamics.

Mindmap

Keywords

💡Entropy Change

Entropy change is a measure of the change in disorder in a system. In the context of the video, it is calculated using the formula where entropy change equals the heat transferred divided by the temperature. The script uses this concept to explain the entropy change during the melting of ice and the heating of water, illustrating how the disorder of a system can be quantified and analyzed in thermodynamic processes.

💡Heat of Fusion

Heat of fusion refers to the amount of heat energy required to change a substance from the solid phase to the liquid phase at its melting point without altering its temperature. In the script, the heat of fusion for ice is given as 334 joules per gram, which is used to calculate the entropy change when 15 grams of ice melts at zero degrees Celsius.

💡Specific Heat Capacity

Specific heat capacity is the amount of heat required to raise the temperature of a unit mass of a substance by one degree Celsius. The video script mentions the specific heat capacity of water as 4184 joules per kilogram per degree Celsius, which is essential for calculating the entropy change when water is heated from zero to 100 degrees Celsius.

💡Temperature

Temperature is a measure of the average kinetic energy of the particles in a substance. The script discusses temperature in both Celsius and Kelvin scales, emphasizing the importance of temperature in calculating entropy change. For example, the temperature is crucial in determining the heat required to melt ice and to heat water.

💡Joules per Kelvin

Joules per Kelvin is the unit of entropy, indicating the amount of heat transferred per unit temperature change. The script mentions this unit in the context of the entropy change calculations for melting ice and heating water, showing how the disorder of a system can be quantified in these units.

💡Average Temperature

Average temperature is used when the temperature of a system is not constant during a process. The video script uses the concept of average temperature to calculate the entropy change during the heating of water, where the temperature changes from 0 to 100 degrees Celsius, by averaging the initial and final temperatures.

💡Natural Logarithm

The natural logarithm, often denoted as ln, is a mathematical function used in the script to calculate the entropy change when heating or cooling a substance. The formula mc * ln(final temperature / initial temperature) is presented as a more precise way to determine entropy change, reflecting the logarithmic relationship between temperature and entropy.

💡Carnot Engine

A Carnot engine is an idealized heat engine that operates on the reversible Carnot cycle. The script discusses the Carnot engine in the context of an energy flow chart, where it absorbs 12,000 joules from a hot reservoir and discards 6,000 joules to a cold reservoir, illustrating the efficiency and entropy changes associated with ideal heat engines.

💡Isothermal Process

An isothermal process is a thermodynamic process in which the temperature of the system remains constant. The script describes isothermal expansion and compression in the Carnot cycle, where heat is absorbed or released at a constant temperature, affecting the entropy change of the system.

💡Adiabatic Process

An adiabatic process is one in which no heat is exchanged with the surroundings. In the video script, adiabatic expansion and compression are mentioned in the context of the Carnot cycle, where the entropy change is zero because there is no heat transfer (q=0).

💡Reversible Process

A reversible process is one that can be reversed by an infinitesimally small change in conditions without any net change in the system or surroundings. The script concludes by stating that for an ideal heat engine like the Carnot engine, the entropy change for a reversible cyclic process is zero, emphasizing the theoretical efficiency of such engines.

Highlights

Calculating the entropy change of melting 15 grams of ice at zero degrees Celsius using the formula ΔS = Q/T.

Entropy change formula involves heat transferred divided by temperature, resulting in units of joules per kelvin.

Heat of fusion for ice is 334 joules per gram, and temperature in Kelvin is calculated as 273.15 K for 0°C.

Entropy change for ice melting process is found to be 18.34 joules per kelvin.

Calculating entropy change for heating 2 kg of water from 0°C to 100°C using an average temperature approach.

Specific heat capacity of water is 4184 joules per kilogram per Celsius, and temperature change formula is applied.

Average temperature for entropy change calculation is determined to be 323.15 K.

Entropy change for heating water is calculated as 2590 joules per kelvin using the average temperature method.

An alternative formula using natural logarithm for calculating entropy change during heating is introduced.

Entropy change for the same heating process is recalculated using the natural logarithm method, yielding 2612 joules per kelvin.

Demonstration of entropy change when mixing 3 kg of water at 70°C with 3 kg at 30°C, resulting in a final temperature of 50°C.

Calculation of entropy change for the hot water sample cooling down from 70°C to 50°C using the average temperature of 60°C.

Entropy change for the cold water sample heating up from 30°C to 50°C is calculated with an average temperature of 40°C.

Total entropy change for the mixing process is the sum of individual changes, resulting in a net increase of 48.1 joules per kelvin.

Derivation of the entropy change formula for heating or cooling a substance from an initial to a final temperature using integration.

Explanation of the relationship between entropy change and temperature change, with positive change indicating increased disorder.

Application of entropy change concepts to a Carnot engine with a heat energy flow of 12,000 joules into the engine and 6,000 joules out.

Calculation of the temperature of the hot reservoir in a Carnot engine using the efficiency ratio of heat energy flows.

Analysis of the entropy change for a Carnot cycle, emphasizing the zero net entropy change due to reversible processes.

Explanation of entropy changes during isothermal expansion and compression in the Carnot cycle, resulting in a net entropy change of zero.

Transcripts

Browse More Related Video

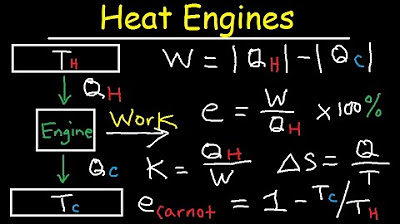
Carnot Heat Engines, Efficiency, Refrigerators, Pumps, Entropy, Thermodynamics - Second Law, Physics

24. The Second Law of Thermodynamics (cont.) and Entropy

Carnot Engine

Thermodynamic entropy definition clarification | Physics | Khan Academy

Carnot cycle and Carnot engine | Thermodynamics | Physics | Khan Academy

Entropy intuition | Thermodynamics | Physics | Khan Academy

Related Tags

Entropy Change Physics Thermodynamics Heat Transfer Ice Melting Heat Capacity Temperature Carnot Engine Cyclic Process Educational Content