Entropy intuition | Thermodynamics | Physics | Khan Academy

Khan Academy

17 Sept 200919:14

EducationalLearning

32 Likes 10 Comments

TLDRThis script explores the concept of entropy in thermodynamics, offering both the thermodynamic definition—relating entropy change to heat divided by temperature—and the statistical definition, which associates entropy with the logarithm of possible states. The second law of thermodynamics is introduced, emphasizing that entropy in the universe always increases. Examples like heat transfer between reservoirs and the function of air conditioners illustrate this principle. The script also clarifies misconceptions about entropy and disorder, explaining entropy as a measure of system states and information, rather than physical order.

Takeaways

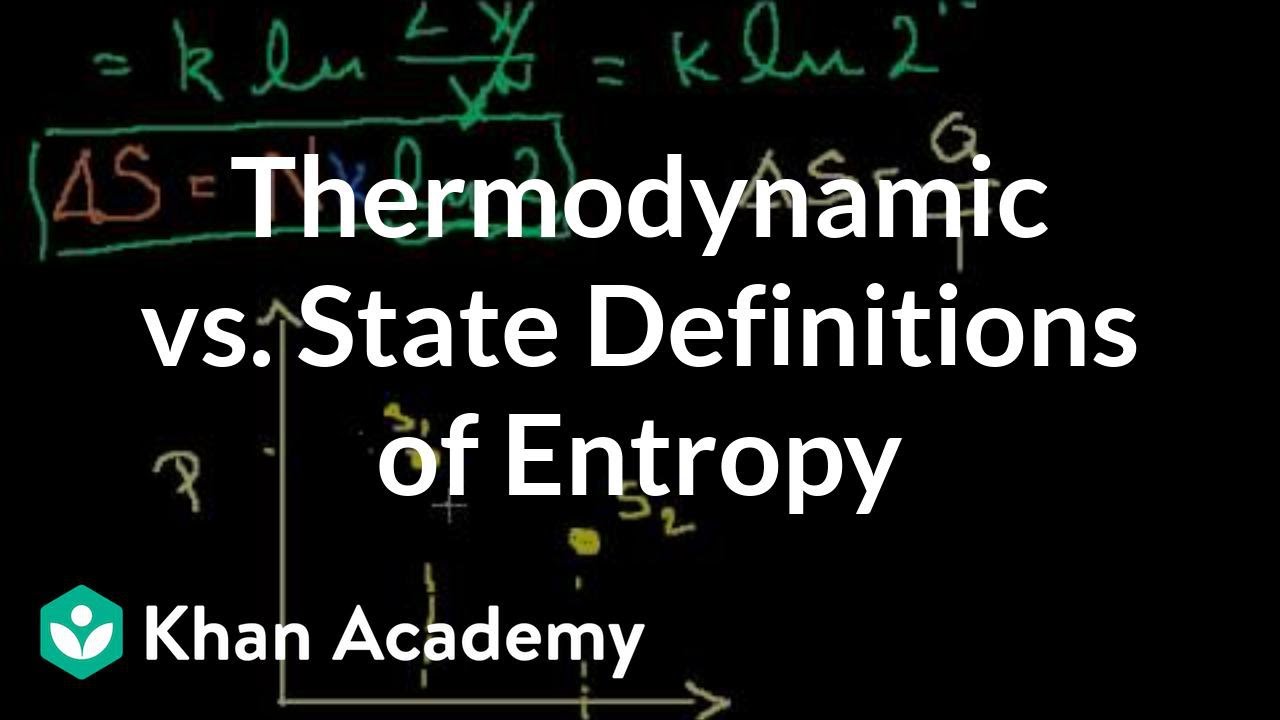
🔥 The concept of entropy is introduced with two definitions: thermodynamic and statistical. The thermodynamic definition states that the change in entropy is the heat added to a system divided by the temperature at which the heat is added.
📚 The statistical definition of entropy is described as being proportional to the natural log of the number of possible states a system can take on, assuming all states are equally probable.
🌡️ The second law of thermodynamics is introduced, stating that the change in entropy for the universe is always greater than or equal to zero, indicating an increase in entropy in any process.
🔄 The script uses the example of heat transfer between two reservoirs to illustrate the second law of thermodynamics, showing that heat naturally flows from a hotter to a colder substance.
🧊 The example of an air conditioner is used to clarify that while it appears to defy the second law by moving heat from a cold to a hot environment, it actually increases the overall entropy by doing work and expelling more heat.
🏠 The script challenges the common misconception that a clean room has less entropy than a dirty one, explaining that entropy is a macroscopic property and does not depend on the cleanliness of a room.
🌐 Entropy is further explained as a measure of disorder at the microscopic level, where the disorder increases when molecules move in more random directions, leading to more possible states.
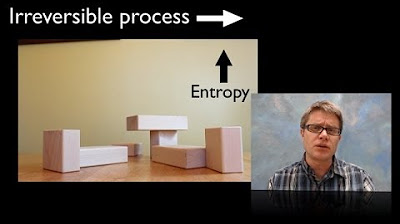
🔧 The script uses the example of a ball falling to the ground to illustrate how kinetic energy is transferred and how this process increases entropy by creating more random motion among molecules.
🤔 The script addresses the low probability of spontaneous reordering of molecules to reverse processes like a ball popping back up, emphasizing the statistical nature of entropy.
🌟 The concept of entropy is connected to information theory, explaining that entropy can be thought of as the amount of information needed to describe the exact state of a system.
🌌 The script concludes by emphasizing that entropy is a macroscopic property and is most useful when we do not know the exact details of what is happening at the microscopic level.

Q & A

What is entropy in thermodynamics?
-In thermodynamics, entropy is a measure of the disorder or randomness in a system. It is defined as the change in entropy being equal to the heat added to the system divided by the temperature at which the heat is added.
How is entropy related to the number of states a system can take on?
-Entropy is related to the number of states a system can take on through the statistical definition of entropy, which states that entropy is equal to a constant times the natural log of the number of states the system can take on, assuming all states are equally probable.
What is the second law of thermodynamics?
-The second law of thermodynamics states that the change in entropy for the universe when any process is undergone is always greater than or equal to zero. This means that the net effect of any process is an increase in the entropy of the universe.
Why does heat flow from a hotter substance to a colder substance?
-Heat flows from a hotter substance to a colder substance because this process increases the entropy of the universe, which is in accordance with the second law of thermodynamics.
How does the second law of thermodynamics apply to the example of two reservoirs in contact?
-In the example of two reservoirs in contact, the second law of thermodynamics predicts that heat will flow from the hotter reservoir to the colder one, leading to a net increase in entropy for the universe, as the change in entropy for the hotter reservoir is negative and for the colder one is positive, and their sum must be greater than zero.
What is the relationship between entropy and disorder?
-Entropy is often associated with disorder, but it is more accurately described as a measure of the number of possible microstates of a system. It is a macroscopic property that does not depend on the specific arrangement of particles but rather on the system's overall state.
Why does the example of a clean room becoming dirty not accurately represent an increase in entropy?
-The example of a clean room becoming dirty does not accurately represent an increase in entropy because entropy is a macroscopic property. Whether a room is clean or dirty, if the temperature and number of molecules remain the same, their entropy remains the same. The concept of disorder in this context does not directly translate to an increase in entropy.
How does an air conditioner seemingly defy the second law of thermodynamics?
-An air conditioner does not defy the second law of thermodynamics because it actively works by using a compressor or engine to transfer heat from the cold room to the outside. The total entropy change for the universe, including the heat expelled by the engine, is still positive.
What is the significance of entropy in understanding the behavior of molecules?
-Entropy helps in understanding the behavior of molecules by indicating the number of possible configurations or states that the molecules can take. It provides insight into the randomness and disorder of molecular motion, especially in systems where the exact state of each molecule is not known.
Why is it unlikely for a ball sitting on the ground to spontaneously pop back up?
-It is unlikely for a ball sitting on the ground to spontaneously pop back up because the probability of all the ground molecules randomly vibrating in just the right way to send the ball back up is infinitesimally small. This is consistent with the second law of thermodynamics, which states that the entropy of the universe tends to increase.

Outlines

00:00

🔥 Thermodynamics and Entropy

This paragraph introduces two definitions of entropy in the context of thermodynamics. The first definition relates entropy to the heat added to a system divided by the temperature at which the heat is added, highlighting the need for calculus when temperature changes. The second definition connects entropy to the natural log of the number of states a system can take on, under the assumption that all states are equally probable. The paragraph then transitions to the second law of thermodynamics, stating that the change in entropy for the universe is always greater than or equal to zero, indicating an increase in entropy with any process. Examples of heat transfer between two reservoirs are used to illustrate this law.

05:01

🌡️ Heat Transfer and the Second Law of Thermodynamics

The paragraph delves deeper into the second law of thermodynamics, using the example of heat transfer between a hot and cold reservoir. It explains how heat naturally flows from a hotter substance to a colder one, leading to temperature equalization. The discussion clarifies that this process aligns with the second law, as the net change in entropy for the universe is positive. The paragraph also addresses a common misconception involving air conditioners, explaining that the work done by the compressor and the heat expelled by the engine ensure that the total entropy of the universe still increases, thus not violating the second law.

10:02

🧹 Entropy and the Concept of Disorder

This paragraph challenges the common misconception that entropy is synonymous with disorder. It emphasizes that entropy is a macroscopic property that describes the number of possible microstates of a system, rather than the cleanliness or orderliness of a physical space. The paragraph uses the example of a clean room becoming dirty to illustrate that entropy does not increase in this scenario, as both states have the same number of molecules and temperature. It further explains that entropy increases when a system has more ways to be configured, such as a hot, clean room having more potential states than a cold, dirty one.

15:05

🎾 Entropy, Randomness, and the Second Law of Thermodynamics

The final paragraph explores the concept of entropy in terms of randomness and the second law of thermodynamics. It uses the example of a ball falling to the ground to explain how kinetic energy becomes more disordered upon impact, increasing entropy. The paragraph also discusses the low probability of a ball spontaneously rising due to random molecular vibrations, reinforcing the idea that entropy tends to increase over time. The discussion concludes by emphasizing that entropy is a macroscopic concept, applicable to the system as a whole rather than individual molecules, and is related to the amount of information needed to describe the system's exact state.

Mindmap

Keywords

💡Entropy

Entropy, denoted by 'S', is a measure of the number of possible microscopic configurations of a system, often associated with the disorder or randomness within that system. In the video, entropy is discussed in both thermodynamic and statistical terms. The thermodynamic definition relates entropy change to the heat added to a system divided by the temperature at which the heat is added, emphasizing the need for calculus when temperature changes. The statistical definition equates entropy to a constant times the natural log of the number of states a system can take on, under the assumption of equal probabilities for all states. The concept is central to understanding the second law of thermodynamics and is illustrated through examples like heat transfer between two reservoirs.

💡Thermodynamics

Thermodynamics is the study of the relationships between heat and other forms of energy, particularly in the context of work and temperature. The video script delves into the concept of entropy within the framework of thermodynamics, highlighting how entropy changes in a system as heat is added or removed. The second law of thermodynamics, a fundamental principle in this field, is discussed in relation to entropy, stating that the entropy of the universe always increases, reflecting the natural tendency towards disorder.

💡Second Law of Thermodynamics

The second law of thermodynamics is a fundamental principle stating that the total entropy of an isolated system can never decrease over time, and is always increasing. In the video, this law is used to explain why heat naturally flows from a hotter to a colder body, and why processes like air conditioning require external work to move heat against this natural flow. The law is illustrated through examples involving heat transfer between two reservoirs and the operation of an air conditioner.

💡Heat Transfer

Heat transfer is the process of thermal energy moving from one body or system to another. In the video, the concept is used to describe the natural flow of heat from a hotter to a colder body, as well as the role of heat transfer in the context of entropy. The script discusses how heat transfer between two reservoirs at different temperatures results in an increase in entropy, aligning with the second law of thermodynamics.

💡Reservoirs

In the context of the video, reservoirs refer to large bodies or systems capable of storing and exchanging heat. The script uses the example of two reservoirs, one hot and one cold, to illustrate how heat naturally flows from the hotter to the colder reservoir, leading to an increase in the system's entropy. This example is used to demonstrate the second law of thermodynamics in action.

💡Air Conditioner

An air conditioner is a device that cools a space by transferring heat from the interior to the exterior. In the video, the operation of an air conditioner is used to challenge the misconception that it might violate the second law of thermodynamics by moving heat from a colder to a hotter environment. The script clarifies that the air conditioner's compressor and engine do work, expelling additional heat, thus maintaining the overall increase in entropy.

💡Disorder

Disorder, in the context of the video, refers to the lack of order or predictability in the arrangement or movement of particles within a system. The script discusses how entropy is often equated with disorder, but cautions that this is a simplification. It explains that disorder in terms of molecular motion is what leads to an increase in entropy, as seen when a ball hits the ground and its kinetic energy is transferred to the ground molecules, causing them to move in more random directions.

💡Macro State

A macro state is a description of a system that does not specify the exact details of the individual particles' states, but rather provides an overview of the system's properties such as temperature, pressure, and volume. The video emphasizes that entropy is a property of the macro state, describing the system's overall disorder or the number of possible microscopic configurations. It contrasts this with the micro state, which would require detailed knowledge of each particle's state.

💡Micro State

A micro state is the specific arrangement of particles within a system, detailing each particle's position and motion. The video script explains that entropy is not concerned with the micro state of individual particles but rather with the macro state, which provides a general understanding of the system's properties. It uses the example of a room being clean or dirty to illustrate that these are different micro states of the same macro state, and therefore have the same entropy.

💡Information Entropy

Information entropy is a measure of the amount of uncertainty or information in a system, often used in the context of information theory. In the video, entropy is related to the amount of information needed to specify the exact state of a system. The script explains that entropy can be thought of as the amount of information required to describe the system in detail, emphasizing that higher entropy corresponds to a greater number of possible states and thus more uncertainty.

💡Potential Energy

Potential energy is the stored energy of an object due to its position in a force field, such as gravity. In the video, potential energy is discussed in the context of a ball falling to the ground. The script explains that as the ball falls, its potential energy is converted into kinetic energy, which is then transferred to the ground upon impact, contributing to the increase in entropy as the motion of the molecules becomes more random.

Highlights

Two definitions of entropy are presented: thermodynamic and statistical.

Thermodynamic definition involves heat added to a system divided by temperature.

Statistical definition of entropy is related to the natural log of possible states of a system.

The second law of thermodynamics states that the change in entropy for the universe is always greater than or equal to zero.

Heat naturally flows from a hotter to a colder substance, aligning with the second law of thermodynamics.

The concept of entropy increase is explored through the example of two reservoirs in contact.

Air conditioners and their role in entropy are discussed, showing they do not violate the second law.

The misconception of entropy being synonymous with disorder is clarified.

A clean room versus a dirty room example is used to explain the macro state of entropy.

The transition from a dirty cold room to a hot clean room is an example of entropy increase.

The ball and ground example illustrates how kinetic energy is transferred and entropy increases.

The probability of molecules spontaneously ordering to reverse entropy increase is extremely low.

Entropy is a measure of the number of possible microstates for a given macrostate.

The concept of information entropy and its relation to the amount of information needed to describe a system is introduced.

The video concludes by emphasizing the macroscopic nature of entropy and its practical implications.

Transcripts

Browse More Related Video

Reconciling thermodynamic and state definitions of entropy | Physics | Khan Academy

What is Entropy in Thermodynamics?

Second Law of Thermodynamics

Statistical Mechanics Lecture 3

Entropy

How Quantum Entanglement Creates Entropy