Wave Equation | Waves | Physics | FuseSchool
TLDRThis educational video script explores the fundamental properties of waves, including frequency, wavelength, and amplitude. It teaches viewers how to calculate wave speed using the formula speed = frequency × wavelength, emphasizing the importance of unit consistency. The script encourages practice with various examples and reminds learners to rearrange the formula to solve for frequency or wavelength when necessary. It concludes by inviting viewers to like, share, and ask questions for further assistance.
Takeaways
- 🌊 Waves have three main properties: frequency, wavelength, and amplitude.
- 📏 Frequency is the number of complete waves passing a point per second and is measured in hertz.
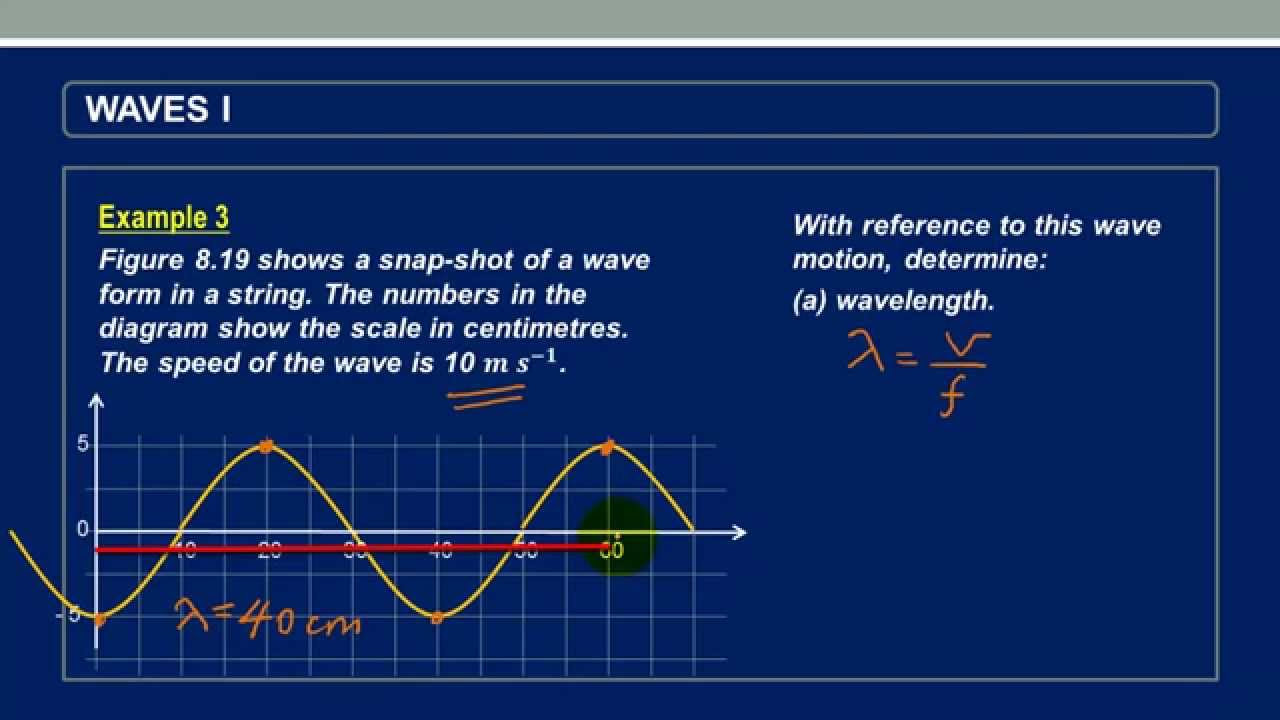
- 🌐 Wavelength is the distance between two consecutive points on a wave and can vary greatly in size.
- 🏗 Amplitude is the maximum distance of displacement from the equilibrium position of a wave.
- 🔢 The speed of a wave can be calculated using the formula: speed = frequency × wavelength.
- 📐 Units for calculating wave speed must be consistent, such as centimeters per second (cm/s).
- 🔄 The formula for wave speed can be rearranged to solve for frequency or wavelength.
- 🔢 Example given: A wave with a frequency of 4 Hz and a wavelength of 3 cm travels at 12 cm/s.
- 📝 It's important to pay attention to the units when performing calculations, as they affect the result.
- 📚 The script encourages viewers to practice by pausing the video and working through the examples.
- 👍 The video creators ask for likes, shares, and comments for further questions or help.
Q & A
What is the frequency of a wave?
-The frequency of a wave is the number of complete waves passing a point every second and is measured in hertz.
What is the definition of wavelength?
-Wavelength is the distance between two consecutive points on a wave.
What is amplitude in the context of waves?
-Amplitude is the distance of maximum displacement of a wave from its equilibrium position.
What is the relationship between frequency, wavelength, and wave speed?
-The speed of a wave is equal to its frequency multiplied by its wavelength, as represented by the equation speed = frequency × wavelength.
How can you calculate the speed of a wave if you know its frequency and wavelength?
-You can calculate the speed of a wave by multiplying its frequency by its wavelength, ensuring that the units are consistent, such as hertz for frequency and centimeters for wavelength.
What units are used to measure the speed of a wave?
-The speed of a wave is measured in units that reflect distance over time, such as centimeters per second or meters per second.
If a wave has a frequency of 4 hertz and a wavelength of 3 centimeters, what is its speed?
-The speed of the wave would be 12 centimeters per second, calculated by multiplying 4 hertz by 3 centimeters.
How do you rearrange the wave equation to find the frequency of a wave?
-To find the frequency, you rearrange the wave equation to frequency = speed ÷ wavelength.
What is the unit of frequency and what does it represent?
-The unit of frequency is hertz, which represents the number of cycles or events per second.
If a wave's wavelength is measured in meters, what should the unit of its speed be?
-If the wavelength is in meters, the speed of the wave should be in meters per second to maintain unit consistency.
How can you calculate the wavelength of a wave if you know its speed and frequency?
-You can calculate the wavelength by dividing the speed of the wave by its frequency, using the rearranged wave equation wavelength = speed ÷ frequency.
Outlines
🌊 Understanding Wave Properties
This paragraph introduces the fundamental properties of waves, including frequency, wavelength, and amplitude. Frequency is the number of complete waves passing a point per second, measured in hertz. Wavelength is the distance between two consecutive points on a wave, and amplitude is the maximum displacement from the wave's equilibrium position. These properties can vary greatly, from a thousandth of a millimeter to hundreds of meters. The paragraph also sets the stage for using these properties in equations to calculate the speed of waves.
🔢 Wave Speed Calculation
This section explains how to calculate the speed of a wave using the formula speed = frequency × wavelength. It provides an example with a wave having a frequency of 4 hertz and a wavelength of 3 centimeters, emphasizing the importance of units (hertz for frequency and centimeters for wavelength), which results in speed being measured in centimeters per second. The paragraph encourages viewers to practice by calculating the speed of a wave with given length and frequency, reminding them to pay attention to the units involved.
📏 Rearranging the Wave Equation
The paragraph discusses the ability to rearrange the wave equation to solve for either frequency or wavelength, depending on the information provided. It illustrates this by showing how to find the frequency when speed and wavelength are known, and how to determine the wavelength when given speed and frequency. The importance of correctly identifying and using units is reiterated, and viewers are encouraged to practice these calculations with provided examples.
📚 Conclusion and Engagement
The final paragraph wraps up the lesson on the wave equation and emphasizes the importance of understanding the units required for each question. It invites viewers to like, share, and comment on the video if they have further questions or need help with other topics. The paragraph serves as a call to action for viewer engagement and a summary of the educational content covered in the video.
Mindmap
Keywords
💡Frequency
💡Wavelength
💡Amplitude
💡Hertz
💡Speed
💡Wave equation
💡Centimeters per second
💡Meters per second
💡Rearranging formulas
💡Units
Highlights
Waves have a frequency measured in hertz, which is the number of complete waves passing a point every second.
Wavelength is the distance between two consecutive points on a wave.
Amplitude is the distance of maximum displacement in a wave.
Frequency, wavelength, and amplitude can range from a thousandth of a millimeter to hundreds of meters.
The wave equation connects frequency, wavelength, and speed: speed equals frequency times wavelength.
To calculate the speed of a wave, multiply the frequency by the wavelength.
Example calculation: A wave with a frequency of 4 hertz and a wavelength of 3 centimeters has a speed of 12 centimeters per second.
Speed is measured in units like centimeters per second, depending on the units of frequency and wavelength.
Example: A wave with a length of 4 centimeters and a frequency of 10 hertz travels at 40 centimeters per second.
When calculating, always check the units to ensure consistency.
Rearranging the wave equation allows calculation of either frequency or wavelength if the other variables are known.
To find frequency: frequency equals speed divided by wavelength.
Understanding units is crucial: frequency is measured in hertz (per second).
Example: If two waves pass every second, the frequency is 2 hertz.
The video teaches the wave equation and emphasizes the importance of unit consistency in calculations.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: