Inferential Statistics – Sampling, Probability, and Inference (7-5)

Research By Design
23 Aug 201608:10
EducationalLearning
32 Likes 10 Comments

TLDRThis script delves into the realm of inferential statistics, emphasizing the use of sample means to infer population characteristics. It introduces the concepts of null and alternative hypotheses in hypothesis testing, explaining how experimental and control groups are used to determine if an intervention has an effect. The script illustrates this with the example of a drug's impact on blood pressure, highlighting the significance of chance variation and the potential for a treatment effect.

Takeaways
  • 🔍 Inferential statistics are used to determine the probability that a sample mean represents the population mean.
  • 🧬 Also known as null hypothesis testing, inferential statistics help establish if a sample or test outcome is representative of the population.
  • 📊 A difference between sample and population means could be due to chance or an effect from an experiment.
  • 🧪 Experiments typically involve an experimental group receiving a treatment and a control group that does not, both drawn from the same population.
  • 💡 If an intervention is ineffective, the sample means of both the experimental and control groups should remain the same as the population mean.
  • 🤔 Hypothesis testing involves suspecting an effect if the experimental group's sample mean differs from the control group's after an intervention.
  • 📝 A hypothesis is a testable prediction; it can be accepted or rejected based on evidence supporting or contradicting it.
  • 🎯 There are two types of hypotheses: the null hypothesis, which assumes no difference due to chance, and the alternative hypothesis, which assumes a difference due to an effect.
  • 📉 The null hypothesis states that the sample mean is the same as the population mean, while the alternative hypothesis states it is different.
  • 👨‍🔬 In practice, a large sample is often divided into groups rather than drawing multiple samples from the population for an experiment.
  • 💊 An example given is a drug's effect on blood pressure, where a significant change in the experimental group's mean suggests the drug had an effect.
Q & A

  • What is the main purpose of inferential statistics?

    -Inferential statistics allow us to determine the probability that a sample mean represents the population from which it was drawn, using probability to assess whether a sample or test outcome is representative of the population.

  • What are the two possible reasons for the sample mean differing from the population mean?

    -The sample mean may differ from the population mean because of chance, or because of an effect, such as an intervention or treatment that has influenced the sample.

  • What is the role of the experimental group in an experiment?

    -The experimental group is the group that receives the treatment or is tested in an experiment, allowing researchers to observe the effects of the intervention.

  • What is the purpose of the control group in an experiment?

    -The control group serves as a baseline, identical to the experimental group but not given the treatment, to compare outcomes and determine the effect of the intervention.

  • What is hypothesis testing and why is it important?

    -Hypothesis testing is the process of examining whether the sample mean differs significantly from the population mean, which helps in determining if an intervention has had an effect and is a form of testing a hypothesis about a real-world phenomenon.

  • What are the two types of experimental hypotheses?

    -The two types of experimental hypotheses are the null hypothesis, which states that there is no effect and any observed differences are due to chance, and the alternative hypothesis, which states that there is an effect and differences are not due to chance.

  • What does it mean to 'accept' or 'reject' a hypothesis in the context of hypothesis testing?

    -Accepting a hypothesis means that the evidence supports it as a good explanation, while rejecting a hypothesis means that the evidence contradicts it, indicating it is a poor explanation.

  • Why might the sample mean differ slightly from the population mean even if the sample is representative?

    -The sample mean may differ slightly from the population mean due to random variation or chance, which is expected in sampling.

  • How does the experimental group's sample mean change after the intervention in the provided script example?

    -In the script example, the experimental group's diastolic blood pressure sample mean decreases from 101 to 86 after the intervention, suggesting the drug had an effect in lowering blood pressure.

  • What does it mean if the experimental group's sample mean is significantly different from the control group's after an experiment?

    -If the experimental group's sample mean is significantly different from the control group's, it suggests that the intervention or treatment had an effect, causing a change in the experimental group that was not due to chance.

  • Why is it important to randomly assign participants to groups in an experiment?

    -Random assignment helps to ensure that both the experimental and control groups are similar and representative of the population, reducing the influence of confounding variables and increasing the validity of the experimental results.

Outlines
00:00
📊 Inferential Statistics and Hypothesis Testing

This paragraph introduces inferential statistics, which is the process of using sample data to make inferences about a population. It explains the concept of null hypothesis testing, where the null hypothesis assumes no difference between the sample and the population, while the alternative hypothesis suggests an effect or difference. The paragraph discusses the importance of experimental and control groups in hypothesis testing, emphasizing the role of random assignment to ensure both groups are comparable. It also touches on the process of accepting or rejecting hypotheses based on evidence.

05:01
🔍 Understanding Variation and Experimental Outcomes

The second paragraph delves into the interpretation of variation in experimental results. It uses the example of blood pressure to illustrate how slight differences between sample means and population means can be attributed to random variation. The paragraph explains the process of assigning samples to control and experimental groups and the importance of random assignment in experimental design. It discusses the expected outcomes of an experiment where a treatment is applied to the experimental group, and how significant changes in the experimental group's mean after treatment can suggest an effect of the treatment. The paragraph concludes by highlighting the difference between attributing changes to chance versus an actual effect from the intervention.

Mindmap
Keywords
💡Inferential statistics
Inferential statistics is a branch of statistics that deals with drawing conclusions from data. It allows us to make inferences about a population based on a sample. In the video, this concept is central to understanding how the probability of a sample mean representing the population is determined. The script uses inferential statistics to explain hypothesis testing, which is a method to determine if an observed difference between sample means is due to chance or an actual effect.
💡Null hypothesis
The null hypothesis is a fundamental concept in hypothesis testing. It is a statement of no effect or no difference. In the context of the video, the null hypothesis posits that the sample mean is the same as the population mean, suggesting that any observed differences are due to chance. It serves as a baseline against which alternative hypotheses are compared.
💡Alternative hypothesis
The alternative hypothesis is the counterpart to the null hypothesis and represents the research hypothesis that the investigator is testing. It is the statement that there is an effect or a difference. The video explains that if the sample mean differs from the population mean, the alternative hypothesis suggests that this difference is not due to chance but is an actual effect, such as the impact of a treatment.
💡Sample mean
The sample mean is the average value of a sample, which is a subset of a larger population. In the video, the sample mean is used to represent the population mean and is the basis for hypothesis testing. The script discusses how differences between the sample mean and the population mean can be attributed to chance or an effect.
💡Population mean
The population mean is the average of an entire population. In the video, the population mean is used as a reference to compare with the sample mean. The script explains that the expectation is for the sample mean to be the same as the population mean, barring any experimental intervention.
💡Experimental group
The experimental group is the subset of a sample that is subjected to a treatment or intervention in an experiment. In the video, the experimental group is given a new drug intended to lower blood pressure, and their response is compared to the control group to determine the drug's effect.
💡Control group
The control group is a standard against which the experimental group is compared. It is identical to the experimental group but does not receive the treatment. In the video, the control group's blood pressure serves as a baseline to measure the impact of the treatment on the experimental group.
💡Hypothesis testing
Hypothesis testing is a statistical method used to make decisions about hypotheses. The video describes it as a process where the null and alternative hypotheses are examined to determine if the observed differences are due to chance or an effect. It is a critical part of experimental design and analysis.
💡Effect
In the context of the video, an effect refers to a change or influence produced by an experimental intervention. The script discusses how if the sample mean of the experimental group differs significantly from the control group and the population mean, it suggests that the intervention had an effect, such as lowering blood pressure.
💡Random variation
Random variation is the natural fluctuation in data that occurs by chance. The video explains that even though the sample mean will always differ slightly from the population mean, this difference is typically due to random variation and not an effect, unless the variation is significant.
💡Blood pressure
Blood pressure is used as an example in the video to illustrate the application of inferential statistics and hypothesis testing. The script discusses diastolic and systolic blood pressure measurements and how they are used to evaluate the effectiveness of a treatment, such as a drug to lower blood pressure.
Highlights

Introduction of two new tools for statistical analysis - samples representing populations and simple probability.

Explanation of inferential statistics for determining the probability that a sample mean represents the population mean.

Definition of null hypothesis testing in inferential statistics to assess if a sample outcome is representative of the population.

Two possible reasons for sample mean differing from population mean: due to chance or an effect.

Description of experimental and control groups in an experiment, and their expected similarity in means.

Hypothesis testing as a method to determine if an intervention had an effect by comparing experimental and control group means.

Definition and purpose of a hypothesis in experimental research.

Process of accepting or rejecting a hypothesis based on supporting or contradicting evidence.

Introduction of the null hypothesis stating no difference between sample and population means.

Introduction of the alternative hypothesis proposing a difference due to an effect.

Explanation of how sample mean differences from population mean can indicate chance variation or an effect.

Example of a population with high blood pressure and the expected similarity of sample means to the population mean.

Illustration of how slight differences in sample means can be attributed to chance.

Procedure of assigning one sample as control and another as experimental group in a study.

Description of a drug intervention study design and its expected outcomes.

Interpretation of post-treatment differences in experimental and control group means as evidence of the drug's effect.

Final hypothesis that the experimental group's lower blood pressure indicates a shift to a population with normal blood pressure.

Transcripts

Browse More Related Video

Hypothesis testing: step-by-step, p-value, t-test for difference of two means - Statistics Help

One-tailed and two-tailed tests | Inferential statistics | Probability and Statistics | Khan Academy

Hypothesis Testing Explained | Statistics Tutorial | MarinStatsLectures

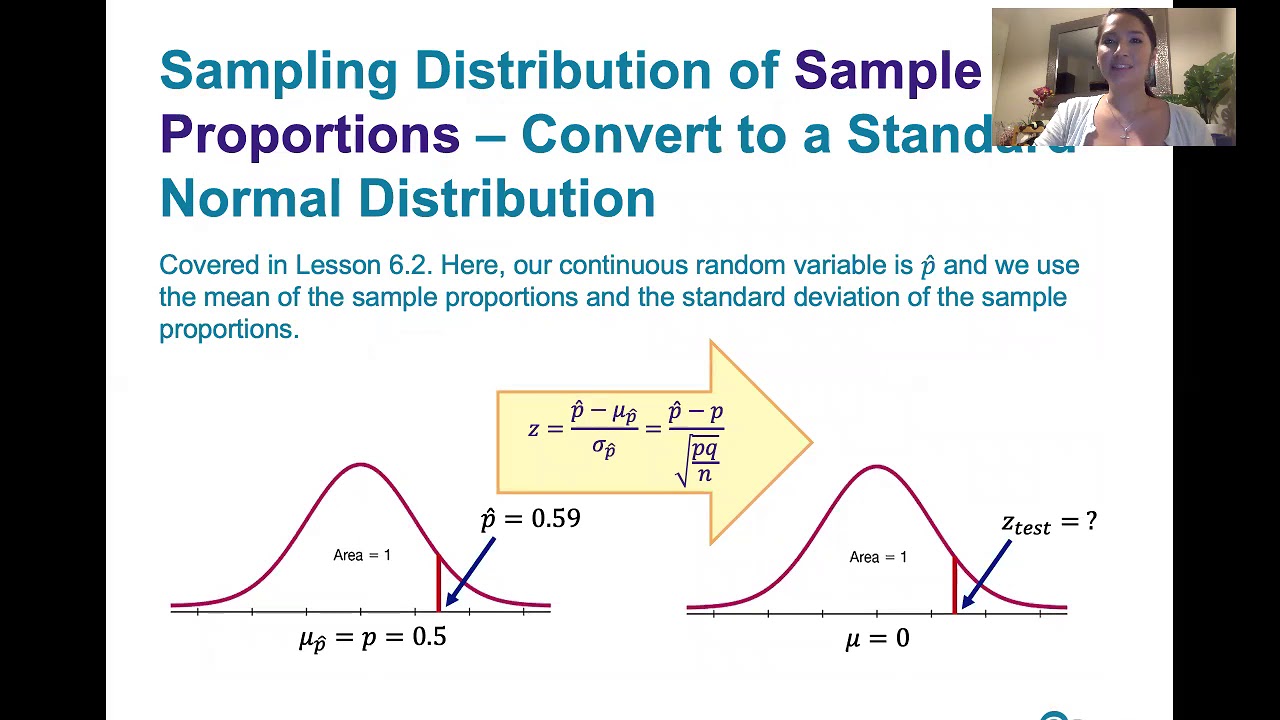
8.1.3 Basics of Hypothesis Testing - Computing and Interpreting Test Statistics

P-values and significance tests | AP Statistics | Khan Academy

How P-Values Help Us Test Hypotheses: Crash Course Statistics #21

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Inferential StatisticsHypothesis TestingSample MeanPopulation MeanExperimental GroupControl GroupStatistical AnalysisHealth InterventionBlood PressureDrug Efficacy