Precalc 5.3 Solving Trig Equations

SupernovaMath

11 Dec 201621:28

EducationalLearning

32 Likes 10 Comments

TLDRThis video script covers solving trigonometric equations, focusing on isolating trig functions and finding solutions within specified intervals. Techniques like the hand trick for reference angles and factoring trig functions are discussed, with examples including equations with sine, cosine, tangent, and their reciprocals.

Takeaways

📘 Find all solutions for each trig equation and then specify solutions within a given interval.
📈 For the equation 4sin²(θ) - 1 = 0, isolate sin(θ) and solve for θ.
🔢 When sin²(θ) = 1/4, take the square root to find sin(θ) = ±1/2 and find all angles where y-coordinate is ±1/2.
🧮 Use the hand trick to determine that sin(θ) = 1/2 at θ = π/6, 5π/6, 7π/6, and 11π/6.
🔄 Since there are infinite solutions, add 2πn to each angle to represent all solutions.
📐 For the equation 2cos(x) + √3 = 0, solve for cos(x) and determine the angles where cos(x) = -√3/2 in quadrants 2 and 3.
📝 Use the hand trick to find cos(x) = -√3/2 at x = 5π/6 and 7π/6, and add 2πn for all solutions.
📏 Solve equations involving multiple angles (like 3x and 2x) by isolating the trig function and using similar methods.
🔍 For equations involving cosecant, convert to sine for easier solving.
📏 Factor equations with both squared and regular trig functions to solve them.

Q & A

What is the first step in solving the trigonometric equation 4 sin^2(θ) - 1 = 0?
-The first step is to isolate sin(θ) by adding 1 to both sides, resulting in 4 sin^2(θ) = 1, and then dividing both sides by 4 to get sin^2(θ) = 1/4.
How do you find the angles for the equation sin^2(θ) = 1/4?
-You take the square root of both sides, resulting in sin(θ) = ±1/2. Then, you determine the angles in the specified quadrants where the sine function yields these values.
What is the 'hand trick' mentioned in the script, and how is it used to find reference angles in trigonometry?
-The 'hand trick' is a mnemonic device used to remember the signs of trigonometric functions in different quadrants. It helps in quickly determining the reference angles for given trigonometric values.
How many angles are there for the equation 4 sin^2(θ) - 1 = 0, and how are they represented in the solution?
-There are an infinite number of angles for this equation, as it represents a periodic function. The general solution is represented by adding 2πn to each of the four found angles, where n is an integer.
What is the process for solving the equation 2 cos(x) + √3 = 0?
-First, isolate cos(x) by subtracting √3 from both sides and then dividing by 2, resulting in cos(x) = -√3/2. Then, find the reference angle and the corresponding angles in the correct quadrants.
How does the script approach solving equations with cosecant (csc)?
-The script suggests converting cosecant to its reciprocal, which is sine, to simplify the problem. For example, if csc(θ) = √2, then sin(θ) = 1/√2.
What is the reference angle for the equation csc(θ) = √2?
-The reference angle for this equation is π/4, as the sine function equals √2/2 at π/4 radians.
How does the script handle equations with tangent (tan)?
-The script identifies the quadrants where tangent is negative (second and fourth quadrants) and finds the reference angle where the ratio of y to x is the given tangent value.
What is the reference angle for the equation tan(θ) = √3?
-The reference angle for this equation is π/3, as the tangent function equals √3 at π/3 radians.
How does the script solve trigonometric equations with squared functions, like sin^2(x)?
-The script suggests factoring the equation, treating the squared trigonometric function as a quadratic equation in terms of the trigonometric function.
What is the general solution for the equation sin^3(x) - sin(x) = 0 within the interval from 0 to 2π?
-The general solution involves finding the angles where sin(x) = 0, sin(x) = 1, and sin(x) = -1 within the specified interval, without extending the solution to include all periodic angles.

Outlines

00:00

📚 Introduction to Solving Trigonometric Equations

This paragraph introduces the topic of solving trigonometric equations, specifically focusing on finding solutions within a specified interval. The video begins with an example of solving the equation 4sin^2(θ) - 1 = 0. The process involves isolating sin(θ), taking the square root, and then determining the angles in the first and fourth quadrants where the sine function equals ±1/2. The hand trick is used to find reference angles, leading to the identification of four angles that satisfy the equation. The concept of adding 2πn to find an infinite number of solutions is also discussed.

05:01

🔍 Solving Trigonometric Equations with Reciprocals

The second paragraph delves into solving equations involving trigonometric functions and their reciprocals, such as cosecant. The example given is cosec(θ) = √2. The approach involves converting cosecant to sine and then finding the reference angle where the sine value equals √2/2. The hand trick is used again to identify the angles in the first and second quadrants that satisfy the equation. The solution set is extended to include all possible solutions by adding 2πn, as with the previous example.

10:01

📐 Using the Hand Trick for Tangent and Cosine

This paragraph discusses the use of the hand trick to solve equations involving tangent and cosine functions. The example provided is 2cos(x) + √3 = 0, which is solved by isolating cos(x) and finding the reference angle where the cosine value equals -√3/2. The hand trick helps identify the angles in the second and third quadrants. The solution set is expanded by adding 2πn to account for all possible solutions. The paragraph also touches on the importance of understanding the hand trick for solving trigonometric equations.

15:02

🔄 Factoring Trigonometric Equations

The fourth paragraph focuses on factoring trigonometric equations, using the example of 2sin^2(x) + 3sin(x) + 1 = 0. The process involves treating the equation as a quadratic in terms of sin(x), factoring it, and then solving for sin(x). The solutions are found by setting each factor equal to zero, leading to three equations. The angles that satisfy these equations are identified using the hand trick, and the solution set is presented for the given interval.

20:29

🔍 Solving Trigonometric Equations with Multiple Angles

This paragraph discusses solving trigonometric equations where the angle is multiplied by a constant, such as cos(3x) = 1/2. The solution involves isolating the cosine function, finding the reference angle, and then determining the angles that satisfy the equation. The hand trick is used to identify the angles in the first and fourth quadrants. The solution set is then expanded by adding 2πn to find all possible solutions. The paragraph also explains how to solve for the original angle by dividing the expressions by the constant multiplier.

📈 Solving Trigonometric Equations Within a Specified Interval

The final paragraph addresses solving trigonometric equations within a specific interval, such as from 0 to 2π. The example given is sin^3(x) = sin(x), which is factored to sin(x)(sin^2(x) - 1) = 0. The solutions are found by setting each factor equal to zero, identifying the angles where the sine function equals 0, 1, or -1. The solution set is presented for the specified interval, without extending it with 2πn, as the focus is on finding solutions within the given range.

Mindmap

Keywords

💡Trigonometric Equations

Trigonometric equations are mathematical equations that involve trigonometric functions, such as sine, cosine, and tangent. In the context of the video, these equations are the focus as the presenter demonstrates how to solve them. The video script provides examples of solving trigonometric equations like 4 sin^2(theta) - 1 = 0, which is foundational to understanding the theme of the video.

💡Solving for Angles

Solving for angles involves finding the values of angles (theta) that satisfy a given trigonometric equation. The video script illustrates this by showing the process of isolating the trigonometric function and then determining the angles that correspond to the solutions, such as finding angles for sin(theta) = 1/2.

💡Isolation

Isolation in the context of the video refers to the algebraic process of getting the trigonometric function by itself on one side of the equation. This is a common step in solving equations, as seen when the script describes isolating sin^2(theta) to solve the equation 4 sin^2(theta) - 1 = 0.

💡Square Root

The square root operation is used to find the values that, when squared, give the original number. In the video, taking the square root is a step in solving for sin(theta) after isolating sin^2(theta) = 1/4, leading to sin(theta) = ±1/2.

💡Reference Angle

A reference angle is the acute angle that a line makes with the x-axis in standard position. The video script uses the concept of reference angles to find the angles that satisfy the trigonometric equations, such as using pi/6 as a reference angle for sin(theta) = 1/2.

💡Quadrants

Quadrants refer to the four equal areas created by the intersection of the x and y axes in the Cartesian coordinate system. The script mentions the first, second, third, and fourth quadrants to explain where certain trigonometric functions are positive or negative, which is crucial for determining the correct angles.

💡Hand Trick

The 'hand trick' is a mnemonic used to remember the signs of trigonometric functions in different quadrants. The video script refers to this trick to help determine the signs of sine and cosine in the process of solving trigonometric equations.

💡Cosecant

Cosecant is the reciprocal of the sine function, denoted as csc(theta) = 1/sin(theta). The video script mentions cosecant when dealing with the equation involving csc(theta) = sqrt(2), and it explains how to convert it to sine to make solving easier.

💡Factoring

Factoring is the process of breaking down a polynomial into a product of its factors. In the video, the presenter factors the equation 2 sin^2(theta) + 3 sin(theta) + 1 = 0 by treating it as a quadratic in sin(theta), which is a key step in solving the equation.

💡Solving within an Interval

Solving within an interval means finding the solutions to a trigonometric equation that fall within a specified range, such as from 0 to 2 pi. The video script demonstrates this by solving the equation sin^3(x) = sin(x) within the given interval and listing the specific angles that are solutions.

Highlights

Introduction to solving trigonometric equations, focusing on finding solutions within specified intervals.

Method of isolating trigonometric functions to solve for angles, demonstrated with the equation 4 sin^2(θ) - 1 = 0.

Technique of adding 1 to both sides and dividing by 4 to simplify the equation sin^2(θ) = 1/4.

Explanation of taking the square root to find sin(θ) = ±1/2 and identifying the corresponding angles in different quadrants.

Use of the hand trick to find angles when the y-coordinate is 1/2, resulting in angles π/6, 5π/6, 7π/6, and 11π/6.

Concept of infinite solutions for trigonometric equations by adding 2πn to the base angles.

Solving the equation 2 cos(x) + √3 = 0 by isolating cos(x) and finding the reference angle π/6.

Identification of angles in quadrants 2 and 3 where the x-coordinate is negative.

Transformation of the equation cosec(θ) = √2 to sin(θ) = 1/√2 for easier angle finding.

Finding reference angles for y-coordinates positive in quadrants 1 and 2, specifically π/4.

Solution of the equation involving tangent, identifying the reference angle π/3 for negative tangent values.

Strategy for factoring trigonometric equations, demonstrated with 2 sin^2(x) + 3 sin(x) + 1 = 0.

Setting up and solving individual equations after factoring: sin(x) = -1, sin(x) = -1/2, and sin(x) = 1.

Solving trigonometric equations with multiple angles, such as cos(3x) = 1/2.

Adjusting solutions for equations with angles multiplied by constants, like dividing by 3 to solve for x.

Approach to solving equations with cosecant, converting to sine and finding angles with y-coordinates positive in specific quadrants.

Finalizing solutions by dividing by constants to solve for x, as shown with the equation cos(2x) - 2/√3 = 0.

Solving trigonometric equations within a specified interval [0, 2π], exemplified with sin^3(x) = sin(x).

Factoring and finding specific solutions within the interval without extending to infinite solutions.

Conclusion of the lesson on solving trigonometric equations, summarizing the methods and techniques covered.

Transcripts

Browse More Related Video

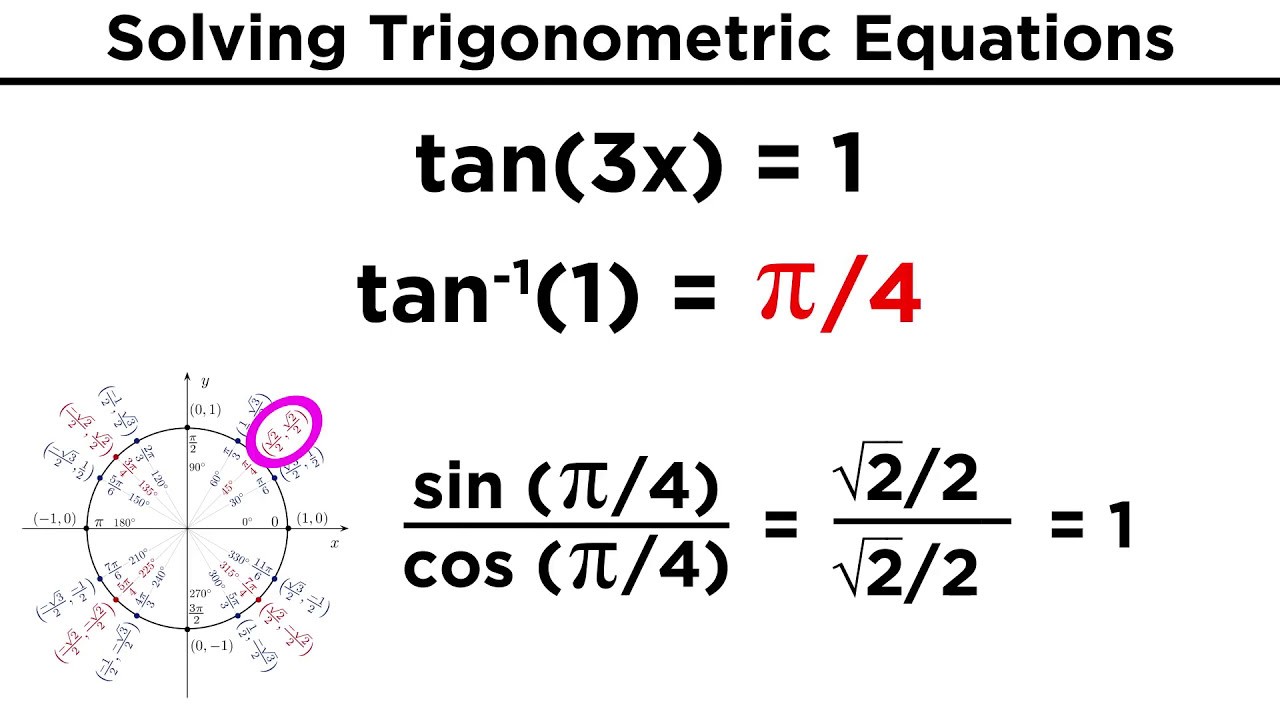
Solving Trigonometric Equations

Trigonometric Functions of Any Angle

Trigonometric Functions and the Unit Circle (Precalculus - Trigonometry 6)

Formulas for Trigonometric Functions: Sum/Difference, Double/Half-Angle, Prod-to-Sum/Sum-to-Prod

Trigonometric Functions: Sine, Cosine, Tangent, Cosecant, Secant, and Cotangent

Reciprocal Identities in Trigonometry (Precalculus - Trigonometry 9)

Related Tags

Trigonometry Hand Trick Solving Equations Math Tutorial Trig Functions Educational Content Interval Analysis Algebraic Techniques Sine Cosine Mathematics Learning