Find the mean and standard deviation for the set of data {3, 5, 6, 7, 9, 11, 22}. Conceptual

Ms Shaws Math Class
31 Dec 202004:07
EducationalLearning
32 Likes 10 Comments

TLDRIn this instructional video, the presenter guides viewers through calculating the mean and standard deviation of a data set. The mean is found by summing the numbers and dividing by the count, resulting in 9 for the given example. For standard deviation, each number is subtracted from the mean, squared, and averaged, yielding approximately 5.83. The process is demonstrated conceptually, with the presenter also suggesting the use of a calculator for efficiency. The video concludes with a brief mention of the sigma notation for standard deviation.

Takeaways
  • ๐Ÿ“ The video is a tutorial on calculating mean and standard deviation for a set of data.
  • ๐Ÿ”ข To find the mean, sum all the numbers in the dataset and divide by the count of numbers.
  • ๐Ÿ“ˆ The example given sums 3, 5, 6, 7, 9, 11, and 22, resulting in a mean of 9 when divided by 7.
  • ๐Ÿ“‰ For standard deviation, each number in the set is subtracted by the mean and squared.
  • ๐Ÿ” The squared differences are then summed up and divided by the number of data points.
  • ๐Ÿ“ The formula for standard deviation is demonstrated visually in the script.
  • ๐Ÿงฎ Squaring negative differences is important to ensure all values are positive before summing.
  • ๐Ÿ“Š The example calculates the squared differences and divides by 7 to find the standard deviation.
  • ๐Ÿ“ฒ Using a calculator's statistical function can simplify the process of finding standard deviation.
  • ๐Ÿ”ค The script mentions the sigma symbol (ฯƒ) as the signifier for standard deviation.
  • ๐Ÿ“š The video concludes with a reminder that the process is a conceptual guide, suggesting the use of a calculator for actual calculations.
Q & A

  • What is the purpose of the video script?

    -The purpose of the video script is to explain the process of finding the mean and standard deviation for a set of data, emphasizing that it's a conceptual explanation and typically done with a calculator.

  • What is the first step in finding the mean of a data set?

    -The first step in finding the mean is to add up all the numbers in the data set.

  • How many numbers are there in the data set provided in the script?

    -There are 7 numbers in the data set provided in the script.

  • What is the calculated mean of the data set mentioned in the script?

    -The calculated mean of the data set is 9.

  • What formula is used to calculate the standard deviation?

    -The formula for standard deviation involves taking each number, subtracting the mean, squaring the result, and then averaging those squared differences.

  • How many numbers are used in the calculation of the standard deviation in the script?

    -The same 7 numbers from the data set are used in the calculation of the standard deviation.

  • What is the approximate standard deviation calculated in the script?

    -The approximate standard deviation calculated in the script is 5.83.

  • What does the script suggest for simplifying the calculation of standard deviation?

    -The script suggests using a calculator to simplify the calculation of standard deviation, especially for a different list of numbers.

  • What is the symbol used to represent standard deviation?

    -The symbol used to represent standard deviation is the lowercase Greek letter sigma (ฯƒ).

  • How does the script describe the process of using a calculator to find standard deviation?

    -The script describes the process as going to the 'stat' function, entering the data set, and then using the 'edit' and 'calculate' options to find the standard deviation.

  • What is the final piece of advice given in the script?

    -The final piece of advice given in the script is to thank the viewers and wish them a nice day.

Outlines
00:00
๐Ÿ“Š Calculating Mean and Standard Deviation

The video script introduces the process of calculating the mean and standard deviation for a set of data. The presenter emphasizes that while the explanation is conceptual, a calculator is typically used for such calculations. The mean is calculated by summing all numbers in the dataset and dividing by the count of numbers (n=7 in this case), resulting in a mean of 9. For standard deviation, the presenter uses a formula involving squaring the difference of each number from the mean, summing these squares, and dividing by n. The result is an approximate standard deviation of 5.83. The script also briefly mentions how to use a calculator to find standard deviation using the 'stat' and 'edit' functions, and concludes with the sigma symbol (ฯƒ) representing standard deviation.

Mindmap
Keywords
๐Ÿ’กMean
The mean, often referred to as the average, is a measure of central tendency in statistics. It is calculated by summing all the numbers in a data set and then dividing by the count of those numbers. In the video, the mean is found by adding the numbers 3, 5, 6, 7, 9, 11, and 22, and then dividing by the total count of numbers, which is 7, resulting in a mean of 9. This is a fundamental concept in the video's explanation of calculating the mean of a data set.
๐Ÿ’กStandard Deviation
Standard deviation is a statistical measure that quantifies the amount of variation or dispersion in a set of values. It is calculated as the square root of the variance, which is the average of the squared differences from the mean. In the context of the video, the standard deviation is approximated to be 5.83 after performing a series of calculations involving subtracting the mean from each data point, squaring the results, and then averaging these squared differences.
๐Ÿ’กData Set
A data set is a collection of data points, which could be numbers, words, or any other form of information. In the video, the data set consists of the numbers 3, 5, 6, 7, 9, 11, and 22. The script discusses how to calculate the mean and standard deviation for this particular data set, which is essential for understanding the statistical concepts being taught.
๐Ÿ’กCalculator
A calculator is an electronic device used to perform arithmetic operations and calculations. The script mentions the use of a calculator for finding the mean and standard deviation, emphasizing that while the conceptual understanding is important, practical application often requires the use of tools like calculators for efficiency and accuracy.
๐Ÿ’กVariance
Variance is a measure that represents the average of the squared differences from the mean. It is used as an intermediate step in calculating the standard deviation. The video script describes the process of finding the variance by squaring the differences between each data point and the mean, and then averaging these squared values.
๐Ÿ’กSigma (ฮฃ)
Sigma, represented by the Greek letter 'ฮฃ', is used in mathematics and statistics to denote the sum of a sequence of terms. In the context of the video, sigma is used to represent the summation of squared differences from the mean, which is a step in calculating the standard deviation.
๐Ÿ’กConceptual Thought
The term 'conceptual thought' refers to the process of understanding and thinking about abstract ideas or concepts. The video script uses this term to introduce the idea that while the calculations can be done manually for a small data set, it's more practical to use a calculator for larger sets, emphasizing the importance of understanding the concepts before applying them.
๐Ÿ’กStatistical Measures
Statistical measures are mathematical calculations used to summarize and describe the features of a data set. The video focuses on two key statistical measures: the mean and the standard deviation. These measures provide insights into the central tendency and dispersion of the data, respectively.
๐Ÿ’กArithmetic Operations
Arithmetic operations are the basic mathematical operations of addition, subtraction, multiplication, and division. The script involves these operations in the process of calculating the mean and standard deviation, such as adding numbers to find the total and dividing to find the average.
๐Ÿ’กSquared
To square a number means to multiply it by itself. In the context of standard deviation, each difference between the data points and the mean is squared before being summed and averaged. The script mentions squaring the differences as part of the process to calculate the variance, which is then used to find the standard deviation.
๐Ÿ’กCalculator Functions
Calculator functions refer to the specific operations or features available on a calculator that can be used to perform various calculations. The video script briefly mentions using the 'stat' and 'edit' functions on a calculator to input and calculate statistical measures like standard deviation, showing an alternative method to manual calculation.
Highlights

Introduction to finding mean and standard deviation for a set of data.

Conceptual thought on using a calculator for calculating mean and standard deviation.

Step-by-step guide to finding the mean by adding numbers and dividing by the count.

Explanation of the mean calculation with a specific set of numbers.

Clarification that the total number of data points is 7.

Demonstration of dividing the sum by the number of data points to find the mean.

Result of the mean calculation, which is 9.

Introduction to the formula for calculating standard deviation.

Process of subtracting the mean from each data point and squaring the result.

Visual demonstration of squaring the differences in a step-by-step manner.

Explanation of the sum of squared differences divided by the number of data points.

Approximate result of standard deviation calculation as 5.83.

Reiteration of the mean value and the total number of data points.

Description of how to use a calculator for mean and standard deviation calculations.

Guidance on using the 'stat' and 'edit' functions on a calculator.

Illustration of the 'calculate' function on a calculator to find standard deviation.

Symbolic representation of standard deviation with the sigma sign.

Conclusion and sign-off with a wish for a nice day.

Transcripts

Browse More Related Video

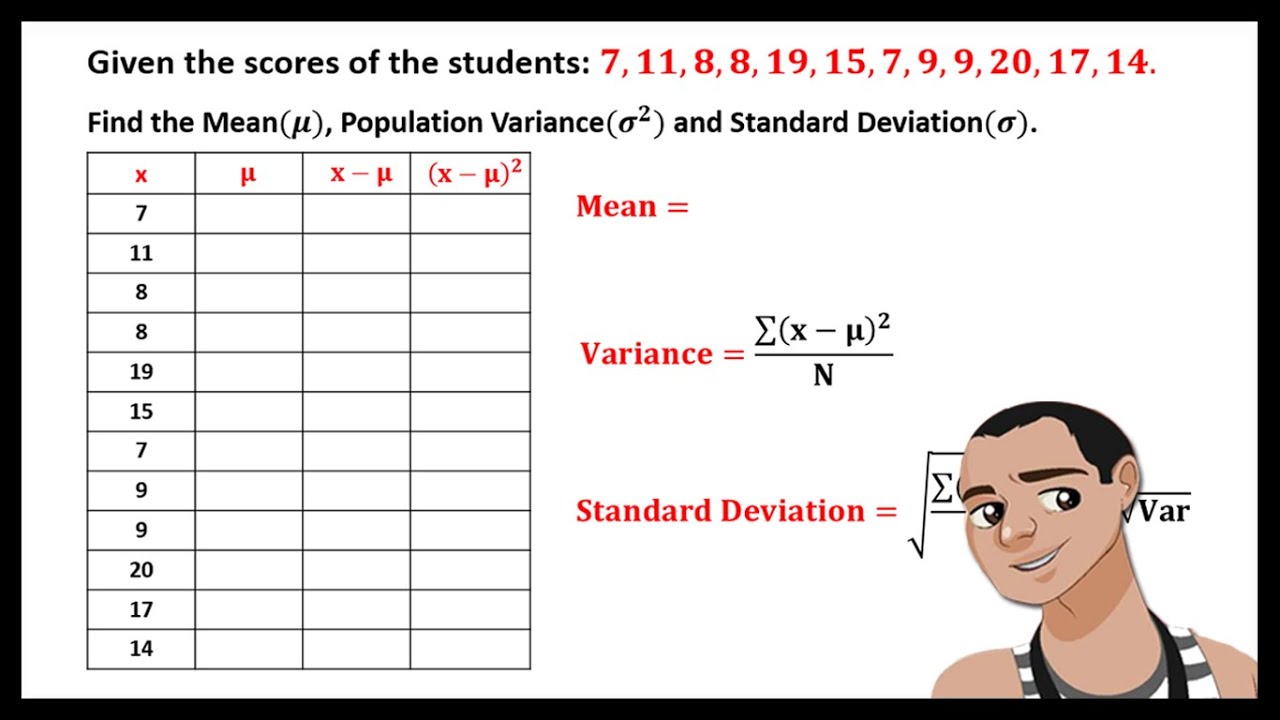
MEAN, VARIANCE, AND STANDARD DEVIATION FOR UNGROUPED DATA

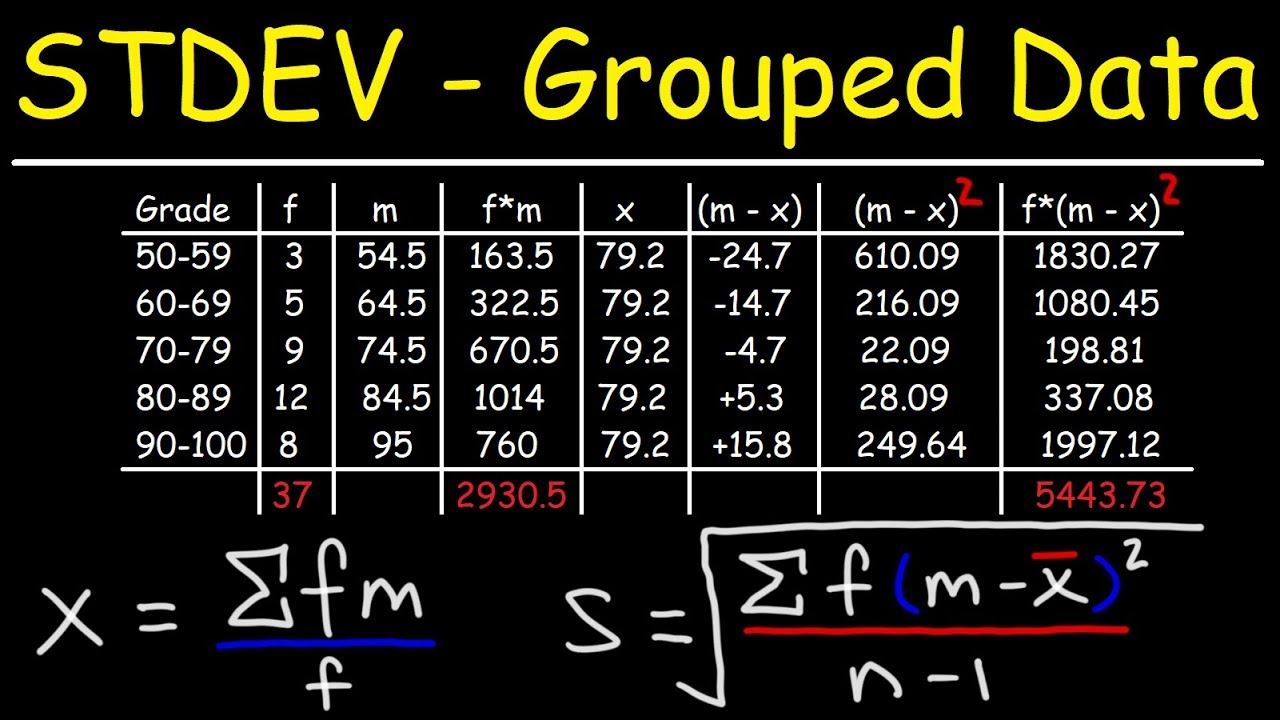
How To Calculate The Standard Deviation of Grouped Data

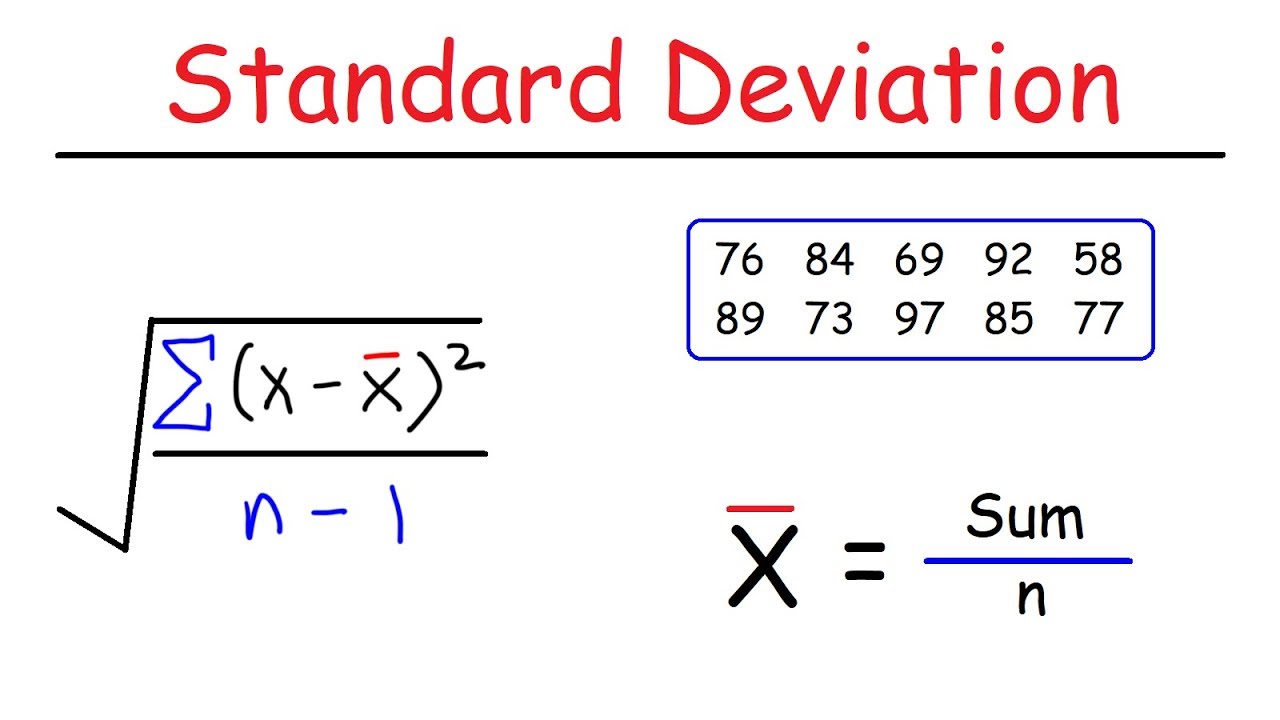
How To Calculate The Standard Deviation

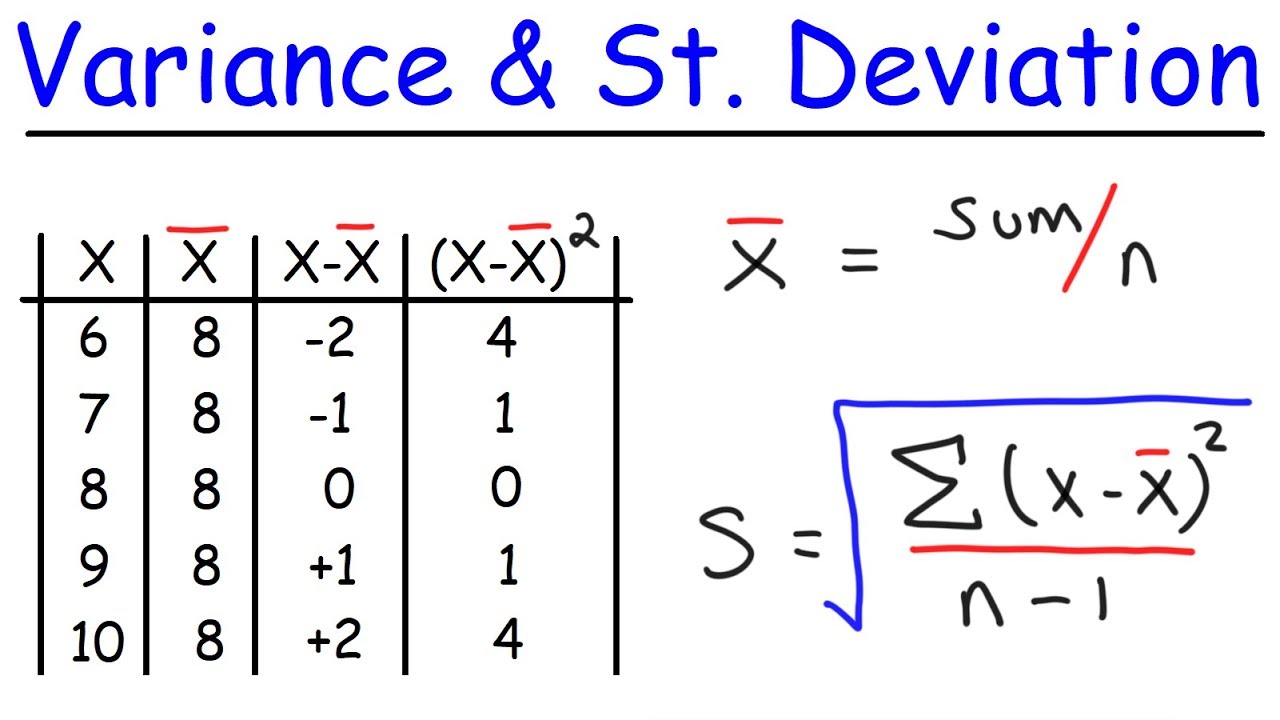
Variance and Standard Deviation With Microsoft Excel - Descriptive Statistics

Statistics: Standard deviation | Descriptive statistics | Probability and Statistics | Khan Academy

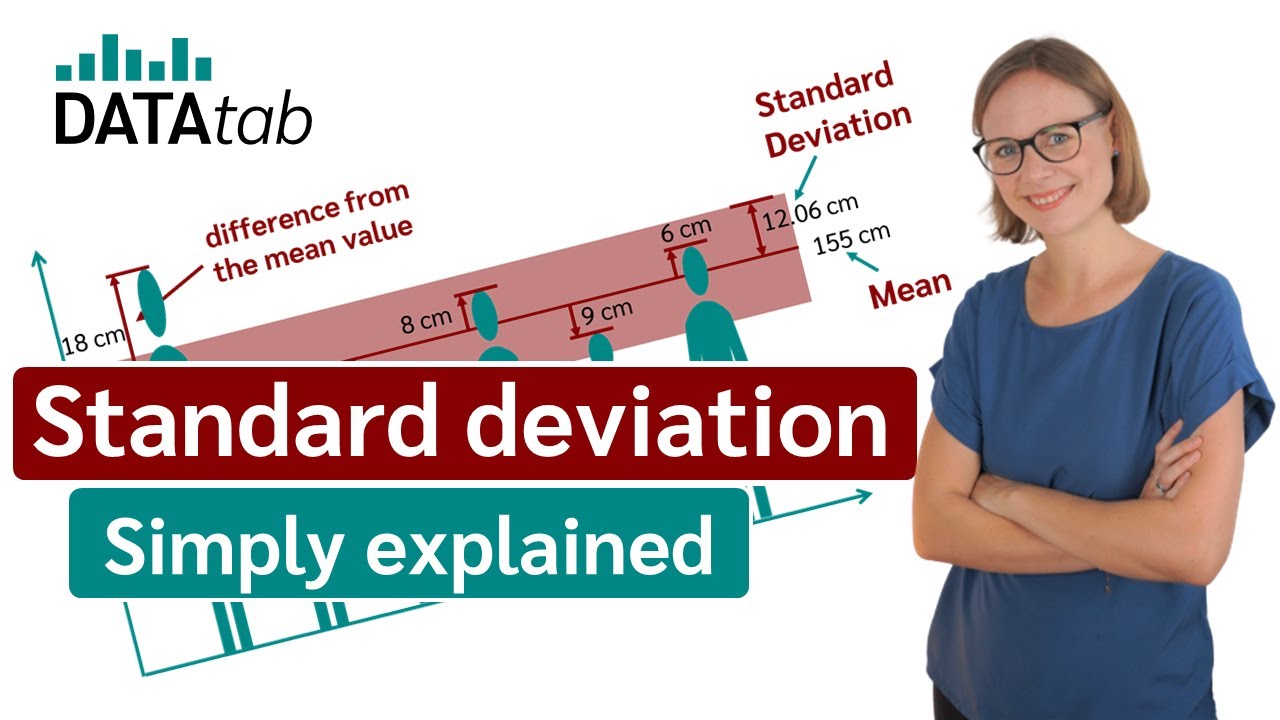
Standard deviation (simply explained)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Mean CalculationStandard DeviationStatistical GuideMath TutorialData AnalysisEducational ContentCalculator TipsStatistical FormulaNumerical ConceptsEducational Video