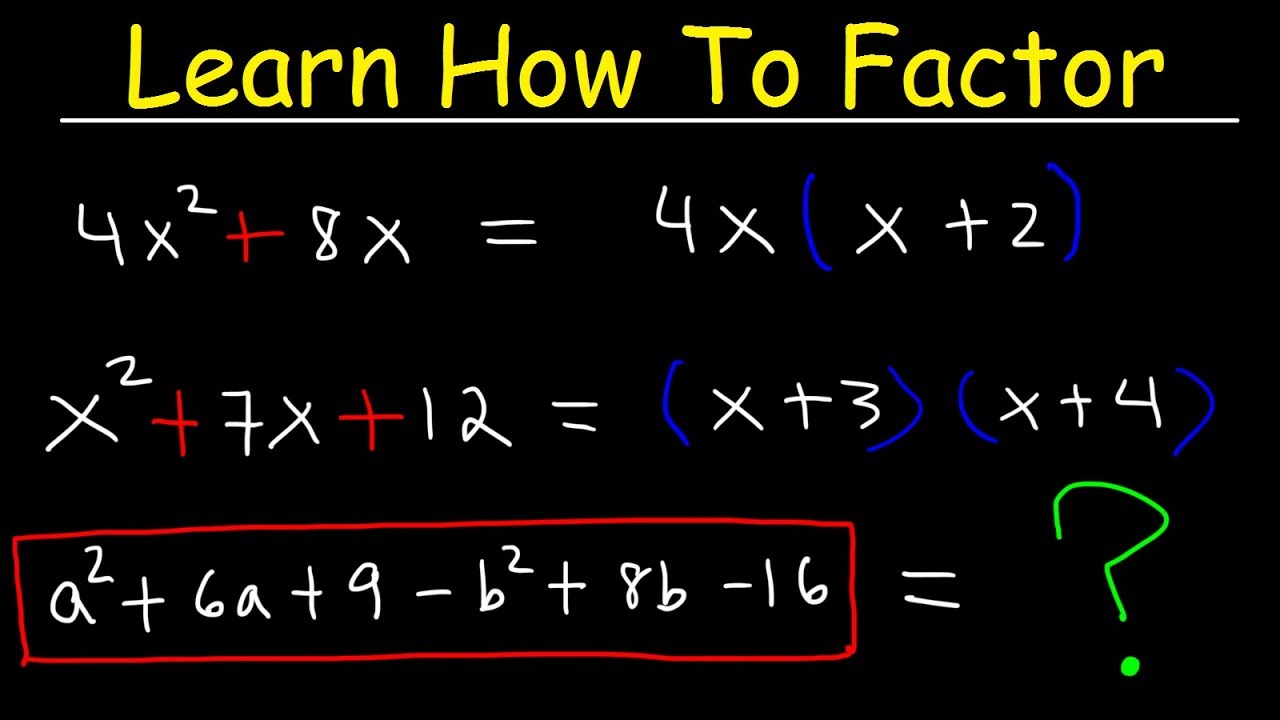
Learn the ac method for factoring and solving a quadratic equation

Brian McLogan

31 Jan 201504:59

EducationalLearning

32 Likes 10 Comments

TLDRIn this instructional transcript, the speaker guides students through factoring by grouping, a technique for solving algebraic equations. The process begins with identifying the factors of 45 and continues with rewriting the equation to facilitate factoring. The speaker emphasizes the importance of recognizing the larger absolute value factor when the sum is negative. The method involves grouping terms and factoring out the greatest common factor (GCF), eventually leading to a factored form that applies the zero product property to solve the equation.

Takeaways

📚 The lesson is about factoring by grouping, a technique previously discussed in class.
🔍 The first step is to calculate the product of 'a' and 'c', which in this case is -45, and then add it to get -12.
🤔 When stuck, students are advised to list all factors of 45, starting from 1 and working through to the number itself.
🔢 Factors of 45 are identified as 1, 3, 5, 9, and 15, with the understanding that the negative factor must be larger in absolute value to result in a negative sum.
📝 The equation is rewritten by separating the middle term into two values, aiming to factor by grouping.
🔄 The process involves rewriting the equation without altering its fundamental form, just presenting it differently for factoring.
📈 The teacher emphasizes the importance of recognizing the larger factor when adding a positive and a negative number to get a negative result.
📉 The equation is broken down into groups of terms that can be factored out, specifically looking for a common factor like 3x.
📌 The common factor (3x - 5) is factored out from the grouped terms, leading to a simplified expression.
📚 The zero product property is applied to solve the factored equation, though the solving steps are not shown in the script.
💡 The script encourages students to think through the process and understand the reasoning behind each step in the factoring method.

Q & A

What is the primary method discussed in the script for solving the given problem?
-The primary method discussed is factoring by grouping, which involves rewriting the middle term as two separate values and then factoring out the greatest common factor (GCF) from each group of terms.
What is the first step in the factoring process as described in the script?
-The first step is to calculate the product of 'a' and 'c', which in the script is given as 9 times 5, resulting in -45.
Why is it important to list all the factors of 45 when stuck during the factoring process?
-Listing all the factors of 45 helps to identify the pairs of factors that multiply to -45 and add up to -12, which are necessary for the factoring by grouping method.
What is the significance of the negative sign in the factors when the sum is negative?
-The negative sign indicates that the larger factor in absolute value should be negative, as the sum of a positive and a negative number resulting in a negative number implies the larger absolute value is from the negative number.
How does the script suggest to approach finding factors of 45?
-The script suggests starting with 45 times one and then working with numbers, testing divisibility by incrementing through the natural numbers until all factors are found.
What is the equation that the script is trying to factor?
-The equation is 9x^2 - 15x + 3x - 5 = 0, which is rewritten as 9x^2 - 12x - 5 = 0 after combining like terms.
What is the greatest common factor (GCF) of the first two terms in the equation?
-The GCF of the first two terms, 9x^2 and -15x, is 3x.
What is the common factor between the two expressions after factoring out the GCF from each group?
-The common factor between the two expressions (3x - 5) and (3x + 1) is (3x - 5).
What property is applied at the end of the script to solve the factored equation?
-The Zero Product Property is applied, which states that if the product of two factors is zero, then at least one of the factors must be zero.
Why is it necessary to factor out something, even if the terms don't have anything in common?
-Factoring out something, even a positive or negative one, is necessary to simplify the expression and to apply factoring techniques such as grouping.

Outlines

00:00

🧠 Problem Solving Approach in Algebra

The speaker explains the process of solving algebraic problems by rewriting the middle term into two separate values and factoring by grouping. This involves multiplying 'a' and 'c' (in this case, 9 and -5 to get -45), and finding factors that add up to -12. The speaker emphasizes the importance of writing down all factors of 45 and discusses which combinations meet the criteria. They highlight that if the sum is negative, the larger absolute value must be negative.

🔍 Finding Factors and Signs

The speaker provides a detailed method for finding factors of 45, including checking divisibility by progressively larger numbers. They explain the necessity of understanding that the sum of a positive and a negative number being negative means the negative number must be larger in absolute value. The correct factors are identified as -15 and 3.

🔄 Rewriting the Equation

The speaker demonstrates rewriting the original equation using the identified factors: 9x² - 15x + 3x - 5 = 0. They clarify that this step does not alter the equation but sets it up for the next process of factoring by grouping.

🔗 Factoring by Grouping

The process of factoring by grouping is explained, where the equation is divided into two groups: (9x² - 15x) and (3x - 5). The speaker instructs on factoring out the greatest common factor (GCF) from each group. For the first group, 3x is factored out, leaving 3x(3x - 5), and for the second group, 1 is factored out, leaving 1(3x - 5).

🧩 Combining and Factoring Out Common Terms

The speaker shows how the common factor (3x - 5) is identified in both groups. This common factor is factored out, resulting in (3x - 5)(3x + 1) = 0. The zero product property is then applied to solve for the values of x, though the speaker does not go through the final steps of solving.

Mindmap

Keywords

💡Factor by grouping

Factor by grouping is a mathematical technique used to factor expressions by rewriting them into a form that allows common factors to be factored out. In the video, this method is applied to the expression 9x^2 - 15x + 3x - 5 by grouping the first two and last two terms separately, which then allows for the common factor of 3x to be factored out from the first group, demonstrating the technique's application in solving the given problem.

💡Factors

Factors are numbers that multiply together to give a product. In the context of the video, the instructor is discussing the factors of 45, which are the numbers that can be multiplied together to result in 45. The script mentions the process of finding factors by starting with 45 times one and working through numbers to find pairs that multiply to 45, which is essential in factoring the quadratic expression.

💡Greatest Common Factor (GCF)

The GCF is the largest number that divides two or more numbers without leaving a remainder. In the video's theme, the GCF is factored out from each group of terms in the expression. For example, 3x is the GCF for the first two terms (9x^2 and -15x), and factoring it out simplifies the expression, which is a crucial step in the factor by grouping process.

💡Quadratic expression

A quadratic expression is a polynomial of degree two, with the highest power of the variable being squared. In the video, the quadratic expression 9x^2 - 15x + 3x - 5 is the main focus, and the instructor demonstrates how to factor it using the technique of factor by grouping, which is a fundamental concept in algebra.

💡Zero product property

The zero product property states that if the product of two numbers is zero, then at least one of the numbers must be zero. In the video, after factoring the quadratic expression into (3x - 5)(3x + 1) = 0, the instructor refers to the zero product property to solve for x, which is a standard method for solving factored quadratic equations.

💡Absolute value

Absolute value is a mathematical concept that represents the distance of a number from zero on a number line, regardless of direction. In the script, the instructor uses the concept of absolute value to explain why the larger factor in a sum that results in a negative number must be the negative number with the greater absolute value, as in the example of negative five plus three.

💡Negative number

A negative number is any number that is less than zero. In the video, the instructor discusses the concept of negative numbers in the context of factoring, specifically noting that when adding a positive and a negative number to get a negative sum, the negative number must have a larger absolute value than the positive number.

💡Polynomial

A polynomial is an algebraic expression made up of variables and coefficients, that involves only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. The quadratic expression in the video is a specific type of polynomial, and the process of factoring it is central to the video's educational content.

💡Mindset

Mindset refers to the mental attitude or inclination towards a particular way of thinking or doing things. In the script, the instructor emphasizes the importance of mindset by encouraging students to write down all the factors of 45 even if they get stuck, which reflects a growth mindset and persistence in problem-solving.

💡Divisibility

Divisibility is the property of a number being evenly divisible by another number without leaving a remainder. In the video, the concept of divisibility is used to find the factors of 45, which is a step in the process of factoring the quadratic expression by grouping.

💡Rewrite

Rewrite in this context means to express the same mathematical idea in a different form. The instructor in the video rewrites the quadratic expression by separating terms and then factoring by grouping, which simplifies the process of finding the factors without changing the underlying mathematical relationship.

Highlights

Introduction of a problem-solving approach without using a box, but applying the same concept.

Emphasis on rewriting the middle term as two separate values for factoring.

Explanation of factoring by grouping, a previously discussed method in class.

Calculation of a times c resulting in negative 45 and adding to give negative 12.

Advice for students to list all factors of 45 when stuck, starting with 45 times one.

Demonstration of finding factors by testing divisibility starting from 2 up to 9.

Concept that the negative number should have a larger absolute value when adding to a positive number to get a negative result.

Rewriting the equation by separating terms and identifying factors that add up to negative 12.

Identification of factors that result in negative 45 and their sum to negative 12.

Rewriting the equation in a new format to prepare for factoring by grouping.

Application of the greatest common factor (GCF) in factoring the first two terms.

Factoring out a positive 1 from the last two terms due to no common factors.

Identification of a common factor (3x - 5) between the expressions.

Factoring out the common term to simplify the equation.

Introduction of the zero product property for solving the equation.

Final factored form of the equation: (3x - 5)(3x + 1) = 0.

Note on not showing the steps to solve the equation, implying it's left as an exercise for the students.

Transcripts

Browse More Related Video

Factoring Review

Factoring Trinomials & Polynomials, Basic Introduction - Algebra

Follow this tip when factoring by grouping

09 - Factor by Grouping in Algebra (Factoring Trinomials, Quadratics, Binomials & Polynomials)

How To Factor Polynomials The Easy Way!

factoring trinomials with "a" greater than 1