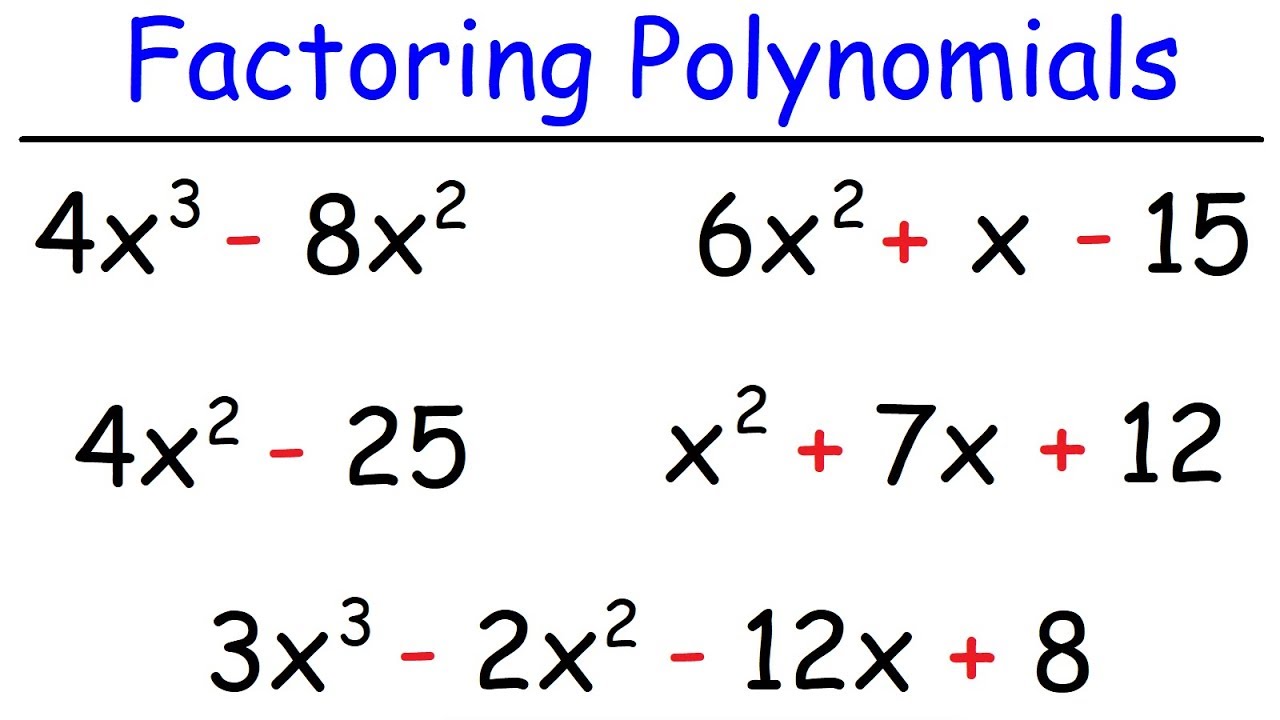
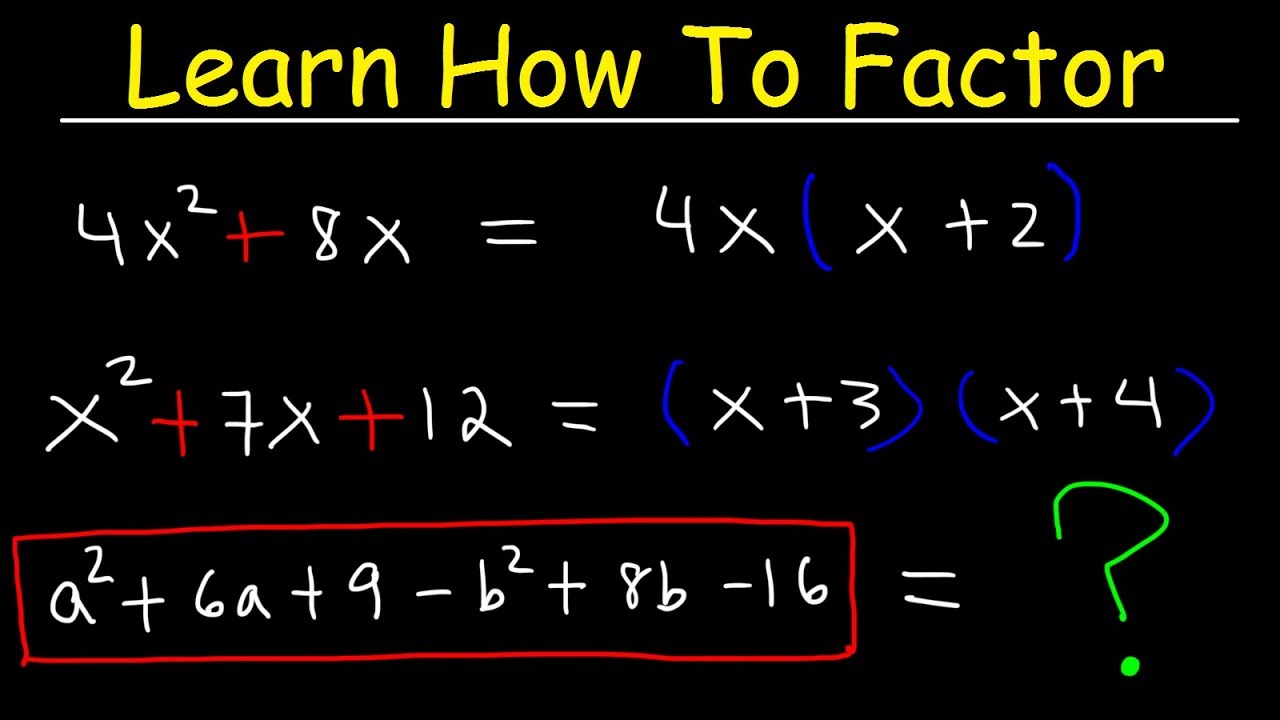
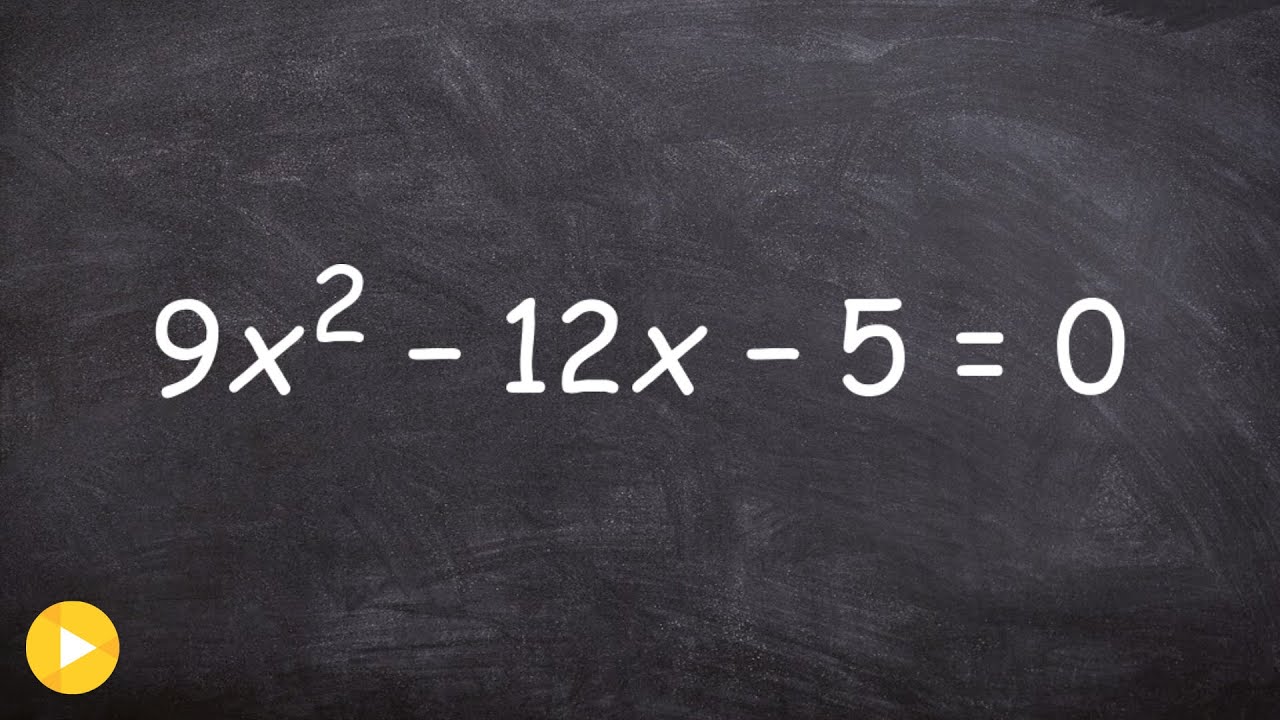09 - Factor by Grouping in Algebra (Factoring Trinomials, Quadratics, Binomials & Polynomials)
TLDRThis algebra lesson focuses on factoring by grouping, a technique to simplify expressions by identifying common factors within terms. The instructor guides through various examples, starting with simple cases and progressing to more complex scenarios where terms need rearrangement or manipulation to reveal commonalities. The goal is to practice recognizing patterns and creatively applying algebraic rules to factor expressions efficiently, ultimately preparing students to solve equations involving these expressions.
Takeaways
- 📚 The lesson focuses on 'factoring by grouping', a method within algebra for simplifying expressions by identifying common factors.
- 🔍 The process involves looking for common terms across different parts of an expression and factoring them out to simplify the equation.
- 📝 In the example of x * y + 2 * y, the common factor y is factored out, demonstrating the basic principle of the method.
- 🔄 Factoring by grouping often requires 'zooming out' to identify larger terms that are common to multiple parts of the expression.
- 💡 The concept is illustrated with the expression x * (y - 3) + 2 * (y - 3), where y - 3 is the common term factored out.
- 📉 When faced with expressions that don't immediately suggest a common factor, like x * y - 3 + 2 * 3 - y, algebraic manipulation can transform the expression into a form that allows for factoring.
- 🔄 An example of this is changing 3 - y to - (y - 3) to reveal a common factor of y - 3 that can be factored out.
- 🔑 The 'secret' of algebra, and indeed much of mathematics, is to use known rules to manipulate expressions into a form that allows for further simplification.
- 📈 The lesson emphasizes the importance of step-by-step problem-solving, where each transformation must be algebraically valid to ensure the solution is correct.
- 📝 The script provides multiple examples to illustrate the process, including the expression P * Q - 2 * Q + 2 * P - 4, which is broken down into smaller parts for factoring.
- 🧩 The final takeaway is that factoring by grouping is about identifying and extracting larger subsets of terms from an expression, which can be a challenging but essential skill in algebra.
Q & A
What is the main topic of this algebra lesson?
-The main topic of this algebra lesson is factoring by grouping, which involves looking for common factors in expressions and simplifying them by pulling out those common factors.
What is the first example given in the script to demonstrate factoring by grouping?
-The first example given is the expression x*y + 2*y, where the common factor y is pulled out, resulting in y(x + 2).
How does the script introduce the concept of looking for larger common terms in factoring by grouping?
-The script introduces the concept by modifying the first example to x*y - 3 + 2*y - 3, where instead of a single variable, the larger term (y - 3) is common to both terms and is factored out.
What is the significance of the expression x*y - 3 + 2*y - 3 in the script?
-This expression is significant because it shows that factoring by grouping can involve identifying and factoring out larger terms that are common to multiple terms in the expression.
How does the script explain the process of factoring out a common term in an expression?
-The script explains the process by first identifying the common term, then factoring it out by placing it outside the parentheses and determining what terms need to be inside the parentheses to maintain the equality.
What is the purpose of rearranging terms in the factoring by grouping process?
-Rearranging terms can help to identify common factors more easily and to group terms in a way that allows for the factoring out of larger common terms, which can simplify the expression.
Why does the script emphasize the importance of following algebraic rules in the factoring process?
-The script emphasizes the importance of following algebraic rules to ensure that each step in the factoring process is mathematically valid and to build a 'bulletproof' chain of steps leading to the correct answer.
What is the role of the distributive property (FOIL) in the script's explanation of factoring by grouping?
-The distributive property is used to show how terms can be multiplied out to verify that the factored form is equivalent to the original expression, thus confirming the correctness of the factoring process.
How does the script use the example of P*Q - 2*Q + 2*P - 4 to illustrate the factoring by grouping process?
-The script breaks down the expression by first factoring out Q from the first two terms and 2 from the last two terms, then recognizing the common factor (P - 2) and factoring it out to arrive at the final factored form.
What is the final expression given in the script to demonstrate the completion of the factoring by grouping process?
-The final expression given is (P - 2)(Q + 2), which is the result of factoring by grouping the original expression P*Q - 2*Q + 2*P - 4.
What is the script's advice for students who find the factoring by grouping process challenging?
-The script advises students to take the process step by step, using their knowledge of algebraic rules to manipulate the expression into a form that allows for the factoring out of common terms.
Outlines
📚 Introduction to Factoring by Grouping
This paragraph introduces the concept of factoring by grouping, a method within algebra for simplifying expressions. The instructor explains that this technique involves identifying common factors in different terms of an expression and pulling them out to simplify the problem. The example given is x*y + 2*y, which factors to y(x+2). The paragraph emphasizes the need for creativity in identifying common factors and sets the stage for more complex examples to follow.
🔍 Factoring by Grouping: Identifying Common Terms
The second paragraph delves deeper into factoring by grouping, illustrating the process with the expression x*y - 3 + 2*y - 3. The instructor shows how to recognize the common term (y - 3) in both pairs of terms and factor it out, resulting in (y - 3)(x + 2). The explanation highlights the importance of looking for larger common terms across the expression and using algebraic manipulation to make terms factorable. It also touches on the idea of 'zooming out' to see the bigger picture when common factors are not immediately apparent.
🎯 Advanced Factoring Techniques: Manipulating Terms
This paragraph discusses an advanced aspect of factoring by grouping, where the expression x*y - 3 + 2*3 - y is manipulated to make it factorable. The instructor demonstrates how to use algebraic rules to transform the expression into a form that reveals a common factor, y - 3, which can then be factored out. The process involves factoring out a negative one to change the signs within the terms and rearranging the terms to make the common factor more evident. The summary emphasizes the importance of step-by-step problem-solving and ensuring each algebraic move is valid.
🧩 Factoring by Grouping: Breaking Down Complex Expressions
The fourth paragraph presents a more complex expression, P*Q - 2*Q + 2*P - 4, and guides the learner through the process of factoring by grouping. The instructor first factors out Q from the first two terms and 2 from the last two terms, then recognizes a common factor (P - 2) that can be pulled out from the entire expression, resulting in (P - 2)(Q + 2). The explanation shows how to break down a complex expression into smaller, more manageable parts and how to look for patterns that can be factored out. It reinforces the idea that factoring is about simplifying expressions to solve equations more easily.
Mindmap
Keywords
💡Factoring
💡Common Factors
💡Grouping
💡Algebraic Manipulation
💡Special Cases
💡Distributive Property
💡FOIL
💡Rearrangement
💡Equivalence
💡Simplification
💡Pattern Recognition
Highlights
Introduction to the concept of factoring by grouping, emphasizing the importance of looking for common factors in algebraic expressions.
Explanation of the basic factoring process, including pulling out common items from parentheses.
Discussion of special cases in factoring, such as the difference of two squares and perfect square trinomials.
Illustration of factoring by grouping with a simple example involving x, y, and constants.
Demonstration of how to identify and factor out the common term (y - 3) from a more complex expression.
The idea of 'zooming out' to see commonalities between terms for factoring by grouping.
Use of parentheses to clarify the process of factoring out common terms.
The significance of multiplication distribution (FOIL) in understanding the equivalence of factored and expanded forms.
Introduction of the concept of transforming terms using algebraic rules to facilitate factoring.
Example of changing the order of addition within an expression to make factoring possible.
Technique of factoring out a negative one to change the sign of terms inside parentheses for easier factoring.
Explanation of how to manipulate algebraic expressions to create common terms for factoring.
Step-by-step walkthrough of a complex factoring problem involving terms with variables and constants.
The importance of ensuring each algebraic manipulation step is legal to maintain the integrity of the solution.
Final summary of the factoring by grouping process, emphasizing the need for creativity and step-by-step problem-solving.
Preview of upcoming lessons on special cases of factoring and general trinomials.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: