5.1.6 Discrete Probability Distributions - Expected Value and Decision Theory

Sasha Townsend - Tulsa
15 Oct 202011:52
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the concept of expected value in decision-making, primarily using gambling as an example. It explains expected value as the average outcome of infinite trials and contrasts it with the unpredictability of single events. The script delves into calculating expected values for roulette and craps, revealing that despite the allure of higher potential winnings in roulette, craps has a lower expected loss per game. It emphasizes the importance of expected value in making informed, long-term decisions, even though it doesn't predict individual outcomes.

Takeaways
  • 🎓 The concept of expected value is discussed as the theoretical mean value of a random variable over infinitely many trials.
  • 📊 Expected value is calculated by multiplying each outcome by its probability and summing these products, representing the long-term average outcome.
  • 💡 Expected value provides insight into the average result of a process repeated indefinitely, not the outcome of a single event.
  • 🎰 The script uses gambling as an example to illustrate the application of expected value in decision-making.
  • 👀 It's important to consider both the probabilities of winning and losing when calculating the expected value of a bet.
  • 🔢 In roulette, the expected value is calculated considering the 38 slots, with one winning scenario and 37 losing scenarios.
  • 🎲 For craps, the probabilities of winning and losing are given, and the expected value is calculated based on these probabilities.
  • 🏁 The expected value for roulette is approximately a 26-cent loss per game, indicating the house's advantage.
  • 📉 The expected value for craps is about a 7-cent loss per game, suggesting a smaller disadvantage compared to roulette.
  • 🤔 The decision between playing roulette or craps should consider the long-term expected loss, not just the potential single win.
  • 💭 Even though the potential win in roulette is higher, the expected value analysis suggests that craps might be the better choice due to a lower expected loss.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the application of expected value in decision-making, particularly in the context of gambling.

  • What is the expected value of a discrete random variable?

    -The expected value E of a discrete random variable X is the theoretical mean value of infinitely many trials, calculated by multiplying each outcome by its corresponding probability and summing these products.

  • How does the expected value relate to the average outcome of a bet over many trials?

    -The expected value represents the average value one would expect if the betting procedure were repeated indefinitely, indicating the long-term average loss or gain per bet.

  • What does the expected value not tell us about a single bet?

    -The expected value does not predict the outcome of a single bet; it only provides information about the average outcome over a large number of trials.

  • What are the two gambling options discussed in the video?

    -The two gambling options discussed are betting on the number seven in roulette and betting on the pass line in craps.

  • What is the cost and potential win of betting on the number seven in roulette according to the video?

    -The cost of betting on the number seven in roulette is five dollars, and the potential win is 180 dollars.

  • What is the potential win of betting on the pass line in craps as presented in the video?

    -The potential win of betting on the pass line in craps is doubling the initial bet of five dollars, resulting in a ten-dollar win.

  • How many slots are on a roulette wheel, and what are the implications for the probability of winning on a single number?

    -A roulette wheel has 38 slots, with 37 ways to lose and one way to win on a single number, making the probability of winning 1 in 38.

  • What is the approximate expected value of playing roulette as calculated in the video?

    -The approximate expected value of playing roulette, as calculated in the video, is a loss of about 26 cents per game.

  • What is the approximate expected value of playing craps, based on the probabilities provided in the video?

    -The approximate expected value of playing craps, based on the given probabilities, is a loss of about seven cents per game.

  • Why might a person choose to play craps over roulette, despite the potential for a larger win in roulette?

    -A person might choose to play craps over roulette because, over a long period, the expected value of craps results in a smaller average loss per game (seven cents) compared to roulette (26 cents).

  • What is the significance of expected value in making sound decisions about gambling?

    -The significance of expected value in gambling decisions is that it provides a measure of the average long-term outcome, helping to identify which game, over many plays, is likely to result in the least loss.

Outlines
00:00
🎰 Understanding Expected Value in Gambling Decisions

This paragraph introduces the concept of expected value in the context of gambling, specifically betting. It explains that expected value (E) is the theoretical mean outcome of a random variable over an infinite number of trials, calculated by multiplying each outcome by its probability and summing these products. The paragraph emphasizes that expected value predicts the long-term average result of repeated gambling, not the outcome of a single bet. It uses the example of choosing between two bets in Las Vegas: betting on the number seven in roulette or betting on the pass line in craps, highlighting the importance of considering probabilities of winning and losing to determine the expected value of each game.

05:01
📊 Calculating Expected Value for Roulette and Craps

The second paragraph delves into the process of calculating the expected value for the two gambling options presented: roulette and craps. It provides a detailed explanation of how to compute expected value, using a table to illustrate the calculation for roulette, where the probabilities of winning and losing are applied to the potential gains and losses. The expected value for roulette is found to be a loss of approximately 26 cents per game. Similarly, the expected value for craps is calculated, revealing a loss of about 7 cents per game. The summary underscores the importance of expected value in making informed gambling decisions, despite its limitations in predicting single-game outcomes.

10:02
🤔 Decision Making Using Expected Value

The final paragraph discusses the application of expected value in decision-making, particularly in choosing between the two gambling options. It contrasts the intuitive appeal of the higher potential winnings in roulette with the lower expected loss in craps. The paragraph concludes that, over the long term, it is more favorable to play craps due to its lower expected loss per game, even though the potential winnings are less than in roulette. This illustrates the practical use of expected value in making sound, long-term decisions in gambling scenarios.

Mindmap
Keywords
💡Expected Value
Expected value is a fundamental concept in probability theory and statistics, representing the average outcome of a random variable over a large number of trials. In the context of the video, it is used to determine the long-term average loss or gain from a gamble, such as betting on roulette or craps. The script explains that the expected value is calculated by multiplying each outcome by its probability and summing these products, which gives an indication of the average result over an infinite number of trials.
💡Gambling
Gambling is the act of staking something of value on an event with an uncertain outcome, with the primary intent of winning additional money or material goods. The video script uses gambling as a practical application to illustrate the concept of expected value, discussing how to make decisions based on the expected outcomes of bets in games like roulette and craps.
💡Discrete Random Variable
A discrete random variable is a type of variable that can take on a countable number of distinct values, each with a specific probability. The script refers to this concept when explaining how to calculate the expected value, emphasizing that it applies to situations where outcomes can be clearly enumerated and assigned probabilities, such as the numbered slots on a roulette wheel.
💡Mean
The mean, often referred to as the average, is a measure of central tendency in statistics, calculated by summing all the values in a data set and then dividing by the number of values. In the video, the mean is related to the expected value as a way to conceptualize the long-term average outcome of repeated trials, such as repeated gambling bets.
💡Roulette
Roulette is a popular casino game where a small ball is spun around a wheel divided into numbered and colored pockets. The video script uses roulette as an example to demonstrate the calculation of expected value, highlighting the probabilities of winning and losing based on the number of slots on the wheel and the payout for a correct bet.
💡Craps
Craps is a dice game played on a table-top, where players wager on the outcome of the roll or a series of rolls. The script discusses craps as an alternative to roulette, comparing the expected value of betting on the pass line, which is a common bet where the player is hoping to roll a specific number before rolling a seven.
💡Probability
Probability is a measure of the likelihood that a particular event will occur, expressed as a number between 0 and 1. The video script discusses the importance of probability in calculating expected value, as it is used to weigh the outcomes of a gamble to determine the average expected result over many trials.
💡Bet
A bet is a wager or a stake with an uncertain outcome, typically associated with gambling. The video script frequently refers to placing bets, using this action as the basis for comparing the expected values of different gambling options and making informed decisions about which bet is more favorable in the long run.
💡Pass Line Bet
In the context of craps, a pass line bet is a wager made by players hoping that the shooter will roll a specific number before rolling a seven. The script uses this type of bet to illustrate the calculation of expected value in craps, comparing it to the expected value of betting on a single number in roulette.
💡Decision Theory
Decision theory is a study of how decisions are made, especially under conditions of uncertainty. The video script applies decision theory by using expected value to make informed choices between different gambling options, demonstrating how understanding the long-term outcomes can guide decision-making.
💡Net Gain
Net gain refers to the difference between the amount won and the amount bet in a gambling scenario. The script discusses net gain in the context of calculating expected value, emphasizing the importance of considering not just the winnings but also the initial bet when determining the average outcome of a gamble.
Highlights

Expected value is the theoretical mean value of a random variable over infinitely many trials.

Expected value is calculated by multiplying each outcome by its probability and summing these products.

Expected value represents the average outcome of a procedure repeated indefinitely.

The concept of expected value is applied to gambling to determine the average loss or win over many bets.

Expected value does not predict outcomes of individual bets but provides an average for repeated trials.

In gambling, expected value helps in making sound decisions by comparing potential losses or gains.

A scenario is presented where a gambler must choose between betting on roulette or craps in Las Vegas.

Roulette has a 1 in 38 chance of winning 180 dollars, with a cost of 5 dollars per bet.

Craps involves betting on the pass line with a probability of losing 251 out of 495, and winning 244 out of 495.

The expected value of roulette is calculated to be a loss of approximately 26 cents per game.

The expected value of craps is calculated to be a loss of about 7 cents per game.

Despite the higher potential win in roulette, craps is the better choice due to a lower expected loss.

The importance of understanding the probabilities of winning and losing in gambling is emphasized.

A detailed explanation of how to calculate expected value for roulette is provided.

A comparison of expected values for roulette and craps shows the practical application of decision theory.

The video concludes that even though craps may not seem as lucrative as roulette, it has a lower expected loss.

The video demonstrates the use of expected value in making informed decisions in the context of gambling.

Transcripts

Browse More Related Video

Mean (expected value) of a discrete random variable | AP Statistics | Khan Academy

Expected Value: E(X)

Law of large numbers | Probability and Statistics | Khan Academy

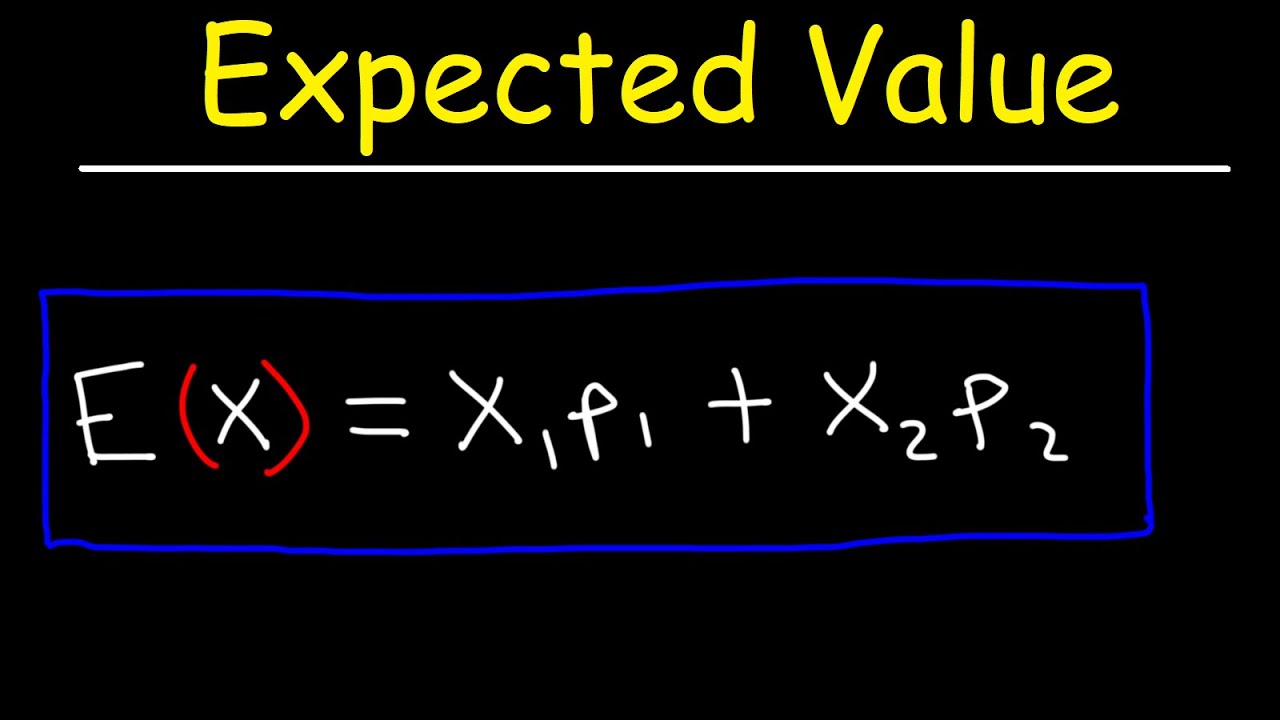
How To Calculate Expected Value

5.1.0 Discrete Probability Distributions - Lesson Overview and Learning Outcomes

Expected value of binomial distribution | Probability and Statistics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Expected ValueGambling DecisionsRoulette OddsCraps StrategyRisk AnalysisBetting AveragesGambling MathCasino GamesProbability TheoryDecision Theory