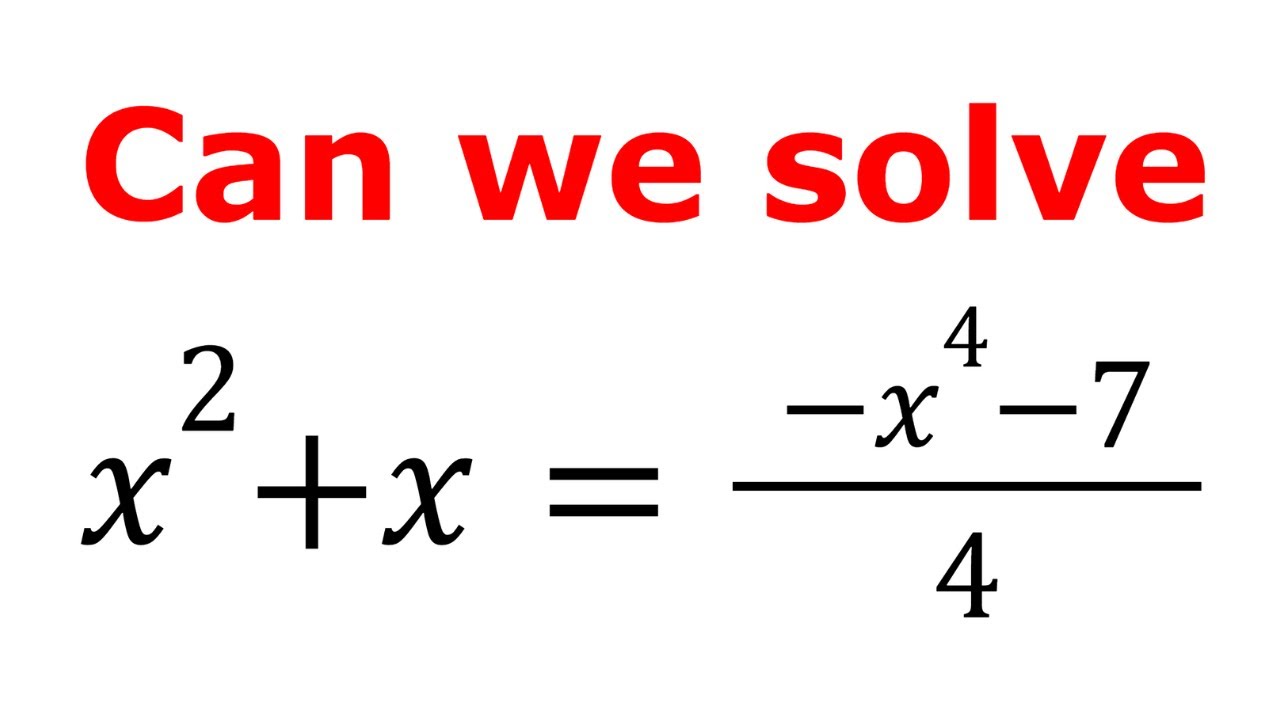EXTREME quintic equation! (very tiring)
TLDRIn this educational video, the presenter embarks on solving an extreme quintic equation, x^5 - 5x + 3 = 0, by attempting to factor it into a quadratic and a cubic. After realizing that simple factoring doesn't work, they decompose the equation into unknown coefficients and equate them to find a solution. Through a series of guesses and algebraic manipulations, they eventually determine the values of a, b, c, d, and e, leading to the factorization of the quintic equation. The presenter also solves for the real solutions using the quadratic formula and introduces the Lagrange Resolvent method for finding the cubic's solutions. The video concludes with a reminder that not all quintic equations are factorable and encourages viewers to engage with the content.
Takeaways
- 🧗♂️ The video discusses solving an extreme quintic equation, x^5 - 5x + 3 = 0, which is a high-degree polynomial equation.
- 🔍 The initial approach is to attempt factoring the equation, which is not straightforward due to the lack of obvious factors.
- 📚 The presenter breaks down the quintic equation into a product of a quadratic and a cubic polynomial, using a general form to represent the unknown terms.
- 🔢 By equating coefficients after expanding the product of the assumed quadratic and cubic forms, a system of equations is derived to find the unknown coefficients.
- 🚫 The attempt to solve the quintic using simple factoring or integer solutions proves to be unsuccessful, indicating the complexity of the problem.
- 🤔 The video suggests guessing and checking integer values for the coefficients, highlighting the trial-and-error nature of this problem-solving approach.
- 🎯 After several attempts, the correct values for the coefficients (a=1, b=-1, c=-1, d=2, e=-3) are found, which satisfy all the derived conditions.
- 🧱 With the coefficients determined, the quadratic part of the equation is solved using the quadratic formula, yielding two real solutions.
- 📉 The cubic part is approached using the Lagrange Resolvent method, which simplifies the cubic equation into a quadratic form that can be solved.
- 📈 The real solution to the cubic equation is found to be approximately 1.27, which was previously obtained using Newton's method in a different video.
- 🌐 The video concludes by mentioning the existence of complex solutions but does not delve into finding them, emphasizing the satisfaction of solving such a complex equation.
Q & A
What type of mathematical problem is being discussed in the script?
-The script discusses solving an extreme quintic equation, which is a polynomial equation of degree five.
What is the quintic equation presented in the script?
-The quintic equation given in the script is x^5 - 5x + 3 = 0.
Why is factoring the quintic equation a challenge?
-Factoring the quintic equation is challenging because it does not easily factor like lower degree polynomials, and there are no simple integer solutions for the coefficients.
What method is suggested to attempt to solve the quintic equation?
-The method suggested in the script is to try and factor the quintic equation by breaking it down into a product of a quadratic and a cubic equation.
What are the general forms of the quadratic and cubic equations used in the script?
-The general forms used are x^2 + ax + b for the quadratic and x^3 + cx^2 + dx + e for the cubic equation.
What is the significance of equating coefficients in the process described?
-Equating coefficients is a crucial step in the process as it allows the solver to set up a system of equations to find the unknown coefficients a, b, c, d, and e.
What is the first condition derived from the absence of an x^4 term in the original expression?
-The first condition derived is that c + a must equal zero, which implies that c is equal to -a.
What strategy is used to find possible integer values for the coefficients?
-The strategy used is to guess and check possible integer pairs for b and e that multiply to 3, and then plug these values into the system of equations to solve for the other coefficients.
What are the integer pairs considered for the coefficients b and e?
-The integer pairs considered for b and e are (1, 3), (3, 1), (-1, -3), and (-3, -1).
What is the solution for 'a' when using the combination of b = -1 and e = -3?
-When using the combination of b = -1 and e = -3, the solution for 'a' is found to be a = -1 or a = 1.
What are the final values found for the coefficients a, b, c, d, and e?
-The final values found for the coefficients are a = 1, b = -1, c = -1, d = 2, and e = -3.
How many real solutions does the quadratic equation derived from the factoring have?
-The quadratic equation derived from the factoring has two real solutions, which are approximately 0.618 (or 1 - the golden ratio) and -1.618 (or the negative golden ratio).
What method is used to solve the cubic equation in the script?
-The method used to solve the cubic equation in the script is the Lagrange Resolvent method, which involves finding the roots of a derived quadratic equation.
What is the general form of the cubic equation solved using the Lagrange Resolvent method?
-The general form of the cubic equation solved is x^3 + ax^2 + bx + c = 0, which is transformed into a form where a, b, and c are coefficients to be determined.
What are the values of a, b, and c used in the Lagrange Resolvent cubic formula?
-The values used for a, b, and c in the Lagrange Resolvent cubic formula are a = -1, b = 2, and c = -3.
What are the two possible values for 'z' derived from the quadratic equation in the Lagrange Resolvent method?
-The two possible values for 'z' derived from the quadratic equation are z = 65 + 15√21/2 and z = 65 - 15√21/2.
How does the script suggest finding the complex solutions of the cubic equation?
-The script suggests that finding the complex solutions involves factoring the cubic equation after finding the real solution and then using the quadratic formula on the resulting quadratic equation.
What is the final step in solving the quintic equation after finding the real solutions?
-The final step is to factor the cubic equation using the real solution as a factor and then solve the resulting quadratic equation to find any remaining complex solutions.
Outlines
🧗♂️ Extreme Math Challenge: Solving a Quintic Equation
The speaker introduces the topic of solving an extreme quintic equation, x^5 - 5x + 3 = 0, which was previously discussed. They suggest attempting to factor the equation, but quickly realize that simple factoring does not work. Instead, the speaker proposes to break down the equation into a quadratic and a cubic component, and sets up a general form to find the unknown coefficients a, b, c, d, and e. The process involves equating coefficients after expanding the broken down equation, leading to a system of equations to solve for these coefficients.
🔍 Digging into the Coefficients: Finding Integer Solutions
The speaker continues the process by identifying possible integer values for the coefficients, specifically focusing on b and e, which when multiplied equal 3. They explore different combinations and substitute these into the system of equations to find a consistent set of values for a, b, c, and d. The goal is to find integer solutions, and the speaker uses a process of elimination and substitution to narrow down the possibilities.
🎯 Solving for the Coefficients: A and D Values
The speaker identifies that a must be either -1 or 1 after solving a quadratic equation derived from the system. They test both possibilities and find that if a is -4, the resulting values for d and e are not consistent, indicating that -4 is not a valid solution for a. They then test a = 1 and find consistent values for the remaining coefficients, d = 2 and e = -3, which satisfy all the conditions of the system of equations.
🧮 Factoring the Quintic Equation: From Coefficients to Factors
With the coefficients determined, the speaker proceeds to express the quintic equation as a product of a quadratic and a cubic equation. They use the quadratic formula to find the roots of the quadratic part and then apply the Lagrange Resolvent Cubic Formula to solve the cubic part. The speaker emphasizes the complexity and satisfaction of solving such an equation and cautions against attempting this with random quintic equations, as they are generally not factorable.
📚 Applying the Lagrange Resolvent Cubic Formula
The speaker explains the Lagrange Resolvent Cubic Formula, which is used to solve the cubic part of the equation. They provide the formula and derive the coefficients for the specific cubic equation they are solving. The process involves finding the roots of a related quadratic equation, which are then used in the cubic formula to find the solutions for x.
🔢 Solving the Quadratic Equation for Cubic Roots
The speaker plugs the values of a, b, and c into the quadratic equation derived from the cubic formula to find the values of z1 and z2. They simplify the equation and solve for z, which are the cube roots needed for the cubic formula. The solutions for z are then used to find the real solutions for x in the cubic equation.
🏁 Wrapping Up: Simplifying and Reflecting on the Solution
The speaker concludes the process by simplifying the expressions for the real solutions of the cubic equation and presenting the final answers. They also briefly touch upon the concept of finding complex solutions, but decide against demonstrating it due to the complexity. The speaker expresses satisfaction with the successful solution of the extreme quintic equation and encourages viewers to share their thoughts and engage with future content.
Mindmap
Keywords
💡Extreme Sports
💡Quintic Equation
💡Factoring
💡Quadratic
💡Cubic
💡Coefficients
💡Lagrange Resolvent
💡Synthetic Division
💡Complex Solutions
💡Newton's Method
Highlights
Introduction to solving an extreme quintic equation x^5 - 5x + 3 = 0.
Attempt to factor the quintic equation, which is a challenging task.
Realization that easy factoring is not possible for the given equation.
Strategy to break down the equation into a quadratic times a cubic form.
Setting up a general form for the equation with unknown coefficients a, b, c, d, and e.
Method of equating coefficients after expanding the factored form.
Deriving a system of equations to find the values of a, b, c, d, and e.
First condition identified: c + a = 0, indicating c is equal to -a.
Exploration of possible integer values for b and e to satisfy the equation be = 3.
Guessing b and e values and plugging them into other equations.
Finding a potential solution with a = 1, b = -1, c = -1, d = 2, and e = -3.
Using the quadratic formula to solve the derived quadratic equation.
Discovery of two real solutions related to the golden ratio.
Introduction to Lagrange's resolvent cubic formula for solving the cubic part.
Solving the cubic equation using the derived quadratic equation for z.
Finding the real solution for the cubic equation using synthetic division.
Explanation of how to find complex solutions using the real solution as a factor.
Final satisfaction in solving the extreme quintic equation.
Warning against attempting to solve random quintic equations without a known method.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: