Calculus 3 - Intro To Vectors
TLDRThis video delves into the fundamental differences between scalar and vector quantities, crucial concepts in physics. Scalar quantities are defined by magnitude alone, such as temperature or mass, whereas vectors possess both magnitude and direction, exemplified by velocity and force. Through intuitive examples, like driving a car or applying force to a box, the video makes these concepts accessible. It further explores vectors' representation through directed line segments, their mathematical description via magnitude and direction or components, and operations like addition and subtraction. Practice problems help reinforce understanding, making this an essential guide for grasping the dynamics of vectors and their impact on understanding physical phenomena.
Takeaways
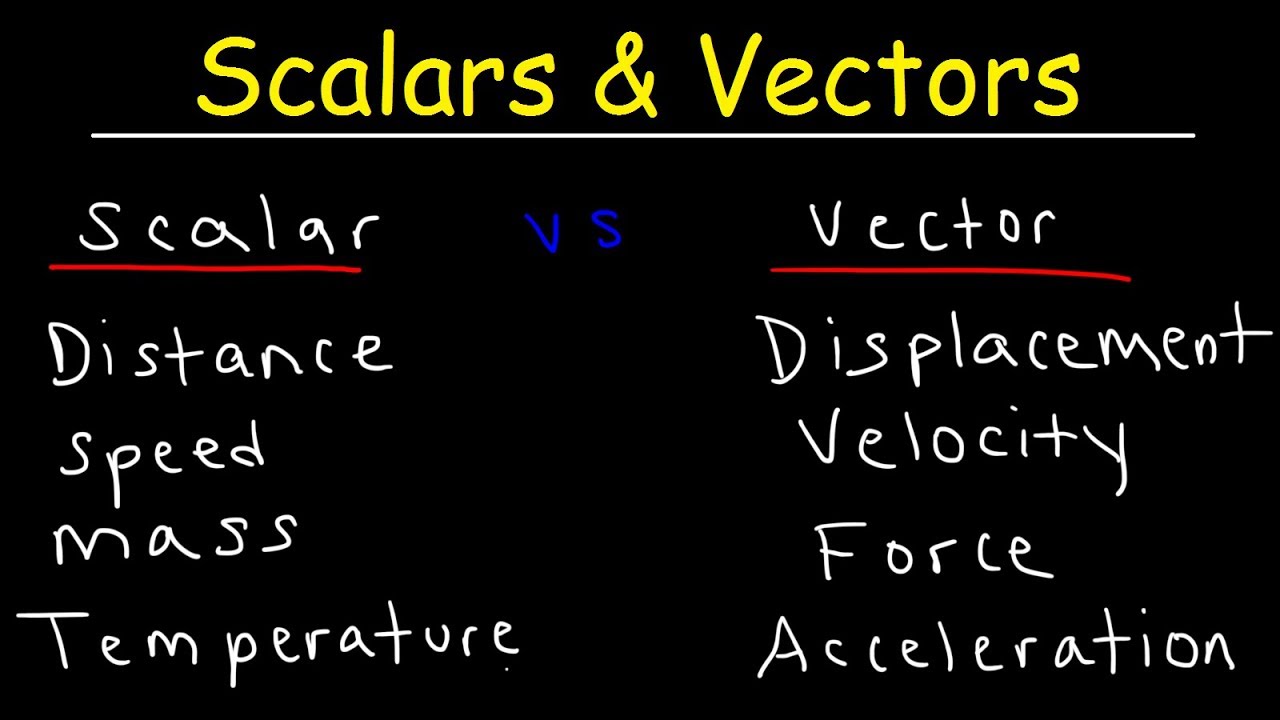
- 📌 Vectors are quantities with both magnitude and direction, unlike scalars which have only magnitude.
- 🚗 Velocity is a vector quantity because it includes speed (magnitude) and direction, while speed alone is a scalar.
- 🔼 The components of a vector can be described using its x and y components in a Cartesian coordinate system.
- 📐 To find the magnitude of a vector, use the Pythagorean theorem: √(x-component² + y-component²).
- 🔄 The direction of a vector can be described by the angle it makes with the positive x-axis, using trigonometric functions.
- 🔽 Vectors can be added or subtracted by connecting them head-to-tail, with the resultant vector starting from the initial point of the first to the terminal point of the last.
- 🔄 Unit vectors are vectors with a magnitude of one, representing direction only, and can be used to express any vector as a linear combination.
- 📍 Position vectors have their initial point at the origin, and their components are the same as the coordinates of the terminal point.
- 🔢 To find a unit vector from a given vector, divide the vector by its magnitude.
- 🔍 The standard unit vectors in 3D space are i (x-axis), j (y-axis), and k (z-axis), each with a magnitude of one.
- 🔎 The unit circle is a circle with a radius of one, useful for finding unit vectors and their x and y components using cosine and sine of an angle.
Q & A
What is the difference between a scalar and a vector quantity?
-A scalar quantity has only magnitude, while a vector quantity has both magnitude and direction.
Can you give an example of a scalar and a vector quantity?
-Speed is an example of a scalar quantity, and velocity is an example of a vector quantity.
Why can't temperature be considered a vector quantity?
-Temperature cannot be considered a vector quantity because it does not have a direction; it only has magnitude.
What does the magnitude of a vector represent?
-The magnitude of a vector represents how long it is, essentially indicating the size or length of the vector.
How can a vector be described in terms of its components?
-A vector can be described using its x and y components, which represent how far it extends along the x and y axes, respectively.
What is the difference between a point and a vector in graphical representation?
-A point is simply a location in space, represented by a dot, while a vector is a directed line segment that has both magnitude and direction.
How do you find the component form of a vector given its initial and terminal points?
-To find the component form, subtract the coordinates of the initial point from the coordinates of the terminal point.
What is a position vector and how is it represented?
-A position vector has its initial point at the origin and is represented by the coordinates of its terminal point.
How can you determine if two vectors are equivalent?
-Two vectors are equivalent if they have the same magnitude and direction, which can be verified by comparing their components or slopes.
What is a unit vector and how is it obtained from a vector?
-A unit vector is a vector with a magnitude of one, pointing in the same direction as the original vector. It is obtained by dividing the vector by its magnitude.
Outlines
📌 Introduction to Vectors and Scalars
This paragraph introduces the fundamental concepts of vectors and scalars. It explains that a scalar quantity possesses only magnitude, such as speed, temperature, and mass, while a vector quantity includes both magnitude and direction, like velocity and force. The key distinction between the two is the presence or absence of direction. The examples provided help clarify the difference, emphasizing that vectors can be represented as directed line segments from an initial to a terminal point.
📐 Describing Vectors through Magnitude, Angle, and Components
This section delves into the different ways to describe a vector. It explains that vectors can be characterized by their magnitude and the angle they make with a reference axis, such as the x-axis. Additionally, vectors can be described in terms of their x and y components, which correspond to their projections along the respective axes. The paragraph also highlights the importance of distinguishing between points and vectors, with points being represented by coordinates in space and vectors having components that indicate both magnitude and direction.
🔍 Practice Problem: Finding Component Form and Magnitude of a Vector
This paragraph presents a practice problem to find the component form and magnitude of a vector given its initial and terminal points. It outlines the process of calculating the x and y components by subtracting the coordinates of the initial point from those of the terminal point. The magnitude of the vector is then found using the Pythagorean theorem, which involves taking the square root of the sum of the squared components. The paragraph also illustrates these concepts with a graphical representation.
🔄 Comparing Vectors for Equivalence
This paragraph discusses how to determine if two vectors are equivalent. Two vectors are considered equivalent if they have the same magnitude and direction. It explains how to find the component forms of the vectors and how to calculate their magnitudes and slopes. The process of comparing the vectors' components, magnitudes, and slopes is detailed, emphasizing that differences in any of these aspects result in non-equivalent vectors.
🤝 Adding and Subtracting Vectors
This section explains the process of adding and subtracting vectors. It describes how to graphically add vectors by connecting them head-to-tail and how to subtract one vector from another by reversing the direction of the vector being subtracted. The paragraph also covers the concept of scalar multiplication, which involves changing the magnitude of a vector while preserving its direction. The rules for adding multiple vectors are outlined, emphasizing that the order of addition does not affect the result.
📈 Operations with Vectors: Practice Problems
This paragraph presents practice problems involving vector operations. It provides step-by-step instructions for calculating the value of expressions involving vector addition, subtraction, and scalar multiplication. The problems require replacing vector variables with their component forms, performing the necessary arithmetic operations, and combining like terms to arrive at the final vector result.
📍 Understanding Position Vectors
This section defines position vectors as vectors whose initial point is at the origin, with coordinates of (0,0). It explains that the components of a position vector are the same as the coordinates of its terminal point. The paragraph emphasizes the importance of understanding position vectors in vector mathematics and their role in representing the location of a point in space.
🛤️ Finding Unit Vectors and Standard Unit Vectors
This paragraph introduces unit vectors, which are vectors with a magnitude of one, and explains how to find the unit vector of a given vector by dividing the vector by its magnitude. It also discusses standard unit vectors, i, j, and k, which are used in three-dimensional systems and have a magnitude of one along the x, y, and z axes, respectively. The paragraph demonstrates how to represent any vector using standard unit vectors and how to perform vector operations using these units.
📐 Using Unit Vectors and the Unit Circle
This section explains how to use unit vectors and the unit circle to represent and manipulate vectors. It describes how the unit circle relates to unit vectors, with the x and y components of a unit vector corresponding to the cosine and sine of the angle it makes with the positive x-axis. The paragraph also covers how to express a vector as a linear combination of standard unit vectors using its magnitude and angle, and how to find the magnitude and angle of a vector given its components.
🌐 Determining Magnitude and Angle for Vectors
This paragraph focuses on finding the magnitude and angle of a vector. It explains how to calculate the magnitude using the Pythagorean theorem and how to determine the angle a vector makes with the positive x-axis using the tangent function and reference angle. The process of finding the angle in different quadrants is detailed, with specific formulas provided for each quadrant. The paragraph also discusses how to find the resultant force vector from two given vectors and how to describe it using unit vectors or magnitude and angle.
🎯 Summary of Vector Operations and Applications
This final paragraph summarizes the key concepts and operations covered in the video. It reviews the methods for adding and subtracting vectors, finding the magnitude and angle of vectors, and using unit vectors and the unit circle in vector calculations. The paragraph also provides a comprehensive example of finding the resultant force of two vectors, demonstrating the practical application of the concepts discussed throughout the video.
Mindmap
Keywords
💡Vector
💡Scalar
💡Magnitude
💡Direction
💡Component Form
💡Unit Vector
💡Vector Addition
💡Vector Subtraction
💡Position Vector
💡Standard Unit Vectors
💡Resultant Force
Highlights
The main difference between a vector and a scalar quantity is that a vector has both magnitude and direction, while a scalar quantity has only magnitude.
Speed is a scalar quantity as it only provides how fast an object is moving, whereas velocity is a vector quantity as it provides both speed and direction.
Force is a vector quantity as it can be described by both magnitude and direction, for example, 300 newtons directed east.
Temperature and mass are examples of scalar quantities because they cannot be associated with a direction.
Volume, like temperature and mass, is a scalar quantity because it cannot be assigned a direction.
A vector can be represented as a directed line segment from an initial point to a terminal point.
Vectors can be described by their magnitude and the angle they make with a reference axis, such as the x-axis.
The component form of a vector can be represented by its x and y components, which correspond to its displacement along the x and y axes.
To determine if two vectors are equivalent, one must check if they have the same magnitude and direction.
The magnitude of a vector can be calculated using the Pythagorean theorem for right triangles.
Vectors can be added or subtracted by connecting them head-to-tail and completing the triangle formed to find the resultant vector.
Multiplying a vector by a scalar quantity changes only the magnitude of the vector, not its direction.
Unit vectors are vectors with a magnitude of one and are used to describe the direction of a vector.
Standard unit vectors are denoted as i, j, and k and correspond to the x, y, and z axes in three-dimensional space.
Any vector can be expressed as a linear combination of its magnitude and its unit vector.
The unit circle is related to unit vectors, where the x and y components of a unit vector are equivalent to the cosine and sine of its angle with the positive x-axis.
To find the angle a vector makes with the positive x-axis, one can use the tangent function and the arc tangent function.
The resultant force vector can be found by adding two force vectors and can be described using unit vectors or by its magnitude and the angle it makes with the positive x-axis.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: