Applying the Definition of the Derivative to 1/x
TLDRThe video script discusses the concept of derivatives in calculus, specifically focusing on the derivative of the function 1/x. The presenter explains the process of finding derivatives algebraically, rather than geometrically, and emphasizes the importance of avoiding division by zero. The script walks through the steps of finding the derivative by first setting up the limit as h approaches 0, then applying algebraic manipulations to simplify the expression. The presenter highlights the need to cancel out terms carefully and to use the limit laws to evaluate the expression as h approaches 0. The final result is the derivative of 1/x, which is -1/x^2, and the presenter clarifies that this derivative is only valid for x not equal to zero, as the original function is undefined at that point. The script serves as an educational guide on how to derive new functions from given functions using the definition of a derivative.
Takeaways
- 📚 The concept of a derivative is introduced as a new function, F prime of X, which represents the rate of change of the original function f at any point X.
- 🔢 The derivative is calculated using the limit as h approaches 0 of the function evaluated at X plus h minus the function evaluated at X, all divided by h.
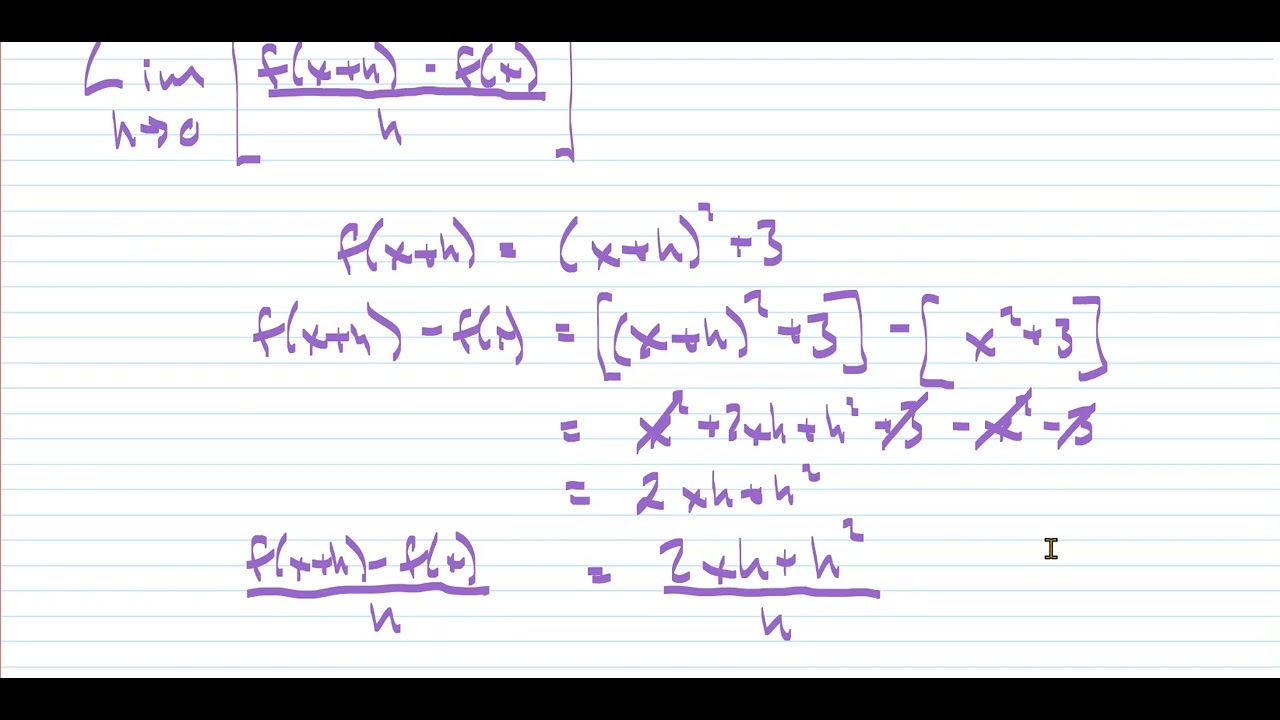
- 🎓 An example is provided to demonstrate the process of finding the derivative of the function 1/X, which is done algebraically rather than geometrically.
- 🧩 The algebraic trick of finding a lowest common denominator is used to simplify the expression for the derivative, which is crucial when dealing with fractions.
- ➗ It is emphasized that we cannot directly substitute h with 0 at the beginning of the derivative calculation due to potential division by zero issues.
- 🚫 The original function f and its derivative F prime are undefined at X equals 0, which is a critical consideration in the analysis.
- 🔑 The limit laws are applied to evaluate the derivative as h approaches 0, which simplifies the expression to -1/(X^2) for the given example.
- 📉 The geometric interpretation of the derivative as the slope of a curve at a particular point is mentioned, highlighting its significance in understanding the behavior of functions.
- 📝 The process of finding the derivative involves careful algebraic manipulation to avoid discontinuities and ensure the function is continuous at the point of interest.
- 📈 The final expression for the derivative of 1/X is derived as -1/(X^2), which is a continuous function where X is not equal to zero.
- ✅ The script concludes by affirming that the algebraic procedure by the definition of the derivative is a method to derive new functions from given functions and understand their slopes at various points.
Q & A
What is the concept of a derivative in the context of the given transcript?
-The derivative in the context of the transcript refers to the mathematical concept that describes the rate at which a function changes with respect to its variable. It is often thought of as the slope of the tangent line to the function at a particular point, and is found by taking the limit of the difference quotient as the interval approaches zero.
Why is it necessary to replace 'a' with 'x' when defining the derivative as a function?
-Replacing 'a' with 'x' allows for a more general representation of the derivative as a function, F'(x), which can be applied to any point 'x' along the function, not just a specific point 'a'. This makes the derivative a function that can be computed for any value within its domain.
What is the algebraic trick used when dealing with a difference of fractions?
-The algebraic trick used when dealing with a difference of fractions is to find a lowest common denominator. This allows the fractions to be combined into a single fraction, simplifying the expression and making it easier to work with.
How does the process of finding the derivative of 1/x illustrate the general method of differentiation?
-The process of finding the derivative of 1/x demonstrates the general method of differentiation by applying the definition of the derivative, which involves evaluating the limit of the difference quotient as h approaches zero. It shows the algebraic manipulation required to simplify the expression and ultimately find the derivative function.
Why is it important to avoid division by zero when finding the derivative?
-Avoiding division by zero is crucial because it is undefined in mathematics. The process of finding the derivative involves simplifying the expression before substituting values to ensure that no undefined operations, such as division by zero, occur. This ensures the continuity and validity of the derivative function.
What is the final result of the derivative of the function 1/x?
-The final result of the derivative of the function 1/x, after applying the limit as h approaches zero and simplifying the expression, is found to be -1/(x^2).
Why is the original function f(x) not defined at x=0, and what does this imply for its derivative?
-The original function f(x) = 1/x is not defined at x=0 because it results in a division by zero, which is undefined. Consequently, the derivative of the function, which is based on the behavior of the function at all points in its domain, is also not defined at x=0.
What is the significance of the limit laws in evaluating the derivative?
-Limit laws are essential in evaluating the derivative because they provide a systematic way to handle the limit process, particularly when dealing with rational functions. They allow for the simplification of expressions and the proper evaluation of the limit as h approaches zero without encountering undefined operations.
How does the geometric interpretation of a derivative relate to its algebraic definition?
-The geometric interpretation of a derivative as the slope of the tangent line to a curve at a particular point is closely related to its algebraic definition. The algebraic process of finding the derivative through limits and difference quotients essentially calculates the slope of the tangent line, providing a precise measure of the rate of change of the function at any given point.
What is the role of the limit in the definition of a derivative?
-The limit plays a fundamental role in the definition of a derivative as it allows for the precise calculation of the rate of change of a function at a specific point. By taking the limit as the interval approaches zero, the derivative captures the instantaneous rate of change, which is the concept of the slope of the tangent line at that point.
Why is it essential to perform algebraic manipulation before evaluating the limit as h approaches zero?
-Performing algebraic manipulation before evaluating the limit is essential to simplify the expression and avoid undefined operations, such as division by zero. It ensures that the limit can be evaluated in a meaningful way, leading to a well-defined derivative function that is continuous and free of discontinuities.
How does the process of finding the derivative of a function help in understanding the function's behavior?
-Finding the derivative of a function helps in understanding its behavior by providing information about the function's rate of change at every point in its domain. This can reveal important characteristics of the function, such as where it is increasing or decreasing, and can also be used to identify local maxima, minima, and points of inflection.
Outlines
📚 Derivative as a New Function
The paragraph explains the concept of derivatives in calculus. The speaker begins by discussing the computation of a derivative at a specific point 'a' and then generalizes this to any point 'x'. The derivative is defined as a new function, F'(x), which represents the limit of the difference quotient (f(x+h) - f(x)) / h as h approaches zero. An example is given to illustrate the process of finding the derivative of the function 1/x algebraically. The process involves evaluating the limit, applying algebraic tricks to simplify the expression, and finally plugging in h=0 to avoid division by zero. The speaker emphasizes the importance of not directly substituting h=0 initially to prevent discontinuities or division by zero. The final result of the derivative of 1/x is -1/x^2, and the speaker clarifies that this derivative is only defined for x not equal to zero.
📈 Understanding the Derivative Geometrically
This paragraph focuses on the geometric interpretation of derivatives. The speaker clarifies that the algebraic procedure used to find derivatives can also be understood geometrically as the slope of a curve at a particular point. The example of the function 1/x is used again to demonstrate that the derivative represents the slope of the curve. The speaker points out that the original function 1/x is not defined at x=0, and therefore, it doesn't make sense to discuss the derivative at that point. The paragraph emphasizes the relevance of the derivative in understanding the rate of change of a function and its geometric significance.
Mindmap
Keywords
💡Derivative
💡Limit
💡Function
💡Slope
💡Algebraic Trickery
💡Common Denominator
💡Continuity
💡Geometric Interpretation
💡Rational Function
💡Division by Zero
💡Undefined
Highlights
The concept of replacing a specific derivative at a point with a new function F prime of X is introduced.
F prime of X is defined as the limit of (f(X + H) - f(X)) / H as H approaches 0.
The importance of considering the derivative as a function rather than a single value is emphasized.
A demonstration of computing the derivative of 1/X algebraically, rather than geometrically.
The use of algebraic tricks, such as finding a lowest common denominator, to simplify the derivative computation.
The process of evaluating the limit as H approaches 0 to find the derivative of a function.
The cancellation of H in the numerator and denominator simplifies the expression for the derivative.
The final derivative of 1/X is found to be -1/(X^2) after evaluating the limit.
The explanation of why H cannot be set to 0 from the beginning due to potential division by zero.
The algebraic procedure is shown to be valid only when X is not equal to zero, as the original function is undefined at zero.
The derivative of the function at X equals zero is not defined, aligning with the original function's undefined nature at that point.
The significance of the algebraic procedure in defining derivatives from functions is clarified.
The geometric interpretation of the derivative as the slope of a function at a particular value of X.
The transcript provides a step-by-step guide on how to compute derivatives algebraically.
The importance of careful algebraic manipulation to avoid discontinuities or divisions by zero is highlighted.
The method presented allows for the computation of derivatives for any given function f.
The final expression for the derivative is simplified to a continuous function at points where X is not zero.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: