First principles grade 12: Practice
TLDRThis video script explains the process of finding the first derivative using first principles. It begins with the definition of the derivative as the limit of the difference quotient as h approaches zero. The script demonstrates replacing x with x+h, finding a common denominator, and simplifying the expression. It emphasizes the importance of writing the limit notation and correctly applying algebraic manipulation to simplify the fraction. The final step involves canceling terms and letting h approach zero to obtain the derivative, which is presented as -2/x^2 in this example.
Takeaways
- 📚 The script discusses the process of determining the first derivative using first principles.
- 🔍 It emphasizes the importance of using the first principle formula for differentiation.
- 📝 The first step involves replacing all instances of 'x' with 'x + h' in the function.
- 🧩 The process requires identifying a common denominator to combine the fraction terms.
- 🔄 The script mentions the need to multiply terms by 'x + h' to achieve a common denominator.
- 📉 The common denominator is identified as '(x + h) * x'.
- 📌 The script highlights the importance of writing the limit notation throughout the process.
- ✂️ Simplification of the expression is achieved by combining like terms and canceling out '2x'.
- 🔢 The script demonstrates the simplification of the expression to 'minus 2h over x(x + h)'.
- 🔄 Further simplification leads to a fraction over a fraction, which is then simplified by considering 'h' as 'h over 1'.
- 🎯 The final step is to let 'h' approach zero, resulting in the derivative '-2 over x squared'.
- 👨🏫 The script humorously notes that teachers get very 'uptight' about proper notation, emphasizing the importance of adhering to mathematical conventions.
Q & A
What is the first principle formula for finding the derivative of a function?
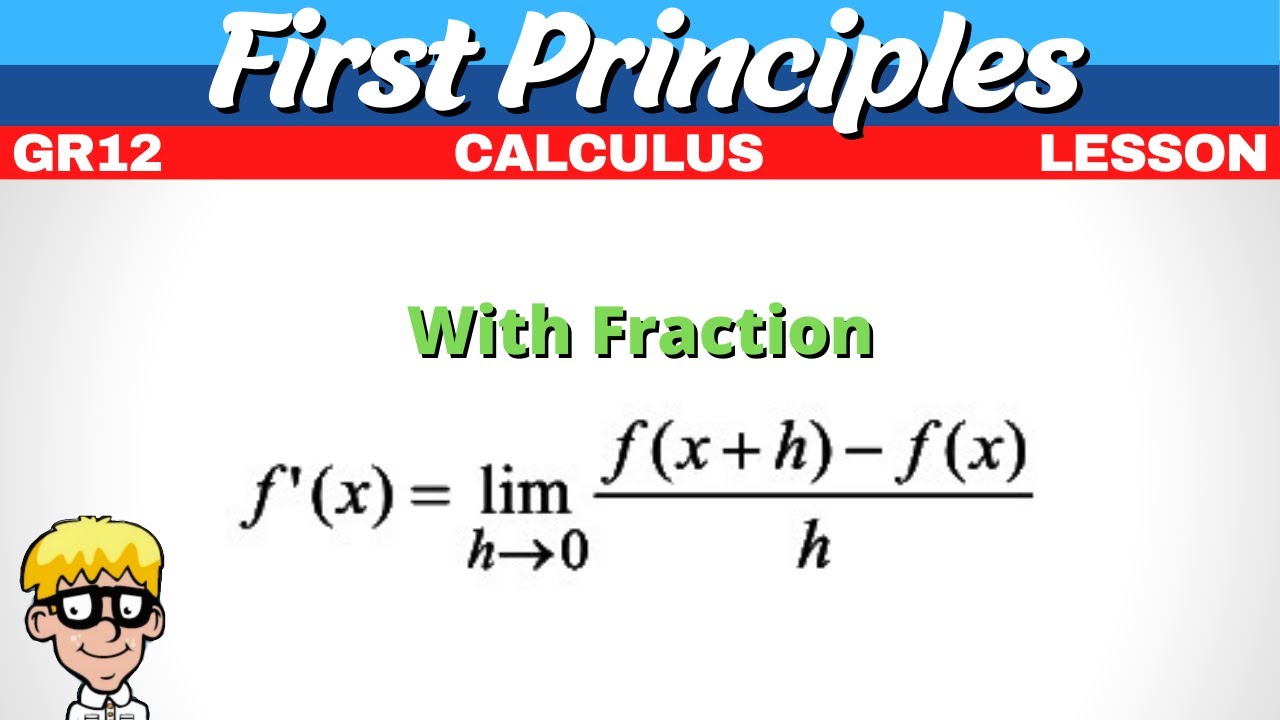
-The first principle formula for finding the derivative of a function f(x) is the limit as h approaches zero of [f(x + h) - f(x)] / h.
What is the first step when applying the first principle formula to find a derivative?
-The first step is to replace all instances of x with (x + h) in the function.
Why is it necessary to find a common denominator when applying the first principle formula?
-A common denominator is necessary to combine the terms in the numerator after replacing x with (x + h), which allows for the simplification of the expression.
What is the significance of writing the limit notation when performing calculus operations?
-Writing the limit notation is important as it clearly indicates that the derivative is being found through the process of taking a limit as h approaches zero.
How do you simplify the expression after finding a common denominator in the first principle formula?
-You combine the terms in the numerator that now have the same denominator and simplify the expression by canceling out like terms.
What happens to the terms 2x - 2x in the numerator after simplification?
-The terms 2x - 2x cancel each other out, leaving no contribution to the numerator.
Why is it important to consider h as h/1 when dealing with fractions within a fraction?
-Considering h as h/1 allows for the application of algebraic manipulation techniques such as flipping and multiplying, which simplifies the expression.
What occurs when the h terms in the expression cancel out?
-When the h terms cancel out, it simplifies the expression to -2/(x(x + h)), which can then be further simplified by letting h approach zero.
Why can the limit notation be omitted when h is set to zero in the final step of the derivative calculation?
-The limit notation can be omitted in the final step because the limit has already been taken, and we are simply evaluating the expression at h = 0.
What is the final result of the derivative calculation in the script?
-The final result of the derivative calculation is -2/x^2, which is obtained after letting h approach zero and simplifying the expression.
Why do teachers emphasize the importance of writing down the limit notation?
-Teachers emphasize the importance of writing down the limit notation because it is a fundamental aspect of calculus that defines the process of finding a derivative, and it ensures clarity and accuracy in mathematical communication.
Outlines
📚 Calculating the First Derivative Using First Principles
This paragraph explains the process of finding the first derivative of a function using first principles. It begins by stating the need to use the first principle formula, which involves taking the limit as h approaches zero of the difference quotient (f(x+h) - f(x)) / h. The script then guides through the substitution of x with x+h, setting up the expression with a common denominator, and simplifying the resulting fraction. It emphasizes the importance of including the limit notation, which is often a point of focus for teachers. The process continues with combining like terms and simplifying the expression further until the h terms cancel out, leading to the final derivative, which is -2/x^2, once h is set to zero.
Mindmap
Keywords
💡First Derivative
💡First Principles
💡Limit
💡Difference Quotient
💡Common Denominator
💡Simplify
💡Cancel
💡Fraction
💡Multiply Across
💡Hypothetical
💡Contextualize
Highlights
The question asks to determine the first derivative using first principles.
First principle formula for derivative is f(x) = lim(h→0) [f(x+h) - f(x)] / h.
Replace all x's with x + h in the function.
Write out the original function f(x) separately.
Identify the need for a common denominator to combine the fractions.
The common denominator is (x + h) * x.
Multiply numerators by x and (x + h) to match the common denominator.
Include the limit notation in the calculation for clarity.
Combine the terms over the common denominator.
Simplify the numerator by canceling out like terms.
Result is (2x - 2x + h) / (x(x + h)) after simplification.
Rearrange the fraction by flipping and multiplying to simplify further.
Cancel out the h terms in the numerator and denominator.
Simplify to -2h / (x^2 + xh) before letting h approach 0.
Final simplification results in -2 / x^2 after h = 0.
The limit notation can be omitted once h is set to 0.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: