Finding Derivative Using Limit Definition
TLDRThe video script provides a step-by-step guide on finding the derivative of a function using the limit definition of the derivative. The function chosen for demonstration is f(x) = x^2 + 3, and the goal is to find its derivative at x = 4. The process begins by writing out the definition of the derivative, which involves calculating the limit as h approaches zero of [f(x+h) - f(x)]/h. The function f(x+h) is first expanded to (x+h)^2 + 3, and then the numerator is simplified by subtracting f(x) and combining like terms, resulting in 2xh + h^2. This expression is then divided by h, leading to 2x + h after factoring out h and canceling it out. Finally, taking the limit as h approaches zero yields the derivative 2x, which is denoted as f'(x). To find the derivative at x = 4, the value 4 is substituted into the derivative to get f'(4) = 8. This video is an excellent resource for those looking to understand the fundamental concept of derivatives without relying on shortcut rules.
Takeaways
- 📚 The video demonstrates how to find the derivative of a function using the limit definition, not shortcut rules.
- 📈 The function given as an example is f(x) = x^2 + 3, and the goal is to find its derivative at x = 4.
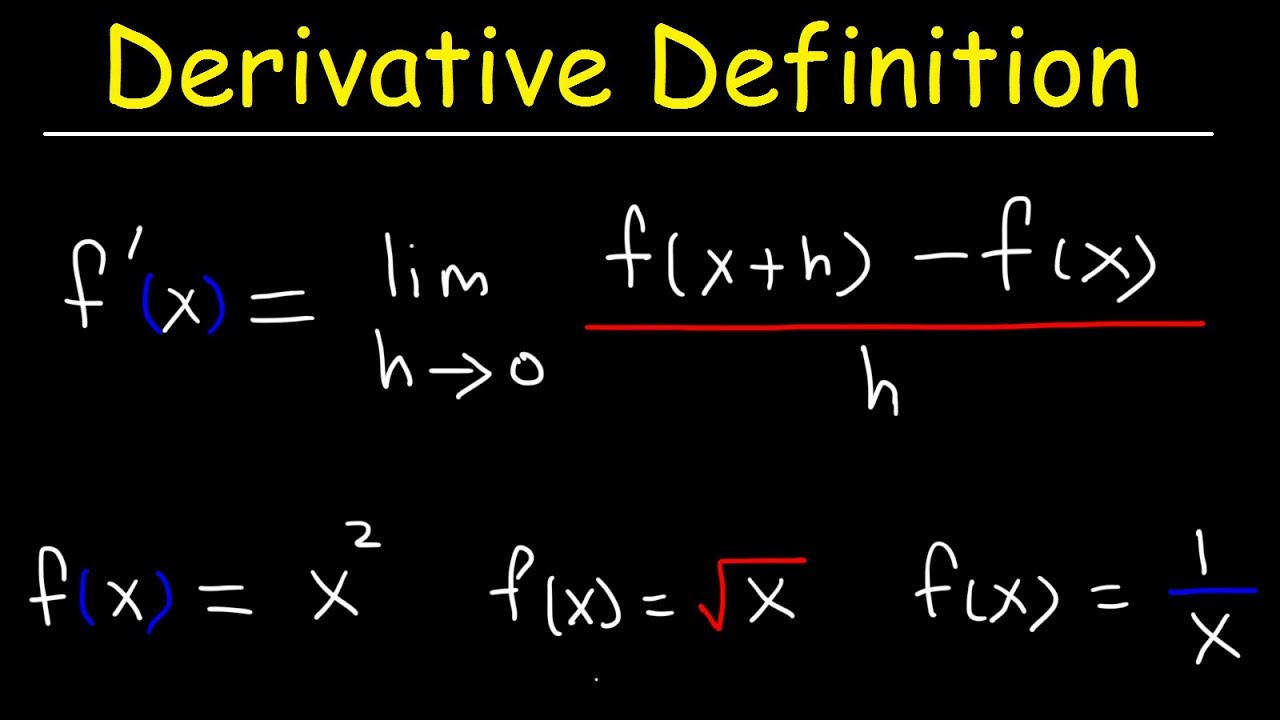
- 🎯 The derivative definition used is the limit as h approaches zero: [f(x + h) - f(x)] / h.
- 🔢 To apply the definition, first find f(x + h) which becomes (x + h)^2 + 3 for the given function.
- 🛠 Subtract f(x) from f(x + h) to get the numerator of the derivative formula: 2xh + h^2.
- ➗ Divide the result by h to simplify the expression to 2x + h.
- ⏱️ Take the limit as h approaches zero to find the derivative, which simplifies to 2x.
- 📝 The derivative of the function, f'(x), is thus 2x, representing the slope of the tangent line to the curve at any point x.
- 👉 To find the derivative at a specific point, such as x = 4, substitute 4 into f'(x) to get f'(4) = 8.
- 📌 The process illustrates the concept of derivatives as a measure of the rate of change of a function at a given point.
- 🧮 The video also implies the importance of understanding the mathematical process behind the derivative, not just memorizing formulas.
- 🔗 The script references other resources, like a quick math video on factoring and binomial expansion, for a more comprehensive understanding.
Q & A
What is the main topic of the video?
-The main topic of the video is to demonstrate how to find a derivative using the limit definition of the derivative, without using shortcut rules.
What function is used as an example in the video?
-The function used as an example is f(x) = x^2 + 3.
What is the value at which the derivative of the function is to be found?
-The derivative of the function is to be found at the value x = 4.
What is the limit definition of the derivative?
-The limit definition of the derivative is the limit as h approaches zero of [f(x + h) - f(x)] / h.
How is the function f(x + h) represented in the example?
-In the example, f(x + h) is represented as (x + h)^2 + 3.
What is the simplified form of the numerator after expanding and simplifying f(x + h) - f(x)?
-The simplified form of the numerator is 2xh + h^2.
How is the expression 2xh + h^2 simplified further?
-The expression is simplified further by dividing by h, which results in 2x + h after factoring out h and canceling it out.
What is the final form of the derivative found using the limit definition?
-The final form of the derivative is 2x, which is represented as f'(x).
How is the derivative evaluated at x = 4?
-The derivative is evaluated at x = 4 by substituting 4 for x, resulting in 8.
What is the notation used to represent the derivative of a function at a specific point?
-The notation used is f'(a), where 'a' is the specific point at which the derivative is evaluated.
What is the significance of finding the limit as h approaches zero in the derivative definition?
-The limit as h approaches zero is significant because it represents the instantaneous rate of change of the function at a specific point, which is the definition of the derivative.
Why does the video emphasize not using shortcut rules for finding derivatives?
-The video emphasizes not using shortcut rules to demonstrate the fundamental concept and process of finding derivatives using the limit definition, which is essential for understanding the underlying theory of calculus.
Outlines
📚 Introduction to Derivatives using Limit Definition
This paragraph introduces the concept of finding a derivative using the limit definition, as opposed to shortcut rules typically taught in calculus. The video aims to demonstrate the fundamental definition of a derivative. The example function given is f(x) = x^2 + 3, and the goal is to find the derivative at x = 4. The process involves writing out the definition of the derivative, which is the limit as h approaches zero of [f(x + h) - f(x)] / h. The function f(x + h) is first expanded to (x + h)^2 + 3, and then the numerator of the derivative is formed by subtracting f(x) from f(x + h). After simplification, the expression 2xh + h^2 is obtained, which is then divided by h to get 2x + h. Finally, taking the limit as h approaches zero results in the derivative being 2x, which is denoted as f'(x). To find f'(4), the value 4 is substituted into the derivative to get 8.
Mindmap
Keywords
💡Derivative
💡Limit Definition
💡Function
💡Binomial Expansion
💡Difference Quotient
💡Factoring
💡Simplifying Expressions
💡Canceling Out Terms
💡Substitution
💡Shortcut Rules
💡Calculus Course
Highlights
Demonstrates finding a derivative using the limit definition of the derivative without using shortcut rules.
Function f(x) = x^2 + 3 is used as an example to find the derivative at x = 4.
Derivative notation f'(a) indicates finding the derivative of the function at a specific point a.
The limit definition of the derivative is introduced: lim(h->0) [f(x+h) - f(x)] / h.
f(x+h) is calculated as (x+h)^2 + 3 to apply the limit definition.
Subtracting f(x) from f(x+h) gives the numerator for the derivative formula.
Binomial expansion is used to simplify the expression (x+h)^2.
The simplified expression results in 2xh + h^2 after distributing and combining like terms.
The derivative formula's numerator is divided by h to simplify the expression.
Factoring out h from the numerator allows for cancellation, resulting in 2x + h.
Taking the limit as h approaches zero simplifies the expression to 2x.
The derivative of the function f(x) is found to be 2x, denoted as f'(x).
Substituting x = 4 into the derivative yields f'(4) = 8.
The process illustrates the application of the derivative definition in a step-by-step manner.
Shortcut rules for derivatives are intentionally not used to focus on the fundamental definition.
A quick math video review on binomial expansion and factoring is referenced for those needing a refresher.
The final derivative result is obtained by substituting the value back into the derived function.
The video serves as a comprehensive guide for those learning the concept of derivatives from first principles.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: