BusCalc 16 Continuity and Differentiability
TLDRThe video script delves into the mathematical concepts of continuity and differentiability. It begins by explaining the informal notion of continuity, where a function can be drawn without lifting the pen, and a discontinuity is where the pen must be lifted. The formal definition of continuity at a point is presented, emphasizing that the function must be defined, the limit exists, and the limit equals the function's value at that point. Discontinuities are also discussed, with examples of when they occur. The script then explores one-sided continuity, which is applicable to endpoints of a closed interval and is somewhat easier to achieve than two-sided continuity. The concept of differentiability is introduced, stating that a function is differentiable if its derivative can be taken. Points where a function is not differentiable, such as when undefined, pointy, or has a vertical tangent line, are highlighted. The script concludes by noting that while differentiability implies continuity, the converse is not always true, as demonstrated by examples of continuous but non-differentiable functions.
Takeaways
- 📐 **Continuity Definition**: A function is continuous if it can be drawn without lifting the pen from the paper, indicating no breaks or gaps.
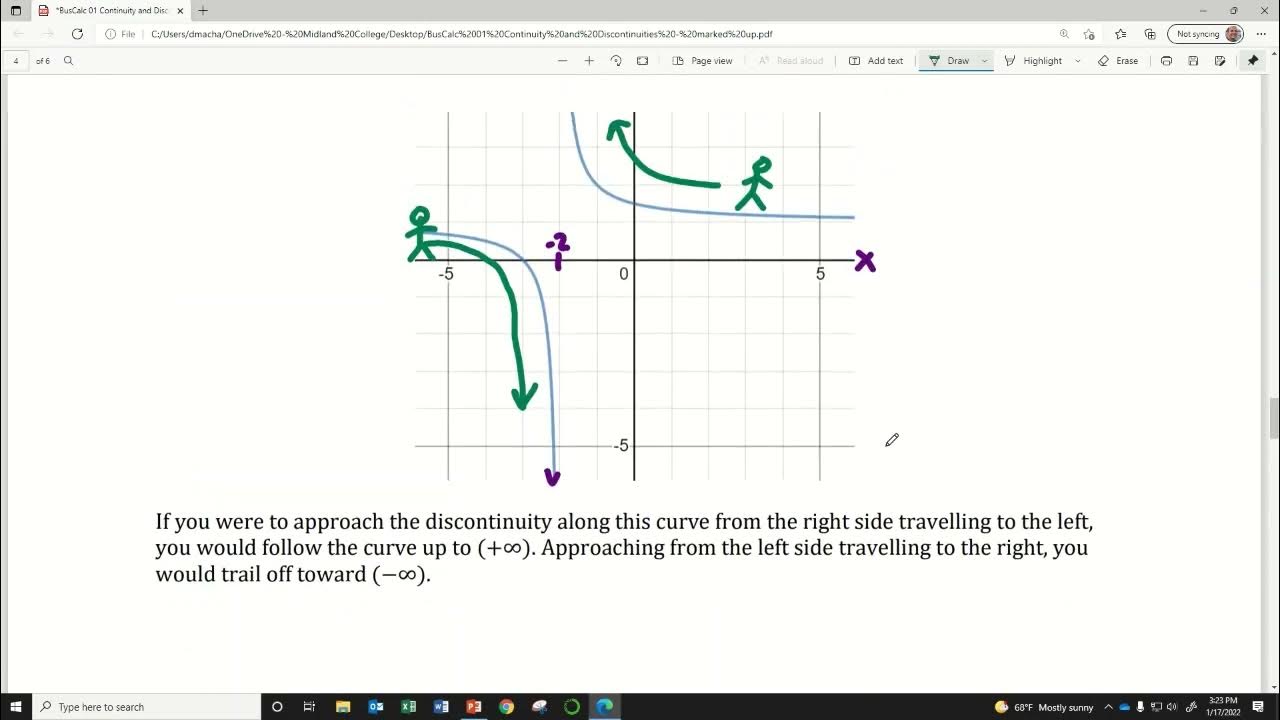
- 🚫 **Discontinuity**: A point where the pen must be lifted while drawing the function is called a discontinuity, representing a break in the function.
- 🔢 **Formal Continuity Criteria**: For a function to be continuous at a point x=c, it must be defined at x=c, the two-sided limit exists as x approaches c, and the limit equals the function value at c.
- ➡️ **One-Sided Continuity**: Continuity from the left or right considers only left-sided or right-sided limits, respectively, which is easier to satisfy than two-sided continuity.
- 🔁 **Interval Continuity**: A function is continuous on an open interval if it is continuous at every point within the interval, and on a closed interval if it meets these conditions and is also continuous from the left at the lower bound and from the right at the upper bound.
- 🔵 **Unit Circle Example**: The function y = √(1 - x^2) represents the upper semicircle of the unit circle and is continuous on the closed interval from -1 to 1.
- 📉 **Differentiability**: A function is differentiable if its derivative exists at a point, meaning there is a well-defined slope to the tangent line at that point.
- ❌ **Non-Differentiable Points**: Functions are not differentiable at points where they are undefined, have a sharp corner (like the absolute value function at the origin), or have a vertical tangent line (like the cube root function at x=0).
- 🔶 **Geometric Interpretation**: A function with a vertical tangent line at a point has an infinite slope, which means it is not differentiable at that point.
- ➿ **Differentiability Implies Continuity**: If a function is differentiable at a point, it is also continuous at that point, but the converse is not necessarily true; a continuous function may not be differentiable.
- ⛔️ **Continuous but Not Differentiable**: There are functions, like the absolute value function at the origin, that are continuous everywhere but not differentiable at specific points.
Q & A
What does it mean for a function to be continuous?
-A function is considered continuous if you can draw its graph without lifting your pen. More formally, a function is continuous at a point x=c if it is defined at that point, the two-sided limit exists as x approaches c, and the limit is equal to the function's value at c.
What is a discontinuity in a function?
-A discontinuity is a point on the graph of a function where the function is not continuous. It's where you would have to lift your pen while drawing the function to continue the graph.
What are the three requirements for a function to be continuous at a point?
-The three requirements are: 1) The function must be defined at the point x=c. 2) The two-sided limit as x approaches c must exist. 3) The two-sided limit must be equal to the function's value at c.
What is the difference between continuity from the left and continuity from the right?
-Continuity from the left refers to the left-sided limit as x approaches a point c, disregarding the right-sided limit. Continuity from the right refers to the right-sided limit as x approaches c, disregarding the left-sided limit. One-sided continuity is easier to attain than two-sided continuity.
What is the relationship between differentiability and continuity?
-If a function is differentiable at a point, it is also continuous at that point. However, the converse is not necessarily true; a function can be continuous without being differentiable.
Why is the function y = |x| not differentiable at the origin?
-The function y = |x| is not differentiable at the origin because it has a sharp corner (pointy point) at x=0, which means there is no unique slope for a tangent line at that point.
What is the domain of the function y = sqrt(1 - x^2)?
-The domain of the function y = sqrt(1 - x^2) is the closed interval between -1 and 1, which means x can take any value between -1 and 1, inclusive.
What is the geometric interpretation of a function not being differentiable at a point?
-Geometrically, a function is not differentiable at a point if there is no unique tangent line that can be drawn at that point. This can occur if the function has a sharp corner, a vertical tangent, or is undefined at that point.
Why is the cube root function not differentiable at x = 0?
-The cube root function is not differentiable at x = 0 because its derivative, which is 1/(3x^(2/3)), becomes undefined at x = 0 as it approaches infinity.
What does it mean for a function to have a vertical tangent line at a point?
-A function has a vertical tangent line at a point if the slope of the tangent line at that point is undefined or approaches infinity, indicating a very steep or vertical incline on the graph of the function.
How does the function y = x^2 illustrate the concept of differentiability?
-The function y = x^2 is differentiable everywhere on its domain because for every point on the curve, there exists a unique tangent line with a well-defined slope, which is the geometric interpretation of the derivative.
What is the formula for the unit circle with center at the origin?
-The formula for the unit circle with center at the origin is x^2 + y^2 = 1. This equation represents all the points (x, y) that are at a distance of 1 from the origin.
Outlines
📐 Understanding Continuity and Differentiability
This paragraph introduces the concepts of continuity and differentiability, two fundamental topics in calculus. Continuity is described informally as the ability to draw a function without lifting the pen, with discontinuities being points where the pen must be lifted. The formal definition of continuity at a point is given, emphasizing that the function must be defined at that point, the two-sided limit must exist, and the limit must equal the function's value at that point. Discontinuities are also explained, followed by a discussion on continuity from the left and right, particularly relevant for endpoints of a closed interval. The paragraph concludes with a definition of continuity on open and closed intervals.
🔴 Continuity and the Unit Circle Example
The unit circle is used as an example to illustrate the concept of continuity. The formula for the unit circle, x^2 + y^2 = 1, is given, and solved explicitly for y to create a function y = sqrt(1 - x^2). This function is shown to be continuous on the closed interval between -1 and 1, as it can be drawn without lifting the pen and satisfies the conditions for continuity at the endpoints. The paragraph also touches on differentiability, stating that a function is differentiable if its derivative can be taken, and not differentiable otherwise, with examples of points where differentiability does not hold.
✏️ Non-Differentiable Functions and Their Characteristics
This section delves into non-differentiable functions, highlighting that a function is not differentiable at a point if it is undefined there or appears 'pointy'. The absolute value function is used to demonstrate a pointy function, which is not differentiable at the origin. The concept of a tangent line and its relation to derivatives is reviewed, with an emphasis on the slope of the tangent line representing the derivative. The example of the absolute value function shows that there is no single slope for a tangent line at the origin, indicating non-differentiability. Additionally, functions with vertical tangent lines, such as the cube root function at x = 0, are also deemed non-differentiable due to the undefined nature of their derivatives at those points.
🔄 Differentiability Implies Continuity, But Not Vice Versa
The final paragraph clarifies the relationship between differentiability and continuity. It states that if a function is differentiable at a point, it is also continuous at that point, but the converse is not necessarily true. There can be continuous functions that are not differentiable, as demonstrated by the examples of the red curve and the absolute value function. The red curve is continuous everywhere but not differentiable at x = 0, while the absolute value function is continuous everywhere but not differentiable at the origin. This distinction is crucial for understanding the separate concepts and their implications in calculus.
Mindmap
Keywords
💡Continuity
💡Differentiability
💡Discontinuity
💡Limit
💡Derivative
💡Tangent Line
💡Closed Interval
💡Open Interval
💡Domain
💡Square Root
💡Absolute Value
Highlights
A function is considered continuous if it can be drawn without lifting the pen off the paper.
A point where the pen must be lifted while drawing a function is called a discontinuity.
Continuity at a point x=c is defined by three requirements: the function is defined at x=c, the two-sided limit exists as x approaches c, and the limit equals the function value at x=c.
If a function is not continuous at x=c, it has a discontinuity at that point.
Continuity from the left and right is a concept useful for discussing endpoints of a closed interval.
One-sided continuity is easier to attain than two-sided continuity as it involves only left-sided or right-sided limits.
A function is continuous on an open interval if it is continuous at every point within that interval.
For a function to be continuous on a closed interval, it must be continuous on the open interval and also at the endpoints from the left and right.
The unit circle can be defined by the equation x^2 + y^2 = 1, and solving for y gives y = sqrt(1 - x^2).
The domain of the function y = sqrt(1 - x^2) is the closed interval between -1 and 1.
The function y = sqrt(1 - x^2) is continuous on the closed interval from -1 to 1.
Differentiability of a function means that its derivative can be taken; if not, the function is not differentiable.
A function is not differentiable at a point if it is undefined there.
Functions that appear pointy at some point, such as |x| at the origin, are not differentiable at that point.
The derivative represents the slope of the tangent line to a function, and a function is differentiable if a tangent line can be drawn.
A function with a vertical tangent line at a point, like the cube root function at x=0, is not differentiable at that point.
Differentiability implies continuity, but the converse is not true; a continuous function may not be differentiable.
The cube root function has a well-defined value at x=0 but is not differentiable at that point due to an undefined derivative.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: