AP Calculus AB: Lesson 2.5 Differentiability

Michelle Krummel

4 Oct 202044:12

EducationalLearning

32 Likes 10 Comments

TLDRThis lesson delves into the concepts of continuity and differentiability, guiding students through the process of determining whether a function is differentiable at a specific point. It reviews the three-part definition of continuity and the formal definition of differentiability, emphasizing the importance of the function's existence, limit, and equality at a point. The instructor uses graphical examples to illustrate key concepts such as corner points, cusps, and vertical tangents, which indicate a function's non-differentiability. The lesson concludes with examples to practice determining continuity and differentiability for piecewise functions, highlighting the necessity for both conditions to be met for a function to be considered differentiable.

Takeaways

📘 Continuity and differentiability are fundamental concepts in calculus that determine the behavior of functions at specific points.
🔍 To establish if a function is continuous at a point x=a, three conditions must be met: f(a) must exist, the limit as x approaches a of f(x) must exist, and these two values must be equal.
📌 A function is considered differentiable at a point x=a if the derivative f'(a) exists, which means the slope of the function at that point is well-defined.
🤔 Differentiability implies local linearity, meaning that if you zoom in close enough on the graph at x=a, it should appear as a straight line, indicating a linear behavior.
🚫 A function is not differentiable at a point if there is a discontinuity, a corner point, a cusp, or a vertical tangent at that point.
📈 The script provides examples of functions and analyzes their continuity and differentiability at specific points, such as absolute value functions and piecewise functions.
📊 The concept of a removable discontinuity is introduced, where if f(a) exists but the limit as x approaches a does not equal f(a), it indicates a hole in the graph and the function is not continuous at x=a.
🤓 The importance of checking both the left and right limits and the value of the function at x=a is emphasized for determining continuity.
🧩 The script also covers the process of finding the derivative of a function, which is necessary for determining differentiability, and explains the steps involved in finding the limit of the derivative as x approaches a specific point.
📚 The lesson concludes with examples that require solving systems of equations to find specific values of constants that make a function both continuous and differentiable at a given point.
🔑 The takeaway that if a function is differentiable at a point, it must also be continuous at that point, as differentiability implies continuity but not the other way around.

Q & A

What is the definition of continuity for a function at a point x equals a?
-A function f(x) is continuous at a point x equals a if three conditions are met: f(a) is defined, the limit as x approaches a of f(x) exists, and the limit as x approaches a of f(x) equals f(a).
Why is it necessary for the limit of f(x) as x approaches a to equal f(a) for the function to be continuous at that point?
-If the limit of f(x) as x approaches a does not equal f(a), it indicates a removable discontinuity at that point, meaning the function is not continuous there.
What does it mean for a function to be differentiable at a point x equals a?
-A function is differentiable at x equals a if the derivative f'(a) exists, which implies that the limit as h approaches 0 of [f(a+h) - f(a)] / h exists, or equivalently, the left and right limits of f'(x) as x approaches a are equal and finite.
Why is a function considered not differentiable at a corner point?
-A function is not differentiable at a corner point because the slopes approaching the point from the left and right are not equal, meaning the limit of the derivative does not exist.
What is the relationship between differentiability and local linearity of a function?
-A function is differentiable at a point if it is locally linear near that point, which means if you zoom in close enough, the graph around that point appears linear, including having a well-defined slope.
How can you determine if a function is not differentiable at a point without calculating the derivative?
-You can determine a function is not differentiable at a point by observing the graph for features such as corner points, cusps, vertical tangents, or discontinuities, as any of these indicate the function is not locally linear and thus not differentiable.
What is the significance of the limit of the derivative of a function as x approaches a in determining differentiability?
-The limit of the derivative as x approaches a must exist and be finite for the function to be differentiable at that point. If the limit does not exist or is infinite, the function is not differentiable there.
Can a function be differentiable at a point without being continuous at that point?
-No, a function cannot be differentiable without being continuous at that point. Differentiability implies continuity, but not vice versa.
What is a cusp and how does it relate to differentiability?
-A cusp is a point on a graph where the slope approaches infinity or negative infinity as x approaches that point from both the left and right. A function is not differentiable at a cusp because it is not locally linear, and the limit of the derivative does not exist.
How does a vertical tangent affect the differentiability of a function at a point?
-A vertical tangent indicates an infinite slope at a particular point. If a function has a vertical tangent at a point, it is not differentiable at that point because the derivative does not exist and the function is not locally linear.

Outlines

00:00

📚 Introduction to Continuity and Differentiability

This paragraph introduces the concepts of continuity and differentiability. It explains the three-part definition of continuity for a function f(x) at a point x=a, which includes the existence of f(a), the limit of f(x) as x approaches a, and the equality of these two. The paragraph also distinguishes between continuity and differentiability, noting that differentiability implies the existence of a derivative at a point, which is further defined through a limit process. The importance of the slope of the function at a point, and the concept of local linearity as a characteristic of differentiable functions, are highlighted.

05:02

🔍 Analyzing Graphs for Continuity and Differentiability

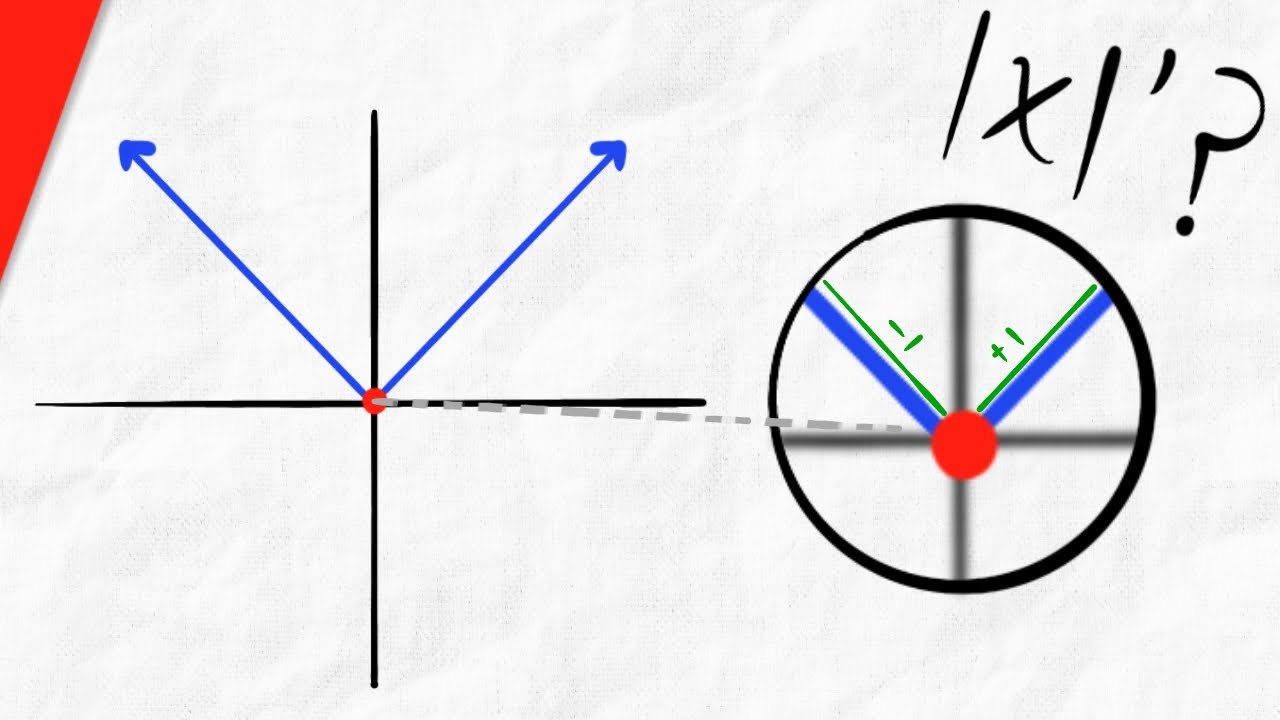
The second paragraph delves into the practical analysis of graphs to determine if a function is continuous or differentiable at specific points. It uses the graph of an absolute value function as an example, demonstrating how to check for continuity by ensuring the function is defined and the limit exists and equals the function's value at that point. The paragraph also discusses differentiability, explaining that a function is not differentiable at a point if the slopes from the left and right do not match or if the function is not locally linear, such as at a corner point.

10:05

📉 Examining Functions with Cusps and Discontinuities

This paragraph examines the properties of functions with cusps and discontinuities, explaining why they are not differentiable. It contrasts a cusp, where the slope approaches infinity or negative infinity, with a corner point, where the slopes differ on either side but do not approach vertical. The importance of checking the left and right limits of the derivative and the function's value at a point is emphasized to determine continuity and differentiability, with examples illustrating the presence of discontinuities and the absence of locally linear behavior.

15:06

🚫 Points of Non-differentiability: Corners, Cusps, and Discontinuities

The fourth paragraph summarizes the conditions under which a function is not differentiable, such as at points of discontinuity, corners, cusps, and vertical tangency. It stresses that continuity is a prerequisite for differentiability, and discontinuities of any kind immediately rule out differentiability. The paragraph provides a methodical approach to evaluating the differentiability of a function by checking for these conditions at specific points on a graph.

20:06

🤔 Continuity and Differentiability at Specific Points on a Graph

This paragraph challenges the viewer to analyze specific points on a graph to determine if the function is continuous and differentiable at those points. It provides a list of x-values and asks the viewer to consider the nature of the function at each point, whether it is continuous but not differentiable, differentiable but not continuous, or neither. The paragraph also clarifies that a function cannot be differentiable without being continuous, highlighting the impossibility of a function being differentiable at a point of discontinuity.

25:10

📝 Applying Continuity and Differentiability to Piecewise Functions

The sixth paragraph applies the concepts of continuity and differentiability to piecewise functions, using a specific function g(x) as an example. It demonstrates how to check for continuity at a point where the domain changes by ensuring the function's value and the limit from both sides are equal. The paragraph also shows the process of finding the derivative of a piecewise function and checking if the left and right limits of the derivative exist and are equal to determine differentiability.

30:13

🧩 Solving for Parameters in Piecewise Functions for Differentiability

The seventh paragraph involves solving for specific parameters in a piecewise function to ensure it is both continuous and differentiable at a certain point. It sets up a system of equations based on the conditions for continuity and differentiability, involving finding the function's value and the left and right limits of the derivative. The paragraph guides through solving these equations to find the values of the parameters that make the function satisfy the desired properties.

35:14

🔚 Concluding the Lesson with Continuity and Differentiability

The final paragraph wraps up the lesson on continuity and differentiability by summarizing the key points and providing a brief overview of the next topic. It reiterates the importance of continuity for differentiability and provides a quick review of the conditions required for a function to be continuous and differentiable. The paragraph ends with a teaser for the upcoming lesson on tangent line approximations.

Mindmap

Keywords

💡Continuity

Continuity in the context of the video refers to a property of a function where the limit of the function as the input approaches a certain value is equal to the value of the function at that point. It's a fundamental concept in calculus that ensures the function is unbroken at a given point. The script emphasizes the 'three-part definition' of continuity, which includes the existence of the function's value at a point, the existence of the limit as x approaches that point, and the equality of these two values. For instance, the script uses the function f(x) to explain that for continuity at x=a, f(a) must be defined, the limit as x approaches a must exist, and the limit must equal f(a).

💡Differentiability

Differentiability is the property of a function that allows it to have a derivative at a certain point. The script explains that if a function is differentiable at a point, it means that its derivative exists at that point, indicating the function has a well-defined slope or rate of change. The video connects differentiability with the existence of a function's derivative, symbolized as f'(a), and the formal definition involving the limit of (f(a+h) - f(a))/h as h approaches 0. It also mentions that differentiability implies the function is locally linear at the point of interest.

💡Removable Discontinuity

A removable discontinuity occurs at a point on a graph where the function is not defined or the limit exists but is not equal to the function's value at that point, yet the discontinuity can be 'removed' by redefining the function's value at that point. The video script illustrates this with an example where the function's value and the limit as x approaches a do not match, indicating a removable discontinuity, which prevents the function from being continuous at that point.

💡Locally Linear

Locally linear is a concept that describes a function that, when examined very closely around a certain point, appears to be a straight line. The video uses this term to describe differentiable functions, explaining that if you zoom in far enough on the function at the point of interest, it should look like a linear function, specifically like its tangent line. This property is key to understanding differentiability, as a function that is not locally linear at a point is not differentiable there.

💡Derivative

The derivative of a function at a certain point is a measure of the rate at which the function's value changes with respect to changes in its input. In the video, the derivative is introduced as a key component of differentiability, with the notation f'(a) representing the derivative of the function f at the point a. The existence of the derivative is synonymous with the function being differentiable at that point, as it indicates the function has a well-defined slope at a.

💡Limit

In the context of the video, a limit is the value that a function's output approaches as the input approaches a certain value. The script discusses the limit in relation to both continuity and differentiability, explaining that for a function to be continuous at x=a, the limit of the function as x approaches a must exist and be equal to the function's value at a. Similarly, for differentiability, the limit of the difference quotient as h approaches 0 must exist.

💡Slope

Slope in the video refers to the steepness or gradient of a function at a particular point, which is a measure of how much the function increases or decreases at that point. The concept is central to the discussion of differentiability, as the existence of a slope at a point (i.e., the derivative) indicates that the function is differentiable there. The script mentions that for a function to be differentiable, the slope must exist and be finite, not approaching infinity or negative infinity.

💡Tangent Line

A tangent line is a straight line that touches a curve at a single point without crossing it. In the video, the concept of a tangent line is used to illustrate the idea of a function being locally linear at a point, which is a characteristic of differentiability. The script explains that if you zoom in on a function at a certain point, the graph should appear indistinguishable from its tangent line at that point, indicating differentiability.

💡Corner Point

A corner point is a type of discontinuity where the function has different slopes on either side of the point, creating a 'corner' or 'kink' in the graph. The script describes a corner point as a place where the function is not differentiable because it does not have a well-defined slope at that point. The function's behavior changes abruptly, and thus it is not locally linear, which is a requirement for differentiability.

💡Cusp

A cusp is a type of point on a curve where the curve has a vertical tangent and the slope of the curve becomes infinite or negative infinity as it approaches the cusp from both sides. The video script uses the term to describe a specific kind of discontinuity where the function is not differentiable because the slope is not defined (approaches infinity). The cusp represents a sharp turn in the graph where the function's behavior changes very rapidly.

💡Vertical Tangent

A vertical tangent is a tangent line to a curve that is vertical, indicating that the function has an infinite slope or derivative at that point. The script mentions vertical tangents in the context of points where the function is not differentiable, as the limit of the derivative does not exist (approaches infinity). The presence of a vertical tangent signifies a sharp turn upwards or downwards in the graph of the function.

Highlights

Lesson focuses on understanding continuity and differentiability in functions and determining if a function is differentiable at a given point.

Three-part definition of continuity explained: function must be defined at a point, limit exists as x approaches the point, and the limit equals the function's value at that point.

Differentiability defined as the existence of a derivative at a point, indicating the function's slope is defined at that point.

Formal definition of differentiability involves the limit of the difference quotient as h approaches 0, which defines the derivative.

Differentiability requires the limit of the derivative from the left and right to be equal and finite, indicating the function's behavior is consistent at the point.

Differentiable functions are described as locally linear, meaning their behavior near a point resembles a straight line when zoomed in closely.

Examples provided to illustrate the concepts, including functions with corner points, cusps, and vertical tangents, which are not differentiable.

Function with an absolute value shifted up is analyzed for continuity and differentiability, showing the function is continuous but not differentiable at x=0 due to differing slopes on either side.

A function with a cusp at x=0 is discussed, explaining why the function is not differentiable due to the slope approaching infinity.

A function with a vertical tangent at x=0 is shown to be continuous but not differentiable because the slope is undefined.

Function with a jump discontinuity at x=12 is identified as neither continuous nor differentiable due to the lack of a left-hand limit.

Exploration of a piecewise function's continuity and differentiability at the point where the domain splits, emphasizing the need for equal left and right limits.

Demonstration of finding the derivative of a piecewise function using the limit definition, showcasing the process for the first subdomain.

Differentiability of a function requires the existence of the derivative's limit as x approaches a certain point, which must be consistent from both sides.

Solving a system of equations to find values of k and m that make a function both continuous and differentiable at x=3.

Final example involves finding values of a and b for a function to be differentiable at x=1, using the principles of continuity and differentiability.

Lesson concludes with a summary emphasizing that a function must be continuous to be differentiable, and the process for determining this involves checking the function's value, limit, and slope at the point in question.

Transcripts

Browse More Related Video

BusCalc 16 Continuity and Differentiability

Differentiability (Formal Definition)

Smoothing a Piece-wise Function | MIT 18.01SC Single Variable Calculus, Fall 2010

Continuity and Differentiability EXPLAINED with Examples

Continuity and Differentiability

|x| is Continuous but not Differentiable | Calculus 1 Exercises

Related Tags

Continuity Differentiability Function Analysis Math Education Derivative Definition Limit Concept Tangent Lines Graph Analysis Piecewise Functions Calculus Lessons