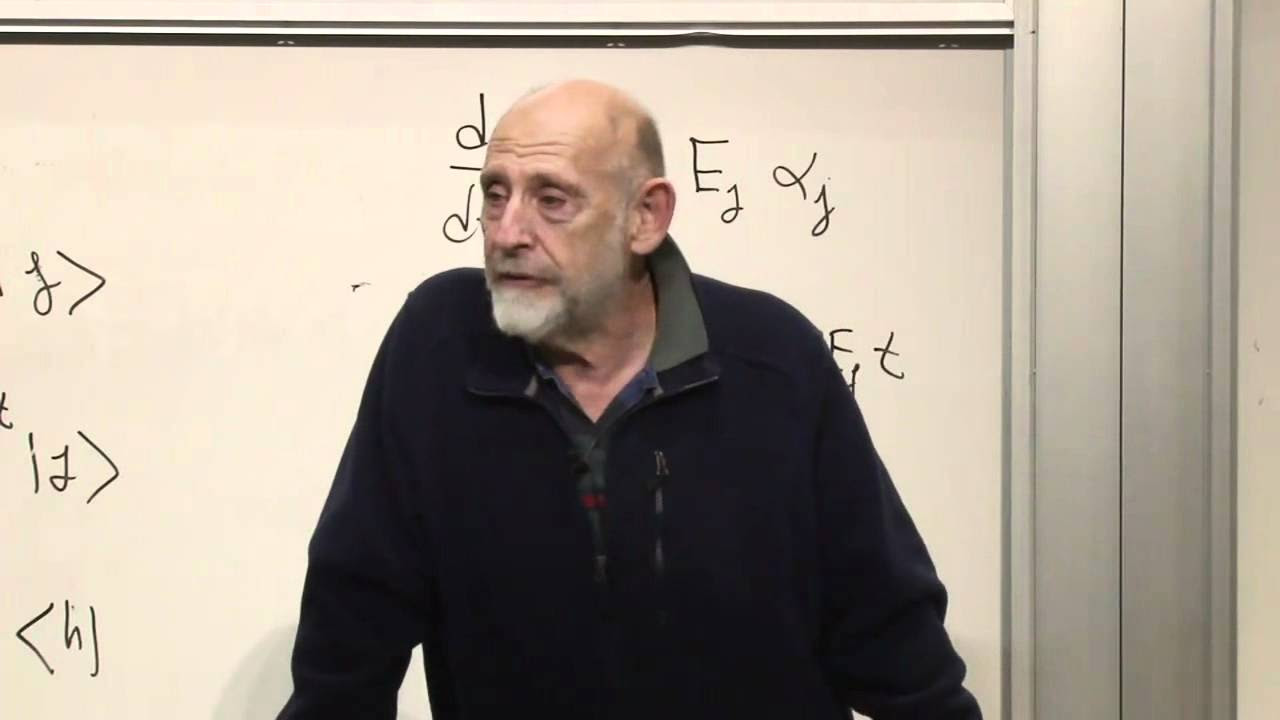Classical Mechanics | Lecture 6
TLDRThe video script presents a detailed exploration of classical mechanics, focusing on the Hamiltonian formulation as a powerful tool for understanding the dynamics of systems. It discusses the concept of generalized coordinates and the Lagrangian (Lran), emphasizing the transition from Newton's equations to the Hamiltonian equations of motion. The lecturer illustrates the process of deriving the Hamiltonian for a harmonic oscillator and explains how these equations describe motion in phase space. The script also touches on the significance of the Hamiltonian in quantum mechanics and the importance of understanding the interplay between quantum mechanics and gravity, hinting at the frontier problems in modern physics such as quantum gravity, dark energy, and the structure of cosmology.
Takeaways
- 📚 The power of Lagrangian mechanics is showcased for solving simple systems and problems, with the emphasis on finding the equations of motion as the key to solving mechanical problems.
- 📐 Two examples are discussed: a double pendulum and a wedge on a frictionless plane with a block sliding up and down, highlighting the similarities and the mechanical approach to solving these problems.
- 🔍 The importance of choosing a set of coordinates to describe the system's motion is emphasized, with the choice not being unique but essential for encoding the system's instantaneous state.
- 📈 The process of writing down the Lagrangian (T - V) and finding the kinetic and potential energy of the system is outlined, leading to the derivation of the equations of motion.
- 🔁 The concept of conservation of momentum is introduced, showing how symmetries in a system can lead to conserved quantities, which are crucial for understanding the system's behavior.
- 🌀 The Hamiltonian formulation of mechanics is introduced as a distinct and powerful approach, focusing on the phase space of positions (q's) and momenta (p's), and how it relates to the conservation of energy.
- ⚙️ Hamilton's equations of motion are derived, showing a direct relationship between the change in the Hamiltonian and the dynamics of the system in phase space.
- 🔢 The units of various quantities in the Hamiltonian formulation are discussed, emphasizing the abstract nature of some variables and the importance of dimensional consistency.
- 🔄 The geometric interpretation of Hamilton's equations is highlighted, showing how they describe the motion of points in phase space, which can be visualized as fluid rotating with a constant velocity.
- 🚫 The script touches on the limitations of certain Lagrangians that cannot be solved for velocities in terms of momenta, indicating that they are not considered legitimate for the Hamiltonian formulation.
- ➡️ The Hamiltonian is identified as a central object in quantum mechanics, suggesting that the study of classical mechanics is a stepping stone towards understanding modern physics, including quantum theory and relativity.
Q & A
What is the primary focus of the Hamiltonian formulation of classical mechanics?
-The primary focus of the Hamiltonian formulation is on the generalized coordinates (q's) and their conjugate momenta (p's), rather than the velocities (Q dots). This approach allows for a more geometrical representation of the system's dynamics in phase space.
Why is it important to choose a good set of coordinates when describing a mechanical system?
-A good set of coordinates simplifies the description of the system's motion and makes the equations of motion easier to solve. The choice of coordinates can greatly affect the complexity of the resulting equations and the ease with which the system's dynamics can be understood.
What is the significance of the Hamiltonian being equal to the total energy of the system?
-The Hamiltonian being equal to the total energy is significant because it is a conserved quantity in the absence of non-conservative forces. This conservation property is fundamental in the analysis of the system's dynamics and is a key feature of the Hamiltonian formulation.
How does the Hamiltonian formulation relate to the principle of least action?
-The Hamiltonian formulation is derived from the principle of least action, which states that the path taken by a system between two points in its configuration space is the one that minimizes the action, a quantity related to the system's Lagrangian. Both formulations are equivalent ways of describing classical mechanics.
What does the Hamiltonian formulation reveal about the motion of a harmonic oscillator in phase space?
-The Hamiltonian formulation reveals that the motion of a harmonic oscillator in phase space is confined to a circle (or an ellipse in the case of a non-ideal oscillator), with the radius of the circle corresponding to the total energy of the system. This circular motion represents the conservation of energy in the system.
What is the relationship between the Hamiltonian equations of motion and the conservation laws?
-The Hamiltonian equations of motion are derived from the conservation laws associated with the system's symmetries. Specifically, the conservation of energy and angular momentum are reflected in the Hamiltonian equations, which describe how the system's coordinates and momenta evolve over time.
How does the Hamiltonian formulation differ from Newton's equations in terms of the order of the differential equations involved?
-The Hamiltonian formulation involves first-order differential equations, which are derived from the Hamiltonian function with respect to the generalized coordinates and their conjugate momenta. In contrast, Newton's equations are second-order differential equations that directly relate the acceleration of a particle to the forces acting on it.
What is the significance of the term 'cyclic coordinate' in the context of the Hamiltonian formulation?
-A cyclic coordinate is one that does not explicitly appear in the Hamiltonian, meaning its time derivative also does not appear. This leads to the conservation of the corresponding conjugate momentum, which is a direct consequence of the system's symmetry associated with that coordinate.
Why is the Hamiltonian formulation considered more powerful or preferable in certain contexts?
-The Hamiltonian formulation is considered more powerful because it provides a clear geometric interpretation of the system's dynamics in phase space, allows for a more straightforward identification of conserved quantities, and is more naturally extended to the quantum mechanical framework, which is essential for modern physics.
What is the role of the potential energy in the Hamiltonian formulation?
-In the Hamiltonian formulation, the potential energy appears as part of the total energy of the system and contributes to the Hamiltonian function. It is used to describe the forces acting within the system and, when the forces are conservative, the potential energy is the integral of these forces.
How does the Hamiltonian formulation help in understanding the dynamics of a system with constraints?
-The Hamiltonian formulation naturally incorporates constraints by focusing on the generalized coordinates and their conjugate momenta, which can be defined in a way that automatically satisfies the constraints. This makes it a powerful tool for analyzing systems with complex constraints or non-Cartesian motions.
Outlines
😀 Introduction to Lagrangian Mechanics
The speaker begins by introducing the power of Lagrangian mechanics for solving simple systems and problems. They discuss the recommendation to study the double pendulum and a wedge on a frictionless plane, both of which are interesting and related problems. The speaker emphasizes that finding the equations of motion is the key to solving mechanical problems, and this can be achieved without necessarily solving the equations themselves. They also mention that the problem can be numerically solved using a computer. The first example presented is a simple problem involving an inclined wedge and a point particle sliding on it.
🔍 Assigning Coordinates and Describing Motion
The speaker explains the importance of assigning a set of coordinates to describe the motion of the system. They choose two generalized coordinates for the example of the wedge and the particle, which are the horizontal coordinates of the corner of the wedge and the position of the particle relative to the corner. The speaker also discusses the kinetic energy of the system, which includes the energy of the moving wedge, the particle, and the potential energy due to gravity acting on the particle.
📐 Deriving the Lagrangian and Conservation Laws
The speaker outlines the process of deriving the Lagrangian (Lran) for the system, which involves calculating the kinetic energy and potential energy. They then discuss the derivation of the equations of motion using the Lagrangian and the concept of conservation of momentum. The speaker highlights that the X-component of momentum is conserved in the system due to translational symmetry.
🌀 Symmetry and Conserved Quantities
The speaker explores the concept of symmetry in the system and how it leads to conserved quantities. They explain that due to the translation symmetry of the system, there is a conserved quantity, which is the X-component of momentum. The speaker also discusses the definition of momentum in the context of the abstract mathematical system of classical mechanics and how the conservation laws can be applied to solve the system.
🔄 Angular Coordinates and the Double Pendulum
The speaker moves on to discuss the double pendulum problem, emphasizing the choice of angular coordinates for describing the system. They explain the choice of using the angle of the first pendulum relative to the horizontal and the angle of the second pendulum relative to the first one. The speaker also touches upon the rotational symmetry of the system in the absence of a gravitational field.
🧲 Torque and Angular Momentum in the Double Pendulum
The speaker delves into the concept of torque and how it affects the motion of the double pendulum. They explain that the forces exerted by the pendulum rods on each other create a net torque, which in turn affects the angular acceleration of the pendulum. The speaker clarifies that while the angular momentum is conserved, the individual angular velocities of the pendulum parts are not.
🚀 Hamiltonian Formulation and Energy Conservation
The speaker introduces the Hamiltonian formulation of mechanics, emphasizing its significance as a representation of energy and its conservation. They discuss the transition from Newton's equations to the principle of least action and then to the Hamiltonian formulation. The speaker also addresses the concept of bad laws in dynamics and how Hamiltonian physics forbids certain types of dynamics that are not physically allowable.
🔄 Hamilton's Equations and Phase Space
The speaker derives Hamilton's equations of motion and explains their significance in terms of the phase space of a system. They illustrate how the Hamiltonian focuses on the generalized coordinates (q's) and their conjugate momenta (p's), and how these quantities define the state of a mechanical system. The speaker also discusses the symmetry between q's and p's in the Hamiltonian formulation and how it differs from the Lagrangian formulation.
📐 Units and Coordinate Transformations
The speaker addresses a question regarding the units of variables in the context of the Lagrangian and Hamiltonian mechanics. They clarify that after a change of variables, the units of the generalized coordinate q are no longer those of length. The speaker also discusses the units of momentum and how they relate to the generalized coordinates and their velocities.
🔗 Relating Lagrangian and Hamiltonian Equations
The speaker explains the relationship between the Lagrangian and Hamiltonian equations, showing how two first-order Hamiltonian equations can be combined to form a single second-order equation, which is the familiar form of Newton's second law (F = ma). They also discuss the role of the Hamiltonian in quantum mechanics and its importance in modern physics, particularly in the unification of quantum mechanics and gravity.
🎓 Modern Physics and Theoretical Challenges
The speaker concludes by reiterating the goal of the course, which is to prepare students to understand the frontier problems in modern physics. They mention areas such as quantum gravity, dark energy, cosmology, elementary particle physics, and string theory as some of the significant challenges in theoretical physics. The speaker expresses optimism about the potential for future discoveries and advancements in these areas.
Mindmap
Keywords
💡Lagrangian Mechanics
💡Generalized Coordinates
💡Conservation Laws
💡Hamiltonian Mechanics
💡Canonical Momenta
💡Cyclic Coordinates
💡Double Pendulum
💡Equations of Motion
💡Symmetry
💡Hamilton's Equations
💡Phase Space
Highlights
The power of Lagrangian mechanics for solving simple systems is demonstrated through two similar problems: a double pendulum and a wedge moving on a frictionless plane with a block sliding up and down.
The importance of choosing a set of coordinates to describe the motion of the system is emphasized, with two coordinates being sufficient for the given problem.
The process of writing down the Lagrangian (T - V) and finding the kinetic energy of the system is explained, including the distinction between the velocity of the block and the particle.
The potential energy in the system is assumed to be due to gravitational force acting vertically, with a specific example of the particle's altitude changing and its potential energy being X.
Hamilton's principle is introduced as a method for deriving the equations of motion, with an example of how to work out the conservation of momentum for the system.
The concept of symmetry in the system is discussed, leading to the identification of a conserved quantity, which in this case is the X component of momentum.
The Lagrangian and Hamiltonian formulations of mechanics are contrasted, with the Hamiltonian being described as a more powerful and compact way to handle complex systems.
The Hamiltonian is derived for a harmonic oscillator, showcasing how to express the Hamiltonian in terms of generalized coordinates and momenta.
Hamilton's equations of motion are derived, highlighting the symmetry between the coordinates (q's) and momenta (p's) and their role in the Hamiltonian formulation.
The motion of a harmonic oscillator in phase space is visualized as circular trajectories, illustrating the conservation of energy in the system.
The Hamiltonian is defined as the conserved energy of the system and is central to the Hamiltonian formulation of classical mechanics.
The change of variables in the Lagrangian is discussed to simplify the Hamiltonian and to illustrate the symmetric nature of the system.
The units of the generalized coordinate (q) and the Hamiltonian are discussed, noting that q does not have units of length after the change of variables.
The Hamiltonian formulation is emphasized as a prerequisite for understanding quantum mechanics and modern physics, particularly the unification of quantum mechanics and gravity.
The potential energy is discussed in the context of conservative forces, with the explanation that forces are the derivatives of potential energy.
The importance of understanding the Hamiltonian in the context of quantum mechanics is stressed, as it has a more intuitive meaning there compared to classical mechanics.
The ultimate goal of the course is to prepare students to understand the frontier problems in physics, such as quantum gravity, dark energy, and the structure of cosmology.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: