Optimization Calculus 1 - 2 Problems
TLDRThe video script delves into the concept of optimization, focusing on the practical application of finding maximums and minimums to solve real-world problems. The presenter outlines a three-step process for optimization: identifying two key equations from the problem, combining these equations through substitution and simplification, and then differentiating the resulting equation to find critical points where the derivative is zero. The script illustrates this process with two examples: maximizing the product of two variables with a given sum and minimizing the amount of fencing needed for a rectangular pasture next to a river. By following the optimization steps, the presenter demonstrates how calculus can be used to find the most efficient solutions to such problems, emphasizing the importance of setting derivatives to zero to identify maximum or minimum values.
Takeaways
- 📐 **Optimization Concept**: The process of using calculus to find maximum or minimum values, which can be applied to real-world problems to achieve the best outcomes.
- 🔍 **Identifying Equations**: In optimization problems, the first step is to identify two key equations from the problem statement.
- 🔗 **Equation Integration**: The second step involves substituting one equation into the other to simplify and reduce the variables to two, which is necessary for differentiation.
- 📉 **Derivative Utilization**: Taking the derivative of the simplified equation and setting it equal to zero is the third step, which helps find critical points that represent maximum or minimum values.
- 🔑 **Product and Sum Equations**: An example given is to find two variables whose product is 192 and whose sum is at a minimum, which requires solving a system of equations.
- 🛠️ **Real-world Application**: Optimization is not just theoretical; it's applicable to practical scenarios like minimizing material costs for construction, managing finances, or planning logistics.
- 🏞️ **Fencing a Pasture**: A specific example involves a dairy farmer who wants to fence a pasture with the least amount of fencing material while utilizing the river as a natural boundary.
- 📏 **Perimeter and Area**: For the pasture problem, the area must equal 180 square meters, and the perimeter (amount of fencing) is what needs to be minimized.
- 🧮 **Solving for Dimensions**: The solution to the pasture problem involves solving for the dimensions that minimize the perimeter, given the area constraint.
- 📈 **Derivative to Find Minimum**: By setting the derivative of the perimeter equation with respect to one variable to zero, the minimum value for that variable is found, which in turn helps find the other variable.
- 🎯 **Critical Points**: The critical points found by setting the derivative to zero are potential locations for maximum or minimum values, which are essential in optimization problems.
- 📝 **Problem-Solving Steps**: The script outlines a straightforward three-step process for optimization problems: identify equations, integrate and simplify, and apply calculus to find optimal values.
Q & A
What is the main concept discussed in the transcript?
-The main concept discussed in the transcript is optimization, which involves using calculus to find maximum or minimum values to solve real-world problems.
What are the three steps to perform optimization as mentioned in the transcript?
-The three steps to perform optimization are: 1) Find two equations from the problem statement, 2) Plug one equation into the other and simplify, and 3) Take the derivative of the new equation and set it equal to zero, then solve.
How does the concept of optimization relate to building a deck?
-In the context of building a deck, optimization is about finding the right amount of material to minimize costs without underbuying or overbuying, ensuring the deck is completed as intended without waste.
What does the transcript suggest as the method to find the dimensions of a rectangular pasture that requires the least amount of fencing next to a river?
-The method involves setting up two equations: one for the area of the pasture (which must equal 180 square meters) and one for the perimeter (which represents the amount of fencing needed). By solving these equations using optimization techniques, one can find the dimensions that minimize the perimeter.
What is the significance of setting the derivative equal to zero in the optimization process?
-Setting the derivative equal to zero is significant because it helps in finding the critical points where the function could have a maximum or minimum value. These points are potential solutions for optimization problems.
How does the transcript use the concept of optimization to solve the problem of feeding employees while maximizing profits?
-The transcript suggests that by using optimization, one can find the best equation or method to balance the costs of feeding employees with the profits made, aiming to maximize profit while minimizing costs.
What is the role of calculus in finding maximum and minimum values in optimization problems?
-Calculus, specifically the process of taking derivatives, is used to determine the slope or rate of change of a function. Setting these derivatives equal to zero helps identify points where the function may reach a maximum or minimum, which are the optimal solutions in the context of optimization.
How does the transcript demonstrate the application of optimization in planning a party?
-The transcript uses the example of buying food for a party to demonstrate optimization. The goal is to neither overbuy, leading to wasted food, nor underbuy, risking not having enough for the guests. By using optimization, one can calculate the right amount of food to purchase.
What is the first step in the optimization process when dealing with a problem that has constraints?
-The first step is to identify and set up two equations based on the constraints and goals of the problem. These equations represent different aspects of the problem that need to be optimized.
In the context of the dairy farmer's problem, why is it unnecessary to consider fencing along the river?
-It is unnecessary to consider fencing along the river because the problem statement specifies that the river acts as a natural boundary, eliminating the need for fencing on that side.
What is the牧场 (pasture) area that the dairy farmer needs to enclose, according to the transcript?
-The pasture area that the dairy farmer needs to enclose is 180 square meters.
How does the transcript illustrate the process of solving for the minimum sum of two variables x and y, given their product is 192?
-The transcript illustrates this by first setting up two equations based on the given conditions: the first being x*y = 192, and the second being x + y = m (where m represents the minimum sum). It then shows how to solve for one variable in terms of the other from the first equation and substitute it into the second equation. After simplification and differentiation, setting the derivative equal to zero helps find the values of x and y that minimize the sum.
Outlines
📈 Introduction to Optimization
The video begins with an introduction to the concept of optimization, which involves finding local maximums or minimums to solve real-world problems. The speaker uses examples like building a deck, managing finances, and feeding employees to illustrate the need to balance costs and benefits. The process of optimization is outlined in three steps: identifying two equations from the problem, plugging one equation into the other and simplifying, and then taking the derivative of the resulting equation and solving for zero to find the optimal point.
🔍 Solving the Product and Sum Optimization Problem
The speaker presents a basic optimization problem where the product of two variables is 192 and their sum is to be minimized. Two equations are formulated from the problem statement: x*y = 192 and x + y = m (minimum sum). To solve, the first equation is solved for x, resulting in x = 192/y, which is then substituted into the second equation to eliminate one variable. The resulting equation is simplified to m = 192y^-1 + y. The derivative of this equation with respect to y is taken, set to zero, and solved to find the value of y that minimizes the sum. The corresponding value of x is then found to complete the solution.
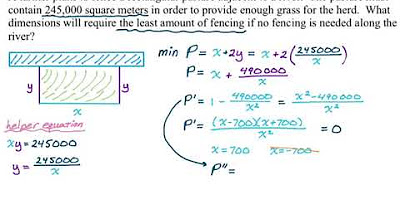
🏡 Optimizing a Rectangular Pasture Along a River
The next problem involves a dairy farmer who wants to fence in a rectangular pasture next to a river, with the area of the pasture being 180 square meters. The goal is to find the dimensions that require the least amount of fencing, with the river side not needing a fence. The area is represented as base times height (A = b*h), and the perimeter, which represents the amount of fencing needed, is represented as 2b + h. The area equation is solved for b, and this expression is substituted into the perimeter equation to create a single-variable equation. The derivative of this equation with respect to h is taken, set to zero, and solved to find the value of h that minimizes the fencing needed. The corresponding value of b is then calculated.
📏 Deriving the Dimensions for Minimum Fencing
The final paragraph details the mathematical steps to find the value of h (height) that minimizes the fencing required for the pasture. The derivative of the perimeter equation with respect to h is calculated, set to zero, and solved, leading to h being the square root of 360,000. Since h represents a distance, only the positive square root is considered, resulting in h = 600 meters. With the value of h known, b (base) is then calculated using the area equation, resulting in b = 180,000 / 600. The process is summarized as identifying two equations, substituting one into the other, simplifying, taking the derivative, setting it to zero, and solving to find the optimal dimensions.
Mindmap
Keywords
💡Optimization
💡Extrema
💡Derivative
💡Product
💡Sum
💡Perimeter
💡Area
💡Critical Numbers
💡Real-world Application
💡River
💡Pasteur
Highlights
Optimization involves using extrema to solve real-world problems by maximizing or minimizing certain variables.
The concept is applied in various scenarios like building a deck, managing finances, and planning travel to minimize costs.
Optimization in calculus is a straightforward process with three main steps: finding two equations, plugging one into the other, and solving the derivative set to zero.
The first step in optimization is identifying two equations from the problem statement.
The second step involves substituting one equation into the other to simplify the problem into a single equation with fewer variables.
The third step is taking the derivative of the simplified equation and setting it equal to zero to find the critical points that represent maximum or minimum values.
An example problem involves finding two numbers whose product is 192 and whose sum is minimized.
The牧场 (pasture) problem involves a dairy farmer fencing a rectangular area next to a river with a minimum amount of fencing, given the area constraint.
The area of the pasture is given by the product of its length and width, which must equal 180 square meters.
The amount of fencing needed is represented by the perimeter of the rectangle, which is a function of its length and width.
By solving for one variable in terms of the other and substituting into the perimeter equation, the problem is reduced to a single-variable optimization.
Taking the derivative of the perimeter equation with respect to one variable and setting it to zero yields the dimensions that minimize the fencing required.
The solution to the pasture problem shows that the dimensions requiring the least amount of fencing are when the length and width are equal, forming a square.
The method of optimization using calculus can be applied to various practical problems, demonstrating its versatility and usefulness.
The牧场 (pasture) problem illustrates how calculus can be used to find the most cost-effective solution to real-world scenarios.
The process of optimization involves critical thinking and mathematical manipulation, making it an essential tool in various fields.
The牧场 (pasture) problem concludes with finding the exact dimensions that minimize the fencing needed, showcasing the practical application of optimization.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: