The Logistic Equation and Models for Population - Example 1, part 2

patrickJMT
17 Oct 201005:09
EducationalLearning
32 Likes 10 Comments

TLDRThe video script details a mathematical problem involving a logistic equation to model the growth of a fish population in a lake. The biologists aim to understand how long it will take for the population to reach 4,000 fish. The solution involves algebraic manipulation of the logistic equation, which is initially set up from Part A of the problem. By substituting the target population into the equation and solving for time (T), the presenter simplifies the equation and applies logarithms to isolate T. After rounding and calculating the natural logarithms of the relevant fractions, the presenter divides them to find the time in years it takes for the population to reach the target. The approximate answer is just over two years, providing a clear and concise conclusion to the problem.

Takeaways
  • 📚 The problem involves a logistic equation used to model the population of fish in a lake over time.
  • 🧮 In Part A, a formula for the population at time T was derived as 10,000 divided by (1 + (19 * 17/57)^T).
  • ⏱️ Part B focuses on calculating the time it will take for the fish population to reach 4,000.
  • 🔍 To solve for time T, the script substitutes 4,000 into the left side of the equation and solves algebraically.
  • 🧹 Simplification of the equation involves dividing both sides by 10,000 and further reducing fractions.
  • 📉 The equation is rearranged to isolate the exponential term, and 1 is subtracted from both sides.
  • 🔢 Both sides of the equation are multiplied by 1/19 to eliminate the 19 from the equation.
  • 📌 Logarithms are introduced to solve for the exponent, specifically using the natural logarithm (ln).
  • 👉 The natural logarithm is applied to both sides of the equation, allowing the exponent 'T' to be removed.
  • ➗ The final step involves dividing the natural logarithms of the respective sides to solve for T.
  • 🔧 Approximations are made during the calculation to simplify the process and obtain a rounded result.
  • ⏳ The approximate time calculated for the fish population to reach 4,000 is just over two years.
Q & A

  • What is the topic of the video?

    -The video is about solving a problem related to the logistic equation, specifically focusing on the population dynamics of fish in a lake.

  • What was the formula derived in Part A for the population at time T?

    -The formula derived for the population at time T was 10,000 divided by (1 + (19 * 17) / 57) raised to the power of T.

  • What is the goal of Part B in the video?

    -The goal of Part B is to determine how long it will take for the fish population to reach 4,000.

  • How is the equation set up to solve for the time it takes to reach a population of 4,000 fish?

    -To solve for the time, the script plugs in 4,000 into the left side of the population formula and then solves for T by using algebraic manipulation and logarithms.

  • What is the final step before applying logarithms in the solution process?

    -The final step before applying logarithms is to isolate the exponential term by multiplying both sides of the equation by the reciprocal of 19.

  • How are logarithms used to simplify the equation and solve for T?

    -Logarithms are used to bring down the exponent, by taking the natural logarithm of both sides of the equation, which allows the T to come out as a coefficient.

  • What approximations were made during the calculation of the natural logarithms?

    -The approximations made were 3/38 rounded to 0.0789, and 17/57 rounded to 0.298, with both approximations rounded to five decimal places.

  • What is the approximate time it takes for the fish population to reach 4,000, according to the video?

    -According to the video, it will take approximately 2.098 years for the fish population to reach 4,000.

  • What property of logarithms is highlighted in the video?

    -The video highlights the property that allows the exponent (T in this case) to come out as a coefficient when taking the natural logarithm of an equation.

  • What is the significance of the logistic equation in the context of the video?

    -The logistic equation is significant as it models the growth of a population that is limited by available resources, which is applicable to the fish population in the lake.

  • How does the video address potential inaccuracies in the calculation?

    -The video acknowledges the use of approximations and rounding during the calculation, noting that the final answer provides a level of accuracy that may be more precise than necessary.

  • What is the final advice given to viewers regarding comments or questions?

    -The video encourages viewers to post any comments or questions they may have, indicating an openness to further discussion and clarification.

Outlines
00:00
📚 Solving the Logistic Equation for Fish Population Growth

This paragraph details the process of solving a logistic equation to determine the time it takes for a fish population to reach a certain number. The logistic equation is initially given, and then the problem of finding when the population reaches 4,000 is approached by substituting the target population into the equation. The solution involves algebraic manipulation, including dividing both sides by 10,000 and then by 1 over 19 to simplify the equation. Logarithms are introduced to solve for the variable T, representing time. The natural logarithm is applied to both sides, and the resulting equation is solved to find T, which is approximately 2.098 years. This indicates it will take slightly over two years for the fish population to reach 4,000.

05:01
💬 Inviting Feedback and Questions

The second paragraph serves as a closing statement, encouraging viewers to share their comments or ask questions if they have any. It emphasizes the presenter's openness to interaction and feedback, fostering a sense of community and engagement with the audience.

Mindmap
Keywords
💡Logistic Equation
The logistic equation is a mathematical model used to describe the growth of populations where the rate of increase is proportional to the current population but also limited by carrying capacity. In the video, it is used to model the population of fish in a lake over time, taking into account the limitations of the environment.
💡Biologists
Biologists are scientists who study living organisms and their relationship with the environment. In the context of the video, biologists are responsible for stocking the lake with fish, which initiates the population growth process that is being mathematically modeled.
💡Population at Time T
This term refers to the number of individuals in a population at a specific point in time, denoted by 'T'. The video discusses how to calculate this using the logistic equation to predict when the fish population will reach a certain size.
💡Carrying Capacity
The carrying capacity is the maximum population size that an environment can sustain without being degraded. In the logistic equation, it's a factor that helps to limit the growth of the population, ensuring it does not exceed the environment's limits. The script mentions this in the context of the lake's ability to support the fish population.
💡Algebra
Algebra is a branch of mathematics that uses symbols and rules to manipulate and solve equations. The video uses algebra to rearrange and solve the logistic equation for the variable 'T', which represents time, to find out when the fish population will be 4,000.
💡Logarithms
Logarithms are mathematical functions that help in solving exponential equations by converting them into linear equations. In the video, logarithms are used to simplify the exponential term in the logistic equation, making it easier to solve for the time 'T' when the fish population reaches 4,000.
💡Natural Logarithm
The natural logarithm is the logarithm to the base 'e', where 'e' is an irrational number approximately equal to 2.71828. It is used in the video to bring down the exponent in the logistic equation to solve for 'T'. This is a common technique in mathematical modeling of growth problems.
💡Approximation
Approximation is the process of finding a value that is close to the exact value but easier to work with. In the video, the speaker approximates certain values for the sake of simplicity when performing calculations, which is a common practice in mathematical modeling to make the problem more tractable.
💡Equation Solving
Equation solving is the process of finding the value of the unknown variable in a given equation. The video demonstrates the steps to solve for 'T' in the logistic equation, which is a critical part of understanding when the fish population will reach a target number.
💡Exponential Growth
Exponential growth is a pattern of growth where the rate of increase accelerates over time. In the logistic equation, the initial growth of the fish population is exponential, but it slows down as the carrying capacity is approached, which is a realistic model for population dynamics.
💡Environmental Limitations
Environmental limitations refer to the constraints that the environment imposes on the growth and survival of a population. In the context of the video, the logistic equation accounts for these limitations by incorporating the carrying capacity, which prevents the fish population from growing indefinitely.
Highlights

The speaker begins by introducing the second part of a problem dealing with the logistic equation.

In Part A, a formula for the population at time T was derived, using a specific mathematical expression.

The population at time T is given as 10,000 divided by 1 plus 19 times 17 over 57 raised to the power of T.

Part B of the problem focuses on determining the time it takes for the fish population to reach 4,000.

To solve this, the speaker suggests substituting 4,000 into the left side of the equation and solving for T.

A step-by-step algebraic manipulation is performed to isolate the variable T.

The equation is simplified by dividing both sides by 10,000 and further reducing fractions.

The speaker uses logarithms to solve for the exponent T, applying the natural logarithm to both sides of the equation.

The natural logarithm properties are utilized to bring down the exponent and simplify the equation.

The speaker approximates the values of the natural logarithms for further calculation.

After rounding and calculation, the speaker finds that it will take approximately 2.098 years for the fish population to reach 4,000.

The final answer is presented with a discussion on the accuracy and practicality of the result.

The speaker emphasizes that the calculation provides an estimate of the time needed for the fish population to grow.

The video concludes with an invitation for comments or questions from the viewers.

The speaker uses clear and step-by-step algebraic and logarithmic methods to solve the problem.

The problem-solving approach is practical, showing how to apply mathematical concepts to real-world scenarios.

The transcript provides a detailed walkthrough of the mathematical process, making it accessible to viewers with some algebraic knowledge.

The speaker's methodical approach to solving the logistic equation demonstrates a solid understanding of mathematical modeling.

The problem is relevant to fields such as biology and ecology, where population dynamics are studied.

The use of the logistic equation in this context highlights its importance in modeling population growth with limited resources.

Transcripts

Browse More Related Video

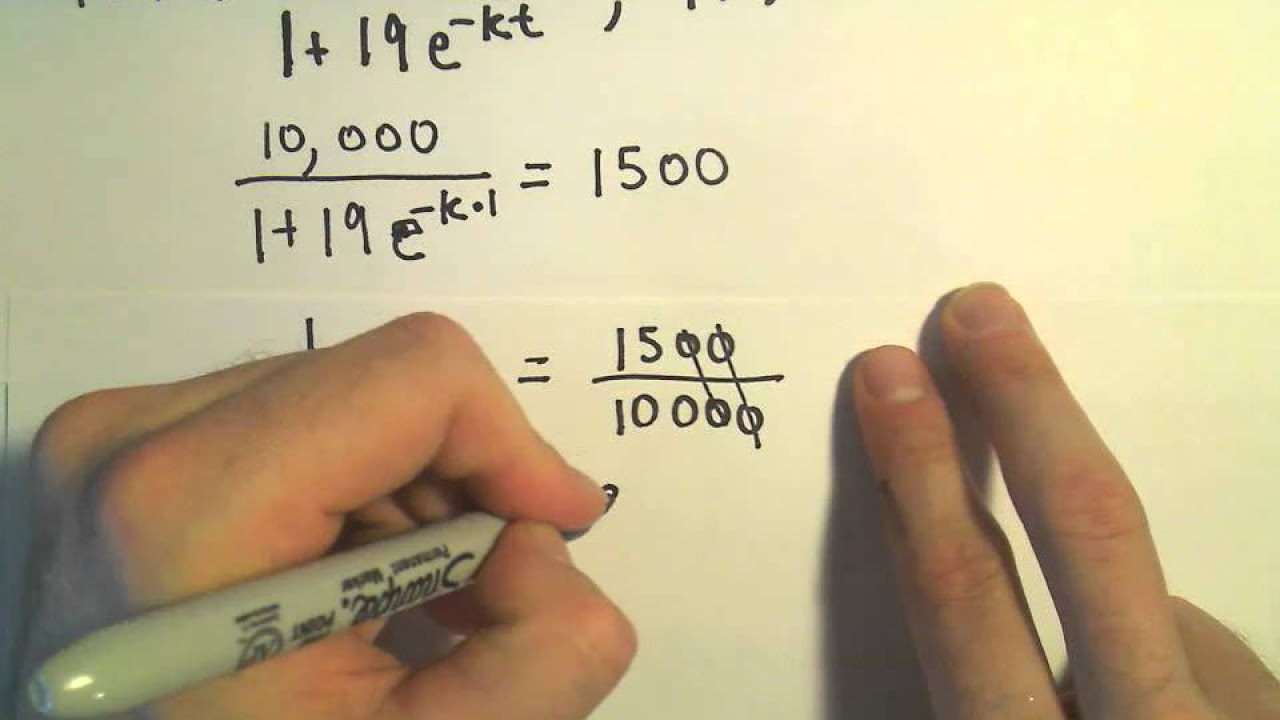
The Logistic Equation and Models for Population - Example 1, part 1

The Logistic Equation and the Analytic Solution

AP Calc BC: How to Solve a Logistic Growth Application Problem

Solving the logistic differential equation part 2 | Khan Academy

Logistic Differential Equation (general solution)

Logistic Growth (Separable Differential Equations)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Logistic EquationPopulation DynamicsMathematical ModelingBiological GrowthFish StockingLogarithmsAlgebraEcologyEnvironmental ScienceProblem SolvingEducational Content