How to Determine Your Units in Chemistry

Melissa Maribel
10 Aug 202006:36
EducationalLearning
32 Likes 10 Comments

TLDRThe video script emphasizes the critical role of units in chemistry, highlighting how mistakes in unit notation can significantly impact exam performance. It outlines six main exponent rules that are essential for understanding and applying mathematical operations in chemistry. The script then delves into unit conversion techniques, using dimensional analysis to demonstrate how to convert between units such as inches squared to feet squared and cubic meters to cubic centimeters. The process involves squaring or cubing conversion factors to match and cancel out units, as illustrated through step-by-step examples. Additionally, the script touches on the application of these concepts in the electromagnetic spectrum, specifically in de Broglie's equation, where unit conversion and cancellation are integral to solving problems. The summary concludes with advice on avoiding mistakes through practice and preparation, and directs viewers to a resource for further practice.

Takeaways
  • 📚 Units are crucial in Chemistry and can impact exam results significantly.
  • 🔢 There are 6 main exponent rules to follow when dealing with powers and roots in mathematical expressions.
  • ➕ When multiplying bases with the same exponent, add the exponents.
  • ➗ When dividing bases with the same exponent, subtract the exponents.
  • 🆗 Any number raised to the power of zero equals one.
  • 🔄 Negative exponents switch places and become positive, moving from the numerator to the denominator or vice versa.
  • √ Raising a number to a fractional exponent is the same as finding a root.
  • 🔄 Dimensional analysis is a method for unit conversion, which is essential in Chemistry.
  • 📐 To convert units, multiply by a conversion factor, and ensure exponents match to cancel out units.
  • ⚖️ Significant figures must be considered when rounding answers in Chemistry calculations.
  • 🧲 Understanding and applying these rules helps in solving complex problems, such as those involving de Broglie's equation.
  • 📉 Practice is key to mastering Chemistry concepts and avoiding common mistakes.
Q & A

  • Why are units crucial in Chemistry and what impact can they have on a student's performance?

    -Units are crucial in Chemistry because they provide the necessary context and scale to measurements. Incorrectly writing or forgetting units can lead to significant errors in calculations, which can be critical on exams, potentially determining whether a student passes or fails.

  • What does the term 'base' refer to in the context of exponent rules?

    -In the context of exponent rules, the term 'base' refers to a specific letter or unit that is being raised to an exponent or power.

  • How do you handle exponents when multiplying bases that are the same?

    -When multiplying bases that are the same, you add the exponents together to get your new answer.

  • What happens to the exponents when you divide bases that are the same?

    -When dividing bases that are the same, you subtract the exponents from one another to get your new answer.

  • How do you calculate the exponent when a power is raised to another power?

    -When a power is raised to another power, you multiply the exponents together to get the new answer.

  • What is the result when any number is raised to the power of zero?

    -When any number is raised to the power of zero, the result is always one.

  • How do negative exponents affect the position and value of a variable in a mathematical expression?

    -Negative exponents cause the variable to change its position; if it's in the numerator, it moves to the denominator and becomes positive, and vice versa. This essentially means the variable is in the reciprocal position with a positive exponent.

  • What does it mean when a number is raised to an exponent that is a fraction?

    -When a number is raised to an exponent that is a fraction, it represents a root. The numerator indicates the power to which the variable is raised, and the denominator specifies the type of root.

  • What is dimensional analysis and why is it important in Chemistry?

    -Dimensional analysis is a method used to convert between different units of measurement. It's important in Chemistry because it allows for accurate unit conversions which are essential for precise calculations and understanding of chemical reactions.

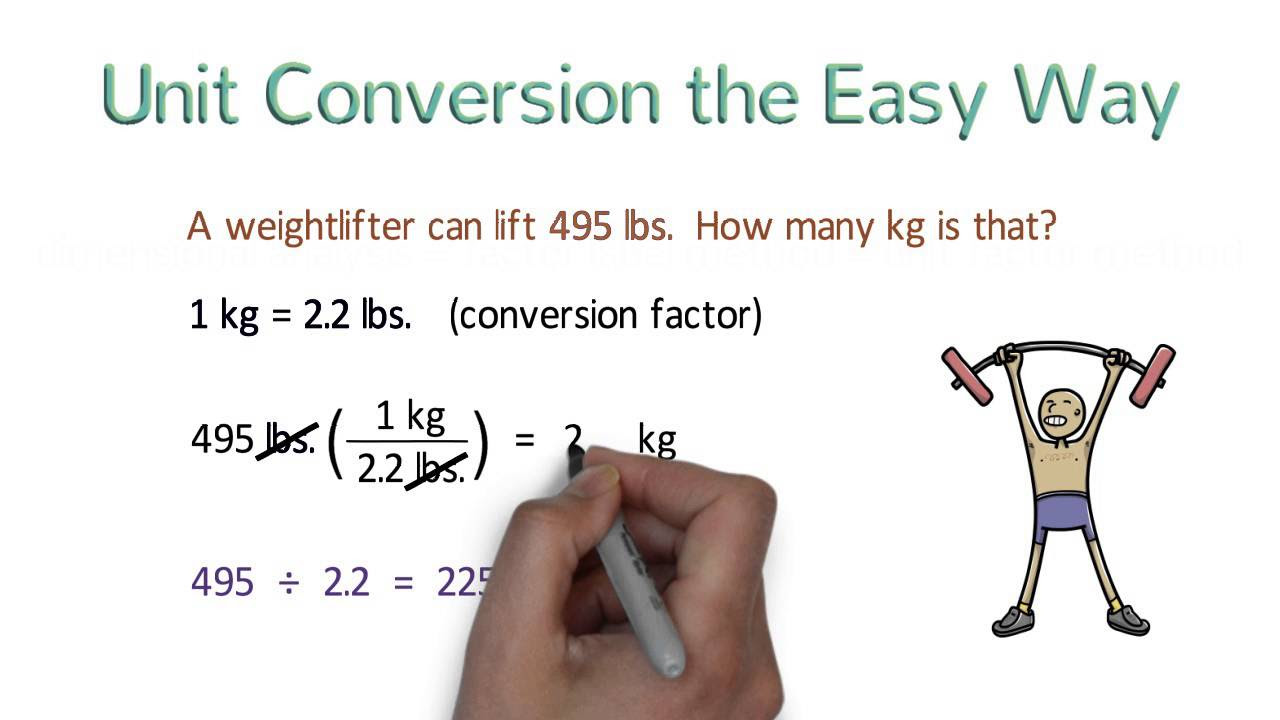
  • How do you convert units using the given conversion factor in dimensional analysis?

    -To convert units using a conversion factor, you multiply the original quantity by the conversion factor. The units that are the same on both the numerator and denominator will cancel out, leaving you with the desired unit.

  • What is the significance of squaring or cubing the conversion factor when converting units with different exponents?

    -Squaring or cubing the conversion factor is necessary to raise each part of the factor to the same power as the exponent in the original quantity. This ensures that the units cancel out correctly when performing the conversion.

  • How do you handle significant figures when performing calculations in Chemistry?

    -When performing calculations, you should round your final answer to the smallest number of significant figures present in the original data. This maintains the precision and accuracy of the result.

Outlines
00:00
🧪 Chemistry Units and Exponent Rules

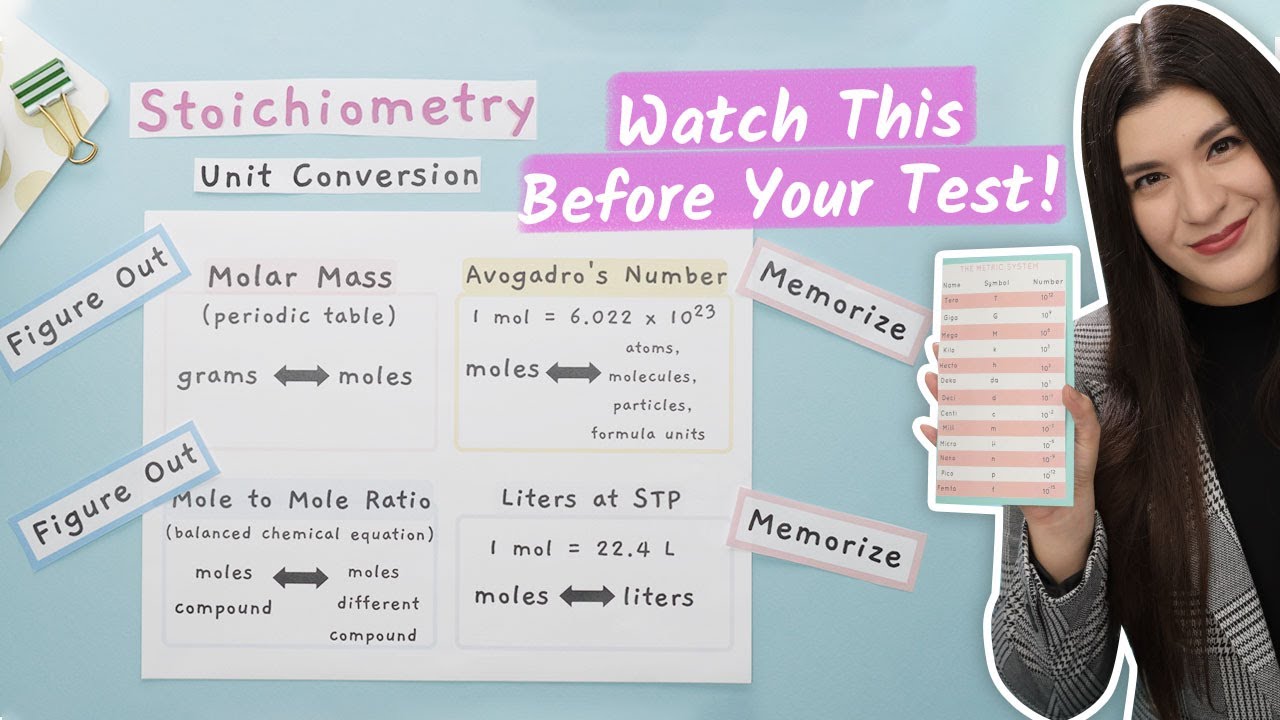
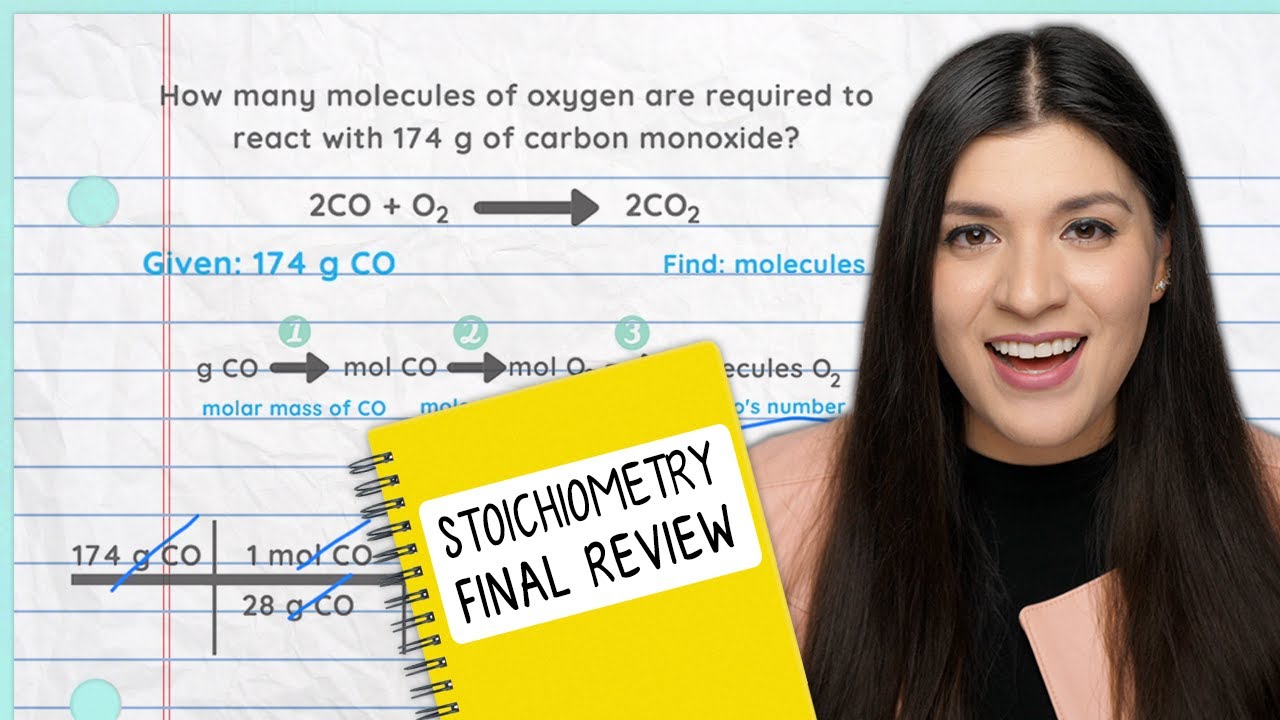
The paragraph emphasizes the critical role of units in Chemistry, highlighting common mistakes made by students such as incorrect unit notation or omission. It introduces six main exponent rules: adding exponents for multiplication, subtracting for division, multiplying for raising a power to another power, treating any power to zero as one, flipping and making negative exponents positive, and interpreting fractions as roots. The paragraph also covers the importance of unit conversion and dimensional analysis in Chemistry 1 and 2, providing examples of converting units from inches squared to feet squared and from cubic meters to cubic centimeters, and explaining the process of exponentiation and unit cancellation in each case.

05:04
📚 Advanced Unit Conversion and Dimensional Analysis

This paragraph delves into more complex unit conversions, using de Brogile's equation from the electromagnetic spectrum as an example. It demonstrates how to plug in given values, convert units, and cancel them out within the equation. The explanation involves applying exponent rules, such as subtracting exponents when dividing with the same base and flipping negative exponents to positive. The paragraph concludes with advice on avoiding mistakes through practice and preparation, directing viewers to a resource for practice problems at melissa.help/practice.

Mindmap
Keywords
💡Units
Units are fundamental in chemistry as they quantify measurements and are essential for accuracy in calculations. In the video, the importance of correctly writing units is emphasized to avoid mistakes that can impact exam results. An example of unit conversion is provided, illustrating the process of converting inches squared to feet squared using a conversion factor.
💡Exponent Rules
Exponent rules are mathematical principles that govern operations with powers. The video outlines six main exponent rules which are crucial for solving chemistry problems involving powers and roots. These rules are applied in various examples throughout the video, such as when converting units or working with equations like de Brogile's equation.
💡Dimensional Analysis
Dimensional analysis is a method used to convert between different units of measurement. It is highlighted in the video as a key technique in chemistry, often referred to as a 'fancy way to say unit conversion.' The process involves multiplying the original quantity by a conversion factor to cancel out the original units and obtain the desired units.
💡Conversion Factor
A conversion factor is a ratio that allows the conversion of a physical quantity from one unit to another. In the video, conversion factors are used to transform units, such as from inches to feet or cubic meters to cubic centimeters. The correct application of conversion factors is critical for accurate unit conversion in chemistry.
💡Significant Figures
Significant figures are digits in a number that carry meaning contributing to its precision. The video mentions the importance of rounding results to the appropriate number of significant figures, which is a rule for multiplication. This ensures that the result reflects the precision of the measurements involved.
💡Negative Exponents
Negative exponents are used in mathematics to represent reciprocal values. In the context of the video, negative exponents are discussed as part of the exponent rules, where they are converted to positive exponents and the variable is moved to the opposite side of the fraction, such as from the numerator to the denominator.
💡Fractions
Fractions are mathematical expressions representing a part of a whole. The video discusses the manipulation of fractions, particularly when dividing, which involves multiplying by the reciprocal of the second fraction. The process of canceling out common factors in the numerator and denominator is also explained.
💡Root
A root, in mathematics, is a value that, when raised to a certain power, gives a specific result. In the video, raising a number to a fractional exponent is described as taking a root. The numerator of the fraction indicates the power, while the denominator indicates the root type.
💡de Brogile's Equation
de Broglie's equation is a fundamental equation in quantum mechanics that relates the wavelength of a particle to its momentum. In the video, it is used as an example to demonstrate unit conversion and cancellation in the context of the electromagnetic spectrum.
💡Practice Problems
Practice problems are exercises that help reinforce learning and prepare students for exams. The video encourages students to practice by providing a resource (melissa.help/practice) where they can try out questions and then return to the video for further learning.
💡Chemistry 1 and Chemistry 2
These terms refer to sequential courses in chemistry, typically taken by students to progress in their understanding of the subject. The video mentions that the exponent rules and unit conversion skills taught are applicable across both courses, indicating the foundational nature of these concepts.
Highlights

Units are crucial in Chemistry and can impact exam results.

Six main exponent rules are introduced for understanding and applying in Chemistry.

When multiplying bases with the same exponent, add the exponents.

When dividing bases with the same exponent, subtract the exponents.

Raising a power to another power involves multiplying the exponents.

Any number raised to the power of zero equals one.

Negative exponents switch the variable's position and become positive.

A fraction exponent indicates a root, with the numerator as the power and the denominator as the root type.

Dimensional analysis is a method for unit conversion, crucial in Chemistry 1 and Chemistry 2.

Conversion factors are squared to cancel out units squared in dimensional analysis.

Rounding to the lowest number of significant figures is essential in Chemistry calculations.

Cubic conversion factors are raised to the power of three to match units.

de Brogile's equation involves unit conversion and cancellation in the electromagnetic spectrum.

Subtracting exponents is required when dividing with the same base.

Negative exponents in fractions switch positions and become positive.

Cancelling out units in fractions simplifies equations.

Practice is key to avoiding mistakes in Chemistry calculations.

All practice problems are available at melissa.help/practice for additional learning.

Transcripts

Browse More Related Video

How to Convert Units in Chemistry

Know This For Your Chemistry Final Exam - Stoichiometry Review

Converting Units with Conversion Factors

Unit Conversion the Easy Way (Dimensional Analysis)

Chemistry Reference table C

Calculate Work from Pulling Springs - Hooke's Law with Integrals

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Chemistry RulesExponent RulesUnit ConversionDimensional AnalysisEducational ContentSTEM LearningScience ExamsPractice ProblemsAcademic SuccessStudy TipsWebinar Series