Calculate Work from Pulling Springs - Hooke's Law with Integrals

Quoc Dat Phung

4 Oct 202126:19

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video script guides viewers through calculating work done on a spring using Hooke's Law. It outlines a four-step process: diagram drawing, unit conversion, spring constant (k) determination, and work calculation via integration. The script provides detailed examples, converting units from pounds and inches to feet-pounds, and from newtons and centimeters to meters and joules, demonstrating how to find k from given work and compute the work done to stretch a spring to specific lengths, simplifying complex physics concepts for students.

Takeaways

📚 The video demonstrates how to calculate work done on a spring using Hooke's Law.
📝 The process involves four main steps: drawing diagrams, unit conversion, finding the spring constant (k), and calculating work.
📏 The first step is to visualize the problem with diagrams representing the spring's natural length and the stretched length.
🔄 The second step requires converting units to ensure consistency, such as converting pounds to newtons and inches to feet or meters.
🔢 The spring constant (k) is found using the formula force = k * distance, where the force is given and the distance is the stretch beyond natural length.
⚙️ Hooke's Law is integral to the process, stating that the force exerted by a spring is proportional to its displacement from the natural length.
📉 The work done on the spring is calculated using the integral of force times distance (dx) from the natural length to the stretched length.
📚 The video provides examples with both imperial (pounds and feet) and metric (newtons and meters) units to illustrate the process.
📉 The anti-derivative of x (which is 1/2 * x^2) is used to evaluate the integral for work done on the spring.
🔢 The final work is calculated by substituting the limits of integration into the anti-derivative formula and simplifying the result.
📋 The video concludes with additional problems to test the viewer's understanding of calculating work done on springs.

Q & A

What is Hooke's Law and how is it used in the context of the video?
-Hooke's Law states that the force needed to extend or compress a spring by some distance is proportional to that distance. In the video, Hooke's Law is used to calculate the work done in stretching a spring, where the force is represented as k times x, with k being the spring constant and x being the distance the spring is stretched.
What are the four steps outlined in the video for solving spring-related work problems?
-The four steps are: 1) Draw diagrams to visualize the problem, 2) Convert units on the x-axis to ensure consistency (e.g., force in pounds and distance in feet implies work in foot-pounds), 3) Find the spring constant k if not given, using the formula for force in terms of k and x, and 4) Calculate the work done using the integral of force with respect to distance from the initial to the final position.
How do you convert units from inches to feet as demonstrated in the video?
-To convert inches to feet, you divide the number of inches by 12 since there are 12 inches in a foot. For example, to convert 4 inches to feet, you would calculate 4/12, which simplifies to 1/3 of a foot.
What is the formula for calculating work done on a spring according to the video?
-The formula for calculating work done on a spring is the integral from initial position 'a' to final position 'b' of force times distance (dx), where the force is given by Hooke's Law (k times x). The work is thus the integral of kx dx from 'a' to 'b'.
How is the spring constant 'k' found in the video?
-The spring constant 'k' is found by using the given force and the distance the spring is stretched or compressed. By rearranging the formula for force (F = kx), 'k' can be calculated as k = F/x.
What is the integral used to calculate the work done on the spring in the video?
-The integral used to calculate the work done is the integral of k times x with respect to x, from the initial position to the final position. Since k is a constant, it can be taken outside the integral, resulting in k times the integral of x dx.
How is the anti-derivative of x with respect to x found in the video?
-The anti-derivative of x with respect to x is found by recognizing that the integral of x dx is one-half times x squared (x^2/2), as x is raised to the power of 2 and then the power rule for integration is applied.
What is the final answer for the work done in stretching the spring from its natural length to six inches beyond, as given in the video?
-The final answer for the work done, as calculated in the video, is 15/4 foot-pounds, after simplifying the expression obtained from evaluating the integral.
How does the video approach the problem of finding the work done when the spring is stretched from 15 centimeters to 18 centimeters?
-The video approaches this problem by first converting all units to meters, finding the spring constant 'k' using the given force and initial stretch distance, and then calculating the work done using the integral of the force over the new distance.
What trick does the video suggest for finding the spring constant 'k' when work is given instead of force?
-The video suggests setting the integral of k times x dx from the initial to the final position equal to the given work, and then solving for 'k' by rearranging the equation and evaluating the integral.

Outlines

00:00

🔍 Calculating Work Done on a Spring Using Hooke's Law

This paragraph introduces a video tutorial on calculating the work done when stretching a spring, using Hooke's Law. It explains that a force of 10 pounds is needed to stretch a spring four inches beyond its natural length and asks how much work is done to stretch it to six inches beyond its natural length. The video outlines four steps to solve the problem: drawing diagrams, unit conversion, finding the spring constant 'k' if not given, and using the integral formula for work done. The paragraph emphasizes that these steps are crucial for solving such problems and encourages viewers to pause and write down the formula for Hooke's Law.

05:00

📚 Detailed Steps to Calculate Work on a Spring: Diagrams and Unit Conversion

The second paragraph delves into the first two steps of the problem-solving process: drawing diagrams and converting units. It illustrates the concept with a spring at rest and explains how to represent the stretching process on an x-axis. The paragraph then details the unit conversion process, converting pounds to newtons and inches to feet, to ensure consistency with the work done in joules or foot-pounds. The explanation is thorough, guiding viewers through the mathematical conversions necessary to proceed with the problem.

10:02

🔧 Finding the Spring Constant 'k' and Calculating Work Done

This paragraph continues the tutorial by addressing the third and fourth steps: finding the spring constant 'k' and calculating the work done. It uses the given force of 10 pounds at a stretch of one-third of a foot to solve for 'k', resulting in 30 pounds per foot. The paragraph then describes how to calculate the work done by integrating the force over the distance from zero to one-sixth of a foot. The integral is simplified by factoring out the constant 'k', leading to the calculation of the work done in foot-pounds, which is simplified to 15/4 foot-pounds.

15:04

📘 Applying Hooke's Law to Another Spring Problem with Newtons and Centimeters

The fourth paragraph presents a new problem involving a spring stretched from 10 centimeters to 15 centimeters with a force of 40 newtons. It guides viewers through drawing the diagram, converting units from centimeters to meters, and finding the spring constant 'k' using the given force and stretch distance. The paragraph then explains how to calculate the work done by integrating from 15 to 18 centimeters, resulting in the work done in joules, which is approximately 1.56 joules.

20:05

🔄 Solving for Work and Spring Extension with Given Force and Work Values

In this paragraph, the video script presents a scenario where 2 joules of work are needed to stretch a spring from 30 to 42 centimeters. It outlines the steps to find the work needed to stretch the spring from 35 to 40 centimeters and to determine the distance a 30-newton force can stretch the spring beyond its natural length. The paragraph explains how to use the given work value to find the spring constant 'k' and then apply it to calculate the work for the new stretch distance, resulting in 25/24 joules for part A.

25:06

📏 Determining Spring Extension with a Given Force

The final paragraph concludes the tutorial by solving for the distance a spring can be stretched with a 30-newton force, using the previously calculated spring constant 'k'. It simplifies the force equation to find the stretch distance in meters, which is then converted to centimeters, resulting in 10.8 centimeters. The paragraph provides a clear answer to the problem and offers the option to keep the distance in meters or convert it to centimeters, depending on the viewer's preference.

Mindmap

Keywords

💡Work

In the context of physics, 'work' is defined as the amount of energy transferred by a force acting upon an object as it moves along the direction of the force. In the video, work is calculated using Hooke's Law to determine the energy required to stretch a spring from its natural length to a certain distance. The script provides examples of calculating work in different units such as foot-pounds and joules, depending on the units of force and distance used.

💡Hooke's Law

Hooke's Law states that the force needed to extend or compress a spring by some distance is proportional to that distance. It is mathematically represented as F = kx, where F is the force applied, k is the spring constant, and x is the displacement from the spring's natural length. The video uses Hooke's Law as the fundamental principle for calculating the work done in stretching springs.

💡Spring Constant (k)

The spring constant, denoted as 'k', is a measure of the stiffness of a spring. It is a key parameter in Hooke's Law and is used to calculate the force needed to stretch or compress the spring by a given distance. The video demonstrates how to determine the spring constant from given information and how it is used in subsequent calculations of work done.

💡Integral

An integral in calculus represents the area under a curve defined by a function between two points. In the video, the integral is used to calculate the work done on the spring by integrating the force (which is a function of displacement) over the distance the spring is stretched.

💡Natural Length

The 'natural length' of a spring refers to its length when no external forces are applied. The video discusses stretching the spring 'beyond its natural length,' which is the starting point for calculating the work done on the spring.

💡Force

In physics, 'force' is any interaction that, when unopposed, will change the motion of an object. The video script discusses the force required to stretch a spring and how it is related to the spring's displacement from its natural length according to Hooke's Law.

💡Units Conversion

The script emphasizes the importance of converting units for force, distance, and work to ensure consistency in calculations. For example, if force is given in pounds, the distance must be in feet to calculate work in foot-pounds. The video provides examples of converting inches to feet and centimeters to meters.

💡Diagrams

The video script instructs viewers to draw diagrams to visualize the problem, showing the spring at rest, stretched, and the distances involved. Diagrams are essential for understanding the physical setup and the variables in the calculations.

💡Anti-Derivative

An anti-derivative, also known as an integral, is a function that reverses the process of differentiation. In the context of the video, the anti-derivative is used to find the area under the force-displacement curve, which represents the work done on the spring.

💡Joule

A 'joule' is the SI unit of energy, work, or amount of heat. The video mentions joules as the unit of work when the force and distance are given in newtons and meters, respectively. It contrasts with foot-pounds, which is used when force is in pounds and distance in feet.

💡Newton

A 'newton' is the SI unit of force. In the video, newtons are used as the unit for force when calculating work in the context of Hooke's Law, particularly when the spring is stretched from one length to another in meters.

Highlights

Introduction to calculating work done on a spring using Hooke's Law.

Explanation of the four-step process to solve spring force problems.

Step 1: Drawing diagrams to visualize the problem.

Step 2: Converting units for force, distance, and work.

Step 3: Finding the spring constant 'k' if not given.

Step 4: Calculating work using the integral of force times distance.

Example problem: Calculating work to stretch a spring 6 inches beyond its natural length.

Conversion of inches to feet for unit consistency.

Solving for 'k' using the given force and stretch distance.

Integral calculation for work done in stretching the spring.

Final answer presentation in feet-pounds.

Second example problem with a spring stretched from 15 to 18 centimeters.

Conversion of centimeters to meters for the second example.

Finding 'k' for the second example using given force and stretch distance.

Calculation of work done using the integral method for the second example.

Answer for the second example presented in joules.

Additional problem involving work needed to stretch a spring to 40 centimeters and force to stretch it further.

Conversion of centimeters to meters for the additional problem.

Using work formula to find 'k' when work is given instead.

Calculation of work to stretch the spring from 30 to 40 centimeters.

Determining the stretch distance for a given force of 30 newtons.

Final answers for both parts of the additional problem in joules and meters/centimeters.

Transcripts

Browse More Related Video

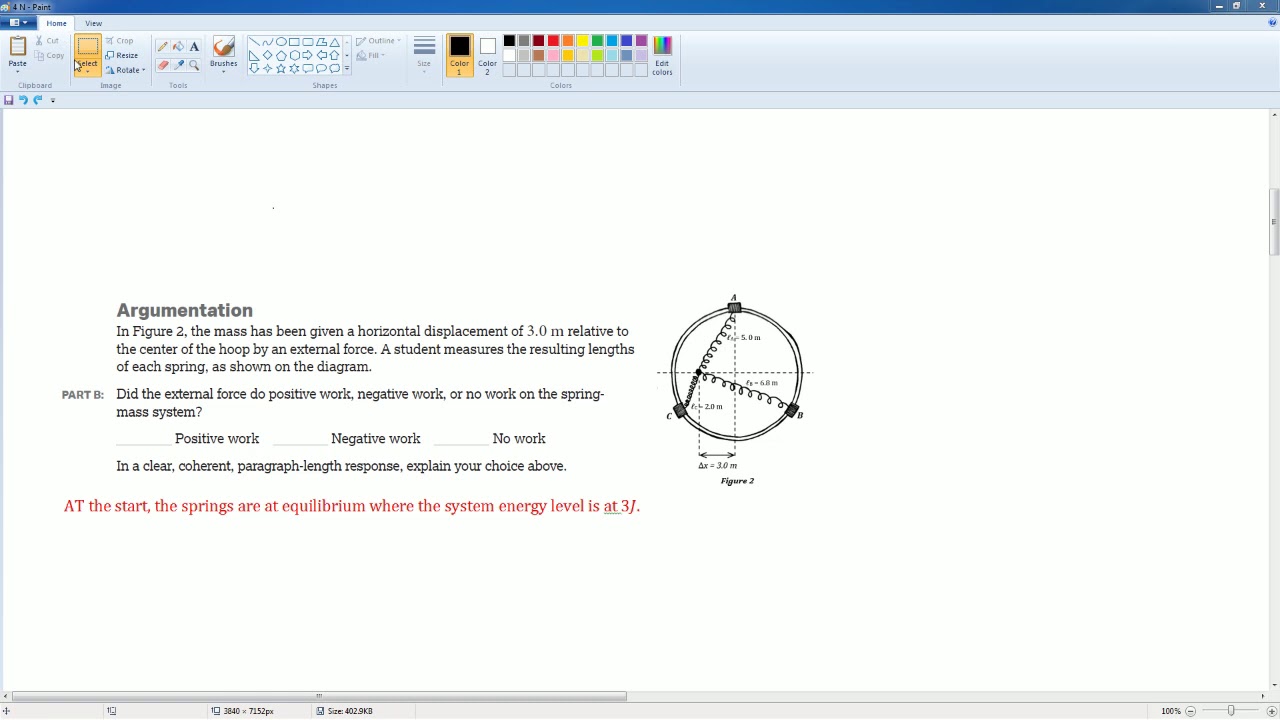
AP Physics Workbook 4.N Potential Energy of Systems

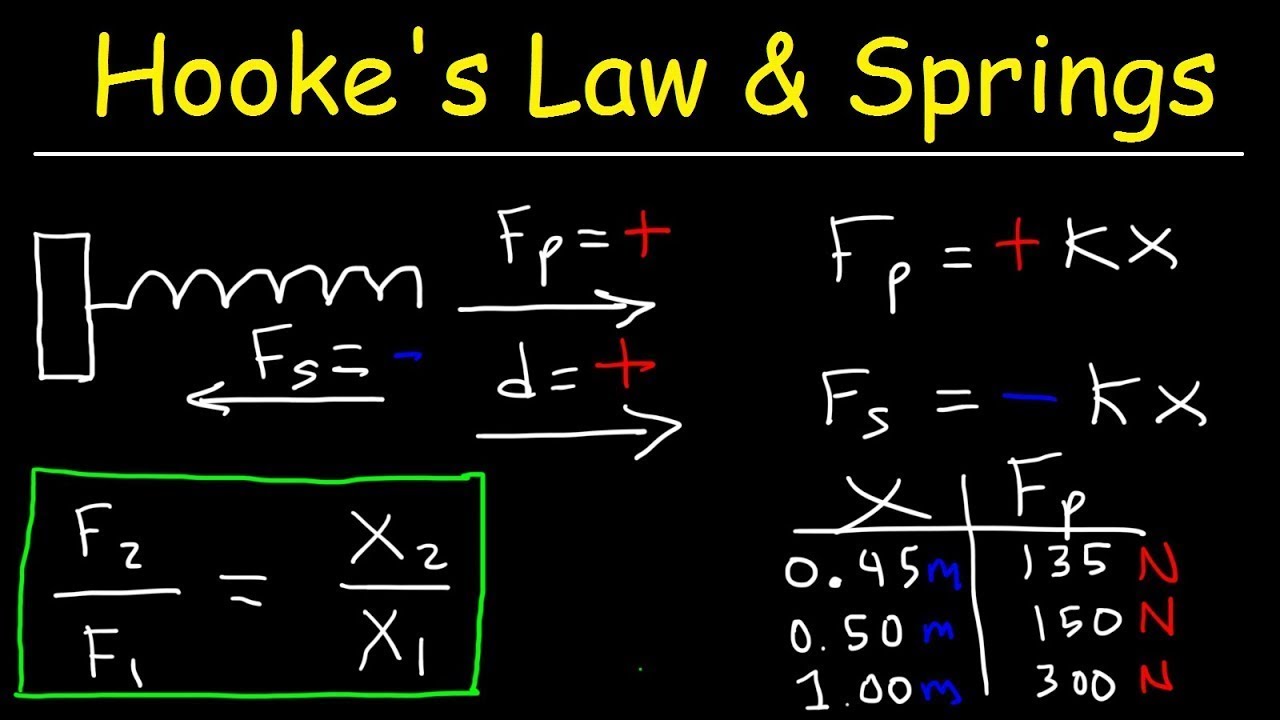
Hooke's Law and Elastic Potential Energy

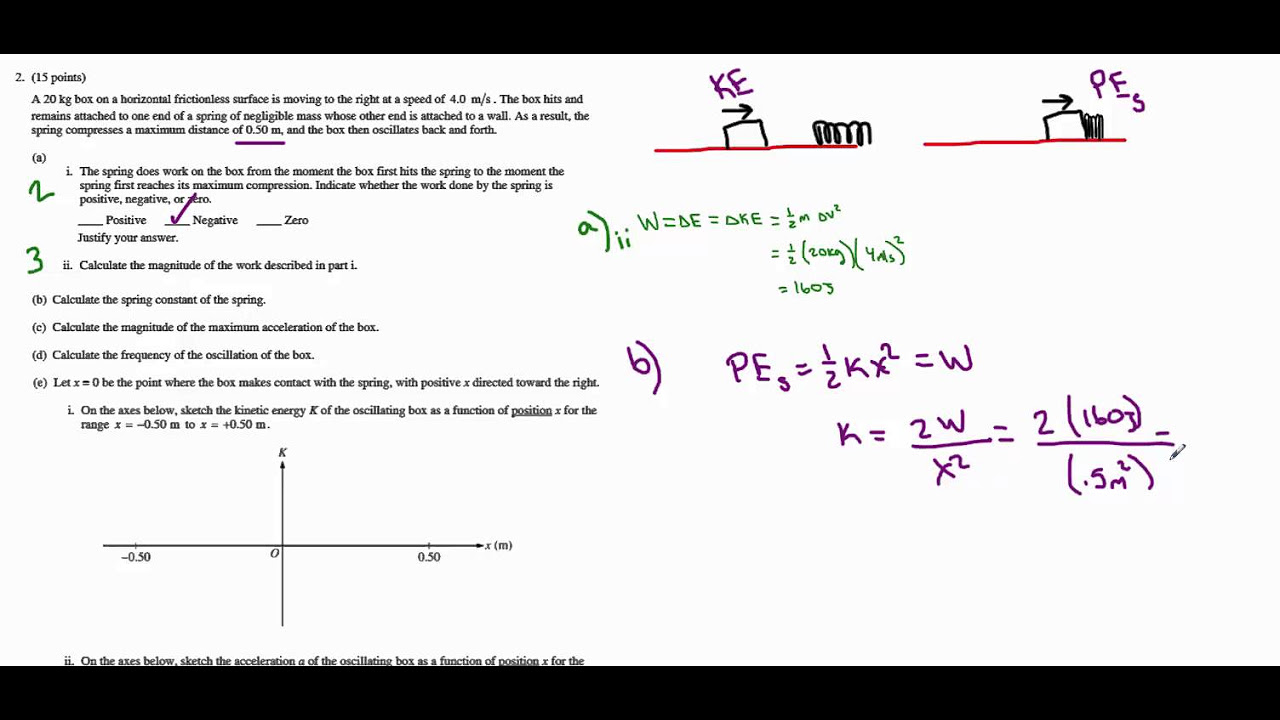
AP Physics B - 2013 #2 (Springs)

Potential energy stored in a spring | Work and energy | Physics | Khan Academy

Work Done Raising a Leaky Bucket with an Integral

AP Physics C: Work, Energy, and Power Review (Mechanics)

Related Tags

Hooke's Law Spring Mechanics Physics Tutorial Work Calculation Educational Video Problem Solving Force Analysis Spring Stretching Energy Conversion Physics Education