Unit Conversion the Easy Way (Dimensional Analysis)
TLDRThe video script introduces viewers to the concept of dimensional analysis, or the factor-label method, for unit conversion. It walks through the process using two examples: converting pounds to kilograms and kilograms to tons. The method involves using conversion factors and setting up a fraction to cancel out the initial units and obtain the desired units. The script emphasizes the versatility of this technique, even for multiple-step conversions, and encourages viewers to apply this method for accurate and efficient unit conversions.
Takeaways
- 📏 The script introduces dimensional analysis, also known as the factor-label or unit-factor method, as a versatile problem-solving technique for unit conversion.
- 🔄 The process of unit conversion begins by identifying the given quantity and its units, which is separate from the conversion factor.
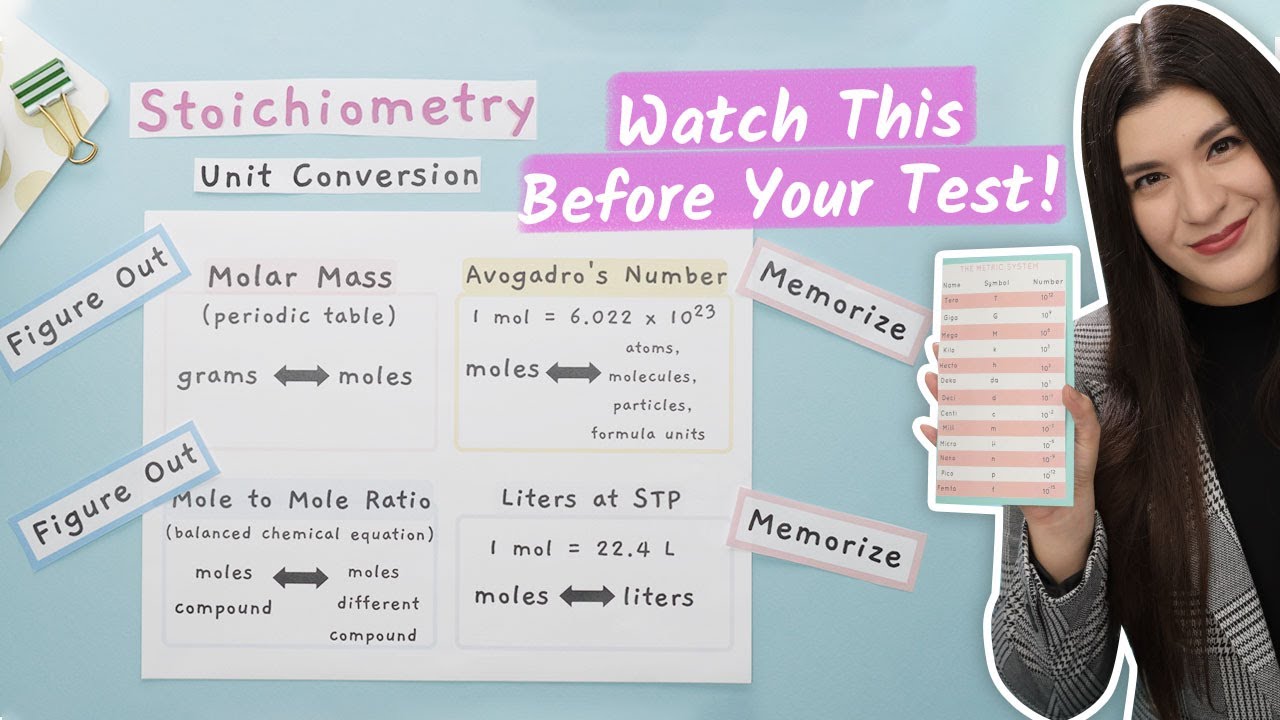
- 🔢 Conversion factors are essential for unit conversion; for example, 1 kg equals 2.2 pounds is used for converting pounds to kilograms.
- ✍️ When setting up the conversion, write the starting quantity with its units and then multiply by a fraction derived from the conversion factor.
- 📉 The numerator of the fraction is the desired unit, and the denominator is the unit you're converting from, ensuring the unwanted units cancel out.
- 🧮 For simple calculations, the fraction setup allows for direct input into a calculator, simplifying the process when the numerator is 1.
- 🚀 The script progresses to a more complex example, showing how to convert kilograms to tons via a two-step process with multiple conversion factors.
- 🔍 It's important to choose the correct conversion factors for each step, ensuring that the final unit desired is the one left after cancellation.
- 🌟 The script highlights a more efficient method for multiple conversions by combining steps into one, using fractions to guide when to multiply or divide.
- 📌 When dealing with numbers in conversions, it's crucial to maintain the correct number of significant figures, as demonstrated with the initial quantity of 1920 kg having three significant figures.
- 💡 The script emphasizes the power of using conversion factors in fraction form, as it simplifies the decision-making process for when to multiply or divide during the conversion.
Q & A
What is the primary method discussed in the script for unit conversion?
-The primary method discussed for unit conversion is dimensional analysis, also known as the factor-label method or the unit-factor method.
How does the script introduce the concept of unit conversion?
-The script introduces unit conversion by presenting a simple problem: converting the weight a weightlifter can lift in pounds to kilograms.
What is the conversion factor between pounds and kilograms?
-The conversion factor between pounds and kilograms is 1 kg equals 2.2 pounds.
How does the script suggest we handle the conversion factor in unit conversion?
-The script suggests writing down the quantity to be converted and then multiplying it by a fraction that includes the numbers from the conversion factor, with the unit we want to cancel out on the bottom and the target unit on the top.
What is the significance of the units in the conversion factor fraction?
-The units in the conversion factor fraction are significant because they determine which units cancel out and which remain, allowing for the conversion to the desired unit.
How does the script address the challenge of not having a calculator that can handle fractions?
-The script suggests that if a calculator cannot handle fractions, one can simplify the problem by recognizing that when the top number of the fraction is 1, the problem becomes a simple division, which can be solved by dividing the original number by the bottom number of the fraction.
What is the second unit conversion problem presented in the script?
-The second unit conversion problem presented is converting the mass of a car from kilograms to tons.
How many conversion factors are needed to convert kilograms to tons?
-Two conversion factors are needed to convert kilograms to tons: one to convert kilograms to pounds and another to convert pounds to tons.
What is the significance of the final answer's significant figures in the script?
-The significance of the final answer's significant figures is to maintain the accuracy of the conversion. The script emphasizes that the answer should only have three significant figures because the original quantity, 1920 kg, also has three significant figures.
How does the script suggest simplifying multi-step unit conversions?
-The script suggests that multi-step unit conversions can be simplified by combining the conversion factors into a single fraction, allowing for all units to cancel out in one step, rather than doing multiple separate calculations.
What is the final answer to the car mass conversion problem?
-The final answer to the car mass conversion problem is 2.11 tons.
What advice does the script give for viewers?
-The script advises viewers to practice the technique, engage with the content by commenting, voting, subscribing, and visiting the provided website for more information.
Outlines
📚 Introduction to Unit Conversion and Dimensional Analysis
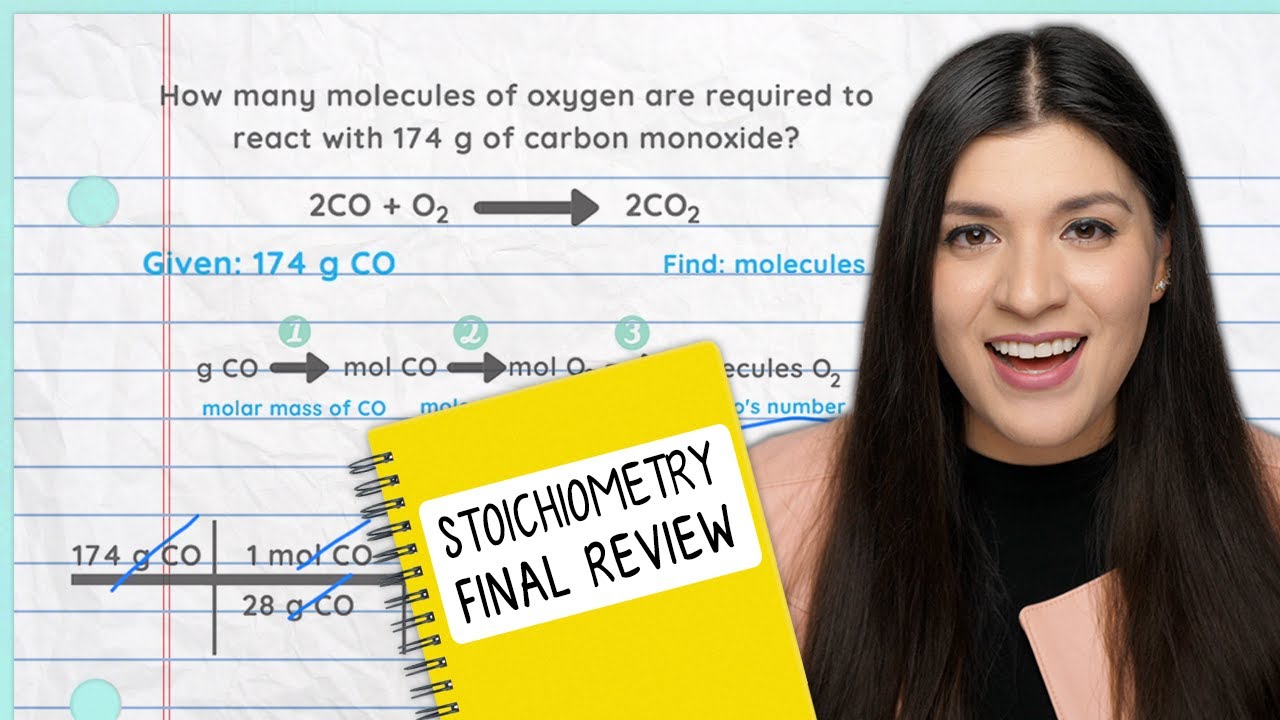
This paragraph introduces the concept of unit conversion using the method of dimensional analysis, also known as the factor-label or unit-factor method. It emphasizes the versatility and power of this technique for problem-solving. The explanation begins with a simple example of converting pounds to kilograms, highlighting the importance of the conversion factor (1 kg = 2.2 pounds). The process involves writing down the quantity to be converted, multiplying it by a fraction derived from the conversion factor, and performing the calculation. The example demonstrates how units cancel out, leaving the desired unit. The paragraph also touches on the concept of significant figures and introduces a slightly more complex problem involving two-step conversions, emphasizing the method's effectiveness and efficiency.
🔄 Solving Multi-Step Unit Conversions with Fractions
This paragraph delves into the process of solving unit conversion problems that require multiple conversion factors. It builds upon the previous example by adding another step to convert kilograms to tons. The explanation shows how to use fractions for conversion factors, with the bottom number being the unit to cancel out and the top number being the target unit. The paragraph explains the rationale behind choosing the correct conversion factors and the order in which to apply them. It also introduces a more efficient method for solving multi-step conversions by combining the steps into a single calculation, thus simplifying the process. The summary emphasizes the power of this method, regardless of the number of conversions needed, and how it helps determine when to multiply or divide. The paragraph concludes with a call to action for the viewer to engage with the content creator's platform.
Mindmap
Keywords
💡Unit Conversion
💡Dimensional Analysis
💡Conversion Factor
💡Pounds (lbs)
💡Kilograms (kg)
💡Tons
💡Fraction
💡Canceling Units
💡Significant Figures
💡Calculator
💡Multiple Conversion Factors
Highlights
Introduction to dimensional analysis for unit conversion.
The method is also known as the factor-label method or unit-factor method.
Dimensional analysis is a versatile and powerful problem-solving technique.
A simple unit conversion example: converting pounds to kilograms.
The importance of knowing the conversion factor for unit conversion.
Writing down the quantity to be converted with its units is the first step.
Multiplying the quantity by a fraction derived from the conversion factor.
Deciding the placement of numbers in the fraction based on the units involved.
Canceling out the starting units to obtain the desired units in the result.
Using a calculator to perform the mathematical operation for the conversion.
Understanding that unit conversions involve multiplying by a fraction that equals one.
An example of converting kilograms to tons with a two-step process.
The necessity of two conversion factors for complex unit conversions.
Combining multiple conversion factors into a single step for efficiency.
The method's power in handling numerous conversions with ease.
Adjusting for significant figures based on the original quantity.
Final answer for the complex conversion problem: 2.11 tons.
The demonstration of a better way to solve problems with multiple conversion factors.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: