How to Do Implicit Differentiation (NancyPi)
TLDRIn this informative video, Nancy explains the concept of implicit differentiation, a technique used when a function is not explicitly expressed in terms of 'y ='. She outlines the two main steps: differentiating both sides with respect to 'x' and then solving for 'dy/dx'. Nancy illustrates this process with examples, emphasizing the importance of the Chain Rule and the Product Rule in handling terms with 'y'. She also warns viewers about common pitfalls, such as neglecting the Product Rule and the proper handling of 'dy/dx' notation, ultimately aiming to demystify implicit differentiation for her audience.
Takeaways
- 📝 Implicit differentiation is necessary when functions are not explicitly solved for y but expressed in terms of both x and y.
- 📱 The first step in implicit differentiation is to differentiate both sides of the equation with respect to x, attaching dy/dx to terms containing y.
- 🔧 Implicit differentiation utilizes the chain rule to handle derivatives of terms with y, as these are indirectly related to x.
- 📈 After differentiating, the next step is to isolate dy/dx on one side of the equation to solve for it, using algebraic manipulation.
- 🔢 The power rule is used for differentiating powers of x and y; however, for y terms, multiply the result by dy/dx.
- 📣 Techniques such as the product rule may be needed if the function includes products of x and y, indicating more complex relationships.
- 🔨 It's important to factor out dy/dx if it appears multiple times, to simplify the equation and solve for dy/dx as a single term.
- 💡 Common pitfalls in implicit differentiation include forgetting to use the product rule when required and not properly isolating dy/dx.
- 📔 The video tutorial aims to provide a clear, step-by-step guide to mastering implicit differentiation, a key tool in calculus.
- 📚 The tutorial suggests that viewers like or subscribe if they find the help useful, highlighting the educational nature of the content.
Q & A
What is the main difference between explicit and implicit differentiation?
-Explicit differentiation involves a function written as 'y =' some expression in terms of x, while implicit differentiation is used when the function is not written explicitly in terms of y but is instead given implicitly as a relationship between x and y.
Why is the Chain Rule used in implicit differentiation?
-The Chain Rule is used in implicit differentiation because the function being differentiated contains an 'inside' function (in terms of y) which is composed of 'outside' variables (x). Multiplying by dy/dx accounts for the derivative of the inside function with respect to x.
How do you perform the first step of implicit differentiation?
-The first step of implicit differentiation is to differentiate both sides of the given equation with respect to x. If a term contains y, you multiply that term by dy/dx.
What is the goal of the second step in implicit differentiation?
-The goal of the second step is to isolate dy/dx on one side of the equation, resulting in an expression that looks like dy/dx equals some function of x and y.
What algebraic manipulation might be necessary to solve for dy/dx in implicit differentiation?
-Algebraic manipulations such as distributing, factoring, combining like terms, and dividing might be necessary to get dy/dx alone on one side of the equation.
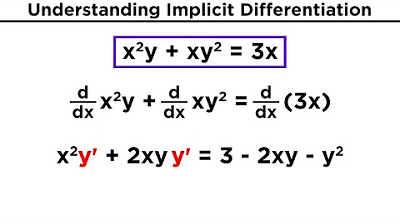
Why is it important to use the Product Rule when differentiating terms like x squared y in implicit differentiation?
-The Product Rule is important because it accounts for the derivatives of both the x and y parts of the term. Ignoring it would lead to an incorrect result, as y is a function of x and its derivative must be included in the differentiation process.
How does the process of implicit differentiation change when dealing with more complex functions, such as those involving trigonometric functions or products of x and y?
-For more complex functions, you still start by differentiating both sides with respect to x. However, you may need to use additional rules like the Product Rule and Chain Rule to handle the different components of the function, and more algebraic manipulation may be required to isolate dy/dx.
What is the significance of the term 'dy/dx' in implicit differentiation?
-The term 'dy/dx' represents the derivative of y with respect to x, which is the rate of change of y as x changes. It is the key component in implicit differentiation as it allows us to express the relationship between x and y in terms of their rates of change.
How does the notation 'y prime' relate to 'dy/dx' in the context of differentiation?
-The notation 'y prime' is an alternative way to represent the derivative of y with respect to x, which is the same as 'dy/dx'. Both notations are used to denote the same concept in calculus.
What are some common mistakes students make when performing implicit differentiation?
-Some common mistakes include forgetting to use the Product Rule when differentiating terms involving both x and y, not factoring when dy/dx appears more than once, and neglecting to differentiate both sides of the equation with respect to x.
How can one ensure they are correctly isolating 'dy/dx' during the second step of implicit differentiation?
-To correctly isolate 'dy/dx', one must carefully manipulate the equation by distributing, combining like terms, and factoring out common terms to ensure that 'dy/dx' only appears once and is alone on one side of the equation.
What is the final result of implicit differentiation expressed as?
-The final result of implicit differentiation is expressed as dy/dx equals some function of x and y, where 'dy/dx' represents the derivative of y with respect to x, and the function represents the relationship between x and y based on the original equation.
Outlines
📚 Introduction to Implicit Differentiation
The first paragraph introduces the concept of implicit differentiation as a method to differentiate functions that are not explicitly written in terms of 'y ='. It contrasts this with explicit differentiation, which is the standard approach for functions written explicitly in terms of 'y ='. The explanation includes an example of how to differentiate a term with 'y' in it, emphasizing the use of the Chain Rule and the necessity of multiplying by dy/dx to account for the 'y' term's dependence on 'x'. The paragraph outlines the two main steps for implicit differentiation: differentiating both sides with respect to 'x' and then solving for dy/dx, highlighting the need for algebraic manipulation to isolate dy/dx.
📈 Differentiating Both Sides and Isolating dy/dx
This paragraph delves into the process of differentiating both sides of an equation when dealing with implicit differentiation. It explains that the derivative of a constant is zero and illustrates how to isolate dy/dx by performing algebraic operations such as distributing, subtracting, and dividing. The paragraph provides a step-by-step breakdown of how to handle terms without dy/dx and how to manipulate the equation to get dy/dx alone on one side. It also touches on the use of the Product Rule and Chain Rule for more complex terms involving 'y' and emphasizes the importance of correctly applying these rules to arrive at the correct solution for dy/dx.
🚫 Common Pitfalls in Implicit Differentiation
The final paragraph serves as a cautionary guide, highlighting common mistakes that people make when performing implicit differentiation. It stresses the importance of using the Product Rule even when 'y' appears to be treated as a constant. The paragraph also points out the need to factor when dy/dx appears more than once and reminds learners that dy/dx can sometimes be written as y prime, which is equivalent. The speaker aims to help viewers avoid these pitfalls and successfully apply implicit differentiation techniques, concluding with an encouragement for viewers to engage with the content by liking or subscribing.
Mindmap
Keywords
💡Implicit Differentiation
💡Explicit Differentiation
💡Power Rule
💡Chain Rule
💡Derivative
💡Algebraic Manipulation
💡Product Rule
💡Constant
💡Term
💡Factoring
💡Simplifying
Highlights
Introduction to implicit differentiation, a method for differentiating equations that are not explicitly written in terms of y as a function of x.
Comparison between explicit differentiation (traditional 'y =' form) and implicit differentiation, emphasizing the need for the latter when the function is not in 'y =' form.
Explanation of the first step in implicit differentiation: differentiating both sides of the equation with respect to x and attaching a dy/dx to terms containing y.
Clarification on the use of the Chain Rule in implicit differentiation, multiplying terms containing y by dy/dx to account for the inner function of x.
The second step in implicit differentiation: isolating dy/dx on one side of the equation to solve for the derivative.
Example of implicit differentiation applied to the equation x^2 + y^2 = 36, demonstrating the process of differentiating both sides and isolating dy/dx.
Explanation of the Product Rule and its application in implicit differentiation when dealing with terms like x^2y.
Illustration of how to handle more complex equations involving the Product and Chain Rules, using the example of x^2y * sin(x) + y^2 * cos(x) = 10x.
Advice on distributing and expanding terms to isolate dy/dx, emphasizing the importance of including all terms within parentheses.
Common pitfalls in implicit differentiation, such as forgetting to use the Product Rule or factor when dy/dx appears more than once.
Mention of alternative notation for dy/dx, such as y prime, and assurance that it represents the same concept.
The importance of implicit differentiation as a tool for solving problems where the function is not explicitly given in terms of y as a function of x.
The process of implicit differentiation being a special kind of Chain Rule application, highlighting the relationship between the outer and inner functions.
The necessity to account for the x-dependency of y when differentiating terms containing y, which is not the case in explicit differentiation.
The role of algebraic manipulation in solving for dy/dx, particularly when the equation is more complex and requires distributing and factoring.
The step-by-step approach to implicit differentiation, providing a clear and structured method for tackling such problems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: