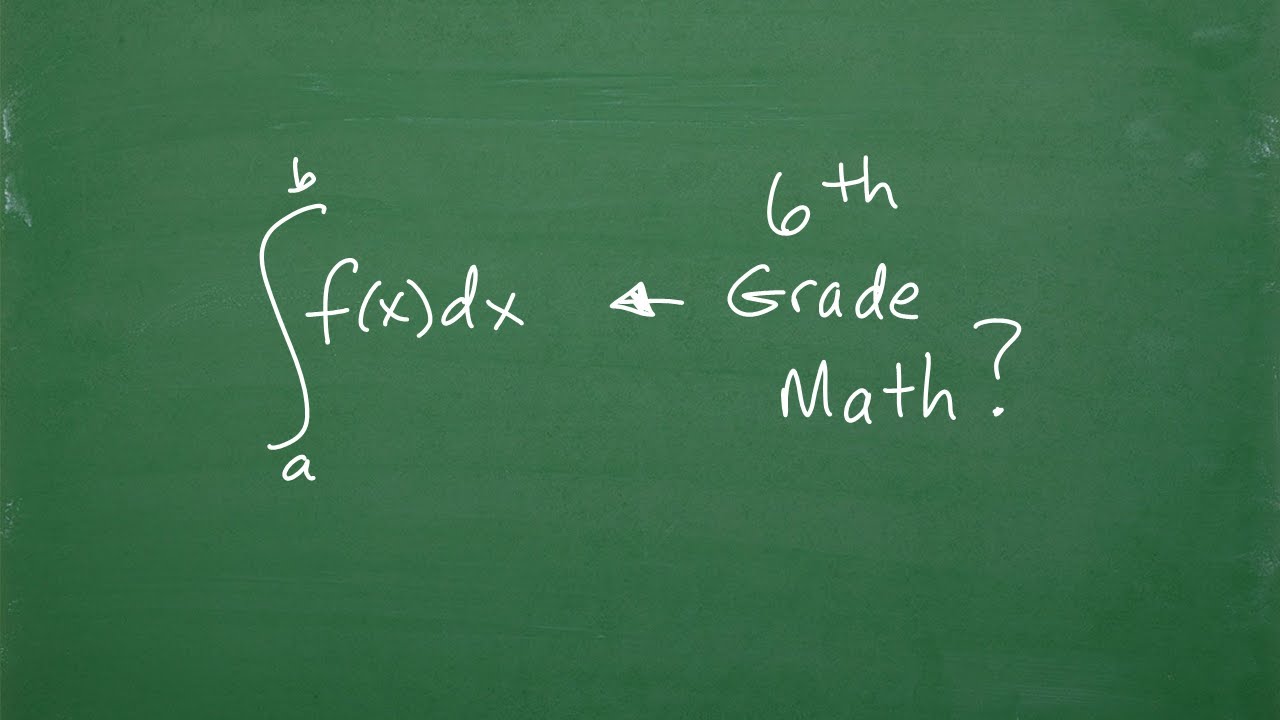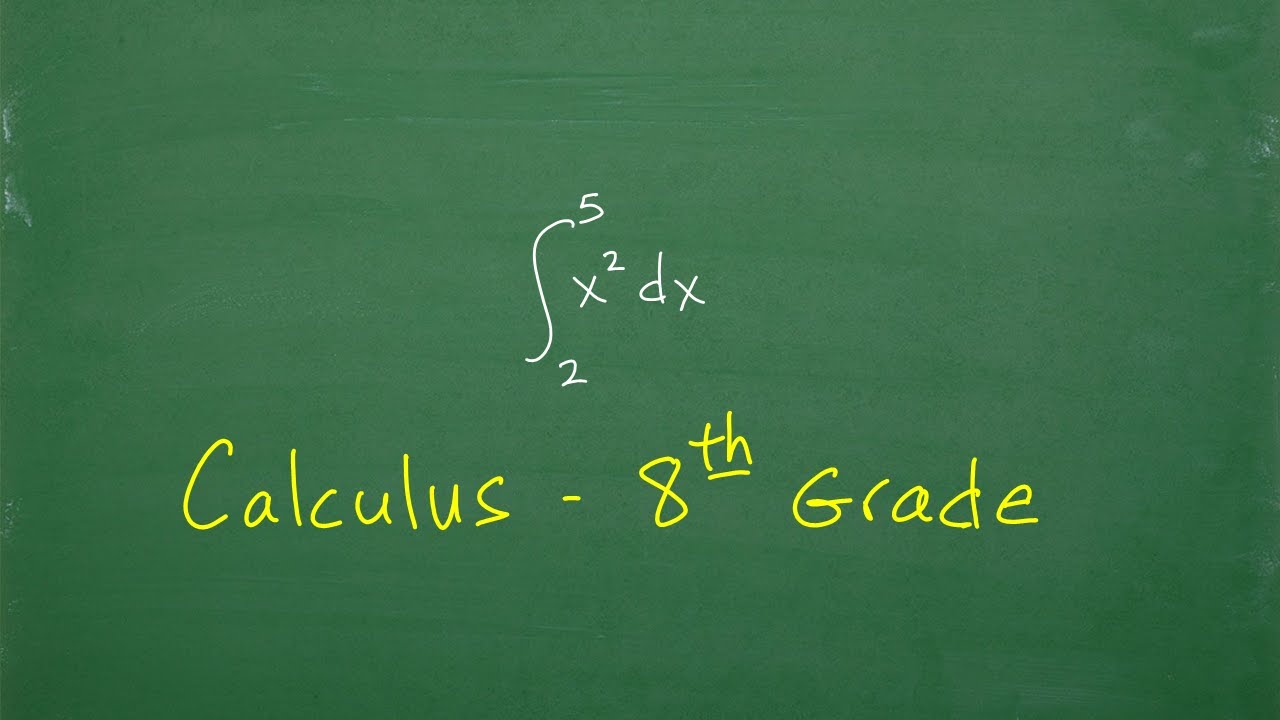Why is calculus so ... EASY ?
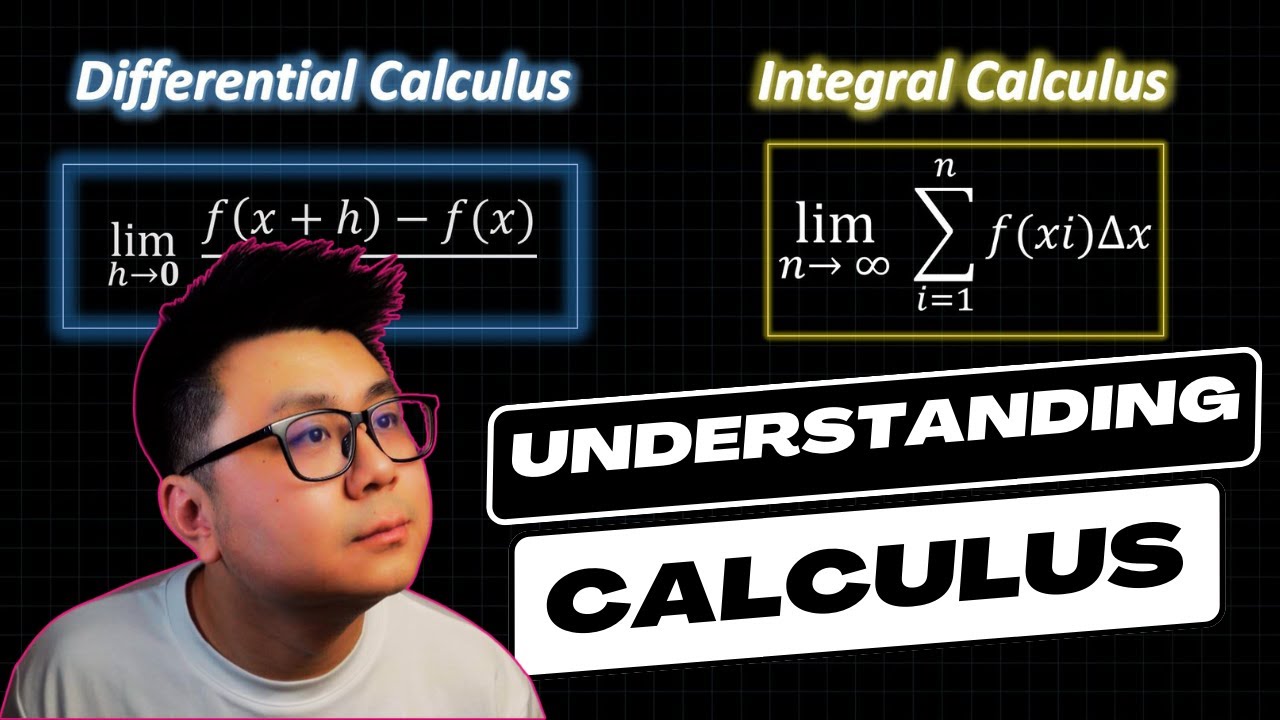
TLDRThe video script demystifies calculus by illustrating its fundamental concepts through everyday examples, such as using a car's speedometer and odometer to explain differential and integral calculus. It emphasizes that calculus is an extension of basic algebra and introduces the audience to key principles, including the Fundamental Theorem of Calculus. The script also discusses the notation system developed by Gottfried Wilhelm Leibniz, which simplifies the process of differentiating and integrating functions. By the end, viewers are left with a newfound appreciation for the elegance and accessibility of calculus.
Takeaways
- 📚 Calculus is simpler than perceived, with its core concepts being akin to basic algebra.
- 🚗 The analogy of a car's speedometer and odometer demonstrates the principles of differential and integral calculus.
- 📈 The area under a speed vs. time curve represents the distance traveled, which is a fundamental concept in integral calculus.
- 🔄 The Fundamental Theorem of Calculus links the concepts of differentiation and integration, the two main branches of calculus.
- 🧠 Understanding calculus can be made accessible even to those without extensive mathematical backgrounds.
- 📊 The concept of derivatives can be applied to a wide range of functions, including powers, exponentials, and trigonometric functions.
- 🔧 The rules for finding derivatives, such as the product, quotient, and chain rules, are essential for advanced calculus operations.
- 🌐 The notation system introduced by Gottfried Wilhelm Leibniz is a powerful tool for expressing calculus concepts and operations.
- 🎓 Even with a basic understanding of calculus, one can perform impressive mathematical feats and solve complex problems.
- 🚀 The video script aims to demystify calculus and inspire interest in the subject through its simplified explanations and practical applications.
Q & A
What is the main message the video tries to convey about calculus?
-The video aims to show that calculus, often perceived as a complex and high-level mathematical concept, can actually be simplified and understood as an extension of basic algebra. It emphasizes the simplicity at the core of calculus and seeks to demystify it through relatable examples and explanations.
How did the author first learn about calculus?
-The author was introduced to calculus as a young teenager through the book 'Calculus Made Easy' by Silvanus P. Thompson, which presents the subject in a simple and accessible manner.
What is the significance of the book 'Calculus Made Easy' by Silvanus P. Thompson?
-The book 'Calculus Made Easy' is significant because it has been instrumental in popularizing the understanding of calculus by presenting it in an easy-to-understand format. Published in 1910, it has remained in print for over a century and has sold over a million copies, indicating its enduring influence and effectiveness in teaching calculus.
How does the video relate cars to the concept of calculus?
-The video uses cars as an analogy to explain the fundamental concepts of calculus. It demonstrates how the speedometer and odometer of a car can be used to perform calculations related to differential and integral calculus, showing that calculus can be applied to everyday situations.
What is the core idea behind differential calculus?
-The core idea behind differential calculus is to find the rate of change (slope) of a function at a particular point, which can be thought of as the slope of the tangent line to the curve at that point. This process is also known as differentiating a function.
What is the relationship between distance and speed in the context of the video's explanation?
-In the context of the video, the relationship between distance and speed is such that the distance traveled by a car is equivalent to the area under the speed-time curve. This concept is used to explain the fundamental theorem of calculus, which connects differentiation and integration.
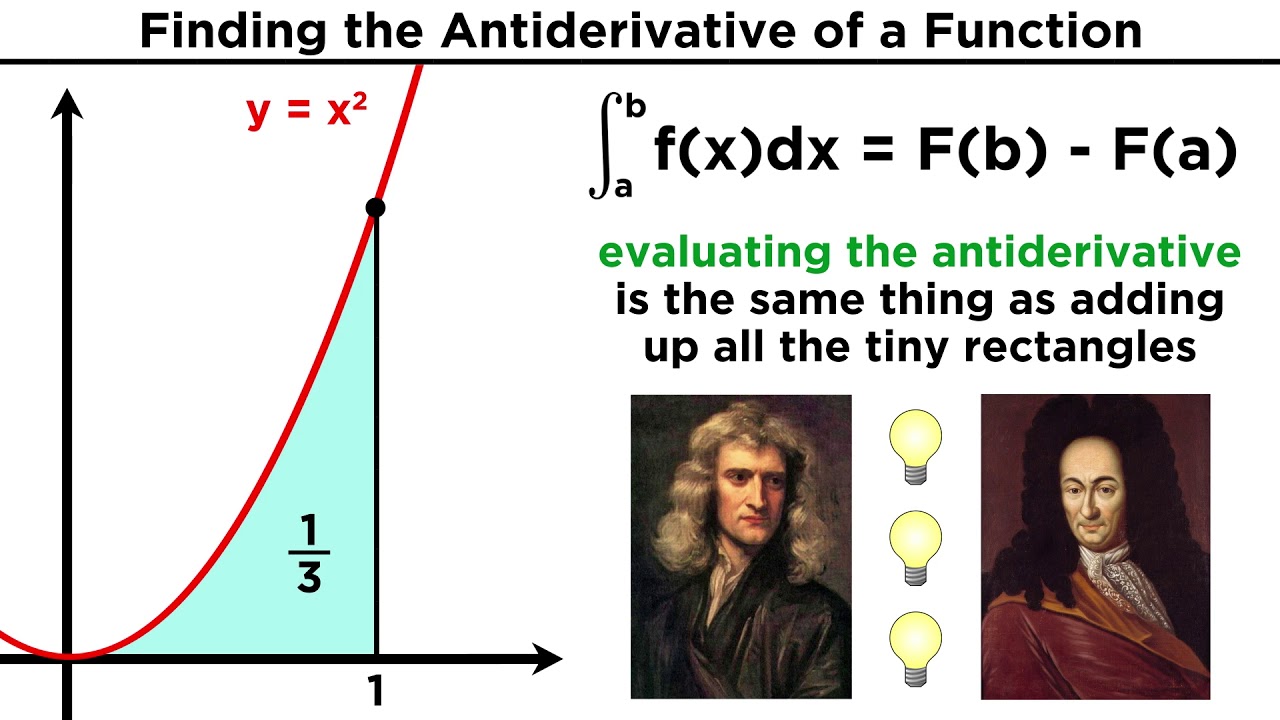
What is the fundamental theorem of calculus?
-The fundamental theorem of calculus establishes a connection between differentiation and integration. It essentially states that every integral function can be obtained by integrating its derivative, and vice versa. This theorem is considered the 'soul of calculus' and is crucial for understanding the subject as a whole.
How does the video address the concept of integral calculus?
-The video addresses integral calculus by discussing the process of finding anti-derivatives, which are functions that, when differentiated, yield the original function. It uses the analogy of a car's odometer to explain how integral calculus can be used to calculate areas under curves, representing the accumulation of change over time.
What are some of the elementary functions mentioned in the video?
-The video mentions several elementary functions, including constant functions, powers of x (like x^n), exponential functions, and trigonometric functions (like sine and cosine). These functions form the basis for more complex mathematical expressions and are fundamental in calculus.
How does the video explain the process of differentiation?
-The video explains differentiation by using the concept of finding the slope of the tangent line to a curve at a particular point. It also introduces the notion of derivatives and how they can be calculated for various types of elementary functions using a set of rules, such as the sum rule, product rule, and chain rule.
What is the role of notation in calculus as highlighted in the video?
-The video highlights the role of notation, particularly the use of 'd' and 'dx' introduced by Gottfried Wilhelm Leibniz, in simplifying and extending school algebra to handle calculus. This notation allows for a more intuitive understanding and manipulation of calculus concepts.
Outlines
📘 Introduction to Calculus
The video begins with an introduction to calculus, addressing the common misconception that it is an overly complicated subject. The speaker shares their personal experience of learning calculus at a young age through a book titled 'Calculus Made Easy' by Silvanus P. Thompson, which has remained popular for over a century. The speaker aims to present their own simplified version of calculus in this video, making it accessible to both novices and experts. The video is dedicated to the speaker's children, Lara and Karl.
🚗 Calculus and Cars
The speaker creatively uses the analogy of a car to explain the fundamentals of calculus. By relating the car's speedometer and odometer to the concepts of differential and integral calculus, the speaker simplifies the idea of translating speed (rate of change) into distance (accumulated change). The video explains how the area under a speed-time graph represents the distance traveled, and how the slope of a distance-time graph represents speed. This analogy is used to introduce the core ideas of differential calculus, showing how the peaks and valleys in a distance-time graph correspond to zeros in the speed-time graph.
📚 The Fundamental Theorem of Calculus
The speaker delves into the Fundamental Theorem of Calculus, emphasizing its importance as the 'soul of calculus'. The theorem links the concepts of differentiation (bottom to top) and integration (top to bottom), highlighting the relationship between the slope of a curve and the area under it. The speaker explains how this theorem allows for the translation between distance and speed, and how it can be applied to a variety of functions, not just those with a constant slope. The video also touches on the idea that the derivative of every elementary function is also an elementary function, making the process of finding derivatives relatively straightforward.
📝 Derivatives of Elementary Functions
This paragraph focuses on the derivatives of elementary functions, providing a list of common functions and their corresponding derivatives. The speaker explains how the derivative of a constant function is zero, the derivative of a power function is the power minus one, and the derivative of trigonometric functions like sine and cosine are each other. The speaker also introduces the concept of the natural logarithm and the exponential function, noting their unique derivatives. The paragraph emphasizes the simplicity of finding derivatives for elementary functions and introduces the idea of using basic algebraic operations to find the derivatives of more complex functions.
🔄 Derivative Rules and Anti-Derivatives
The speaker discusses the rules for finding the derivatives of more complex functions by combining simpler ones. The paragraph introduces the product rule, sum rule, and quotient rule, showing how they can be used to find the derivatives of functions that are the result of basic operations on elementary functions. The speaker also addresses the concept of anti-derivatives, explaining how they can be found by reading the list of derivatives in reverse. The paragraph highlights the usefulness of these rules and the power they provide in solving calculus problems, while also acknowledging the limitations and complexities that arise when dealing with certain elementary functions.
🌀 Challenges in Calculus and Leibnitz Notation
The speaker acknowledges the challenges in calculus, particularly with functions that do not have elementary antiderivatives and the difficulty in determining which functions do. The paragraph discusses the absence of an 'elementary' chain rule for antiderivatives and the problems this presents. The speaker then introduces Leibnitz notation, a powerful system that allows for the extension of school algebra to calculus. The video demonstrates how this notation can be used to derive the rules of calculus and perform calculations efficiently, including the product rule and the concept of the anti-derivative denoted by the integral sign.
Mindmap
Keywords
💡Calculus
💡Differential Calculus
💡Integral Calculus
💡Speedometer and Odometer
💡Derivative
💡Anti-derivative
💡Fundamental Theorem of Calculus
💡Gottfried Wilhelm Leibniz
💡Silvanus P. Thompson
💡Elementary Functions
💡Chain Rule
Highlights
Calculus, often perceived as a complex subject, can be simplified to a level comparable to basic algebra when viewed correctly.
The book 'Calculus Made Easy' by Silvanus P. Thompson has been effective in demonstrating the simplicity of calculus, selling over a million copies since its publication in 1910.
The video aims to present a fresh perspective on calculus that is accessible and enjoyable for both novices and experts.
A car can be considered a calculus machine, using its speedometer and odometer to perform differential and integral calculus by driving and repurposing these tools.
The core of differential calculus can be taught to a monkey, indicating its simplicity, while integral calculus has more complex aspects.
The distance traveled by a car can be calculated by understanding the area under the speed/time curve, which is a fundamental concept in calculus.
The speed of a car at any time can be determined by the slope of the tangent line to the speed/time curve at that point.
The Fundamental Theorem of Calculus establishes the relationship between the area under a curve (distance) and the slope of the curve (speed), which is crucial in understanding calculus.
The process of finding the maximum and minimum values (peaks and valleys) in a curve can be simplified by understanding the concept of differential calculus.
The derivative of a function is a straightforward process for a vast array of functions, including powers, exponential functions, and trigonometric functions.
The derivative of a product of functions can be calculated using the product rule, which is a fundamental operation in differential calculus.
Elementary functions can be constructed from basic functions using addition, subtraction, multiplication, division, and substitution.
The derivative of every elementary function is also an elementary function, which is a powerful property that makes calculus extremely useful.
The process of finding the derivative of a function is automatic and can be compared to algebra autopilot, showcasing the user-friendly nature of calculus.
The notation used in calculus, introduced by Gottfried Wilhelm Leibniz, allows for an extension of school algebra and has been streamlined over the years for ease of use.
The video includes an animation that derives all the most important formulas of calculus, encapsulating the essence of the subject in just five minutes.
The concept of differential calculus can be used to find the maxima and minima of functions by identifying the zeros of the derivative, which is a practical application in various fields.
The video discusses the challenges in finding antiderivatives for certain elementary functions and the absence of elementary counterparts for some calculus rules.
The video concludes with a demonstration of Leibniz's notation in action, showcasing its power in deriving calculus rules and performing calculations efficiently.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: