High School Physics - Standing Waves
TLDRIn this informative session, Mr. Fullerton elucidates the concept of standing waves, emphasizing their formation through the interaction of waves with identical frequency and amplitude moving in opposite directions. He explains the difference between nodes, points of zero amplitude, and antinodes, where amplitude is maximized. The discussion highlights the relevance of standing waves in musical instruments, altering pitch through wavelength adjustments. The session progresses with practical examples, including a rope experiment, to demonstrate wave properties and calculations, fostering a deeper understanding of wave mechanics.
Takeaways
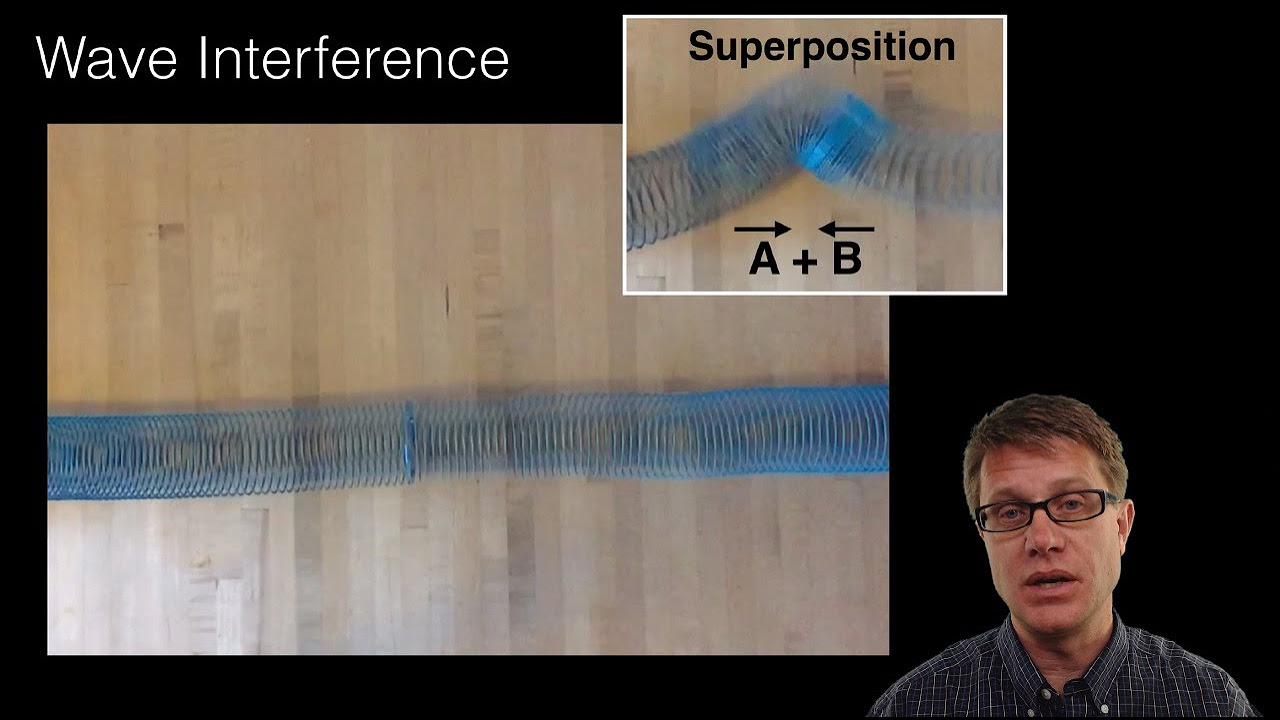
- 🌟 Standing waves are formed by the interaction of two waves with the same frequency and amplitude traveling in opposite directions.
- 🎵 Nodes in a standing wave are points that appear to be standing still, resulting from destructive interference.
- 💥 Antinodes are points of maximum vibration amplitude in a standing wave, indicating constructive interference.
- 📈 The number of antinodes in a standing wave is always one less than the number of nodes.
- 🎻 Musical instruments such as guitars, pianos, and flutes rely on the principles of standing waves to function.
- 🤔 When analyzing a standing wave, the presence of nodes and antinodes can help determine the wave's characteristics.
- 🌐 In a standing wave, the wavelength can be deduced from the observable pattern, such as seeing half a wavelength.
- 🔄 The frequency of a standing wave is determined by the source of the wave, such as a rotating drill in the example provided.
- 📏 The speed of a wave can be calculated using the wave equation: velocity equals frequency times wavelength.
- 🔧 Practical applications of standing wave analysis include adjusting the pitch of musical instruments by altering the wavelength of the string.
- 💡 Understanding standing waves is essential for comprehending wave behavior and its applications in various fields.
Q & A
What is a standing wave?
-A standing wave is a wave pattern that appears to be stationary, formed by the interaction of two waves with the same frequency and amplitude traveling in opposite directions.
What are the distinguishing features of nodes and antinodes in a standing wave?
-Nodes are points in a standing wave that appear to be standing still, with no movement, and they result from destructive interference, leading to zero amplitude. Antinodes are points that vibrate with maximum amplitude, above and below the axis of the wave.
How is the number of antinodes related to the number of nodes in a standing wave?
-There is always one less antinode than nodes in a standing wave. This is because even though another antinode position may appear, the wave is only in one of those positions at any given time, so it is not counted twice.
What role do standing waves play in musical instruments?
-Standing waves are crucial in the functioning of musical instruments. They are used to produce sound in a variety of instruments, such as guitars, pianos, drums, flutes, trombones, xylophones, pipe organs, and harps. The standing wave pattern is adjusted to change the wavelength and frequency, which in turn alters the pitch of the sound produced.
How does fretting a guitar string affect the standing wave pattern and pitch?
-Fretting a guitar string changes the effective length of the string, which in turn alters the wavelength of the standing wave pattern. This change in wavelength results in a change in the frequency of the standing wave, and consequently, the pitch of the note produced by the string is higher or lower depending on the adjustment.
In the example of two children creating a standing wave in a rope, what is the significance of the wavelength?
-The wavelength is significant as it determines the frequency and the pitch of the standing wave. In the example, one full wavelength is observed between the drill and the wall, which is 3 meters long. This wavelength is used to calculate the speed of the wave in the rope.
How can the speed of the wave in the rope be calculated?
-The speed of the wave in the rope can be calculated using the wave equation, which is velocity equals frequency times wavelength. Given the frequency (20 Hertz) and the wavelength (3 meters), the speed of the wave is 60 meters per second (20 Hertz * 3 meters).
What is the relationship between the frequency of the waves and the standing wave formed?
-For a standing wave to be formed, the two interacting waves must have the same frequency. This is essential for the consistent formation of nodes and antinodes, and for the wave pattern to remain stationary.
How does the amplitude of the interacting waves affect the standing wave?
-The amplitude of the standing wave is determined by the amplitudes of the two interacting waves. If the waves have the same amplitude, the resulting standing wave will also have that amplitude at the points of constructive interference (antinode positions).
What is destructive interference and how does it result in the formation of nodes?
-Destructive interference occurs when two waves meet in such a way that they cancel each other out, leading to a reduction in amplitude. At the points of destructive interference, the amplitude is zero, resulting in the formation of nodes, which are points of no movement in the standing wave.
In the context of the rope and drill example, how does the mass attached to the rope affect the standing wave?
-The mass attached to the rope does not directly affect the formation of the standing wave in terms of nodes and antinodes, as these are determined by the wavelength and frequency. However, the mass, along with the tension in the rope, can affect the speed at which the waves travel through the rope, which in turn can influence the wavelength and frequency of the standing wave.
Outlines
🌟 Introduction to Standing Waves
This paragraph introduces the concept of standing waves, explaining that they are formed by waves of the same frequency and amplitude traveling in opposite directions. It highlights the difference between nodes, which appear stationary, and antinodes, which have maximum vibration amplitude. The paragraph emphasizes the relevance of standing waves in musical instruments, as they are essential for the functioning of a wide range of instruments, from stringed to percussion and wind instruments. It also presents a sample problem involving the creation of a standing wave in a rope, demonstrating the relationship between wavelength and the physical setup.
Mindmap
Keywords
💡Standing Waves
💡Nodes
💡Antinodes
💡Frequency
💡Amplitude
💡Wavelength
💡Destructive Interference
💡Constructive Interference
💡Wave Equation
💡Musical Instruments
💡Variable-Speed Drill
Highlights
Standing waves are formed by waves of the same frequency and amplitude traveling in opposite directions.
Nodes are points on a standing wave that appear to be standing still.
Antinodes are points on a standing wave that vibrate with maximum amplitude.
The number of antinodes is always one less than the number of nodes in a standing wave.
Many musical instruments, such as guitars, pianos, and flutes, rely on the principles of standing waves.
Fretting a guitar string changes the wavelength of the string and the frequency of the standing wave pattern.
When two children create a standing wave in a rope, the wavelength can be determined from the diagram.
Wave Y, interacting with Wave X to produce a standing wave, must travel in the opposite direction with the same frequency and amplitude.
Point A on the standing wave is identified as a node, resulting from destructive interference.
Destructive interference results in a node with zero amplitude.
The wavelength of the standing wave can be determined by observing one full wavelength between the drill and the wall.
The speed of the wave in the rope can be calculated using the wave equation, velocity equals frequency times wavelength.
A variable-speed drill and a 5 kg mass can be used to set up standing waves in a rope.
The rope draped over a hook on a wall is used to study the properties of standing waves.
The frequency of the standing wave in the rope is 20 Hertz.
The wavelength of the standing wave is 3 meters, as seen in the diagram.
The velocity of the wave in the rope is calculated to be 60 meters per second.
Understanding standing waves is crucial for studying wave properties and their applications in various fields.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: