Standing Waves

Super Awesome Fun Times with Physics

5 Jun 201825:54

EducationalLearning

32 Likes 10 Comments

TLDRThe video script delves into the fascinating world of standing waves, focusing on their fundamental properties and how they are formed by the superposition of two waves with the same amplitude and frequency, but traveling in opposite directions. The discussion primarily revolves around standing waves on a string and in a pipe, emphasizing the importance of boundary conditions in determining the allowable frequencies and wavelengths. The script explores different scenarios, such as fixed and loose ends for strings, and closed and open ends for pipes, illustrating how these conditions affect the wave patterns. By examining various boundary conditions, the presenter demonstrates how to deduce the general form for all permitted frequencies and wavelengths. The summary also touches on the representation of sound waves in terms of displacement and pressure, and how these relate to the nodes and antinodes in the system. The script concludes by showing that the allowed wavelengths can be derived from the boundary conditions and further used to calculate the corresponding frequencies, providing a comprehensive understanding of standing wave phenomena.

Takeaways

🌊 Standing waves oscillate in time but do not propagate, hence the name 'standing' as opposed to 'traveling' waves.
🔁 Standing waves are created by the superposition of two waves with the same amplitude and frequency, but traveling in opposite directions.
📍 Boundary conditions are critical for solving for the allowed wavelengths or frequencies in standing waves, particularly for strings and pipes.
🎼 For strings, two types of boundary conditions are considered: fixed ends (zero displacement, nodes) and loose ends (derivative of wave function vanishes, pressure antinodes).
📏 The general form for allowed frequencies and wavelengths can be deduced by drawing and analyzing standing wave patterns under different boundary conditions.
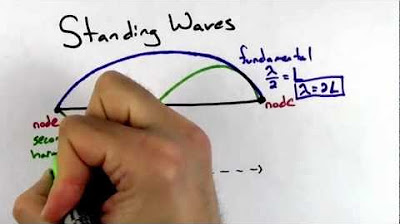
📉 In the case of fixed ends for strings, there is a node at each end, and allowed solutions can be represented by sine or cosine waves fitting within the string's length.
📚 For pipes, two types of boundary conditions are considered: closed pipes (displacement nodes) and open pipes (pressure nodes or zero pressure at the ends).
🔄 The displacement and pressure representations of sound waves in pipes are related, with nodes and antinodes swapping roles between the two representations.
🔢 The allowed wavelengths for standing waves can be expressed as integer multiples of the wavelength over two, based on the boundary conditions.
🎓 For mixed boundary conditions (one fixed, one loose end for strings), the allowed wavelengths can be described using odd integers or alternatively as (2n - 1) times lambda over 4, where n is an integer.
🧮 The allowed frequencies can be found by using the relationship between wave velocity, wavelength, and frequency, once the allowed wavelengths are determined.

Q & A

What are standing waves?
-Standing waves are oscillating waves that do not propagate. They are formed by the superposition of two waves with the same amplitude and frequency, but traveling in opposite directions.
What are the two types of boundary conditions for waves on a string?
-The two types of boundary conditions for waves on a string are fixed ends, where the displacement is zero, and loose ends, where the partial derivative of the wave function with respect to x vanishes, indicating no slope at the boundary.
How are standing waves created in a pipe?
-Standing waves in a pipe are created by enforcing boundary conditions at the ends of the pipe. There are two types of conditions: closed pipes, which have zero displacement (like fixed ends on a string), and open pipes, where the pressure goes to zero (equivalent to the derivative of displacement going to zero).
What is a node in the context of standing waves?
-A node is a point in a standing wave where the displacement function is zero at all times. It is a location of no vibration and is often represented by a dot in diagrams of wave patterns.
How can you determine the allowed wavelengths or frequencies for standing waves?
-The allowed wavelengths or frequencies for standing waves can be determined by enforcing the specific boundary conditions and observing the pattern of nodes and antinodes that form. The general form for all allowed frequencies and wavelengths can be deduced by drawing and analyzing the wave patterns under different boundary conditions.
What is the relationship between the length of a pipe and the allowed wavelengths for standing waves with open-open boundary conditions?
-For standing waves with open-open boundary conditions, the length of the pipe (L) is related to the wavelength (λ) by the formula L = nλ/2, where n is an integer representing the harmonic number.
How do the boundary conditions for mixed cases (one fixed end and one loose end) differ from those with both ends fixed or both ends loose?
-In mixed cases, the allowed wavelengths are fractions of the full wavelength, specifically a quarter, three-quarters, and five quarters for the first three harmonics. This contrasts with both ends fixed or both ends loose, where the length of the medium is an integer multiple of half a wavelength.
What is the significance of the pressure antinode in standing waves?
-A pressure antinode is a point in a standing wave where the displacement is at its maximum value. It is significant because it represents a location of maximum vibration or energy transfer, which is important for understanding the behavior of waves, especially in the context of sound waves in a pipe.
How can you represent standing waves using pressure instead of displacement?
-Standing waves can be represented using pressure by noting that where there is a displacement node (zero displacement), there is a pressure antinode (maximum pressure), and vice versa. This dual representation is useful for visualizing and understanding the behavior of sound waves in pipes with different boundary conditions.
What is the formula that relates the velocity, wavelength, and frequency of a wave?
-The formula that relates the velocity (v), wavelength (λ), and frequency (f) of a wave is v = λf. This relationship is used to calculate the allowed frequencies for standing waves once the allowed wavelengths have been determined.
How can you find the first three harmonics for standing waves on a string with both ends fixed?
-For standing waves on a string with both ends fixed, the first three harmonics can be found by placing nodes at both ends and adding additional nodes at a quarter wavelength, a half wavelength, and three-quarters wavelength from one end to the other, respectively.
What is the general formula for the length of a medium in relation to the wavelength for standing waves with mixed boundary conditions?
-For standing waves with mixed boundary conditions (one fixed end and one loose end), the general formula relating the length of the medium (L) to the wavelength (λ) can be written as L = (2n - 1)λ/4, where n is an integer.

Outlines

00:00

🌊 Introduction to Standing Waves

The video begins with an introduction to standing waves, which oscillate in time but do not propagate. Standing waves are created by the superposition of two waves with the same amplitude and frequency, but traveling in opposite directions. The focus is on solving for the allowed frequencies and wavelengths under various boundary conditions for waves on a string and in a pipe.

05:00

📍 Boundary Conditions for Strings and Pipes

The video explains the importance of enforcing boundary conditions to solve for the allowed wavelengths and frequencies. Two types of boundary conditions for strings are discussed: fixed ends, where the string displacement is zero, and loose ends, where the derivative of the wave function with respect to position must vanish. For sound waves in pipes, closed pipes have zero displacement (nodes), while open pipes have zero pressure (antinodes). The video emphasizes that these boundary conditions are fundamentally similar for both strings and pipes.

10:02

📈 Visualizing Standing Wave Solutions

The presenter illustrates how to visualize standing wave solutions by drawing different configurations based on boundary conditions. For strings with fixed ends, nodes are placed at each end, and the allowed waveforms are shown with varying numbers of nodes in between. The video also covers scenarios with one fixed and one loose end, as well as both ends being loose, each with unique waveform solutions. The concept of nodes and antinodes is further explained through these visual representations.

15:06

🎼 Sound Waves in Pipes: Displacement and Pressure

The video moves on to discuss sound waves in pipes, highlighting two common ways to represent them: by displacement or by pressure. For closed-end pipes, displacement nodes and pressure antinodes are explained, and the relationship between the two is clarified. The presenter also addresses the representation of open-end pipes, where pressure nodes and displacement antinodes are present. The importance of understanding these representations is emphasized for solving standing wave problems.

20:09

🔢 Determining Allowed Frequencies and Wavelengths

The presenter demonstrates how to determine the allowed frequencies and wavelengths for standing waves by analyzing the drawn diagrams. For fixed-end strings and closed pipes, a pattern emerges where the length of the medium is related to the wavelength by an integer multiple of half-wavelengths. For open-open boundary conditions, the pattern is similar, with the length being a multiple of half-wavelengths. Mixed boundary conditions, such as one fixed and one loose end, exhibit a different pattern involving odd integer multiples of a quarter-wavelength. The video guides viewers in deriving these relationships and understanding the underlying principles.

25:09

🧮 Calculating Frequencies Using Wave Velocities

The final paragraph explains how to calculate the allowed frequencies for standing waves using the relationship between wave velocity, wavelength, and frequency. Since the velocity is constant for a given medium, the frequency can be found by solving for the wavelength from the boundary condition equations and then substituting it into the velocity equation. The video concludes by summarizing the method for solving standing wave problems for different boundary conditions on strings and in pipes.

Mindmap

Keywords

💡Standing Waves

Standing waves are oscillating waves that do not propagate or move through space. They are created by the superposition of two waves with the same frequency and amplitude but traveling in opposite directions. In the context of the video, standing waves are a central theme as they discuss their properties and how they are influenced by different boundary conditions, such as those found on strings or within pipes.

💡Boundary Conditions

Boundary conditions are constraints applied to physical systems to define the behavior of waves at the edges of their medium. In the video, different types of boundary conditions, such as fixed ends and loose ends for strings, and closed or open ends for pipes, are crucial in determining the allowed wavelengths and frequencies of standing waves. The script explores how these conditions affect the formation and characteristics of standing waves.

💡Fixed Ends

Fixed ends refer to the condition where the ends of a string or pipe are held in place and do not move. This is exemplified in the video by the description of a guitar string fixed at both ends, leading to a node—a point of zero displacement—at each end. Fixed ends are a key boundary condition for creating standing waves, as they influence the possible wavelengths and frequencies that can exist on the string.

💡Loose Ends

Loose ends describe a boundary condition where one end of a string is free to move, resulting in a pressure antinode—a point of maximum displacement—at that end. The video script uses the example of a string attached to a loop around a pole to illustrate loose ends. This condition is significant as it affects the types of standing wave patterns that can form on the string.

💡Nodes

In the context of standing waves, nodes are points where the displacement of the wave is zero at all times. The video explains that in a string with fixed ends, there is a node at each end, and these nodes are represented by pink dots in the illustrations. Nodes are essential in determining the allowed harmonics and frequencies of standing waves on strings or within pipes.

💡Antinodes

Antinodes are points of maximum displacement in a standing wave system. The video script mentions pressure antinodes, which occur at the ends of a string with loose ends or at the open ends of a pipe. Antinodes are illustrated with blue dotted lines and are used to describe the behavior of standing waves under different boundary conditions.

💡Harmonics

Harmonics, in the context of standing waves, refer to the different allowed patterns or solutions that can form based on the boundary conditions. The video script discusses the first few harmonics, which are the simplest standing wave patterns, such as a half-wavelength, a full wavelength, and so on, between the fixed nodes. These harmonics are crucial for understanding the vibrational modes of strings and pipes.

💡Wavelength

Wavelength is the distance between two successive points in a wave that are in the same phase, such as from one crest to the next crest. In the video, the wavelength is used to describe the length of the standing wave patterns that fit within the boundaries of the string or pipe. The allowed wavelengths are determined by the boundary conditions and are key to calculating the frequencies of the standing waves.

💡Frequency

Frequency refers to the number of oscillations or cycles a wave completes in a unit of time. The video script discusses how the allowed frequencies of standing waves are derived from the allowed wavelengths, using the relationship between wave velocity, wavelength, and frequency. The calculation of frequencies is essential for understanding the vibrational modes of the system.

💡Velocity

Velocity, in the context of waves, is the speed at which a wave propagates through a medium. The script mentions that the velocity of the wave is constant for a given medium, which is important when calculating the allowed frequencies of standing waves using the relationship between wavelength, frequency, and velocity.

💡Displacement

Displacement is the change in position of a point in a wave from its equilibrium position. In the video, displacement is used to describe the movement of air molecules in a pipe or the movement of a string as it vibrates. Displacement is a fundamental concept for understanding how standing waves form and behave under different boundary conditions.

Highlights

Standing waves are waves that oscillate in time but do not propagate, hence the term 'standing'.

Standing waves are created by the superposition of two waves with the same amplitude and frequency, but traveling in opposite directions.

Two primary types of media for standing waves are waves on a string and standing sound waves in a pipe.

Boundary conditions are critical for solving for allowed wavelengths or frequencies in standing waves.

Fixed ends in strings, such as on a guitar, have zero displacement, resulting in nodes at the ends.

Loose ends in strings have a soft boundary where the slope of the waveform (partial derivative with respect to x) must vanish.

For sound waves in pipes, closed pipes have zero displacement (nodes), while open pipes have zero pressure (antinodes).

The boundary conditions for strings and sound waves are fundamentally similar despite different physical interpretations.

Allowed wavelengths and frequencies can be deduced by applying boundary conditions to the wave equations.

Harmonics or wavelengths for standing waves can be visualized by adding nodes between fixed ends.

Mixed boundary conditions (one fixed, one loose end) in strings allow for unique wave patterns involving pressure antinodes.

The general form for all allowed frequencies and wavelengths can be derived from the patterns observed in standing wave diagrams.

For closed pipes, the allowed wavelengths are in the form of L = (2n - 1)λ/4, where n is an integer.

Open pipes exhibit allowed wavelengths in the form of L = nλ/2, where n is an integer.

The relationship between the length of the medium and the wavelength is key to determining the allowed frequencies.

The velocity of the wave in the medium is constant across all cases, allowing for the calculation of frequency from wavelength.

Understanding the behavior of standing waves has practical applications in musical instruments and acoustics.

The method of solving standing wave problems involves a combination of mathematical analysis and physical intuition.

Transcripts

Browse More Related Video

Standing Waves on a String, Fundamental Frequency, Harmonics, Overtones, Nodes, Antinodes, Physics

Standing waves on strings | Physics | Khan Academy

AP Physics 1 - Standing Waves in Instruments

Waves part 2 (Double Slit, Single Slit, and Standing Waves)

The Physics of Music: Crash Course Physics #19

Mechanical Waves

Related Tags

Standing Waves Wave Harmonics Boundary Conditions String Theory Acoustic Physics Wave Patterns Frequency Analysis Wavelength Calculation Physics Education Sinusoidal Waves Sound Waves