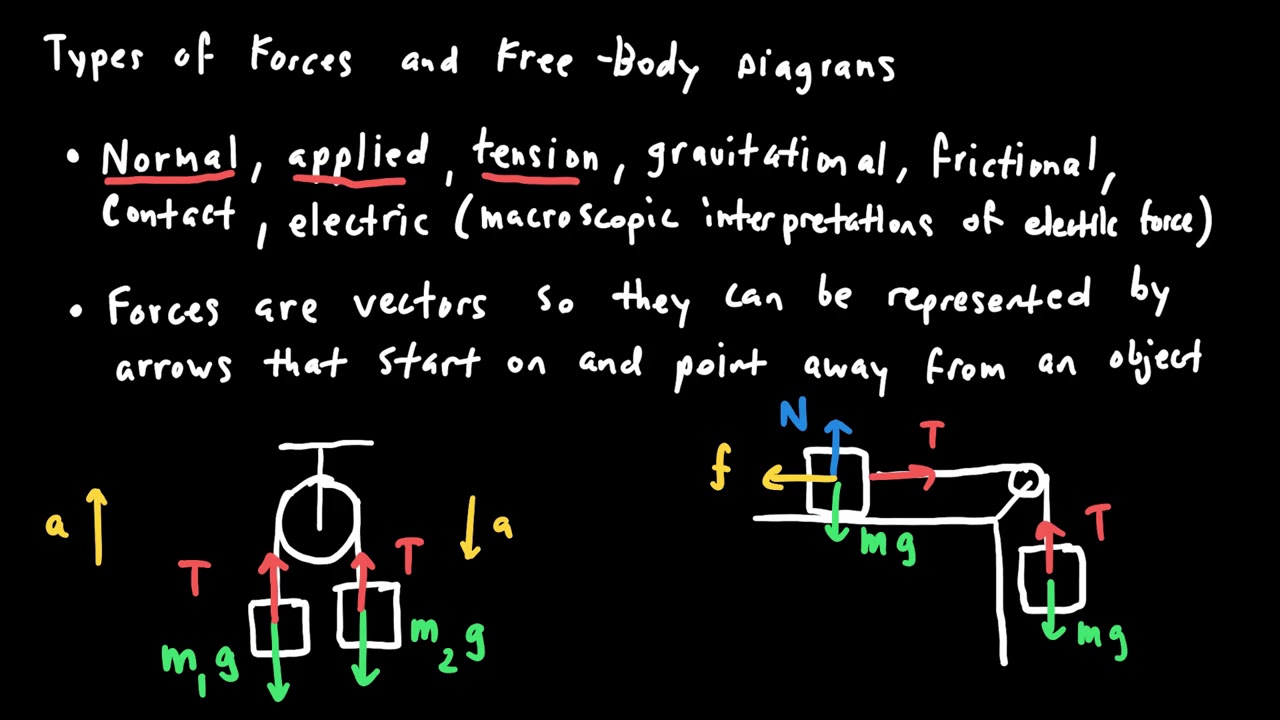
AP Physics 1 - Elevators
TLDRIn this educational video, Dan Fullerton explores the dynamics of elevators to demonstrate Newton's second law of motion. He explains how scales measure normal force rather than mass, and uses Freebody diagrams to analyze the forces acting on a person in an elevator. Through various scenarios, such as constant velocity motion and acceleration, Fullerton shows how the scale readings change and provides equations to calculate these readings. The video is an engaging way to understand the application of physics in everyday situations.
Takeaways
- 📈 Understanding elevators can help practice Newton's second law of motion effectively.
- 🔢 Scales measure the normal force exerted by the scale on an object, not the object's true mass or weight.
- 🚀 When in an elevator at rest or moving at a constant velocity, the scale reads the person's weight (mg).
- 📉 During upward acceleration in an elevator, the scale reads a value higher than the person's weight due to increased apparent weight.
- 📴 Conversely, during downward acceleration, the scale reads a value lower than the person's weight because of reduced apparent weight.
- 🔄 When an elevator is accelerating upwards, the scale reading equals the person's mass times the sum of gravitational acceleration and the elevator's acceleration.
- 🔢 For an object moving at a constant velocity (whether upwards or downwards), the scale reading equals the object's weight (mg).
- 🐶 In the case of an object with a mass of 25 kg in an accelerating elevator, the scale reading changes according to the equation normal force = mass * (G + a).
- 🐥 Daffy Duck's weight in the elevator, when accelerating downwards at 3 m/s², would be calculated using the equation normal force = mg - ma.
- 🔍 To solve for the scale reading during acceleration, one must consider the direction and magnitude of the elevator's acceleration relative to gravity.
Q & A
What is the main objective of the lesson discussed in the transcript?
-The main objective of the lesson is to utilize Freebody diagrams to identify the forces exerted on an object and solve Newton's second law equations corresponding to given Freebody diagrams or pseudo Freebody diagrams. Additionally, it aims to predict the motion of an object due to multiple forces by applying Newton's second law of motion using elevators as a practical example.
How does the scale measure weight, and what does it actually measure?
-The scale measures weight by reading the normal force exerted by the scale back up on the person or object. It doesn't directly measure mass or weight; instead, it measures the force with which it pushes back against the person or object, which correlates to the weight due to gravity.
What happens to the scale reading when the elevator accelerates upwards?
-When the elevator accelerates upwards, the scale reading initially remains the same as when the elevator is at rest because the normal force equals the weight of the person (mg). However, if the elevator continues to accelerate upwards, the scale reading may decrease due to the reduced apparent weight caused by the upward acceleration.
Why does the scale reading change when the elevator decelerates while moving downwards?
-When the elevator decelerates while moving downwards, the scale reading increases momentarily because the normal force exerted by the scale becomes greater than the weight of the person (mg). This is due to the deceleration causing an increased sensation of weight, which is the result of the upward acceleration during deceleration.
How can we calculate the scale reading when the elevator is accelerating downwards according to the given example?
-To calculate the scale reading when the elevator is accelerating downwards, we can use Newton's second law (F_net = m*a) and the Freebody diagram. The net force (F_net) is the difference between the gravitational force (mg) and the normal force (N). By setting up the equation as N = mg - ma and solving for N, we find that the scale reading will be the person's mass times the gravitational acceleration minus the acceleration of the elevator.
What is the scale reading when Lizzy is riding the elevator upward at a constant speed?
-When Lizzy is riding the elevator upward at a constant speed, the scale reading is the same as when she is at rest because there is no acceleration (a = 0). In this case, the normal force equals the gravitational force (mg), so the scale simply reads Lizzy's weight.
How does the scale reading change when Dave's dog, with a mass of 25 kilograms, is in an elevator accelerating upward?
-When Dave's dog is in an elevator accelerating upward, the scale reading increases due to the additional force required to accelerate the dog along with the elevator. The normal force (N) is calculated as N = mg + ma, where m is the mass of the dog (25 kg), g is the acceleration due to gravity (9.8 m/s^2), and a is the upward acceleration of the elevator (3 m/s^2). The scale reading would be approximately 320 Newtons in this case.
What will the scale read for Daffy Duck when the elevator accelerates downward at 3 meters per second squared?
-When the elevator accelerates downward at 3 m/s^2, the scale reading for Daffy Duck will be less than his normal weight of 230 Newtons. The normal force (N) is calculated as N = mg - ma, where m is Daffy Duck's mass (which can be calculated as 230 Newtons divided by 9.8 m/s^2), g is the acceleration due to gravity (9.8 m/s^2), and a is the downward acceleration of the elevator (3 m/s^2). The scale reading will be approximately 160 Newtons.
How does the concept of apparent weight relate to the scale readings in an elevator?
-Apparent weight is the force exerted on a person or object as perceived by them, which can change depending on the acceleration of the elevator. When an elevator accelerates upwards, the apparent weight decreases because the scale reading shows a lower value than the actual weight due to reduced normal force. Conversely, when the elevator accelerates downwards, the apparent weight increases, and the scale reading shows a higher value than the actual weight.
What is the significance of using elevators to study Newton's second law?
-Elevators provide a controlled environment to study Newton's second law because they allow for varying states of motion, such as constant velocity, acceleration, and deceleration. These changes in motion affect the normal force exerted by the scale on the person or object, which can be measured and used to understand and apply Newton's second law, offering a practical and illustrative example of the concepts discussed.
How can we determine the mass of an object if we know its weight and the acceleration due to gravity?
-The mass of an object can be determined by dividing its weight (the force of gravity acting on it) by the acceleration due to gravity (g). The formula to calculate mass (m) is m = W / g, where W is the weight in Newtons and g is the acceleration due to gravity (approximately 9.8 m/s^2).
Outlines
📚 Introduction to Elevators and Newton's Second Law
This paragraph introduces the topic of elevators as a practical application for studying Newton's second law of motion. The speaker, Dan Fullerton, explains that the lesson's objectives are to use Freebody diagrams to identify forces on an object and solve related Newton's second law equations. It also touches on the concept that scales measure normal force, not actual mass or weight, and sets the stage for exploring how an elevator's motion affects the scale reading.
🚀 Elevator Motion and Scale Readings
In this paragraph, the discussion focuses on how the motion of an elevator affects the readings on a scale. The speaker conducts experiments in an elevator to demonstrate how scale readings change when the elevator is moving upwards, downwards, accelerating, or decelerating. The explanation includes the use of Freebody diagrams and Newton's second law to predict the motion of an object in response to multiple forces. The paragraph also presents a series of sample problems to illustrate how the scale readings vary with different types of motion, such as constant velocity and acceleration.
🧐 Analyzing Elevator Acceleration Effects on Scale Readings
The final paragraph delves deeper into the analysis of how elevator acceleration impacts the scale readings. It uses several hypothetical scenarios involving different subjects (a man, Lizzy, a dog, and Daffy Duck) and varying conditions of elevator motion (accelerating upwards, downwards, and at rest). The speaker applies Newton's second law and Freebody diagrams to calculate the expected normal force and scale readings in each situation. The explanations are detailed, showing the mathematical relationships between the object's mass, gravitational force, and the elevator's acceleration. The paragraph concludes with a summary of the insights gained from the analysis and encourages further exploration of dynamics and Newton's laws.
Mindmap
Keywords
💡Freebody diagrams
💡Newton's second law
💡Elevator
💡Acceleration
💡Normal force
💡Mass
💡Weight
💡Scale
💡Constant velocity
💡Gravitational field
💡Forces
Highlights
Today's lesson focuses on elevators and utilizing Freebody diagrams to identify forces exerted on an object.
The objective is to solve Newton's second law equations corresponding to given Freebody diagrams and predict the motion of an object due to multiple forces.
Scales measure the normal force exerted by the scale back up on you, not your actual mass or weight.
When standing on a scale in an elevator, the reading can change due to the elevator's motion, providing a way to practice Newton's second law.
In the experiment, Mr. Fullerton's mass is approximately 200 pounds or 93 kilograms as measured by the scale.
When the elevator travels upwards at constant velocity, the scale reading remains consistent with the person's weight.
As the elevator slows down to stop, the scale reading momentarily increases, indicating a change in the normal force.
The scale reading is affected by the elevator's acceleration; it decreases when the elevator accelerates downwards and increases when it accelerates upwards.
A sample problem demonstrates that when a person in an elevator accelerates downwards, the scale reads the person's mass times gravity minus the elevator's acceleration.
When Lizzy stands on a scale in an elevator moving upward at a constant speed, the scale reads the same as when the elevator is at rest because there is no acceleration.
For Dave, a dog with a mass of 25 kilograms, the scale reads its weight whether the elevator is moving at a constant speed or at rest.
When the elevator accelerates upward, the scale reads the dog's mass times gravity plus the acceleration of the elevator.
Upon the elevator coming to a complete stop, the scale reads the dog's weight, which is the mass times gravity.
Daffy Duck's weight is 230 Newtons, and the scale reading changes when the elevator accelerates downward due to the difference in acceleration forces.
During the downward acceleration of the elevator, the scale reads approximately 160 Newtons for Daffy Duck, accounting for the acceleration.
These examples illustrate the relationship between an object's weight, the normal force, and the acceleration of the elevator.
The lesson provides a practical application of Newton's second law of motion using elevator scenarios to understand the dynamics of forces at play.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: