AP Physics 1: Algebra-based - Unit 2 Review - Dynamics
TLDRThe video script delves into the fundamentals of dynamics, focusing on Newton's first law of motion, the concept of equilibrium, and the creation of Freebody diagrams to analyze forces. It explains the difference between static and kinetic friction, the role of normal force, and how to apply Newton's laws to solve for acceleration in various scenarios, including the use of machines with and without mass. The script concludes with a challenge to verify the solutions with specific values, reinforcing the understanding of dynamics principles.
Takeaways
- 📐 Newton's First Law (Law of Inertia) states that an object will maintain its state of constant velocity or rest unless acted upon by an unbalanced force.
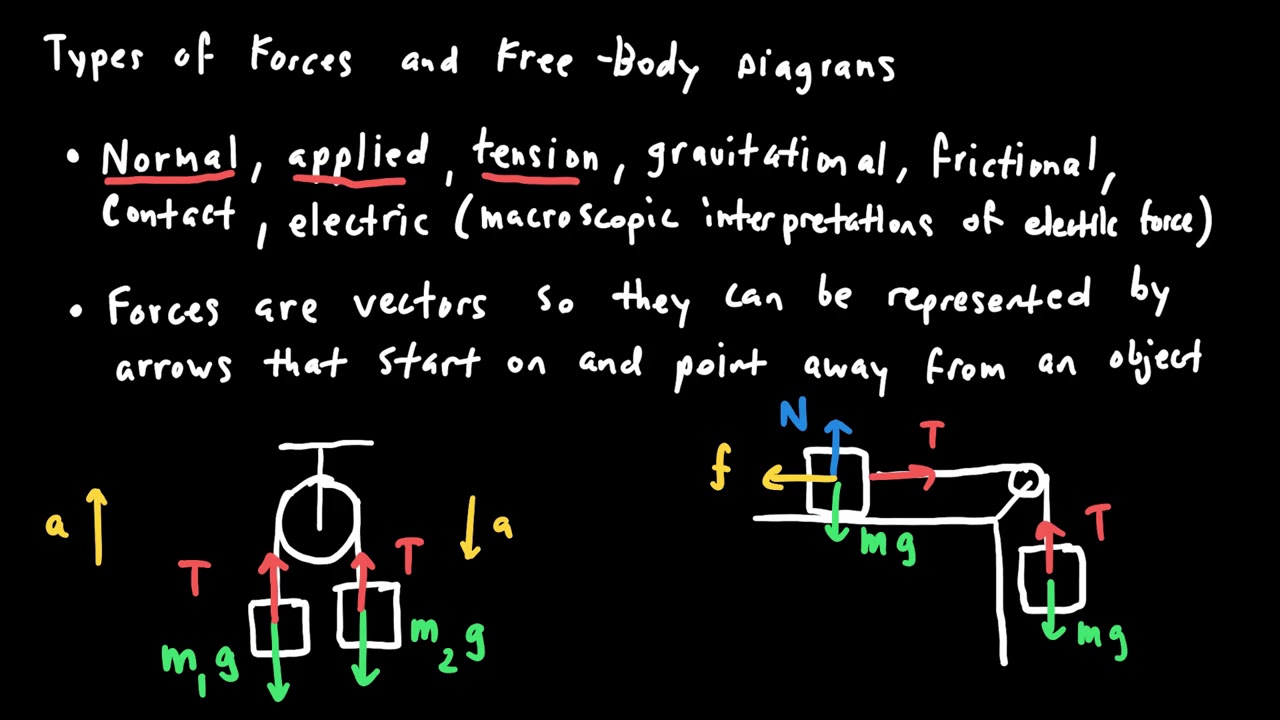
- 🚀 Freebody diagrams illustrate the forces acting on an object and are used to determine acceleration, not the direction of motion.
- ⚖️ When forces are in equilibrium, there is no acceleration, but the object can still be moving at a constant velocity.
- 📐📊 In a Freebody diagram, only draw forces that exist for the scenario and avoid adding irrelevant or nonexistent forces.
- 🔄 Never draw vector components on a Freebody diagram; instead, represent each force with a single vector in its applied direction.
- 📌 The normal force is perpendicular to the surface of contact, and its direction depends on the orientation of the surface.
- 🚫 Frictional force acts parallel to the surface of contact and opposes the motion (or potential motion) of an object.
- 🔄 Static friction adjusts to maintain equilibrium up to a maximum value, beyond which the object transitions to kinetic friction, which is generally less.
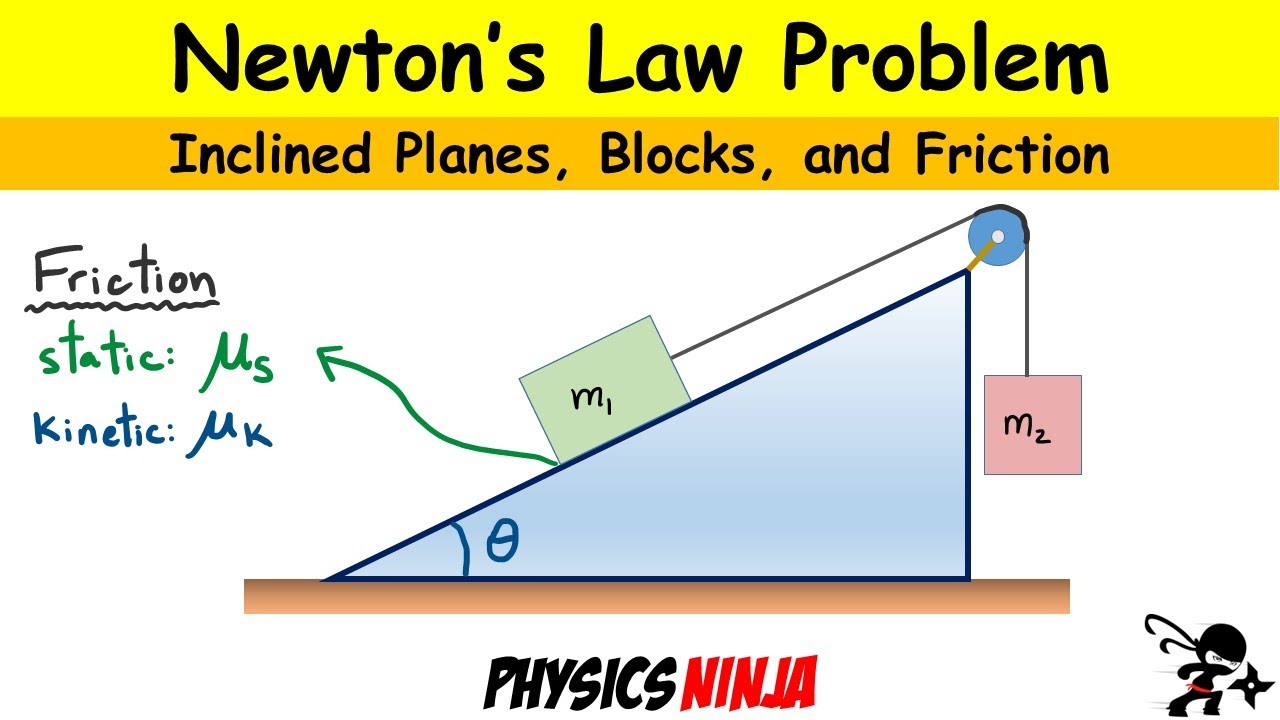
- 🔧 Machines with connected weights (like blocks on a rope) require analyzing both contact and tension forces to determine the system's behavior.
- 📐 When dealing with inclined planes, use trigonometry to resolve forces into components parallel and perpendicular to the incline.
- 🔄 For systems involving pulleys, consider the rotational inertia of the pulley and the fact that the net force must account for both translational motion and rotation.
Q & A
What is Newton's first law of motion?
-Newton's first law, also known as the law of inertia, states that an object will not accelerate unless acted upon by a net external force. This means that an object will maintain its state of constant velocity or remain at rest if the forces acting upon it are balanced.
How do you interpret a Freebody diagram?
-A Freebody diagram is a graphical representation that shows all the external forces acting on an object. It helps to visualize and calculate the net force or acceleration on the object. In the diagram, forces are represented by vectors, and their direction, magnitude, and point of application are indicated. It's important to only draw forces that actually exist and to not add irrelevant or nonexistent forces.
What is the difference between static and kinetic friction?
-Static friction is the frictional force that acts on an object at rest, preventing it from starting to move. It adjusts to match the applied force up to a maximum value, which is determined by the coefficient of static friction and the normal force. Kinetic friction, on the other hand, is the frictional force experienced by an object in motion. It is generally less than static friction and is calculated using the coefficient of kinetic friction and the normal force.
How do you calculate the maximum static friction?
-The maximum static friction can be calculated using the equation: Maximum Static Friction = Coefficient of Static Friction * Normal Force. Once the applied force exceeds this value, the object will begin to move, and kinetic friction comes into play.
What are the main forces acting on an object in a pulley system?
-In a pulley system, the main forces acting on an object include the weight of the object (gravitational force), the normal force from the surface (if applicable), and the tension in the rope. If the pulley has mass and is rotating, additional considerations include rotational inertia and torque.
How does the presence of a pulley with mass affect the acceleration of the system?
-A pulley with mass not only has to accelerate the blocks but also has to overcome its own rotational inertia. This additional mass requires a larger net force to achieve the same acceleration as a system without the pulley, resulting in a decrease in the overall acceleration of the system.
What is the relationship between rotational inertia and the pulley's mass and radius?
-The rotational inertia of a pulley, which is a measure of its resistance to rotational motion, is given by the formula I = 1/2 * M * R^2, where M is the mass of the pulley and R is the radius.
How do you calculate the net torque acting on a rotating object?
-The net torque (τ) acting on a rotating object is calculated using the formula τ = ΣF * r, where F is the force applied and r is the radius (distance from the axis of rotation). The torque is the product of the force and the lever arm (distance from the pivot point).
What is the rotational analog of Newton's second law?
-The rotational analog of Newton's second law is given by the equation τ = I * α, where τ is the net torque, I is the rotational inertia, and α is the angular acceleration.
How can you solve for acceleration in a system with a massless pulley?
-In a system with a massless pulley, you can assume that the tension in both ropes is the same because the pulley does not add any mass or resistance to the system. By applying Newton's second law (ΣF = Ma) to each block and using the assumptions of equal tension and equal acceleration, you can solve for the acceleration.
What happens to the tensions in the ropes when a pulley has mass and is rotating?
-When a pulley has mass and is rotating, the tensions in the ropes are no longer equal. One side of the pulley experiences a greater tension because it has to provide enough force not only to move the blocks but also to rotate the pulley itself. This results in a difference in tensions, which can be calculated using the formula: ΔT = 1/2 * M_pulley * a, where M_pulley is the mass of the pulley and a is the acceleration.
Outlines
📚 Introduction to Dynamics and Newton's Laws
The paragraph begins with an overview of dynamics and proceeds to discuss Newton's first law, known as the law of inertia. It explains that when two forces are in equilibrium, there is no acceleration, which means an object could either be at rest or moving at a constant velocity. The concept of Freebody diagrams is introduced, emphasizing their role in illustrating acceleration rather than the direction of motion. The importance of correctly representing forces and the distinction between static and kinetic friction are also covered, with a focus on the conditions under which each type of friction applies.
🔍 Analysis of Freebody Diagrams and Friction
This section delves deeper into the analysis of Freebody diagrams, highlighting the rules for drawing them and the significance of not including irrelevant forces. It discusses the role of normal and frictional forces, explaining their directions and the conditions for their existence. The difference between static and kinetic friction is clarified through a graphical representation, with an emphasis on the role of the coefficient of friction in determining these forces. The paragraph also presents the fundamental equations for calculating frictional forces and discusses their implications for the motion of objects.
🤔 Problem Solving with Machines and Ropes
The paragraph focuses on problem-solving techniques involving machines, specifically when two weights are connected by a rope under the influence of gravity. It outlines the process of drawing Freebody diagrams for such scenarios, emphasizing the importance of identifying all real forces acting on the objects. The concept of tensional forces along the rope is introduced, and the method for determining the acceleration of the blocks is discussed. The paragraph also introduces the idea of making assumptions to simplify the problem and the use of substitution to solve for unknowns. Additionally, it presents an alternative method of defining a system, which allows for a more straightforward calculation of the overall acceleration.
🔧 Incorporating Pulley Systems and Rotational Inertia
This part of the script explores the impact of pulley systems on the dynamics of connected weights. It introduces the concept of rotational inertia and explains how the mass of a pulley affects the system's acceleration. The paragraph describes how to calculate torque and the changes in tension within the system due to the pulley's mass. The process of solving for acceleration when a massless pulley is replaced with a massive one is detailed, highlighting the decrease in acceleration due to the additional rotational inertia. The paragraph concludes with a challenge for the viewer to verify the results by plugging in specific values and comparing the outcomes with the previous scenarios.
Mindmap
Keywords
💡Law of Inertia
💡Freebody Diagram
💡Normal Force
💡Frictional Force
💡Static vs Kinetic Friction
💡Tension
💡Rotational Inertia
💡Net Torque
💡Newton's Second Law
💡Trigonometry in Forces
💡System Definition
Highlights
Review of Newton's first law, the law of inertia, emphasizing that equilibrium does not necessarily mean rest but could also be constant velocity.
Explanation of how Freebody diagrams work, including the importance of drawing only real forces and the direction of vectors.
Clarification on the concept of normal force, which is perpendicular to the surface of contact and not necessarily upward.
Discussion on frictional force, its relationship with the normal force, and its role in maintaining rest or slowing motion.
Explanation of static versus kinetic friction, including the graph that illustrates their differences and the equations for each.
Introduction to the concept of machines, specifically systems with two weights connected by a rope and the forces acting on them.
Procedure for drawing Freebody diagrams for complex systems, such as blocks on an inclined plane.
Use of Newton's second law to determine the acceleration of block two in the system, highlighting the importance of identifying unbalanced forces.
Application of Newton's first law in equilibrium situations, particularly for forces perpendicular to the incline.
Explanation of how to perform trigonometry to find vector components in inclined axes, using sine and cosine functions.
Method of solving for multiple unknowns using substitution, demonstrated through the calculation of tension and acceleration.
Alternative approach using the method of defining a system, considering multiple objects as one for simplicity.
Calculation of the pulley's rotational inertia and its impact on the system, including the change in tensions due to the pulley's mass.
Use of the rotational analog of Newton's second law, net torque equals I alpha, for problems involving rotation.
Solution for acceleration when a massless pulley is replaced with one that has mass, showing the decrease in acceleration.
Challenge to verify the solution by plugging in values for sine and mass of the pulley, confirming the accuracy of the derived formulas.
Summary of the dynamics review, emphasizing the understanding of forces, friction, and the application of Newton's laws in various scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: