AP Physics 1 Dynamics Review
TLDRThis video script offers a comprehensive review of dynamics in AP Physics 1, focusing on the study of forces that cause objects to move. It explains the relationship between force, mass, and acceleration as described by Newton's second law of motion. The importance of understanding net force, unbalanced forces, and the vector nature of force and acceleration is emphasized. The script also delves into various types of forces, such as gravitational, frictional, and electric, and their macroscopic interpretations. The concept of Freebody diagrams is introduced to visually represent forces acting on an object, and the differences between static and kinetic friction are clarified. The video concludes with a discussion on Newton's third law and Hooke's law, highlighting the application of kinematic equations in conjunction with dynamics problems.
Takeaways
- 📚 Dynamics is the study of the causes of motion, focusing on the relationship between force, mass, and acceleration as described by Newton's second law of motion.
- 🚀 To accelerate an object (change its speed), a force must be applied. This is quantified by Newton's second law, F = ma, where F is the net force, m is mass, and a is acceleration.
- 🔽 The direction of the force is crucial as force is a vector quantity. This means it has both magnitude and direction, which is represented in diagrams with arrows.
- ⚖️ When multiple forces act on an object, only unbalanced forces (net force not zero) result in acceleration. Balanced forces (net force zero) mean the object remains at rest or continues moving at a constant velocity, in accordance with Newton's first law.
- 🤝 There are various types of forces such as gravitational, frictional, normal, and tension forces. These are macroscopic interpretations of the electric force at the atomic level.
- 📐 Freebody diagrams are used to represent all forces acting on an object. Forces are drawn as arrows with appropriate labels, and acceleration vectors are not included in these diagrams.
- 🔄 Newton's third law states that every action has an equal and opposite reaction. This law helps explain why objects can move despite the presence of these reaction forces.
- 📉 The graph of static frictional force versus applied force shows an increase until the maximum static friction is reached, after which the object enters the region of kinetic friction where the force remains constant.
- 🔧 Hooke's law relates the force exerted by a spring to its extension or compression and the spring constant. The force is proportional to the displacement from the equilibrium position.
- 🔄 Kinematic and dynamic equations are often used together to solve physics problems. They both involve acceleration and can be used to calculate various aspects of motion.
- 🛠 Newton's second law can also be expressed in terms of changes in velocity over time (F = mΔv/Δt), highlighting that acceleration is the rate of change of velocity.
Q & A
What is dynamics in the context of AP Physics 1?
-Dynamics is the study of what causes objects to move, focusing on the relationship between the forces applied to an object, its mass, and the resulting acceleration.
How is Newton's second law of motion expressed mathematically?
-Newton's second law of motion is expressed as F = ma, where F represents the net force applied to an object, m is the mass of the object, and a is its acceleration.
What is the significance of force being a vector?
-Since force is a vector, it has both magnitude and direction. This means that the effect of a force depends not only on its strength but also on the direction in which it is applied.
What happens when multiple forces act on an object?
-When multiple forces act on an object, the net force is the resultant of all the individual forces. It is the unbalanced net force that causes the object to accelerate.
What is the difference between static and kinetic friction?
-Static friction is the force that prevents an object from starting to move, while kinetic friction is the force that opposes the motion of an object that is already sliding. The maximum static friction is usually greater than the kinetic friction.
How are forces represented in a Freebody diagram?
-In a Freebody diagram, forces are represented by arrows that indicate both the direction and magnitude of the force. The length of the arrow can help understand the relative size of the forces.
What is Hooke's law and how is it related to force?
-Hooke's law states that the force exerted by a spring is proportional to the displacement from its equilibrium position, which can be expressed as F = -kx, where k is the spring constant and x is the displacement.
How can Newton's second law be used to solve for velocity changes?
-Newton's second law can be rewritten as F = ma = m(Δv/Δt), which relates force to the change in velocity (Δv) over a time interval (Δt), allowing us to solve for velocity changes when the force and time are known.
What is the role of unbalanced forces in causing an object to accelerate?
-Unbalanced forces are necessary for an object to accelerate. If the forces acting on an object are balanced, the object will not accelerate but will either remain at rest or continue moving at a constant velocity.
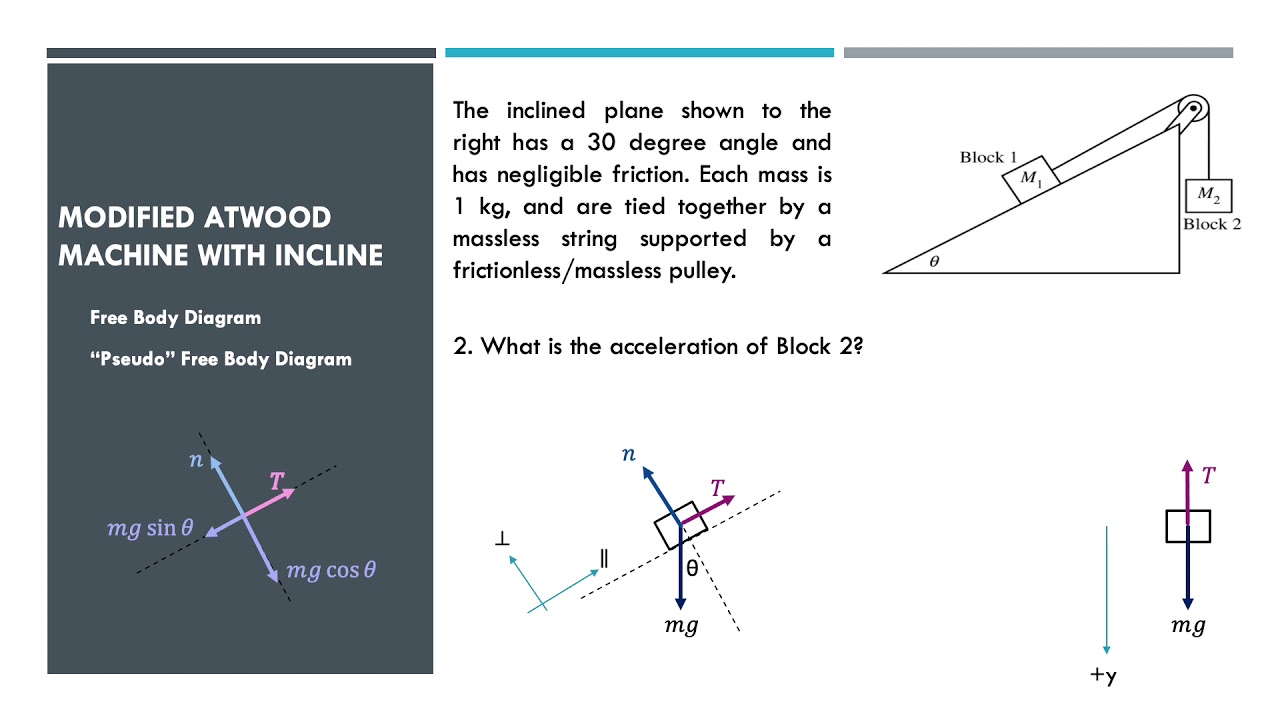
How does the weight vector break down on an inclined plane?
-The weight vector on an inclined plane breaks down into two components: one perpendicular to the plane (mg cos θ) and one parallel to the plane (mg sin θ), where θ is the angle of inclination.
What is the significance of Newton's third law in understanding motion?
-Newton's third law states that for every action, there is an equal and opposite reaction. This law helps explain why objects move; despite the presence of equal and opposite forces, the net force (unbalanced force) on an object determines its motion.
Outlines
🌟 Dynamics and Newton's Laws
This paragraph introduces the study of dynamics within AP Physics 1, focusing on the causes of object movement. It explains the necessity of force to accelerate an object and the relationship between force, mass, and acceleration as described by Newton's second law of motion. The vector nature of force and acceleration is emphasized, with the equation F=ma being highlighted. The importance of unbalanced forces for creating acceleration is discussed, as well as the concept of balanced forces and their relation to an object's state of motion. Various types of forces, such as gravitational, frictional, and electric forces, are introduced, with a particular note on how macroscopic forces can be interpreted as electric forces at a microscopic level.
📐 Freebody Diagrams and Force Representation
This section delves into the concept and application of Freebody diagrams in analyzing forces on a large scale with examples like blocks and pulleys. It explains how forces are represented as vectors through arrows in diagrams, indicating both direction and magnitude. The paragraph uses the example of an Atwood machine to illustrate the representation of gravitational force and the importance of labeling different masses. It also touches on the necessity of identifying and representing all forces correctly, including normal, tension, and frictional forces, and how they contribute to the overall dynamics of a system.
🔄 Component Analysis of Forces
This paragraph discusses the need to break down force vectors into components, specifically using the example of an object on an inclined plane. It explains how forces like the normal force, frictional force, and gravitational force act on the object and how these forces must be resolved into components along the X and Y directions to write accurate Newton's second law equations. The concept of positive and negative directions in a coordinate system is introduced, and the method of breaking down the gravitational force vector (mg) into its components using trigonometric relationships is explained.
📚 Newton's Second Law Application
The application of Newton's second law is detailed in this paragraph, with a focus on how to write the law as equations for both the X and Y directions. It emphasizes the need to define the coordinate system and align it with the physical situation, such as an inclined plane. The paragraph provides a step-by-step explanation of how to write the force equations, including the components of gravitational force, and how to solve for the acceleration of an object based on the net force in the X direction. It also addresses the special case of an object at rest on an inclined plane, where the net force in the Y direction is zero due to the absence of acceleration in that direction.
🚫 Static and Kinetic Friction
This section provides an in-depth look at the two types of friction: static and kinetic. It explains static friction as the force that prevents an object from moving up to a certain maximum value, represented by the coefficient of static friction (μs). The concept of kinetic friction is introduced as a constant value once the object starts moving, characterized by the coefficient of kinetic friction (μk). A graphical representation is used to illustrate the relationship between the applied force and the frictional force, highlighting the transition from static to kinetic friction. The paragraph also discusses the equation for friction (f=μN), where N is the normal force, and emphasizes the need to understand the difference between static and kinetic friction for problem-solving.
🔄 Further Dynamics Concepts and Hooke's Law
The paragraph continues the discussion on dynamics by introducing Newton's third law, which states that every action has an equal and opposite reaction. It explores the implications of this law on the movement of objects and how forces can act on different objects without necessarily causing movement. The concept of Hooke's law is introduced, explaining the relationship between the force exerted by a spring and the distance it is stretched, characterized by the spring constant (k). The paragraph also highlights the importance of combining kinematic equations with dynamics problems, such as using Newton's second law (F=ma) alongside kinematic equations to solve for acceleration and other variables. Lastly, it presents an alternative form of Newton's second law, relating force to the change in velocity over time (F=MΔV/ΔT), offering another perspective for solving dynamics problems.
📝 Summary of Dynamics Principles
In conclusion, this paragraph summarizes the key principles of dynamics covered in the video script. It reiterates the importance of understanding the difference between static and kinetic friction, the calculation of frictional forces using coefficients and normal force, and the significance of Newton's third law in the context of forces and motion. The paragraph also underscores the utility of Freebody diagrams in visualizing and analyzing forces, the necessity of resolving forces into components for accurate problem-solving, and the interplay between kinematic and dynamic equations in comprehensive physics problem analysis.
Mindmap
Keywords
💡Dynamics
💡Acceleration
💡Force
💡Mass
💡Newton's Second Law
💡Freebody Diagram
💡Friction
💡Inclined Plane
💡Tension Force
💡Static Friction
💡Kinetic Friction
Highlights
Dynamics is the study of what causes objects to move.
To get an object moving, its speed must be increased, which requires acceleration.
Acceleration occurs when a force is applied to an object.
Newton's second law of motion relates the forces applied to an object, its acceleration, and its mass.
The net force on an object affects its acceleration; if the net force increases, so does the acceleration.
For a given force, if the mass of an object increases, the acceleration decreases.
Forces are vectors, having both magnitude and direction, and are measured in Newtons.
An object will accelerate when unbalanced forces act upon it.
If the net force is zero, an object will not accelerate and will either remain at rest or continue moving at a constant speed.
Newton's first law of motion states that an object will maintain its state of rest or uniform motion unless acted upon by an external force.
There are various types of forces, including gravitational, frictional, normal, and applied forces.
Many macroscopic forces can be interpreted as electric forces at a microscopic level.
Freebody diagrams represent forces as arrows indicating direction and sometimes magnitude.
In an Atwood machine, tension forces in the string connecting two objects are the same everywhere.
When analyzing forces, it's important to define the positive and negative directions in a coordinate system.
Force equations must be constructed in a single direction, either X or Y, not both.
The weight vector of an object can be broken down into components for analysis on inclined planes.
Frictional forces can be static or kinetic, with static friction being the force that prevents an object from starting to move.
Kinetic friction is the constant force that acts on an object once it starts sliding.
Newton's third law states that for every action, there is an equal and opposite reaction.
Hooke's law relates the force exerted by a spring to its spring constant and the distance it is stretched.
Kinematic equations are often used alongside dynamics problems to solve for variables related to motion.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: