Determining Sample Size | Cochran & Yamane Formula | Finite & Infinite population | Examples

Solomon Getachew
16 Jul 202316:26
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the critical factors influencing sample size determination in statistical research, emphasizing the importance of the margin of error, confidence level, and degree of variability. It introduces the Cochran Formula for large populations and the Yamane Formula for finite populations, providing examples to illustrate their application. This comprehensive overview is essential for researchers aiming to achieve accurate and reliable results in their studies.

Takeaways
  • 📊 The importance of determining an appropriate sample size in research is emphasized, as it affects the reliability and generalizability of results.
  • 🔢 The three main factors influencing sample size are the margin of error, confidence level, and degree of variability.
  • 📈 Margin of error is the degree to which a sample estimate may differ from the true value in the population, and it directly impacts the sample size needed.
  • 💡 A higher confidence level (typically expressed as a percentage) results in a larger sample size, as it reflects the level of certainty that the results are close to the true value.
  • 🔄 Degree of variability refers to the heterogeneity within the population; greater variability requires a larger sample size to ensure accurate representation.
  • 🌐 Cochran Formula is mentioned as a method for calculating sample size in large populations, taking into account the population size and the other factors.
  • 📏 An example is provided to illustrate how the Cochran Formula is applied in practice, showing the calculation process and its outcomes.
  • 🔢 Yamane Formula is introduced for finite populations, offering an alternative approach to determining sample size when the population is known and limited.
  • 📌 The script highlights the balance between sample size and practicality, as very large sample sizes may be unnecessary and impractical.
  • 🔍 The importance of considering the research context and objectives when determining sample size is stressed, as different studies have varying requirements.
  • 📋 The process of sample size determination is presented as a critical step in research design, influencing the study's validity and the interpretation of findings.
Q & A

  • What is the primary purpose of determining sample size in a research study?

    -The primary purpose of determining sample size is to ensure that the results of the study are representative of the population and to minimize the impact of sampling error on the findings.

  • What are the key factors that affect the sample size in a study?

    -The key factors affecting sample size include the margin of error, confidence level, and degree of variability. These factors determine the precision and reliability of the study's outcomes.

  • What does the margin of error represent in the context of sample size determination?

    -The margin of error represents the range within which the true population value is likely to fall, given the sample results. A smaller margin of error requires a larger sample size to achieve the desired level of precision.

  • How does the confidence level influence the sample size?

    -A higher confidence level means that there is a greater probability that the true population value lies within the margin of error. To increase the confidence level, a larger sample size is needed.

  • What is the significance of the degree of variability in determining the sample size?

    -The degree of variability, or the amount of diversity in the data, affects the sample size. Greater variability requires a larger sample size to ensure that the sample is representative of the entire population.

  • What is the Cochran Formula, and in what situations is it used?

    -The Cochran Formula is used to determine the sample size for a population when dealing with a large, heterogeneous population. It takes into account the population size and the desired confidence level and margin of error.

  • Can you provide an example of how the Cochran Formula is applied?

    -Suppose a researcher wants to estimate the proportion of people in a city who support a particular policy with a 95% confidence level and a margin of error of 5%. The Cochran Formula would be used to calculate the appropriate sample size based on the total population of the city.

  • How does the Yamane Formula differ from the Cochran Formula?

    -The Yamane Formula is specifically designed for finite populations. It takes into account the actual size of the population being studied, whereas the Cochran Formula assumes a large, virtually infinite population for simplification purposes.

  • What is the main advantage of using the Yamane Formula over the Cochran Formula?

    -The main advantage of the Yamane Formula is that it provides a more accurate sample size calculation for finite populations. It accounts for the fact that the sample is taken from a specific, known population size, which can lead to a more precise estimate.

  • In what scenarios would a researcher opt for the Yamane Formula over the Cochran Formula?

    -A researcher would choose the Yamane Formula when working with a well-defined, finite population. This is because the Yamane Formula considers the actual population size, leading to a more accurate sample size calculation in such cases.

  • How does the concept of 'finite population' impact the sample size calculation?

    -In a finite population, the sample size calculation must take into account the actual number of individuals or units in the population. This can affect the sample size, as the formulae are adjusted to consider the finite nature of the population, leading to potentially different sample sizes than if the population were considered infinite.

  • What are the potential consequences of not accurately determining sample size in a research study?

    -Failing to accurately determine sample size can lead to results that are not representative of the population, increased sampling error, and reduced confidence in the study's findings. This can ultimately undermine the validity and reliability of the research.

Outlines
00:00
📊 Sample Size Determination Essentials

This paragraph delves into the critical factors that influence the determination of sample size in a study. It emphasizes the importance of the Margin of Error, Confidence Level, and Degree of Variability, which are essential in achieving accurate and reliable results. The Cochran Formula is introduced as a statistical tool for calculating sample sizes, particularly in large populations, ensuring that the sample is representative and the findings can be generalized to the larger population with confidence.

06:36
🌐 Large Population Sampling Techniques

The second paragraph focuses on the sampling techniques applicable to large populations. It provides an example and introduces the Yamane Formula, which is specifically designed for finite populations. This formula helps researchers estimate the required sample size to ensure that the sample is sufficiently representative of the population. The discussion on finite populations highlights the practical considerations in sampling and the mathematical approaches to achieving a balance between precision and feasibility in research studies.

Mindmap
Keywords
💡Sample Size Determination
Sample size determination is a critical statistical process used to calculate the number of observations or participants needed for a study to achieve reliable and valid results. It is essential in research to ensure that the conclusions drawn from the data are representative of the population being studied. In the context of the video, this concept is central to understanding how researchers decide on the appropriate sample size for their studies, which directly impacts the margin of error and confidence level of their findings.
💡Margin of Error
Margin of error is a statistical term that refers to the range within which the true value of a parameter (such as a population mean) is likely to fall, given a certain level of confidence. It is an essential consideration in sample size determination because a smaller margin of error typically requires a larger sample size to achieve. The video emphasizes the relationship between the margin of error and sample size, highlighting how researchers must balance these factors to ensure their study's precision and reliability.
💡Confidence Level
The confidence level is a statistical measure that quantifies the level of certainty that the true value of a parameter lies within a specified range. It is often expressed as a percentage (e.g., 95% confidence level) and is directly related to the margin of error. A higher confidence level means that researchers are more certain that their results accurately reflect the population's characteristics, but it also typically requires a larger sample size to achieve. The video underscores the importance of selecting an appropriate confidence level when determining the sample size for a study.
💡Degree of Variability
Degree of variability refers to the extent to which individual data points in a population differ from the mean or from each other. A higher degree of variability means that the data points are more spread out, which can make it more challenging to draw accurate conclusions from a sample. In the context of sample size determination, a greater degree of variability often requires a larger sample size to ensure that the sample is representative of the population and that the results are reliable. The video highlights the role of variability in determining the necessary sample size for a study.
💡Cochran Formula
The Cochran Formula, named after the statistician G.V. Cochran, is a widely used formula for calculating the sample size needed for a stratified or cluster sampling design. It takes into account the population size, the desired confidence level, and the margin of error, as well as the design effect, which accounts for the potential increase in variability due to the sampling method. The video's mention of the Cochran Formula suggests that it is a key method for determining sample size, especially in complex sampling designs where the population is divided into strata or clusters.
💡Large Population
In statistical terms, a large population refers to a group of individuals or observations that is so large that the probability of any single individual being selected in a sample is negligible. The concept of a large population is important in the context of sample size determination because it affects the formulas and methods used to calculate the required sample size. For very large populations, certain simplifications can be made in the calculations, such as ignoring the population size in the sample size formula. The video's mention of large populations indicates that the sample size determination process may vary depending on the size of the population being studied.
💡Yamane Formula
The Yamane Formula is a statistical formula used to determine the sample size for a finite population. Unlike the Cochran Formula, which is designed for large populations, the Yamane Formula takes into account the specific size of the population being studied. It adjusts the sample size calculation to account for the fact that the population is not infinitely large, which can affect the precision of the estimates. The video's reference to the Yamane Formula indicates that it is an important tool for researchers when working with populations of a known and limited size.
💡Finite Population
A finite population refers to a group of individuals or observations that is limited in size and can be counted. In the context of sample size determination, the concept of a finite population is important because it affects the precision of the estimates and the formulas used to calculate the required sample size. When the population size is known and finite, specific adjustments are made to the sample size calculations to ensure that the results are representative and accurate. The video's mention of finite populations indicates that the sample size determination process must consider whether the population is large and effectively infinite or small and countable.
💡Example
In the context of the video script, the term 'example' implies the use of specific cases or scenarios to illustrate the concepts being discussed. Examples are crucial in educational content as they help viewers understand abstract concepts by relating them to real-world situations or hypothetical scenarios. They can also demonstrate how the statistical formulas and methods discussed in the video are applied in practice. The use of examples in the video is likely intended to clarify the process of sample size determination and make the content more accessible to viewers.
Highlights

The importance of determining sample size in research studies is emphasized, highlighting the need for accurate and reliable data collection.

Margin of Error is identified as a critical factor affecting sample size, with smaller margins requiring larger sample sizes for increased precision.

The Confidence Level is discussed as a significant determinant of sample size, with higher confidence levels necessitating larger sample sizes for more reliable results.

Degree of Variability is introduced as an influential factor, where greater variability in the population leads to the need for a larger sample size to capture the range of differences.

Cochran Formula is introduced as a method for determining sample size in large populations, providing a mathematical approach to achieve representative sampling.

An example of applying the Cochran Formula is given, illustrating how to calculate the required sample size for a specific research study with a large population.

Yamane Formula is presented as a tool for finite populations, offering a different calculation method for when the population size is known and limited.

A practical example of the Yamane Formula is provided, demonstrating its application in determining the sample size for a research study with a finite population.

The balance between sample size and practicality is discussed, emphasizing the need to consider resources and feasibility in addition to statistical considerations.

The impact of non-sampling errors is considered, highlighting the importance of not only the sample size but also the methodology of data collection.

The use of statistical software in calculating sample sizes is mentioned, showcasing the role of technology in facilitating complex calculations.

The concept of stratified sampling is introduced, explaining how it can improve the representativeness of a sample by ensuring proportional representation of different subgroups.

The transcript discusses the ethical considerations in sampling, such as ensuring that the sample size is sufficient to avoid harm or bias to the participants.

The role of pilot studies in determining sample size is highlighted, as they can provide valuable insights and help refine the sampling strategy.

The transcript emphasizes the iterative nature of sample size determination, as researchers may need to adjust their calculations based on preliminary findings or changing conditions.

The importance of considering the sample size in the context of the research question is stressed, as the size should be aligned with the objectives and scope of the study.

The transcript concludes with a reminder of the need for a comprehensive understanding of statistical principles when determining sample sizes, to ensure the validity and reliability of research findings.

Transcripts

Browse More Related Video

7.1.0 Estimating a Population Proportion - Lesson Overview, Key Concepts and Learning Outcomes

Margin of Error & Sample Size for Confidence Interval | Statistics Tutorial #11| MarinStatsLectures

Elementary Stats Lesson #16

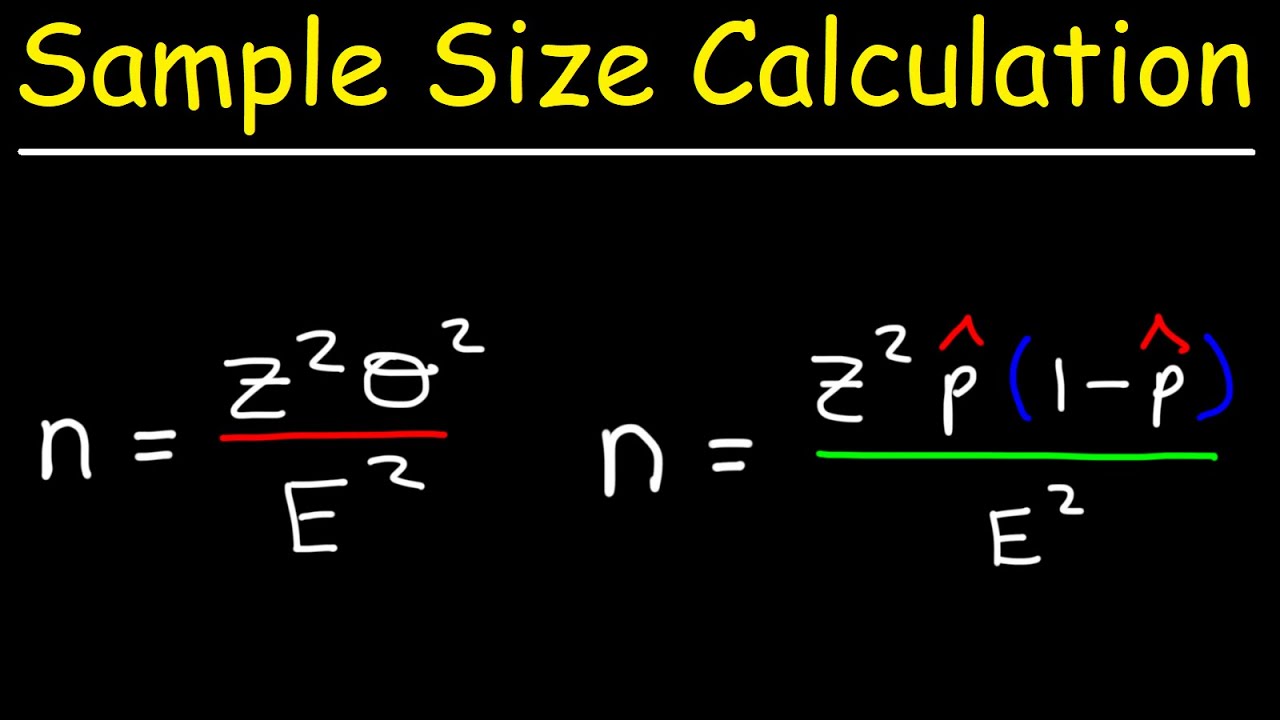
How To Calculate The Sample Size Given The Confidence Level & Margin of Error

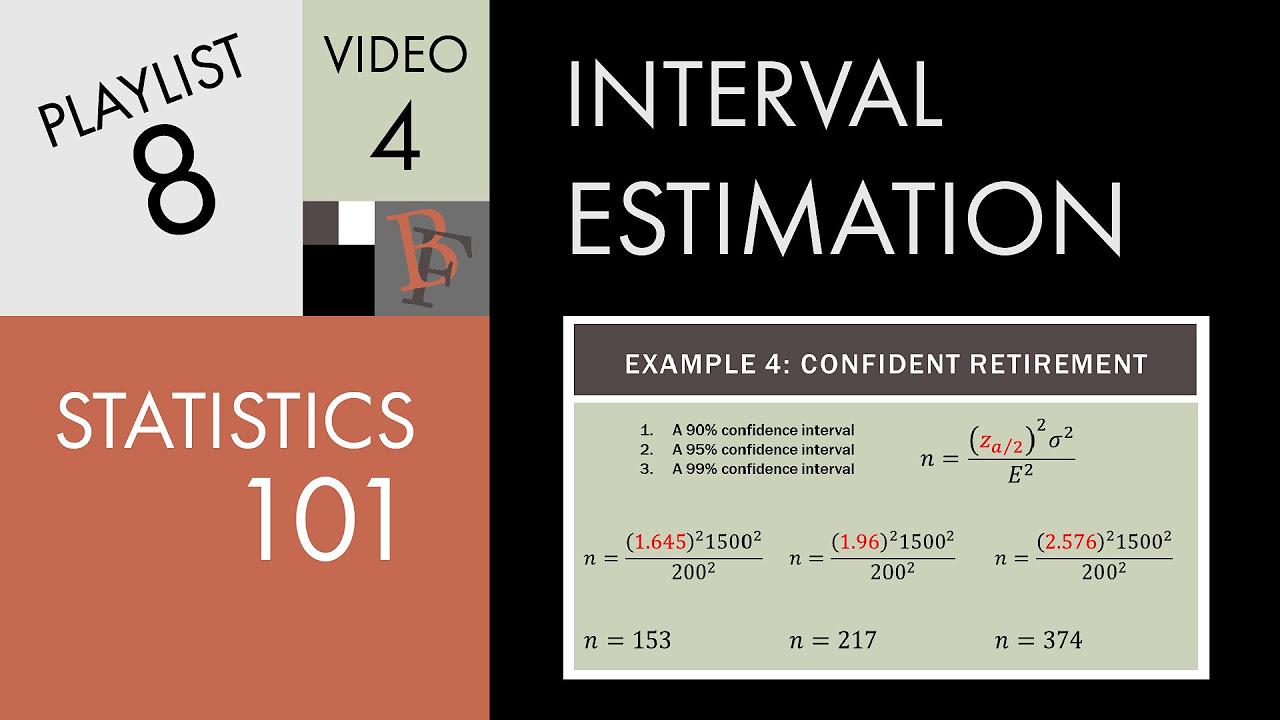
Statistics 101: Confidence Intervals, Estimating Sample Size Needed

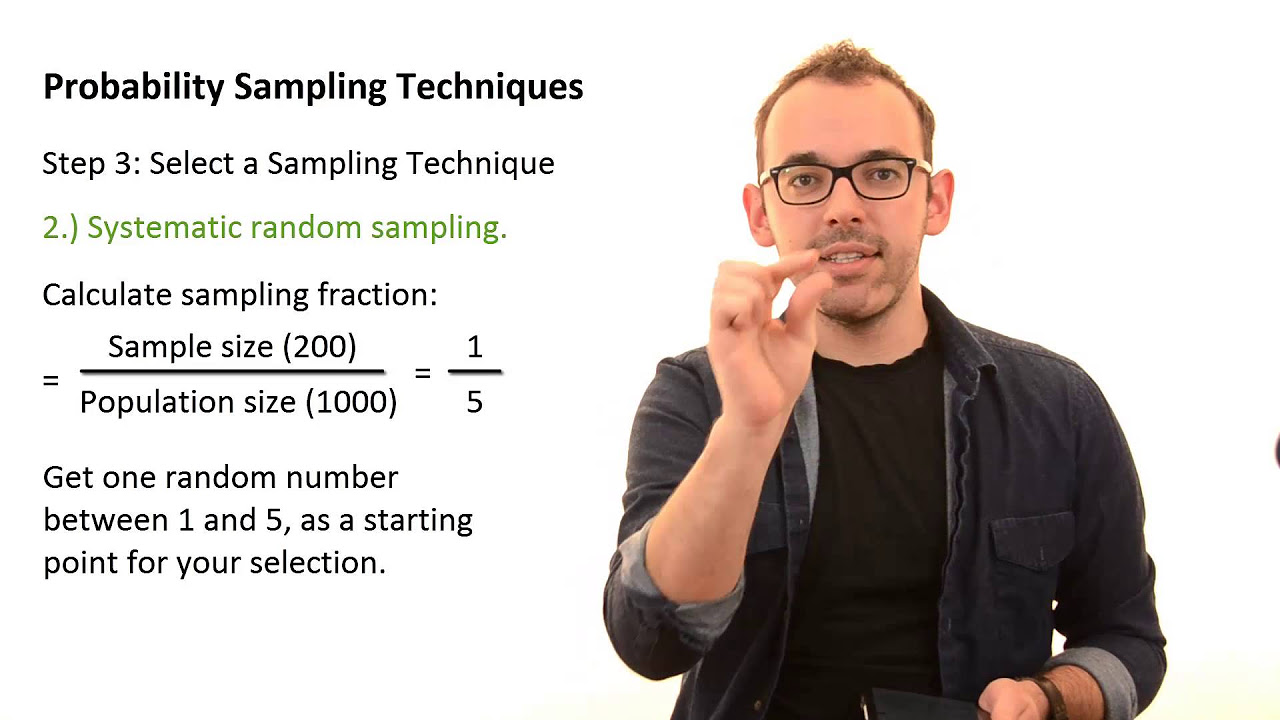
4.2 Probability Sampling Techniques

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
SampleSizeMarginOfErrorConfidenceLevelCochranFormulaLargePopulationYamaneFormulaFinitePopulationStatisticalAnalysisDataScienceResearchMethods