AP Physics Workbook 3.K Friction as the Centripetal Force

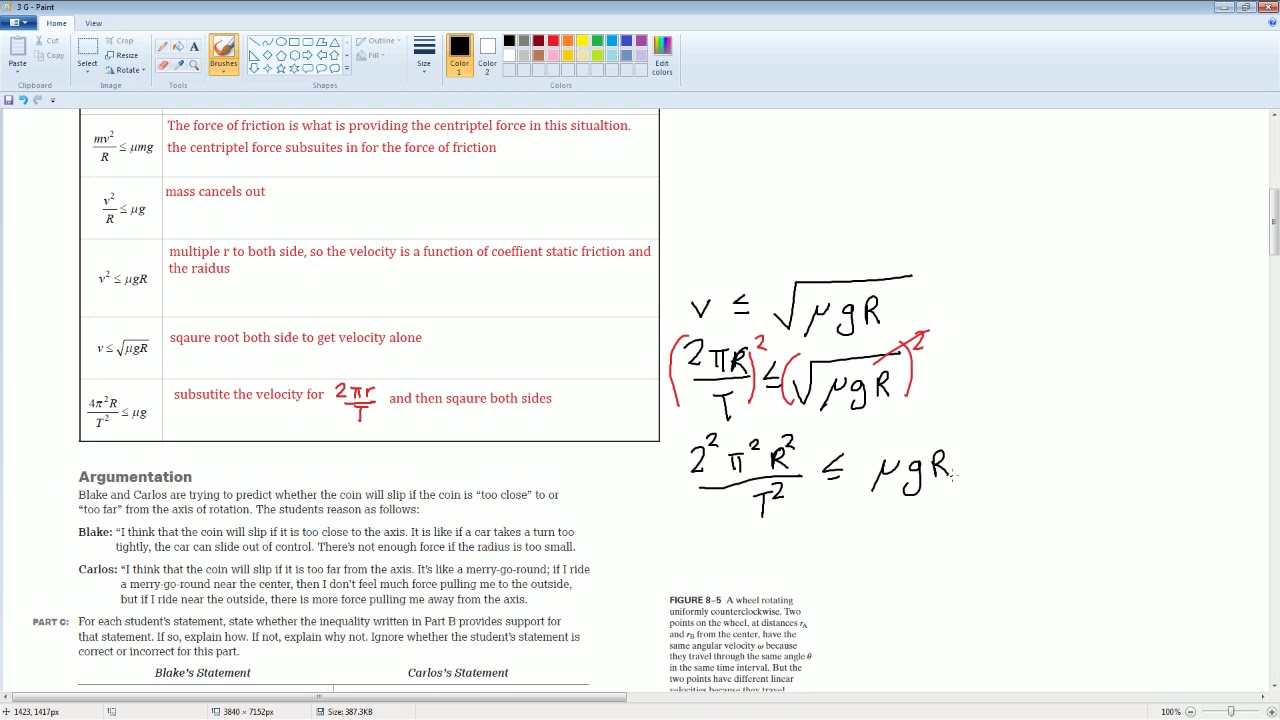
This paragraph introduces the AP Physics workbook focusing on Unit Three, which deals with circular motion and gravitation. The specific section, 3.K, is about friction as an internal force. The scenario involves a student who is trying to determine the coefficient of static friction (mus) between a coin and a steel plate. The student sets up the steel plate with the center attached to a freely rotating axis and places the coin at different positions on the plate. The student measures the distance (R) from the center to the coin and uses a stopwatch to measure the period (t) of the plate's rotation. The goal is to explain how the student can set up the experiment to take measurements that will allow the calculation of the coefficient of friction.

In this paragraph, the focus is on understanding the role of static friction as a centripetal force that keeps the coin in circular motion. The static friction force is directed radially towards the center of the circular path, calculated as MV^2/R, which is equal to the maximum static friction (mu times the normal force). The student is advised to start with a slow rotation and gradually increase the speed until the coin slips off the plate. The speed at which the coin slips off is crucial and must be recorded, as well as the rotational period. The period (T) is defined as the time for one complete cycle or revolution. To minimize experimental error, the student can conduct multiple trials or use a motion sensor to detect the moment the coin slips.

This paragraph delves into the derivation of the equation for the period (T) using Newton's second law of motion. The force of friction is what provides the necessary centripetal force. The equation is set up with the friction force (mu times the normal force, represented as mg) being equal to the mass times the velocity squared over R (MV^2/R). By canceling out the mass, the equation simplifies to ugR = V^2 / (2πR). The velocity (V) is then expressed as 2πR/T. Substituting this back into the equation and performing algebraic manipulations, the period squared (T^2) is related to the radius (R) and gravitational force (G), leading to the equation T^2 = (4π^2R^3)/(muG). The student is then guided to plot a graph of T^2 versus R^2, find the best line fit, and calculate the slope to determine the value of mu.

The paragraph explains the process of calculating the slope from the graph of T^2 versus R^2 and using it to find the coefficient of static friction (mu). The slope represents the ratio of the change in period squared (ΔT^2) to the change in radius (ΔR). The equation derived earlier is rearranged to solve for mu, resulting in muG = (4πR * T^2) / R^2. After calculating the slope using the given data points and performing the necessary algebraic steps, the value of mu is computed by multiplying the slope by the constant (4π^2R^3/G). The final value of mu is given as approximately 0.24, emphasizing the importance of rounding to a reasonable number of significant digits in physics.

The final paragraph corrects a mistake made in the previous calculation regarding the slope interpretation. It clarifies that the slope actually represents T^2 / R, not R / T^2 as previously stated. The correct equation for the slope is T^2 / R, which is found to be 16. The calculation is then revised accordingly, with the slope being the reciprocal of the corrected equation. The final value of the coefficient of static friction (mu) is recalculated using the correct formula and the previously determined slope, resulting in a value of approximately 0.24. The paragraph concludes by emphasizing the importance of understanding the correct relationships and formulas when solving physics problems.