AP Physics Workbook 2.O Spring Force and Acceleration

Mr.S ClassRoom
28 Sept 202016:49
EducationalLearning
32 Likes 10 Comments

TLDRThe video script outlines an experiment in which a student named Angelica uses a spring, meter stick, and stopwatch to measure the spring constant. The procedure involves hanging the spring vertically, adding known masses, and measuring the resulting spring stretch (delta x). The weight of each object is calculated, and the spring's force is equated to the gravitational force. The experiment aims to graph the weight versus spring stretch to determine the spring constant (k). The script also discusses how the measurements can be used to calculate maximum velocity (v max) and acceleration (a) during an elevator's upward movement and deceleration.

Takeaways
  • 📝 The experiment aims to determine the spring constant using a spring, masses, a meter stick, and a stopwatch.
  • 🔢 Measure the weight of each object by multiplying its mass with the acceleration due to gravity (9.8 m/s²).
  • 📐 Record the spring stretch length (delta x) when a mass is hanging to determine the extension caused by the object's weight.
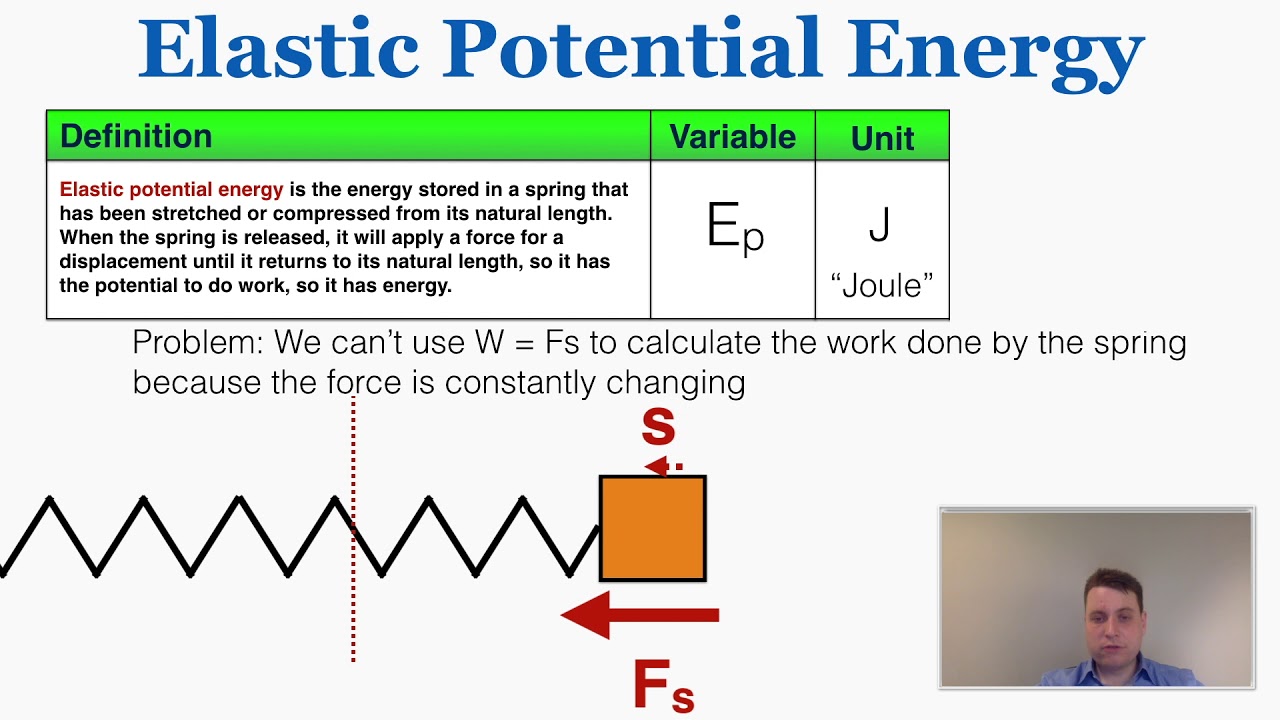
  • 🎢 The theoretical background involves Hooke's Law, where the spring force (k * delta x) equals the gravitational force (mg) at equilibrium.
  • 🔄 At least five different masses should be used to reduce error and improve the accuracy of the spring constant calculation.
  • 📈 Students should graph the weight of the object (mg) versus the spring stretch (delta x), with the slope representing the spring constant (k).
  • 🚀 In part B, the procedure involves hanging the mass on the spring, starting the stopwatch when the elevator accelerates, and stopping it when acceleration ceases.
  • ⏱️ The time measured on the stopwatch represents the duration of the elevator's acceleration phase.
  • 📊 During acceleration, the spring stretch (delta l) is determined by subtracting the initial spring length from the final spring length.
  • 🌟 The spring's length returns to its original length (10 cm) when the elevator reaches maximum speed and is in equilibrium, as forces are balanced.
  • 🔽 When the elevator decelerates while moving upwards with the same acceleration it had while speeding up, the spring's length will be shorter than 10 cm because the net force and thus the spring force is less.
Q & A

  • What is the main goal of the experiment described in the transcript?

    -The main goal of the experiment is to determine the spring constant (k) of a spring using a set of objects with known masses and a spring scale.

  • How can the weight of each object be calculated?

    -The weight of each object can be calculated by multiplying its mass by the acceleration due to gravity (approximately 10 meters per second squared).

  • What is the significance of measuring the spring stretch length (delta x)?

    -Measuring the spring stretch length (delta x) is important to determine the extension of the spring when a mass is hung from it, which is necessary for calculating the spring constant.

  • What is the theoretical basis for the spring force equation?

    -The theoretical basis for the spring force equation is Hooke's Law, which states that the force exerted by a spring (F) is proportional to the displacement from its equilibrium position (delta x), expressed as F = k * delta x, where k is the spring constant.

  • How does the experiment ensure accurate measurement of the spring constant?

    -The experiment ensures accurate measurement of the spring constant by repeating the procedure with different masses and calculating the average value, which helps to reduce error.

  • What is the relationship between the weight of the object and the spring stretch?

    -The weight of the object (mg) is equal to the spring force (k * delta x) at equilibrium. This relationship is used to determine the spring constant by graphing the weight of the object versus the spring stretch (delta x) and finding the slope of the line.

  • How can the measurements from the experiment be used to calculate maximum velocity (v max)?

    -The measurements can be used to calculate maximum velocity (v max) by first determining the acceleration (a) using the spring force equation and then applying the kinematic equation v max = a * t, where t is the time taken for the elevator to accelerate.

  • What happens to the spring length when the elevator reaches its maximum speed?

    -When the elevator reaches its maximum speed, the spring returns to its original length (10 centimeters) because the object is in equilibrium, and the forces are balanced.

  • Does the spring stretch length change when the elevator slows down with the same magnitude of acceleration as when it sped up?

    -Yes, the spring stretch length changes. When the elevator slows down with the same magnitude of acceleration as when it sped up, the spring actually becomes shorter than 10 centimeters because the gravitational force becomes greater than the spring force, causing the spring to compress.

  • How does the concept of equilibrium relate to the spring's length during the experiment?

    -The concept of equilibrium relates to the spring's length during the experiment in that when the object is at rest or moving at a constant velocity (equilibrium), the spring's length returns to its natural state, which was initially 10 centimeters.

  • What is the significance of the spring force and gravitational force in the context of the experiment?

    -In the context of the experiment, the spring force and gravitational force are significant because they are the two opposing forces that determine the extension or compression of the spring. The spring force is calculated based on the spring constant and the displacement, while the gravitational force is determined by the mass of the object and the acceleration due to gravity.

Outlines
00:00
🧪 Experimental Design for Measuring Spring Constant

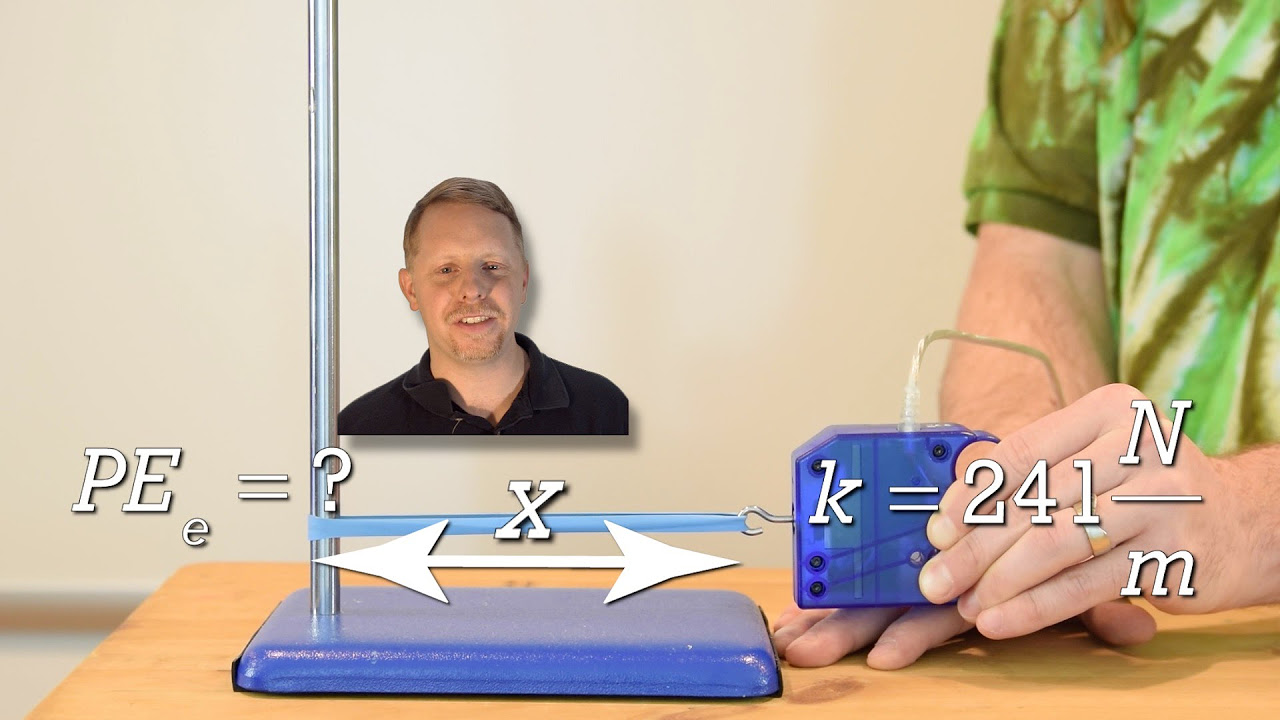
This paragraph introduces an experimental setup for determining the spring constant using a spring, meter stick, stopwatch, and objects of known masses. The goal is to measure the spring force and acceleration and apply the theoretical concept that at equilibrium, the force of gravity is balanced by the spring force. The procedure involves hanging the spring vertically, measuring the initial and final lengths to calculate the stretch (delta x), and using the mass and gravity to calculate the weight. The spring constant (k) is then determined by the relationship between the weight and the stretch length of the spring.

05:00
📈 Data Analysis and Graphing for Spring Constant

The second paragraph focuses on the data analysis part of the experiment, where the student is expected to graph the weight of the object hung from the spring against the spring stretch (delta x). The slope of this graph represents the spring constant (k). The paragraph also explains the theoretical basis behind the experiment, emphasizing that the spring force is equal to the weight of the object (mg = kx) and how this relationship can be used to calculate the spring constant. Additionally, it describes the procedure for measuring the initial and final spring lengths and calculating the stretch length (delta l) during an elevator's acceleration and deceleration.

10:04
🚀 Applying Spring Constant to Elevator Acceleration Scenarios

This paragraph delves into the application of the spring constant in scenarios involving an elevator's acceleration. It describes a situation where an object connected to a spring changes the spring's length based on the elevator's motion (accelerating upwards or decelerating). The explanation covers the concept of equilibrium, where the spring's length returns to its natural state when the object's acceleration equals zero. The paragraph also discusses how the spring's length changes when the elevator's acceleration is upwards or downwards, using the principles of force and acceleration to explain why the spring compresses or stretches in each case.

15:05
🌐 Real-World Implications of Elevator Acceleration on Perception of Weight

The final paragraph explores the real-world implications of elevator acceleration on the perception of weight. It explains the feeling of being lighter when an elevator is accelerating downwards and heavier when it accelerates upwards, due to the change in the supporting force required to counteract gravity. The paragraph uses the concept of net force and vector addition to illustrate how the body's sensation of weight changes with the elevator's acceleration. It concludes by reinforcing the importance of understanding both the experimental design and the concept of weight in the context of spring forces and acceleration.

Mindmap
Keywords
💡Spring Constant (k)
The spring constant, denoted by 'k', is a measure of the stiffness of a spring. In the context of the video, it represents how much force is needed to stretch or compress the spring by a certain distance. Angelica's experiment aims to determine the value of 'k' for a given spring by observing the stretch (delta x) caused by attaching objects of known masses. This is critical as it helps understand the force exerted by the spring in response to applied weights, illustrating Hooke's Law in practice.
💡Delta x (Δx)
Delta x, or Δx, refers to the change in length of the spring from its initial (unstretched) position to its final (stretched) position when a mass is hanging from it. In the video, measuring Δx is essential for calculating the spring constant 'k', as it provides a direct measurement of the spring's deformation in response to the applied weight. The experimental procedure outlines how to accurately measure this stretch for various masses to ensure precision in calculating 'k'.
💡Equilibrium
Equilibrium in the context of this video refers to the state where the upward force exerted by the spring (due to its stretching) equals the downward gravitational force on the mass. This balance of forces is central to determining the spring constant, as at equilibrium, the forces are equal and can be described by the equation Fg = kΔx. Understanding equilibrium is crucial for interpreting the experimental data, particularly in explaining why the spring returns to its initial length when the elevator stops accelerating.
💡Weight (Fg)
Weight, denoted as Fg in the video, is the force due to gravity acting on a mass. It is calculated by multiplying the mass (m) by the acceleration due to gravity (g, approximately 10 m/s^2). The concept of weight is fundamental in the experiment as it provides the downward force countered by the spring's force. The accurate measurement of each object's weight is necessary for calculating the spring constant 'k' through the relation Fg = kΔx.
💡Experimental Design
Experimental design refers to the structured plan developed to conduct experiments and collect data systematically. In the video, Angelica's experimental design includes setting up equipment, measuring initial and final lengths of the spring, and calculating its stretch (Δx) for different masses. This design aims to determine the spring constant 'k' by observing how the spring stretches under varying weights, illustrating the principles of physics in a controlled experiment.
💡Procedure
The procedure in the video outlines the specific steps Angelica must follow to conduct her experiment accurately. It includes hanging the spring, measuring its initial length, attaching masses, measuring the final length (and thus the stretch, Δx), and repeating the process for different masses. The procedure is crucial for ensuring consistency in data collection, reducing errors, and ultimately, calculating the spring's constant 'k' accurately.
💡Acceleration (a)
Acceleration, denoted as 'a', refers to the rate of change of velocity of an object. In the context of the video, acceleration plays a significant role in the later part of the experiment involving an elevator. The script explores how the spring's behavior changes under acceleration, such as when the elevator moves upwards or downwards, affecting the spring's stretch and thus enabling calculations related to the spring's force and the object's acceleration.
💡Velocity Max (Vmax)
Velocity Max, or Vmax, is the maximum speed reached by the elevator during the experiment. The video discusses how to calculate Vmax using the time of acceleration and the stretch of the spring (Δx). This concept is crucial for understanding how the spring's deformation under acceleration can provide insights into dynamic forces at play, beyond the static equilibrium scenarios initially considered.
💡Kinematics Equation
Kinematics equations are mathematical formulas used to describe the motion of objects without considering the forces causing the motion. In the video, a kinematics equation is used to relate the maximum velocity (Vmax) of the elevator to the spring's stretch (Δx) and the time of acceleration. This equation helps bridge the concepts of mechanical physics with motion, illustrating how physical properties like the spring constant influence dynamic systems.
💡Net Force (Fnet)
Net Force, denoted as Fnet in the video, is the total force acting on an object, accounting for all individual forces considered in a particular direction. Understanding net force is essential for analyzing the elevator's motion and the spring's stretch under different conditions of acceleration. By calculating the net force, one can deduce the overall effect of combined forces on the system's motion, crucial for understanding dynamics in physics.
Highlights

Angelica's experiment involves measuring spring force and acceleration using a spring, meter stick, stopwatch, and objects of known masses.

The goal of the experiment is to obtain a value for the spring constant of the spring using the provided equipment.

The weight of each object is calculated by multiplying its mass by the acceleration due to gravity (10 m/s^2).

The spring stretch length (delta x) is determined when a mass is hanging, which is the difference between the final and initial lengths of the spring.

At equilibrium, the force of gravity is equal to the spring force, which is defined by the equation F = k * delta x.

The experiment setup involves hanging the spring vertically from a stand and measuring the initial and final lengths to determine delta x.

The procedure includes hanging different masses on the spring and measuring the initial and final lengths at least five times to reduce error.

The student should graph the weight of the object versus the spring stretch (delta x), with the slope representing the spring constant (k).

The experiment also involves measuring the time it takes for an elevator to accelerate upwards and the spring stretch during this period.

The velocity max (v max) can be determined using the spring stretch measurements and the time it takes for the elevator to accelerate.

The acceleration (a) can be calculated using the equation a = (2 * k * delta l) / m.

At maximum speed, the spring length should return to its original length (10 cm) as the system reaches equilibrium.

When the elevator slows down with the same magnitude of acceleration as it had when speeding up, the spring will be shorter than 10 cm due to the downward acceleration.

The support force is less than the gravitational force when the elevator is accelerating downwards, making the spring compress.

The experiment demonstrates the relationship between spring force, mass, acceleration, and the concept of equilibrium.

The theoretical background of the experiment is based on Hooke's Law and the principles of equilibrium and Newton's Laws of Motion.

The experiment provides a practical application of physics concepts, allowing students to visualize and quantify theoretical principles.

Transcripts

Browse More Related Video

AP Physics Workbook 6.D Measuring Spring Constants

2022 AP Physics 1 Free Response #5

AP Physics Workbook 2.L Hooke's Law Spring

Elastic Potential Energy - IB Physics

AP Physics Workbook 6.E Equilibrium on an Incline

(previous version) Introduction to Elastic Potential Energy with Examples

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics ExperimentSpring ConstantHooke's LawAccelerationWeight MeasurementElevator DynamicsForce AnalysisEquilibrium ConceptsLab ProcedureKinematics