AP Physics Workbook 3.I The Conical Pendulum
TLDRThe video transcript discusses a physics problem involving a conical pendulum, also known as a tetherball. It explains why, regardless of the speed at which the ball is swung, the string cannot become completely horizontal. The explanation involves analyzing the forces acting on the ball, including gravity, tension, and the components of these forces. By setting up equations based on Newton's second law and trigonometric relationships, the video derives an equation that relates the ball's speed, the length of the string, and the angle between the string and the vertical. The conclusion is that as the angle approaches 90 degrees, the required velocity would approach infinity, making it impossible for the string to lie flat.
Takeaways
- 📚 The transcript discusses a physics problem related to circular motion and gravitation, specifically focusing on a conical pendulum scenario.
- 🏐 The scenario involves a tetherball, where a ball of mass M is attached to a string of length L and swung in a horizontal circle by a student.
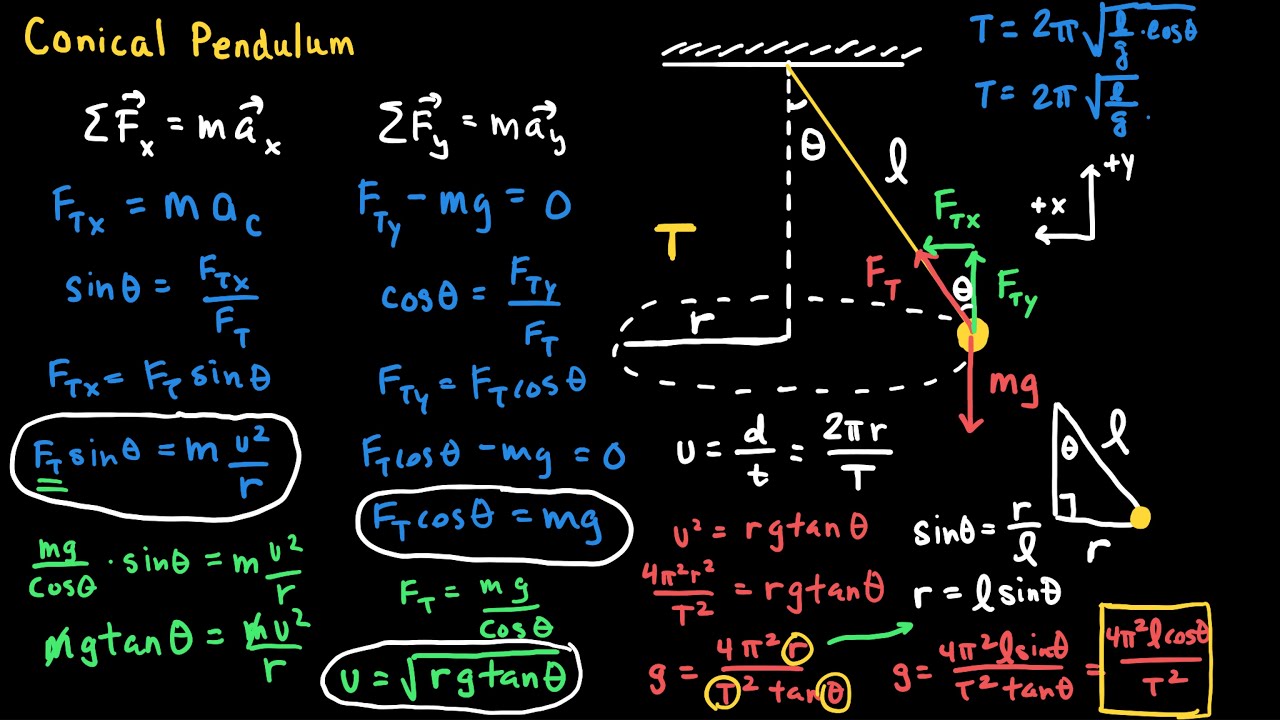
- 📈 A Freebody diagram is used to illustrate and label all the forces acting on the ball, including gravity, tension, and the forces tangential to the motion.
- 🔢 The problem explores why the string (rope) cannot become completely horizontal regardless of the speed at which the ball is swung.
- 🌐 The force of gravity always acts vertically downward, preventing the tension force from becoming completely horizontal.
- 📐 An equation is derived that relates the speed of the ball (V), the length of the string (L), and the angle (theta) between the string and the vertical.
- 🔄 The problem emphasizes the importance of understanding Newton's second law and its application to the system's acceleration.
- 📊 The solution process involves setting up and solving a system of equations, demonstrating the use of algebraic and trigonometric concepts.
- 🧮 The concept of tangent theta (tan θ) is crucial in understanding why the rope cannot be horizontal; as theta approaches 90 degrees, tan θ approaches infinity.
- 🛣️ The principles discussed are also applicable to real-world scenarios, such as banking roads for vehicles to travel around circular paths without the need for friction.
- 🎓 The transcript serves as a comprehensive guide for students studying physics, particularly in tackling conceptual problems involving circular motion and forces.
Q & A
What is the scenario described in the transcript?
-The scenario involves a student swinging a ball in a horizontal circle with a string of length L, attempting to make the string horizontal but never succeeding, regardless of the speed.
What is the significance of the angle Theta in this context?
-Theta represents the angle between the string and the vertical. As the student tries to swing the ball faster, the angle changes, affecting the forces acting on the ball.
What are the forces acting on the ball in the described scenario?
-The forces acting on the ball include the force of gravity (mg), downward; the force of tension (T), diagonally; the tangential force, horizontally inward; and the normal force, perpendicular to the surface.
Why does the string connected to the ball never become completely horizontal?
-The string cannot become completely horizontal because the force of gravity acting vertically will always have a component opposing the horizontal component of the tension force, preventing the string from laying flat.
How is the speed of the ball related to the angle Theta and the length L of the string?
-The speed of the ball (V) is related to Theta and L through the equation derived from the forces acting on the ball: V^2 = g * L * tan(Theta) / R, where R is the radius of the circular path and is equal to L * sin(Theta).
What does the equation V^2 = g * L * tan(Theta) / R imply about the ball's motion?
-This equation shows that the square of the ball's velocity is directly proportional to the product of the gravitational acceleration (g), the length of the string (L), and the tangent of the angle Theta, divided by the radius (R) of the circular motion. It helps to determine the required speed for a given angle and string length.
How does the concept of a conical pendulum relate to the tetherball scenario?
-A conical pendulum is a physical system where an object is attached to a string and swung in a circular path, similar to the tetherball. Both systems involve the interplay of gravitational, tension, and centripetal forces to keep the object in circular motion.
What is the significance of the tangent function in the equation for the ball's speed?
-The tangent function (tan) is the ratio of the opposite side to the adjacent side in a right triangle, which in this case corresponds to the ratio of the vertical and horizontal components of the tension force. It determines the relationship between the speed of the ball and the angle Theta.
What would happen if Theta approached 90 degrees?
-As Theta approaches 90 degrees, the tangent of Theta (tan(Theta)) would approach infinity, which is undefined. This means that the required velocity for the ball to maintain a horizontal string would also approach infinity, making it impossible to achieve.
How does the concept of a banked road relate to the physics discussed in the transcript?
-A banked road uses the principles of circular motion and centripetal force to keep vehicles on the road without relying solely on friction. The banking angle helps provide the necessary centripetal force to maintain circular motion, similar to how the tension and gravity forces work together in the tetherball scenario.
What is the role of Newton's second law in setting up the equations for this problem?
-Newton's second law (F = ma) is used to establish the relationship between the forces acting on the ball and its acceleration. By considering the components of forces in both vertical and horizontal directions, the law allows us to derive the equations needed to solve for the ball's speed and the angle Theta.
Outlines
📚 Introduction to Tetherball Physics
The video begins with an introduction to a physics problem involving a tetherball, a game where a ball is swung around in a circle by a rope. The focus is on understanding the forces acting on the ball, particularly when the string connecting the ball to the player makes an angle with the vertical. The scenario describes an impossible situation where, no matter how fast the ball is swung, the string cannot become horizontal due to the forces of tension, gravity, and the centripetal force required for circular motion.
🔍 Analyzing Forces and Components
The second paragraph delves into a detailed analysis of the forces acting on the ball, including gravity, tension, and the components of these forces in both horizontal and vertical directions. It explains how the vertical component of the tension force cannot be zero because it is counteracted by gravity. The paragraph also introduces the concept of using Newton's second law to derive an equation that relates the ball's speed, the radius of the circle, and the angle theta.
🧮 Deriving Equations for Motion
This paragraph focuses on the mathematical derivation of equations that describe the motion of the tetherball. It explains how to use the equations of motion to find the relationship between the ball's velocity, the length of the string, and the angle theta. The explanation includes the application of trigonometric identities and the concept of the radius being equal to the length of the string times the sine of the angle theta.
📈 Demonstrating the Impossibility of a Horizontal Rope
The fourth paragraph concludes the analysis by showing that the rope cannot be horizontal regardless of the ball's speed. It explains this by examining the limit as the angle theta approaches 90 degrees, where the tangent of theta becomes undefined, indicating that the velocity would have to be infinite to achieve a horizontal rope. This part of the video script reinforces the understanding of the physical limitations of the system and the importance of trigonometric functions in analyzing such problems.
🚗 Applying Physics to Real-World Scenarios
The final paragraph of the script applies the learned concepts to a real-world scenario of a car traveling around a circular road. It explains how banking the road can help a car maintain traction without relying on friction, as the centripetal force required for circular motion is provided by the road's incline. This example demonstrates the practical application of the principles discussed in the previous paragraphs.
Mindmap
Keywords
💡Circular Motion
💡Gravitation
💡Tension Force
💡Freebody Diagram
💡Theta (θ)
💡Components of Forces
💡Newton's Second Law
💡Tangent (tan)
💡Velocity
💡Banked Road
Highlights
The scenario involves a conical pendulum, specifically a ball attached to a string and swung in a horizontal circle by a student.
The angle between the string and the vertical is denoted as Theta.
No matter the speed at which the ball is swung, the string never becomes completely horizontal.
A free body diagram is drawn to illustrate and label all the forces acting on the ball.
The forces include the force of gravity (mg) acting downwards, the tension force (T) diagonally, and the tangential force in the x-direction.
The problem is analogous to a tetherball, where the rope is tight and the ball is swung horizontally.
An equation is derived that relates the speed of the ball (V), the length of the string (L), and the angle (Theta).
The vertical component of the tension force in the Y-direction is balanced by the force of gravity, resulting in no net acceleration in the Y-direction.
The horizontal component of the tension force is given by T sine Theta and is equal to the centripetal force required for circular motion (M V^2 / R).
The radius (R) of the circular motion is defined as L sine Theta.
The final derived equation shows that the velocity of the ball is V = sqrt((g L tan Theta) / sin Theta).
The rope cannot be completely horizontal because the tangent of Theta (tan Theta) approaches infinity as Theta approaches 90 degrees, which is undefined.
The problem demonstrates the application of Newton's second law and the principles of circular motion.
The analysis involves setting up and solving a system of equations using algebraic and trigonometric principles.
The discussion highlights the importance of understanding the relationship between the forces acting on an object in circular motion and its velocity.
The practical application of the concepts is shown through the analogy of a car traveling around a circular road with banking.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: