Indefinite integral of 1/x | AP Calculus AB | Khan Academy

Khan Academy
25 Jan 201307:34
EducationalLearning
32 Likes 10 Comments

TLDRThe video explores the antiderivative of 1/x, highlighting the limitations of using the natural log of x due to its domain restrictions. It suggests using the natural log of the absolute value of x as a more suitable antiderivative, as it extends the domain to include negative values. The video visually demonstrates how the derivative of the natural log of the absolute value of x equals 1/x for all x not equal to 0, thus providing an antiderivative with the same domain as the original function.

Takeaways
  • 📚 The antiderivative of 1/x can be approached by considering x to the negative 1 power.
  • 🚫 Applying an anti-power rule directly leads to an undefined expression, x^0/0.
  • 🤔 The natural log of x (ln x) has a derivative equal to 1/x, but its domain is limited to positive numbers.
  • 📈 Expanding the domain, the antiderivative of 1/x should be defined for all real numbers except x = 0.
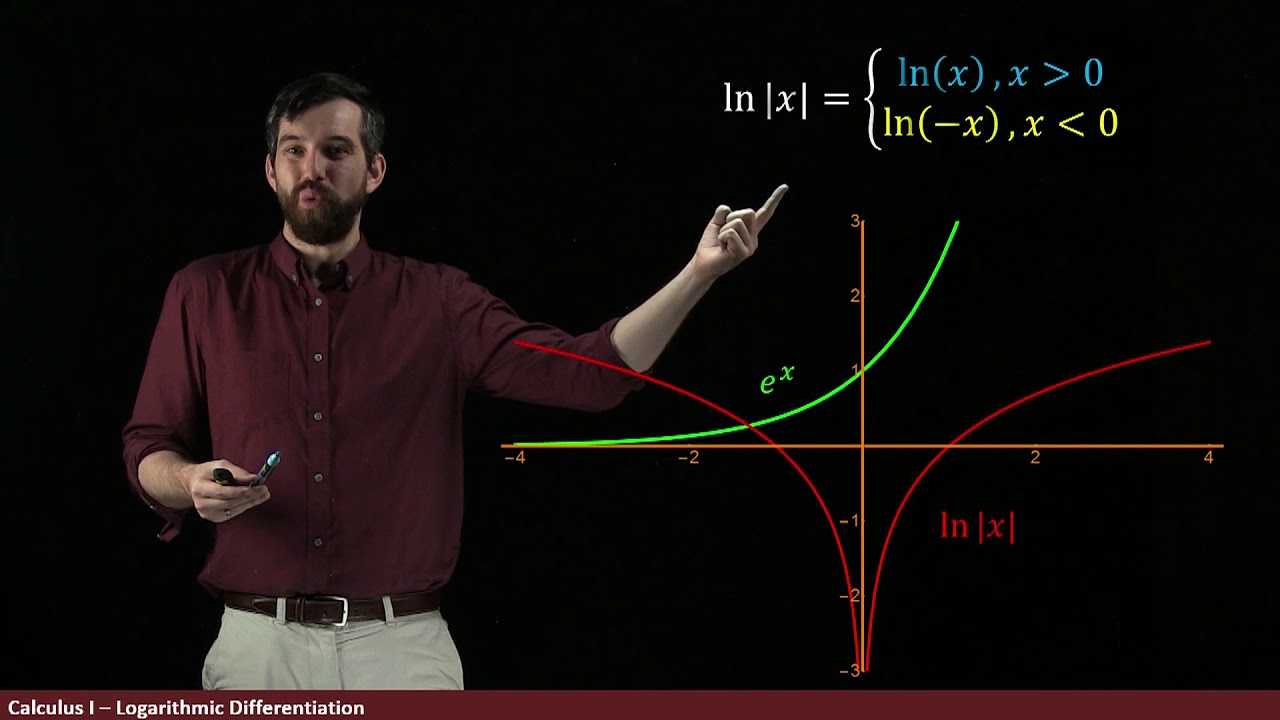
  • 🌀 Considering the natural log of the absolute value of x (ln |x|) extends the domain to include negative values.
  • 🔄 The graph of ln |x| is symmetric around the y-axis, providing a function defined everywhere except at x = 0.
  • 📊 The derivative of ln x for positive x values is 1/x, which matches the original function we are integrating.
  • 📐 For negative x values, the derivative of ln |x| mirrors the positive side around the y-axis, maintaining the relationship with 1/x.
  • 🎭 A visual understanding supports that the derivative of ln |x| equals 1/x for all x not equal to 0, making it a suitable antiderivative.
  • 📝 The antiderivative of 1/x is the natural log of the absolute value of x plus a constant (ln |x| + C), matching the domain of the original function.
Q & A

  • What is the antiderivative being discussed in the video?

    -The antiderivative being discussed is the antiderivative of 1/x, which can also be written as the antiderivative of x to the negative 1 power.

  • Why is applying the anti-power rule directly to 1/x not possible?

    -Applying the anti-power rule directly to 1/x leads to an undefined expression, x to the 0 over 0, which does not make sense.

  • What is the issue with equating the antiderivative of 1/x to the natural log of x plus c?

    -The issue is that the domain of the natural log of x is only positive numbers, while the original function 1/x has a domain of all real numbers except x equals 0. Therefore, equating them does not cover the entire domain of the original function.

  • How does the video suggest modifying the antiderivative to cover a broader domain?

    -The video suggests modifying the antiderivative to the natural log of the absolute value of x, which would then be defined for both positive and negative values, excluding only x equals 0.

  • What does the graph of the natural log of x look like?

    -The graph of the natural log of x is a curve that starts from the left side for positive x's and is a mirror image for negative x's, reflecting around the y-axis, excluding the point x equals 0.

  • What is the derivative of the natural log of the absolute value of x for x greater than 0?

    -For x greater than 0, the derivative of the natural log of the absolute value of x is equal to the derivative of the natural log of x, which is 1/x.

  • How does the derivative of the natural log of the absolute value of x behave for x less than 0?

    -For x less than 0, the derivative of the natural log of the absolute value of x is symmetric to the derivative for x greater than 0 across the y-axis, starting with a slope close to 0 and becoming increasingly negative as x approaches 0.

  • What is the final expression for the antiderivative of 1/x that matches its domain?

    -The final expression for the antiderivative of 1/x that matches its domain is the natural log of the absolute value of x plus c.

  • How does the visual representation in the video support the understanding of the antiderivative of 1/x?

    -The visual representation in the video helps to understand the behavior of the antiderivative by showing the graph of the natural log of x and its reflection for negative values, as well as the behavior of the derivative for both positive and negative x values.

  • Why is it important for the antiderivative to have the same domain as the original function?

    -It is important for the antiderivative to have the same domain as the original function to ensure that the antiderivative is a valid reverse operation of the derivative, covering all the points where the original function is defined.

Outlines
00:00
📚 Introduction to the Antiderivative of 1/x

The paragraph begins with an exploration of the antiderivative of the function 1/x, or x to the negative 1 power. It highlights the challenge of applying an anti-power rule, which leads to an undefined expression of x to the 0 over 0. The speaker then poses a question about using the natural log of x as the antiderivative, noting that this solution is not broad enough due to the domain restrictions of the original function (all real numbers except for x equals 0) and the proposed antiderivative (only positive numbers). The paragraph aims to find an antiderivative that matches the domain of the original function, suggesting the natural log of the absolute value of x as a potential solution. The speaker provides a conceptual understanding without a rigorous proof and discusses the graph of the natural log of x, indicating how the graph of the natural log of the absolute value of x would look, including its symmetry around the y-axis for negative values of x.

05:01
📈 Derivative and Graph Analysis of the Natural Log of Absolute Value of x

This paragraph delves into the derivative of the natural log of the absolute value of x, providing a visual understanding of its behavior. It describes the high value of the derivative near 0 and how it becomes less steep as x moves away from 0, never reaching a completely flat slope. The paragraph also discusses the symmetry of the slope for negative values of x, where the slope on one side is the negative of the slope at a symmetric point on the other side. The speaker explains that the derivative of the natural log of the absolute value of x is equal to 1/x for all x not equaling 0, making it a more satisfying antiderivative for 1/x since it has the same domain as the function. The paragraph concludes with a visualization of the derivative and its behavior, reinforcing the idea that the natural log of the absolute value of x plus c is an appropriate antiderivative for 1/x.

Mindmap
Keywords
💡antiderivative
The antiderivative is a fundamental concept in calculus that represents the reverse process of differentiation. It is used to find the original function from its derivative. In the context of the video, the antiderivative of 1/x is explored, which is related to the natural log function and its domain restrictions.
💡natural log
The natural log, or logarithm with base e, is a mathematical function that calculates the power to which e must be raised to obtain a given value. It is closely related to the exponential function and is used extensively in various fields of mathematics, science, and engineering. In the video, the natural log of x is used as a basis for finding the antiderivative of 1/x.
💡domain
The domain of a function is the set of all possible input values (x-values) for which the function is defined. It is crucial in understanding the range of values a function can accept. In the video, the domain of the original function (1/x) and its antiderivative (natural log of x) are discussed, highlighting the importance of their alignment.
💡absolute value
The absolute value of a number is its distance from zero on the number line, regardless of direction. It is used to represent distances or magnitudes without considering the sign of the number. In the video, the absolute value of x is used to extend the domain of the natural log function to include negative values of x.
💡derivative
The derivative of a function is a measure of how the function changes as its input changes. It is a fundamental concept in calculus that describes the rate of change or slope of a function at any given point. The video discusses the derivative of the natural log function and how it relates to finding the antiderivative of 1/x.
💡asymptote
An asymptote is a line that a function approaches but never actually intersects, no matter how far the input values extend. It is used to describe the behavior of a function at its limits. In the video, the behavior of the function 1/x and its antiderivative are discussed in relation to their asymptotes.
💡slope
The slope of a function at a particular point is the rate of change of the function's output with respect to a small change in the input at that point. It represents the steepness of the function's graph. The video discusses the concept of slope in relation to the derivative and the behavior of the function 1/x and its antiderivative.
💡reflection
Reflection, in the context of functions and their graphs, refers to the process of flipping a graph across a line, typically the y-axis or the x-axis. This transformation does not change the shape of the graph but its orientation. In the video, the concept of reflection is used to describe the graph of the natural log of the absolute value of x.
💡symmetry
Symmetry in mathematics refers to a property of an object or function that allows it to be mapped onto itself in a certain way. For functions, this often means that for every positive value, there is a corresponding negative value that yields the same result. The video discusses the symmetry of the derivative of the natural log of the absolute value of x around the y-axis.
💡visual understanding
Visual understanding is the ability to comprehend and interpret information presented in a visual format, such as graphs or diagrams. It is a crucial skill in mathematics and science for grasping complex concepts. The video aims to provide a visual understanding of the antiderivative of 1/x through the use of graphs and conceptual explanations.
Highlights

The antiderivative of 1/x is explored, which is another way of writing the antiderivative of x to the negative 1 power.

Applying the anti-power rule to 1/x leads to an undefined expression, x to the 0 over 0.

The derivative of the natural log of x is equal to 1/x, which is a known result from calculus.

The antiderivative of the natural log of x plus c is suggested but noted to have a limited domain of only positive numbers.

The need for an antiderivative that matches the domain of the original function, which is all real numbers except for x equals 0, is emphasized.

The concept of using the natural log of the absolute value of x is introduced as a potential antiderivative that could work for both positive and negative values.

A conceptual understanding is provided by visualizing the graph of the natural log of x and its absolute value.

The graph of the natural log of the absolute value of x is described as being the original graph reflected around the y-axis for negative x values.

The derivative of the natural log of the absolute value of x is discussed to be equal to 1/x for all x not equal to 0, which matches the original function's domain.

The derivative of the natural log of x for positive values is restated as 1/x.

The behavior of the derivative of 1/x near 0 is described as having a very steep positive slope on the right and a very negative slope on the left.

The symmetry of the natural log of the absolute value of x is highlighted, with the slope for negative x being the negative of the slope for the symmetric positive x value.

The visual representation of the derivative of the natural log of the absolute value of x is discussed, showing a transition from a positive to a negative slope.

The antiderivative of 1/x is concluded to be the natural log of the absolute value of x plus c, which has the same domain as the original function.

The approach taken is more of a visual understanding rather than a rigorous mathematical proof.

The antiderivative found is considered more satisfying as it matches the domain of the function it is derived from.

Transcripts

Browse More Related Video

The Derivative of ln(x) via Implicit Differentiation

Integration by parts: ºln(x)dx | AP Calculus BC | Khan Academy

Definite integral involving natural log | AP Calculus AB | Khan Academy

2011 Calculus AB free response #6b | AP Calculus AB | Khan Academy

Natural Log Function Integration

Area between a curve and and the _-axis | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusAntiderivativeNatural LogLimitsDomainSlopeAsymptotesMathematicsEducationalVisual Explanation