Integration by parts: ºln(x)dx | AP Calculus BC | Khan Academy

Khan Academy
13 Feb 201303:49
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a step-by-step guide to finding the antiderivative of the natural log of x using integration by parts. It explains the process of selecting functions f and g, and how to apply the integration by parts formula. The video simplifies the integral to x times the natural log of x minus the antiderivative of 1 over x, which is x, and then adds a constant of integration, c. It encourages viewers to verify the result by differentiating the antiderivative to obtain the original function.

Takeaways
  • 📚 The video's objective is to determine the antiderivative of the natural log of x (∫ln(x)dx).
  • 🤔 Initially, it's not clear how to approach the problem, as there's only one function (ln(x)) and integration by parts requires two functions.
  • 📝 By rewriting the integral as ∫(ln(x) * 1)dx, we create a product of two functions, allowing the use of integration by parts.
  • 🌟 Integration by parts is a method for integrating the product of two functions, and it can be thought of as the reverse of the product rule for derivatives.
  • 🎯 The choice for f(x) should be a function whose derivative is easy to compute and simplifies the integral, in this case, f(x) = ln(x) with f'(x) = 1/x.
  • 🔄 For g'(x), we want a function whose antiderivative is easy to find, and since g'(x) = 1, g(x) should be x.
  • 🧩 Applying integration by parts, we get the result as x*ln(x) - ∫f'(x)g(x)dx, simplifying to x*ln(x) - ∫(1/x)dx.
  • 📊 The integral of 1/x is ln(x), and thus the antiderivative of 1 (with respect to x) is -x, leading to the final result of x*ln(x) - x + C.
  • 🔧 The video encourages viewers to verify the result by differentiating the antiderivative to see if they obtain the original function, ln(x).
  • 📈 The process demonstrates the utility of integration by parts and the product rule in solving more complex integrals.
Q & A

  • What is the main goal of the video?

    -The main goal of the video is to determine the antiderivative of the natural log of x.

  • Why might it be difficult to initially approach the problem?

    -It might be difficult because the natural log of x appears as a single function, not as a product of two functions, which is typically required for integration by parts.

  • How does rewriting the integral as ∫(natural log of x) * 1dx help?

    -Rewriting the integral this way creates a product of two functions, which allows the use of integration by parts to solve for the antiderivative.

  • What is the formula for integration by parts?

    -Integration by parts states that ∫(f * g')dx = f * g - ∫(f' * g)dx, where f and g are functions of x, and f' and g' are their respective derivatives.

  • What criteria should be considered when choosing f and g for integration by parts?

    -For f, choose a function whose derivative is easy to calculate and simplifies the expression. For g', choose a function whose antiderivative is easy to find.

  • What are the chosen functions f and g' in this context?

    -The chosen function f is the natural log of x, with its derivative f' being 1/x. The chosen function g' is 1, which implies that g is x.

  • How does the antiderivative of the natural log of x simplify?

    -The antiderivative simplifies to x * (natural log of x) - (antiderivative of 1/x * x), which further simplifies to x * (natural log of x) - x, as 1/x * x equals 1.

  • What is the final form of the antiderivative of the natural log of x?

    -The final form of the antiderivative is x * (natural log of x) - x, with the addition of a constant 'c' for the general class of antiderivatives.

  • How can you verify the solution is correct?

    -You can verify the solution by taking the derivative of the antiderivative and checking if it results in the original function, which is the natural log of x.

  • What rule is suggested to use for verification?

    -The product rule is suggested for verification when taking the derivative of the found antiderivative.

  • What is the significance of the video's approach?

    -The video's approach demonstrates a method for solving more complex integrals, particularly those that do not immediately lend themselves to standard techniques like integration by parts.

Outlines
00:00
📚 Introduction to Finding the Antiderivative of ln(x)

This paragraph introduces the video's objective, which is to determine the antiderivative of the natural logarithm of x (ln(x)). It acknowledges the initial challenge in approaching this problem, especially when considering the use of integration by parts. The speaker suggests that the problem might become clearer by rewriting the integral as the product of ln(x) and 1dx, thus satisfying the criteria for using integration by parts. The paragraph explains the concept of integration by parts, which involves breaking down an integral into the product of two functions, one of which is the derivative of the other. The goal is to identify functions f(x) and g(x) such that their product can be integrated, and their roles can be reversed for the antiderivative calculation. The paragraph sets the stage for the subsequent steps by identifying ln(x) as a potential function f(x) and 1 as g'(x), leading to the conclusion that g(x) could be x. The paragraph ends with the formation of the integral expression x*ln(x) - ∫(1/x)dx, setting the foundation for the next steps in the video.

Mindmap
Keywords
💡antiderivative
The antiderivative is a fundamental concept in calculus that represents a function whose derivative is another given function. In the context of this video, the main goal is to find the antiderivative of the natural log of x, which is a key step in solving integrals. The antiderivative is used to reverse the process of differentiation, allowing us to find the original function from its derivative.
💡integration by parts
Integration by parts is a technique used in calculus to evaluate integrals when the integrand is a product of two functions. The method is based on the product rule for derivatives, which is reversed to find antiderivatives. In the video, integration by parts is suggested as a method to find the antiderivative of the natural log of x, by identifying u and dv, which leads to the formula for the integral in terms of the product of the functions and the antiderivative of the other function.
💡natural log of x
The natural log of x, denoted as ln(x), is a logarithmic function where the base of the logarithm is the mathematical constant e (approximately equal to 2.71828). It is a fundamental concept in mathematics and has applications in various fields, including calculus. In the video, the focus is on finding the antiderivative of ln(x), which is a key concept in understanding the integration process.
💡product of two functions
A product of two functions refers to the result of multiplying two functions together, which is a common scenario in integration problems. In the video, the integral of the natural log of x is initially seen as a single function, but it is rewritten as the product of ln(x) and 1, which allows the application of integration by parts. This highlights the importance of recognizing and manipulating products of functions in solving integrals.
💡derivative
A derivative is a mathematical concept that represents the rate of change or the slope of a function at a particular point. In the process of finding antiderivatives, derivatives are the starting point, as antiderivatives 'undo' the differentiation process. The video discusses the derivative of the natural log of x, which is 1/x, and this understanding is crucial for applying integration by parts.
💡f and g functions
In the context of integration by parts, f and g are the two functions that make up the product being integrated. The selection of these functions is crucial to simplify the process and make the calculations manageable. In the video, f(x) is chosen to be the natural log of x, whose derivative is easily found, and g'(x) is chosen to be 1, which has a straightforward antiderivative, leading to the solution of the integral.
💡1dx
1dx is an expression used in integral calculus to denote an infinitesimal amount of x, where 'dx' represents an infinitesimally small change in the variable x, and the '1' signifies that it is multiplied by the function's value at that point. In the video, after applying integration by parts, the integral simplifies to the antiderivative of 1dx, which is simply -x, as the integral of 1 with respect to x is -x.
💡product rule
The product rule is a fundamental calculus rule that describes how to differentiate the product of two functions. It states that the derivative of a product is the derivative of the first function times the second function plus the first function times the derivative of the second function. In the video, the product rule is mentioned in the context of verifying the solution by differentiating the found antiderivative to ensure it yields the original function, ln(x).
💡constant
A constant is a value that does not change within the context of a given function or equation. In the script, the number 1 is considered a constant because it does not vary with x and is used as a placeholder in the integral expression. The concept of constants is important in calculus as they can simplify the integration process and are often factored out or treated separately.
💡x natural log of x
The expression 'x natural log of x' represents the product of the variable x and the natural logarithm of x. This is the result of the integration by parts process in the video, where the antiderivative of the natural log of x is found to be x times the natural log of x, minus the antiderivative of the derivative of the chosen function, which simplifies to -x.
💡antiderivative of 1
The antiderivative of 1, with respect to x, is a basic concept in calculus that represents the function whose derivative is the constant 1. As per the fundamental theorem of calculus, the antiderivative of 1 is simply x, as the derivative of x is 1. In the video, this concept is used to simplify the integral of 1dx, which is part of the process of finding the antiderivative of the natural log of x.
Highlights

The video's objective is to determine the antiderivative of the natural log of x, a fundamental concept in calculus.

Integration by parts is suggested as the method to find the antiderivative, even though it might not be immediately apparent how to apply it.

The natural log of x is rewritten as the integral of the natural log of x times 1dx to facilitate the use of integration by parts.

Integration by parts is expressed as the product of two functions, one of which is the derivative of another, which is key to solving the integral.

The choice of functions f and g is critical; f should have an easy derivative, and g should have an easy antiderivative.

The natural log of x is chosen as f(x) because its derivative is 1/x, simplifying the process.

g'(x) is chosen to be 1, which implies that g(x) could be x, making the integration process straightforward.

The antiderivative is expressed as x natural log of x minus the antiderivative of 1/x times x, which simplifies to x natural log of x - x.

The integral of 1dx, or the antiderivative of 1, is simply -x, which is a crucial step in the calculation.

The final antiderivative expression includes a constant 'c', which is a standard part of any antiderivative.

The video encourages viewers to take the derivative of the found antiderivative to verify that it results in the natural log of x, reinforcing the correctness of the method.

The use of integration by parts for a single function is an innovative approach that can be applied to other complex integrals.

The process of selecting f and g based on their derivatives and antiderivatives is a strategic decision that can be generalized to other problems.

The explanation is clear and methodical, making it an excellent resource for learning integration techniques.

The video demonstrates the product rule in reverse, highlighting the connection between differentiation and integration.

The step-by-step breakdown of the integration by parts method is thorough and educational, providing a strong foundation for understanding complex integrals.

The video's approach to solving the integral of the natural log of x is both practical and theoretically sound, offering a valuable learning experience.

Transcripts

Browse More Related Video

Integration by parts: ºx____dx | AP Calculus BC | Khan Academy

Definite integral involving natural log | AP Calculus AB | Khan Academy

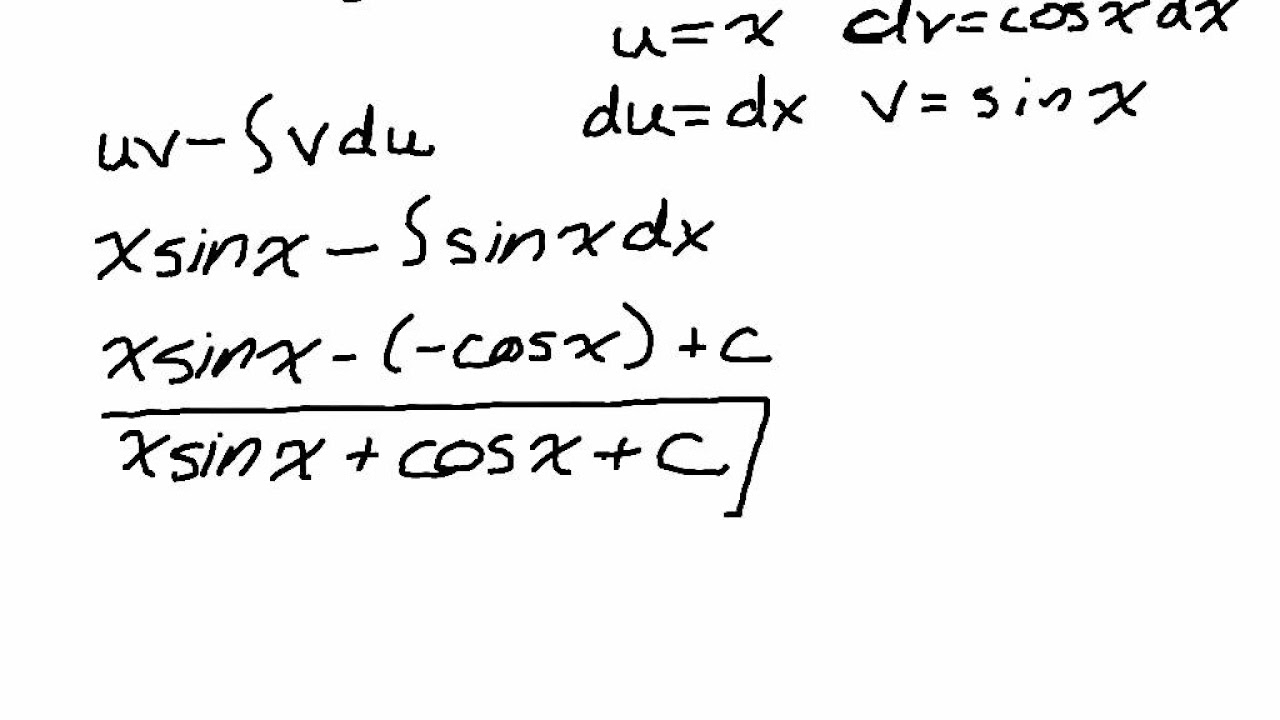
Calc BC - Integration by Parts - Part 1

(2^ln x)/x Antiderivative Example

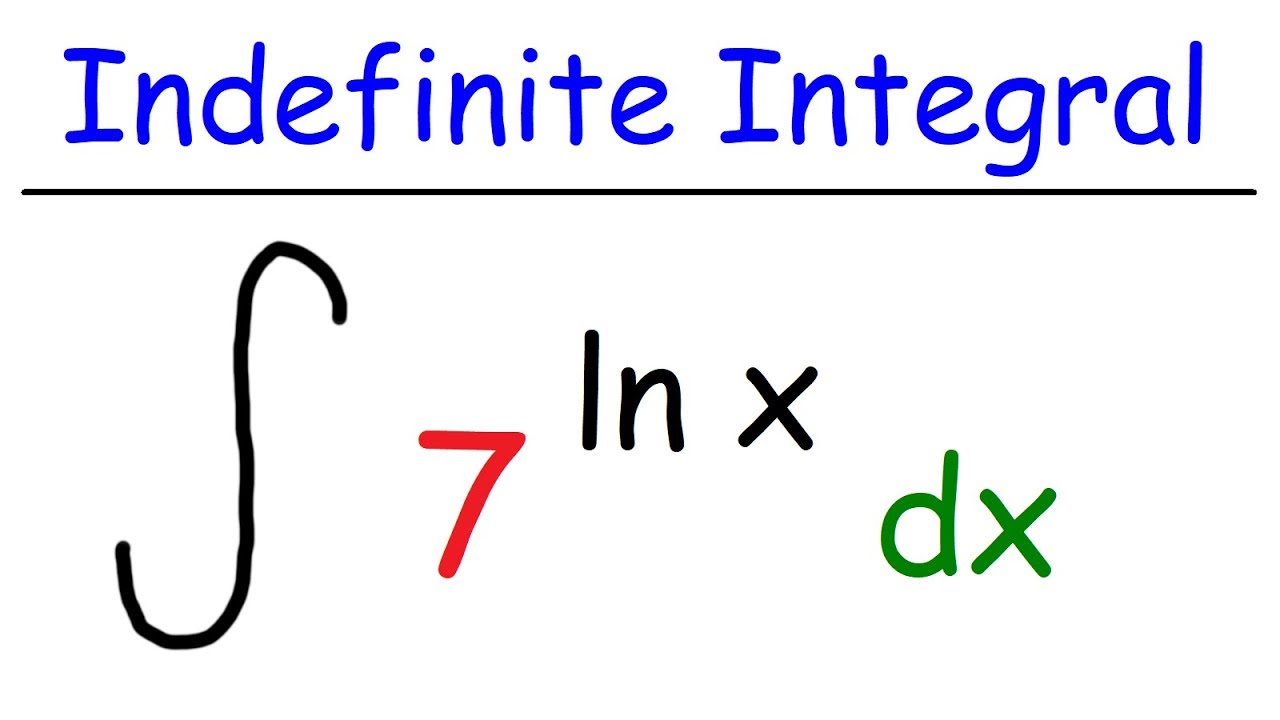
Indefinite Integral of 7^lnx

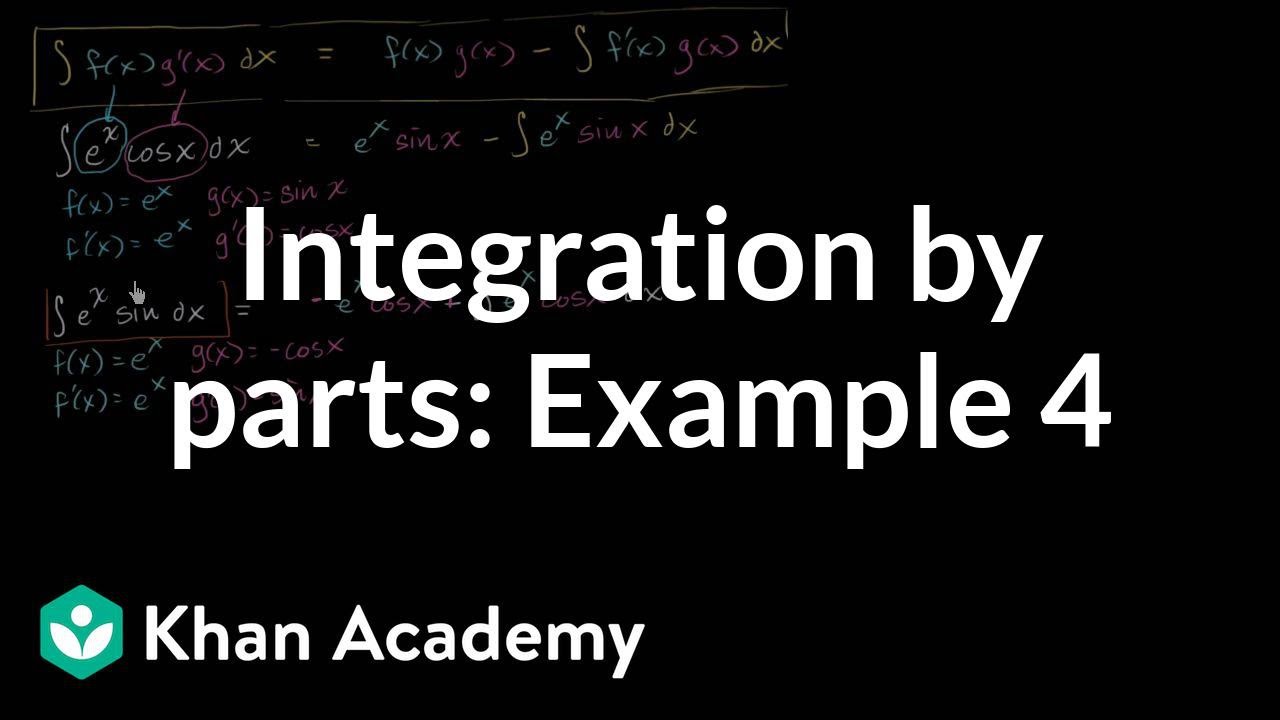
Integration by parts: º___cos(x)dx | AP Calculus BC | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegrationAntiderivativesNatural LogarithmIntegration by PartsMathematicsTutorialEducationMath TipsDifferential Calculus