The Derivative of ln(x) via Implicit Differentiation
TLDRThe video script discusses the natural logarithm (Ln) function, which is the inverse of the exponential function e^x. It explains that the natural logarithm is only defined for positive numbers, resulting in a restricted domain for its inverse function. To find the derivative of the natural logarithm, the video employs implicit differentiation, starting with y = ln(x) and transforming it into x = e^y. Applying the chain rule to differentiate both sides with respect to x yields dy/dx = 1/(e^y), which simplifies to 1/x when considering the original equation e^y = x. The video also explores the natural logarithm of the absolute value of x, which extends the domain to include negative numbers by considering the natural logarithm of -x. The derivative of ln(|x|) is shown to be 1/x for both positive and negative values of x, excluding zero. This comprehensive explanation of the natural logarithm and its derivative is crucial for understanding antiderivatives and other advanced calculus concepts.
Takeaways
- 📈 The natural logarithm, denoted as ln(x), is the inverse function of e^x.
- 🚫 The domain of ln(x) is restricted to positive numbers only, meaning x must be greater than zero.
- 🔄 When graphed, ln(x) and e^x are reflections of each other over the line y=x.
- 🧮 To find the derivative of ln(x), implicit differentiation is used because it is an inverse function.
- ⛓ Applying the chain rule to x = e^y helps in finding the derivative of ln(x) with respect to x.
- 💡 The derivative of ln(x) with respect to x is found to be 1/x, considering the restricted domain.
- 🔁 The function 1/x has a domain of all real numbers except zero, which contrasts with ln(x)'s domain.
- 🌐 To extend the domain of ln(x), consider ln(|x|), which includes negative values by using the absolute value.
- 📉 For x < 0, ln(|x|) is equivalent to ln(-x), which reflects the graph of ln(x) across the y-axis.
- 🔄 The derivative of ln(-x) for x < 0 is found using the chain rule, resulting in -1/x.
- ✅ The final derivative of ln(x), considering both positive and negative x values, is 1/x with a domain of all real numbers except zero.
Q & A
What is the natural logarithm, ln(x), defined as?
-The natural logarithm, ln(x), is defined as the inverse function to e^x. This means that when you graph e^x and its inverse, you get a reflection over the line y=x, resulting in the natural logarithm function.
What is the domain restriction for the natural logarithm function?
-The natural logarithm function is only defined for positive numbers. This means that x must be greater than zero for ln(x) to be computed.
How do you find the derivative of the natural logarithm function?
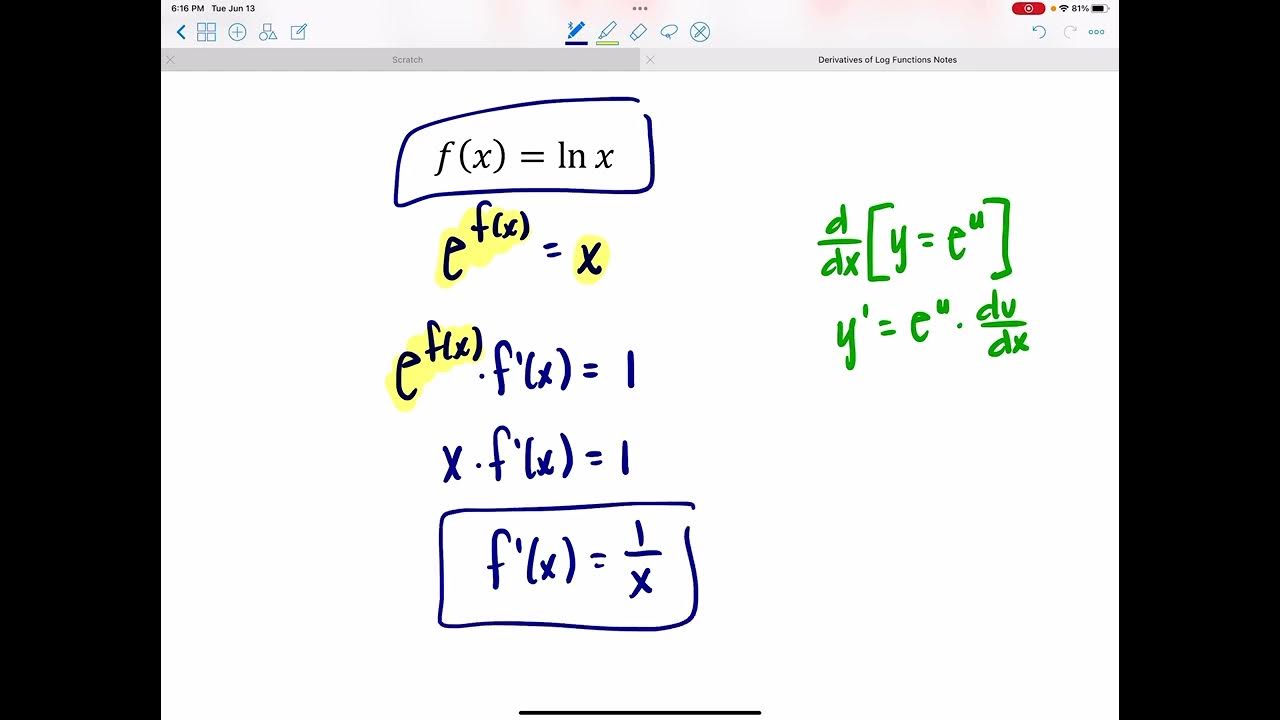
-To find the derivative of the natural logarithm function, you use implicit differentiation. You start by expressing y as ln(x), which implies that x = e^y. Then, you differentiate both sides with respect to x, applying the chain rule where necessary, to find that dy/dx = 1/x.
What is the domain of the function 1/x?
-The function 1/x has a domain of all real numbers except zero, as division by zero is undefined.
How does the domain of ln(x) differ from that of 1/x?
-The domain of ln(x) is only positive numbers, whereas the domain of 1/x includes all real numbers except zero. This creates an asymmetry between the two functions.
What is the natural logarithm of the absolute value of x, and how is it defined?
-The natural logarithm of the absolute value of x, denoted as ln(|x|), is a piecewise-defined function. When x is greater than zero, it is simply ln(x). When x is less than zero, it is ln(-x), which is the same as -ln(x).
How does the graph of ln(|x|) differ from the graph of ln(x) when x is less than zero?
-When x is less than zero, the graph of ln(|x|) reflects the negative values of x to positive values, effectively creating a mirror image of the ln(x) graph over the y-axis for negative x values.
What is the domain of ln(|x|)?
-The domain of ln(|x|) is all real numbers except zero, as it includes both positive and negative values of x, with the absolute value ensuring that x is never zero.
How do you find the derivative of ln(|x|) when x is less than zero?
-When x is less than zero, the derivative of ln(|x|) is found using the chain rule. Since ln(|x|) is ln(-x) in this case, the derivative is the derivative of the natural logarithm function, which is 1/x, multiplied by the derivative of the inside function, which is -1. This results in a derivative of -1/x.
What is the domain of the derivative of ln(x) and ln(|x|)?
-The domain of the derivative of both ln(x) and ln(|x|) is all real numbers except zero, as the derivative 1/x is undefined at x = 0.
Why is the derivative of ln(|x|) useful in calculus?
-The derivative of ln(|x|) is useful in calculus because it allows for the integration of functions involving absolute values, which can be important in various applications where the context requires consideration of magnitude regardless of direction or sign.
Outlines
📈 Introduction to Natural Logarithm and its Derivative
The video begins by focusing on the natural logarithm, denoted as ln(x), which is the inverse function of e^x. The presenter explains that when graphed, the natural logarithm and its inverse e^x are symmetrical over the line y=x. It is noted that the natural logarithm is only defined for positive values of x, which consequently restricts its inverse to positive values as well. The derivative of the natural logarithm is then explored using implicit differentiation. By differentiating x = e^y (where y is a function of x), the presenter derives that dy/dx = 1/(x), given that x > 0 to avoid division by zero. The video also touches on the concept of natural logarithm of the absolute value of x, which extends the domain of the function to all real numbers except zero, and its graphical representation.
Mindmap
Keywords
💡Natural logarithm
💡Inverse function
💡Domain
💡Implicit differentiation
💡Chain rule
💡Derivative
💡Absolute value
💡Piecewise function
💡Exponential function
💡Antiderivatives
💡Graph
Highlights
The natural logarithm, ln(x), is the inverse function to e^x.
The graph of e^x and ln(x) shows a reflection over the line y=x.
The domain of ln(x) is restricted to positive numbers only.
To find the derivative of ln(x), implicit differentiation is used.
The derivative of ln(x) is found by differentiating x = e^y with respect to x.
Applying the chain rule to e^y gives dy/dx = 1/(e^y).
Since e^y equals x, the derivative simplifies to 1/x for x > 0.
The function 1/x has a domain of all real numbers except zero, contrasting with ln(x)'s domain of positive numbers only.
Considering ln(|x|) extends the domain to include negative numbers by using a piecewise definition.
When x < 0, ln(|x|) is equivalent to ln(-x), which reflects the graph over the y-axis.
The domain of ln(|x|) is all real numbers except zero.
Deriving ln(|x|) for x < 0 involves the chain rule and the derivative of -x.
The derivative of ln(-x) is obtained by multiplying 1/x with the derivative of -x, which is -1.
The final expression for the derivative of ln(x) is 1/x, valid for all x except zero.
The domain of both ln(x) and 1/x is all real numbers except zero, which is useful for future antiderivatives.
The video provides a comprehensive understanding of the natural logarithm's properties and its derivative.
The use of absolute value allows for a broader application of the natural logarithm function.
The video demonstrates the importance of understanding the domain and range of mathematical functions.
Transcripts
Browse More Related Video
Derivatives of Logarithmic Functions - More Examples
Derivatives of Log Functions
Derivatives of Logarithmic and Exponential Functions
Calc AB & Calc BC Natural Logs Review

Derivative of x^(x^x) | Taking derivatives | Differential Calculus | Khan Academy

Unit V: Lec 1 | MIT Calculus Revisited: Single Variable Calculus
5.0 / 5 (0 votes)
Thanks for rating: