The Chain Rule for Differentiation

Starfish Maths
1 Apr 202015:42
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational episode of Starfish Maths, Sara delves into the intricacies of the chain rule, a fundamental concept for differentiating composite functions, or functions within functions. She introduces viewers to both the formal method and a quicker, intuitive approach to applying the chain rule, illustrating these techniques with clear examples that include basic functions and trigonometric expressions such as sine, cos, and tan. The session culminates in solving an exam-style question on finding the equation of a tangent to a curve, encouraging viewers to practice alongside with pen and paper for a deeper understanding. Sara's guidance is aimed at making complex mathematical concepts accessible and manageable.

Takeaways
  • 📚 The chain rule is used for differentiating composite functions, which are functions of functions.
  • 🔢 Examples are provided to illustrate the process of applying the chain rule, including both the formal and quicker methods.
  • 📱 Understanding the chain rule is crucial as it simplifies the differentiation process compared to other methods like binomial expansion.
  • 🌀 The video script introduces the concept of using a substitution variable (like 'u') to represent the inner function for clarity.
  • 📊 The chain rule is applied to various functions, including polynomials and trigonometric functions like sine, cosine, and tangent.
  • 🔄 The process of differentiating involves differentiating the outer function first, then multiplying by the derivative of the inner function.
  • 📈 The script also covers the differentiation of trigonometric functions when they are used as inner or outer functions in composite functions.
  • 🎓 The derivative of sine is cosine, the derivative of cosine is -sine, and the derivative of tangent is sec^2(x).
  • 📝 The final exam-style question demonstrates how to find the equation of a tangent to a curve at a given point by using the chain rule to find the gradient.
  • 🧠 Practice is emphasized as essential for mastering the chain rule and its application to various mathematical problems.
  • 👍 The video aims to make the process of differentiation more approachable and less intimidating for learners.
Q & A

  • What is the chain rule used for in calculus?

    -The chain rule is used for differentiating a composite function, which means differentiating a function that is the result of one function being input into another function.

  • How do you identify a composite function?

    -A composite function is identified when you have one function that is the output of another function. It is represented as an inner function and an outer function.

  • What is the purpose of using a substitution like 'u' in the chain rule?

    -Using a substitution like 'u' helps to simplify the process of differentiation by allowing us to treat the inner function as a single variable, making it easier to apply the chain rule.

  • What is the long-form method of applying the chain rule?

    -The long-form method of applying the chain rule involves writing out the expression again, replacing the inner function with a variable (like 'u'), and then differentiating both the inner and outer functions step by step.

  • Can you describe the quick method for applying the chain rule?

    -The quick method for applying the chain rule involves differentiating the outer function first and then multiplying that by the derivative of the inner function, without going through the full substitution process.

  • What are the derivatives of sine, cosine, and tangent functions when using the chain rule?

    -The derivative of the sine function is cosine, the derivative of the cosine function is negative sine, and the derivative of the tangent function is the square of the secant function.

  • How does the chain rule change when differentiating trigonometric functions that are raised to a power?

    -When differentiating trigonometric functions that are raised to a power, the entire function within the power is raised to the next power, and then the chain rule is applied as usual.

  • What is the process for finding the equation of a tangent to a curve?

    -To find the equation of a tangent to a curve, you first need the coordinates of the point of tangency and the gradient of the curve at that point. The gradient is found by differentiating the function and evaluating it at the given x-coordinate.

  • How do you apply the chain rule to differentiate a function that involves a square root?

    -When differentiating a function that involves a square root, you first differentiate the function inside the square root using the chain rule, and then simplify the expression by placing the square root back over the result.

  • What is the formula for the equation of a straight line?

    -The formula for the equation of a straight line is y = mx + b, where m is the gradient (slope) and b is the y-intercept.

  • How can you practice applying the chain rule?

    -To practice applying the chain rule, work through a variety of examples involving different types of composite functions, including those with trigonometric and power functions, until the process becomes familiar and quick.

Outlines
00:00
📚 Introduction to the Chain Rule

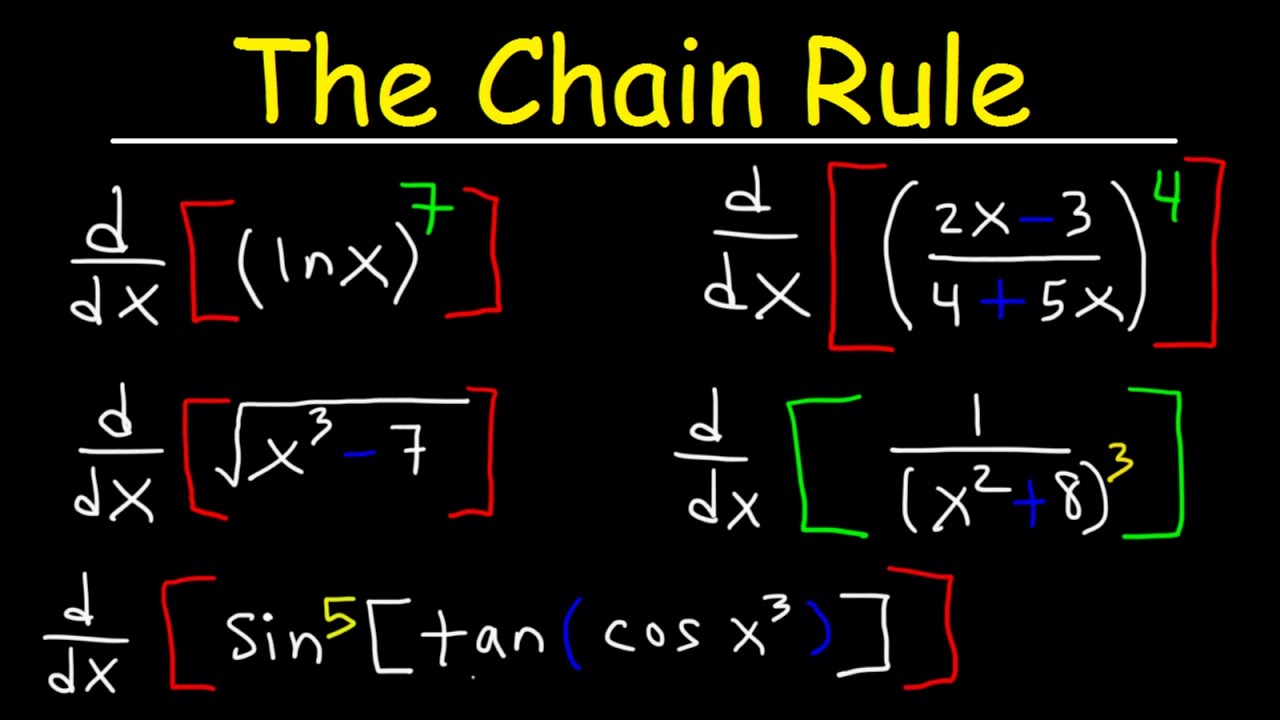
This paragraph introduces the concept of the chain rule in calculus, emphasizing its application in differentiating composite functions. The speaker, Sara, explains that the chain rule is used when one function is the input for another function. She plans to illustrate this with examples and also cover the differentiation of sine, cosine, and tangent functions, which can be tricky with the chain rule. Sara encourages viewers to follow along with pen and paper, and to pause the video as needed. The first example involves differentiating a function that is the cube of another function, with the inner function being (x + 3) and the outer function being its cube. Sara presents both a formal and a quicker method for applying the chain rule, highlighting the importance of understanding both approaches for effective differentiation.

05:02
🧠 Understanding the Chain Rule Application

In this paragraph, Sara continues to explore the application of the chain rule through additional examples. She uses the shorthand method to differentiate more complex expressions, demonstrating how to simplify the process by focusing on the outer and inner functions separately. Sara emphasizes the importance of practice in mastering the chain rule, noting that while it may seem complicated at first, with time and repetition, it becomes easier and quicker. She also touches on the differentiation of trigonometric functions such as sine, cosine, and tangent, noting that the chain rule's application differs depending on whether these functions are the inner or outer part of the composite function. Sara provides examples of these scenarios, reinforcing the concept with practical illustrations.

10:05
📈 Trigonometric Functions and Chain Rule

This paragraph delves into the specifics of using the chain rule with trigonometric functions. Sara explains the derivatives of sine, cosine, and tangent, noting the changes in sign that occur when differentiating these functions. She provides examples where the trigonometric function is either the inner or outer function within a composite function, showing how to apply the chain rule in each case. Sara emphasizes the importance of memorizing these derivatives for various qualifications and uses practical examples to solidify the understanding of the chain rule with trigonometric functions.

15:05
📊 Exam Style Question - Tangent to a Curve

The final paragraph focuses on solving an exam-style question involving the equation of a tangent to a curve. Sara outlines the process of finding the equation of a tangent, which requires determining a point on the curve and the gradient at that point. She demonstrates how to use the chain rule to differentiate the given function to find the gradient. Sara then combines the point's coordinates and the gradient to form the equation of the tangent line. She suggests that viewers can use a calculator for these computations and offers an alternative method for finding the equation of the tangent. Sara concludes the video by encouraging viewers to practice and thanking them for watching.

Mindmap
Keywords
💡Chain Rule
The Chain Rule is a fundamental concept in calculus used to differentiate composite functions, which are functions where one function is nested within another. In the context of the video, the Chain Rule is introduced as the method to differentiate complex expressions where one function is applied to the result of another. The video provides examples of how to apply the Chain Rule both formally, by setting up a substitution, and informally, by directly applying the derivative of the outer function to the derivative of the inner function.
💡Composite Function
A composite function is a function that is formed by combining two or more functions in such a way that the output of one function becomes the input of another. In the video, composite functions are the primary focus when discussing the application of the Chain Rule. The concept is central to understanding how to differentiate more complex mathematical expressions that involve one function being evaluated before another.
💡Differentiation
Differentiation is the process of finding the derivative of a function, which represents the rate of change or the slope of the function at any given point. In the video, differentiation is the main mathematical operation being discussed, with a focus on using the Chain Rule to differentiate composite functions. Differentiation is a crucial skill in calculus and is used to analyze various properties of functions, such as their tangents and rates of change.
💡Trigonometric Functions
Trigonometric functions, including sine (sin), cosine (cos), and tangent (tan), are mathematical functions that relate angles to real numbers. They are widely used in mathematics, physics, and engineering to model periodic phenomena. In the video, the Chain Rule is applied to differentiate these functions, which have unique derivatives: the derivative of sin is cos, the derivative of cos is -sin, and the derivative of tan is sec^2(x).
💡Derivative
A derivative is a concept in calculus that represents the rate at which a function changes with respect to its input variable. It gives the slope of the function at any point and can be thought of as the instantaneous rate of change. In the video, the derivative is the result of differentiating a function using the Chain Rule and other differentiation techniques.
💡Inner Function
In the context of composite functions, the inner function is the function that is being nested or is on the inside of the composition. It is the function that takes the input and produces an output which then serves as the input for the outer function. The video emphasizes the importance of identifying and differentiating the inner function when applying the Chain Rule.
💡Outer Function
The outer function in a composite function is the function that is applied to the result of the inner function. It is the function 'on the outside' of the composition. In the process of differentiating composite functions using the Chain Rule, the outer function is the part that is differentiated after the inner function's derivative has been determined.
💡Substitution
Substitution is a mathematical technique used in calculus to simplify complex expressions by replacing one or more variables with other variables or constants. In the context of the Chain Rule, substitution involves replacing the inner function with a new variable (typically 'u') to make the differentiation process easier and more manageable.
💡Examination Question
An examination question, often found in tests or assessments, is a problem or task designed to evaluate a student's understanding and application of a particular concept or skill. In the video, an exam-style question is presented to the viewer, requiring the application of the Chain Rule and other differentiation techniques to find the equation of a tangent line to a curve at a given point.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. The slope of the tangent line at any point on a curve is equal to the derivative of the function at that point. In the video, the concept of a tangent line is discussed in the context of an exam-style question, where the viewer is asked to find the equation of the tangent line to a curve at a specific x-value.
💡Slope
The slope of a line is a measure of its steepness, indicating the rate of change between the y and x values on the line. In the context of the video, the slope is a critical component in finding the equation of a tangent line to a curve, as it represents the instantaneous rate of change of the function at a specific point.
Highlights

Introduction to the chain rule for differentiating composite functions.

Explanation of the chain rule with a visual example using green and red to differentiate between the inner and outer functions.

Detailed demonstration of the formal method for applying the chain rule with a step-by-step breakdown.

Presentation of a quicker method for thinking about the chain rule without extensive writing.

Working through four examples to illustrate the chain rule, including setting up substitutions and simplifying expressions.

Explanation of the importance of practice in mastering the chain rule for efficiency and ease.

Introduction to differentiating sine, cosine, and tangent functions using the chain rule.

Clarification on the changes in signs when differentiating cosine and tangent functions.

Examples of trigonometric functions as both inner and outer functions in chain rule applications.

Demonstration of how to find the equation of a tangent to a curve using the chain rule for differentiation.

Emphasis on the practical application of the chain rule in solving exam-style questions.

Encouragement for viewers to practice the chain rule with provided examples.

Use of visual aids and color-coding to enhance understanding and retention of the chain rule concepts.

Inclusion of a comprehensive example involving the square root and power functions to demonstrate the chain rule.

The presenter's approach to breaking down complex concepts into understandable parts, making the chain rule approachable.

Transcripts

Browse More Related Video

Derivatives of Trig Functions - Calculus | MCV4U

The Chain Rule

Calculus - The chain rule for derivatives

Chain Rule For Finding Derivatives

Derivatives of Composite Functions - Chain Rule, Product & Quotient Rule

The Chain Rule - Part 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusChain RuleComposite FunctionsTrigonometrySine CosineTangent FunctionDerivativesMathematics EducationTutorial VideoExam Preparation