Finding derivative with fundamental theorem of calculus: x is on both bounds | Khan Academy

Khan Academy
8 Feb 201305:04
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript discusses the process of finding the derivative of a function using the Fundamental Theorem of Calculus. It highlights the challenge of dealing with a definite integral where both the upper and lower bounds contain the variable x. The solution involves breaking the integral into two parts using a constant c between x and x squared, applying the theorem to each part, and then combining the results to find the derivative. The final answer is expressed as a simplified expression involving the cosine function and the variable x.

Takeaways
  • 📚 The goal is to find the derivative of a given expression, specifically capital F prime of x.
  • 🔍 The fundamental theorem of calculus is mentioned as a potential tool to achieve this, but its usual application involves x only on the upper boundary.
  • 📈 The challenge is to rearrange the expression to fit the familiar form used when applying the fundamental theorem of calculus.
  • 🎨 Visualization through graphing helps to understand the problem better, representing the area under the curve as the definite integral.
  • 🔄 By introducing a constant c between x and x squared, the area under the curve can be split into two integrals, simplifying the problem.
  • 🌟 The fundamental theorem of calculus can then be applied to each of the two integrals, with the derivative operator yielding negative cosine x over x for the first part.
  • 📐 The second part involves taking the derivative of x squared with respect to x, which is 2x, and applying it to the integral.
  • 🔢 The final result is a simplified expression combining the two parts: negative cosine of x over x plus 2 cosine of x squared over x.
  • 💡 The process demonstrates the power of breaking complex problems into simpler, manageable parts and the application of calculus theorems.
  • 📝 The script emphasizes the importance of understanding the structure of the problem and the rules of calculus to solve it correctly.
  • 🎓 The explanation serves as a lesson in calculus, showcasing the step-by-step approach to tackling problems involving derivatives and integrals.
Q & A

  • What is the main objective of the expression discussed in the transcript?

    -The main objective is to find the derivative, capital F prime of x, for a given expression using calculus techniques.

  • What theorem is suggested for use in solving the expression?

    -The fundamental theorem of calculus is suggested for use, specifically for taking the derivative of a definite integral that results in a function of x.

  • Why is the presence of x on both the upper and lower boundary a challenge for applying the fundamental theorem?

    -The fundamental theorem of calculus, as typically presented, is applied when x is only on the upper boundary. Having x on both boundaries complicates the direct application of the theorem.

  • How does the speaker propose to overcome the challenge with the boundaries?

    -The speaker suggests introducing a constant c between x and x squared, breaking the area into two different areas which can then be represented as separate integrals, allowing for the application of the fundamental theorem.

  • What is the significance of graphing the function f(t) over the interval between x and x squared?

    -Graphing the function helps in visualizing the area under the curve that the definite integral represents, which is crucial for understanding how to break down the problem and apply calculus theorems.

  • How does the process of breaking the integral into two parts affect the application of the fundamental theorem?

    -By breaking the integral into two parts with a constant c as the lower bound for one and the original lower bound x for the other, the expression can be rewritten in a form that fits the standard application of the fundamental theorem, with x as the upper bound.

  • What is the result of applying the derivative operator to the rewritten expression?

    -Applying the derivative operator results in the expression: negative cosine x over x plus 2 cosine of x squared over x, which simplifies to 2 cosine of x squared minus cosine of x, all over x.

  • How does the chain rule come into play in this problem?

    -The chain rule is used when taking the derivative of the second part of the expression with respect to x squared, where t is replaced with x squared, and the derivative of x squared with respect to x is considered.

  • What is the final simplified form of the derivative of the given expression?

    -The final simplified form of the derivative is (2 cosine of x squared - cosine of x) / x.

  • What is the significance of the negative sign in the derivative result?

    -The negative sign comes from the fact that when applying the fundamental theorem to the rewritten expression, a negative sign is introduced due to the order of the bounds and the nature of the cosine function.

  • How does this problem illustrate the flexibility of calculus in handling complex expressions?

    -This problem demonstrates that by introducing constants and breaking down expressions, calculus can be applied to a wider range of scenarios, including those that don't immediately fit standard theorem applications, showcasing its adaptability and problem-solving power.

Outlines
00:00
🤔 Understanding the Derivative of an Integral with Variable Limits

The video script begins by addressing the challenge of taking the derivative of an integral with variable limits, specifically when both the upper and lower limits are functions of x. It highlights a common misconception that the fundamental theorem of calculus applies only when the variable x appears in the upper limit. The narrator then introduces a graphical representation of the function f(t) over the interval between x and x^2 to visualize the problem. By introducing a constant c within this interval, the integral is divided into two parts, allowing for a breakdown that fits the application of the fundamental theorem of calculus. The solution involves applying the chain rule, reversing the limits of integration with a sign change, and ultimately simplifying the expression to find the derivative of the original function. The explanation culminates in a simplified formula for the derivative, showcasing the integration of calculus concepts to solve complex problems.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function at a particular point. In the context of this video, the derivative is used to find the rate of change of a definite integral with respect to the variable x, which is crucial for understanding the behavior of the function and its applications in various problems. The script discusses finding the derivative of an expression, specifically capital F prime of x, as part of the process of applying the fundamental theorem of calculus.
💡fundamental theorem of calculus
The fundamental theorem of calculus is a core theorem that links integration and differentiation, two of the primary operations in calculus. It states that a function is the integral of its derivative over a certain interval if and only if it is the derivative of some function over that same interval. In the video, the theorem is mentioned as a tool to help find the derivative of a definite integral with respect to x, which involves understanding how the theorem applies when the variable x appears in both the upper and lower bounds of the integral.
💡chain rule
The chain rule is a fundamental principle in calculus that is used to find the derivative of composite functions. It states that the derivative of a composite function is the derivative of the outer function multiplied by the derivative of the inner function. In the video, the chain rule is referenced as a method that could potentially be used to break down the given expression into a form that allows for the application of the fundamental theorem of calculus, especially when dealing with expressions involving functions of functions.
💡definite integral
A definite integral represents the signed area under a curve within a specified interval. It is a fundamental concept in calculus used to calculate quantities such as the total distance traveled by a moving object, electrical charge, and other quantities that can be accumulated over a period of time or along a space. In the video, the focus is on taking the derivative of a definite integral, which involves understanding how to break down the integral into simpler parts and how the variable x affects the area under the curve.
💡constant
In mathematics, a constant is a value that does not change. In the context of the video, a constant (denoted as 'c') is introduced as a fixed point between 'x' and 'x squared' to help break the definite integral into two separate parts. This division allows for the application of the fundamental theorem of calculus in a more straightforward manner, as it transforms the problem into a form that is more familiar and easier to handle.
💡area under the curve
The area under the curve of a graph is a measure of the quantity represented by the integral of a function over a certain interval. In the video, the area under the curve is the main focus, as the problem involves finding the derivative of this area with respect to 'x'. The process of breaking down the area into two parts and then applying the fundamental theorem of calculus is central to solving the problem.
💡variable 'x'
In the context of this video, 'x' is the variable with respect to which the derivative is being taken. It appears both as the upper and lower bound of the definite integral, which complicates the direct application of the fundamental theorem of calculus. The video discusses how to manipulate the expression to make 'x' the upper bound only, which simplifies the process of finding the derivative.
💡negative cosine
The term 'negative cosine' refers to the function that is the negative of the cosine function. In the video, it is part of the final expression obtained after applying the fundamental theorem of calculus and taking the derivative of the definite integral. The negative cosine term is a result of the process of differentiating the integral with respect to 'x' and is part of the final solution to the problem.
💡cosine of x squared
The phrase 'cosine of x squared' refers to the cosine function evaluated at 'x squared'. In the video, this term appears in the final expression after the derivative of the definite integral is calculated. It is a result of replacing 't' with 'x squared' in the function being integrated, as part of the process of applying the fundamental theorem of calculus.
💡derivative of x squared with respect to x
The derivative of 'x squared' with respect to 'x' is a basic calculus operation that results in '2x'. This term is used in the video when discussing the second part of the final expression, which involves the derivative of 'x squared' with respect to 'x'. Understanding this derivative is crucial for completing the process of finding the derivative of the definite integral.
💡simplify
The process of simplification in mathematics involves reducing complex expressions to their simplest form. In the video, simplification is an essential step in obtaining the final expression after applying the fundamental theorem of calculus and taking the derivative of the definite integral. The goal is to make the expression as straightforward and easy to understand as possible, which is crucial for further analysis or application.
Highlights

Introduction to taking the derivative of a definite integral with both upper and lower bounds as functions of x.

Identification of a potential application of the fundamental theorem of calculus despite variable bounds.

Recognition that typical applications of the fundamental theorem involve only the upper boundary varying with x.

Introduction of the chain rule to handle the square of x in the boundary.

Proposal to graph the function within the variable bounds to better understand the integral's representation.

Graphical representation of the function f(t) over the interval between x and x squared.

Breaking the area under the curve into two parts using a constant, to simplify the expression.

Expression of the total area as the sum of two separate integrals over the intervals [x, c] and [c, x^2].

Rearrangement of the integral bounds to fit the conventional form used with the fundamental theorem of calculus.

Application of the fundamental theorem and the chain rule to derive F prime of x.

Derivation of the final expression involving cosine functions and simplification of the result.

Highlighting the importance of understanding and manipulating bounds in integrals for differentiation.

Illustration of the power of graphical interpretation in solving complex calculus problems.

Emphasis on the versatility of the fundamental theorem of calculus when applied with creativity.

Final expression simplification showcasing the subtractive relationship between the integral's bounds.

Transcripts

Browse More Related Video

Derivatives of Integrals (w/ Chain Rule)

Finding derivative with fundamental theorem of calculus: x is on lower bound | Khan Academy

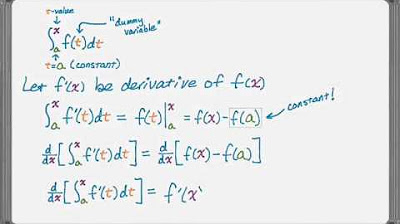
Second Fundamental Theorem of Calculus 2nd FTC

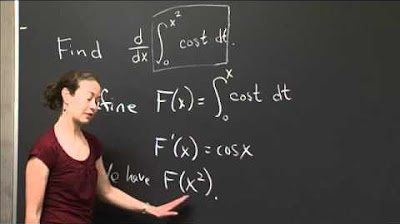
Second fundamental theorem and chain rule | MIT 18.01SC Single Variable Calculus, Fall 2010

Worked example: Finding derivative with fundamental theorem of calculus | Khan Academy

Calculus (Version #2) - 9.1 The Second Fundamental Theorem of Calculus

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus ConceptsDerivative ApplicationsIntegral AnalysisTheoretical MathEducational ContentAdvanced Math TechniquesFunction VisualizationMathematical SimplificationOnline LearningMathematics Tutorial