Finding derivative with fundamental theorem of calculus: x is on lower bound | Khan Academy

Khan Academy
8 Feb 201303:00
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of finding the derivative of a definite integral with respect to x, where x serves as the lower bound of integration. It highlights the importance of understanding how switching bounds affects the integral and demonstrates how to apply the fundamental theorem of calculus by changing the bounds and taking the negative of the integral to find the derivative. The final result is expressed as the negative of the square root of the absolute value of the cosine function evaluated at x.

Takeaways
  • 📚 The discussion is about finding the derivative of a function related to a definite integral with respect to x.
  • 🤔 The confusion arises from the usual application of the fundamental theorem of calculus with x as the upper bound, not the lower bound.
  • 🔄 The key realization is understanding what happens when you switch the bounds of integration in a definite integral.
  • 📈 The fundamental theorem of calculus, in its corollary form, states that the definite integral from a to b of f(t) dt is F(b) - F(a), where F is the antiderivative of f.
  • ➖ When the definite integral is negated, it becomes the negative of F(b) - F(a), which is equivalent to F(a) - F(b, meaning the bounds are switched.
  • 🔄 Switching bounds in a definite integral is akin to changing their signs; they become negatives of each other.
  • 🎯 The problem can be reformulated by switching the bounds and taking the negative of the same definite integral, allowing the fundamental theorem to be applied directly.
  • 📝 The derivative with respect to x of the integral expression becomes the negative of the integral with switched bounds, leading to the final answer.
  • 🌟 The final result is expressed as the negative of the square root of the absolute value of the cosine function, but with x substituted for t.
  • 💡 The lesson emphasizes the importance of understanding how to manipulate bounds and apply the fundamental theorem of calculus in various contexts.
Q & A

  • What is the main concept being discussed in the transcript?

    -The main concept discussed in the transcript is finding the derivative with respect to x of a definite integral where x is the lower bound of integration.

  • Why might the application of the fundamental theorem of calculus seem challenging in this context?

    -It seems challenging because the fundamental theorem of calculus is typically applied with x as the upper bound of integration, not the lower bound as in this case.

  • How does the speaker suggest dealing with the issue of x being the lower bound of integration?

    -The speaker suggests dealing with this issue by understanding what happens when you switch the bounds of a definite integral, which is akin to changing the sign of the integral.

  • What is the fundamental theorem of calculus, and how does it relate to the problem at hand?

    -The fundamental theorem of calculus states that the derivative of a definite integral with respect to a variable is equal to the antiderivative of the integrand evaluated at the upper bound minus the antiderivative evaluated at the lower bound. It relates to the problem as it provides a method to find the derivative of the integral in question.

  • How does the speaker use the concept of switching bounds to solve the problem?

    -The speaker uses the concept of switching bounds by rewriting the integral with x as the upper bound and 3 as the lower bound, and then taking the negative of the integral to apply the fundamental theorem of calculus.

  • What is the final result of differentiating the given integral with respect to x?

    -The final result of differentiating the integral with respect to x is the negative of the square root of the absolute value of cosine of x.

  • How does the negative sign affect the interpretation of the integral?

    -The negative sign effectively switches the order of the bounds of integration, which means the integral is evaluated from the upper bound to the lower bound rather than the conventional lower to upper bound.

  • What is the significance of the absolute value in the integral?

    -The absolute value ensures that the value of the cosine function is always non-negative, regardless of the value of t, which is important when taking the square root of the expression.

  • How does the fundamental theorem of calculus part two relate to the problem?

    -The fundamental theorem of calculus part two, also known as the second fundamental theorem of calculus, allows us to evaluate definite integrals by taking the antiderivative of the integrand evaluated at the bounds of integration. This is crucial for understanding how to differentiate the integral with respect to x.

  • What is the role of the antiderivative in the process of differentiating a definite integral?

    -The antiderivative plays a key role in the process as it is used to evaluate the definite integral. When differentiating the integral with respect to x, the antiderivative of the integrand at the bounds of integration is what contributes to the final result.

  • What is the significance of the square root in the integral?

    -The square root is part of the integrand and is carried through the differentiation process. It indicates that the final result will involve taking the square root of the absolute value of the cosine function evaluated at x.

Outlines
00:00
📚 Understanding Definite Integrals and Derivatives

This paragraph discusses the process of finding the derivative with respect to x for a given function. It highlights the importance of recognizing the role of x as a boundary of integration and addresses the common confusion when x serves as the lower bound rather than the upper bound in the context of the fundamental theorem of calculus. The explanation delves into the concept of switching bounds for a definite integral and how it affects the evaluation of the integral. The paragraph clarifies that the negative of a definite integral from a to b is equivalent to the integral from b to a, with the bounds switched, which is a crucial insight for solving the problem at hand. The solution involves rewriting the integral with switched bounds and applying the fundamental theorem of calculus to find the derivative, ultimately leading to the conclusion that the derivative is the negative square root of the absolute value of the cosine function evaluated at x.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, the derivative is being sought for a particular function defined by a definite integral. The process of finding the derivative involves applying the rules of calculus, particularly focusing on how the function changes as its input variable 'x' changes.
💡integral
An integral in calculus represents the accumulation of a quantity, often understood as the area under a curve. The video discusses a definite integral, which is a specific type of integral that calculates the area between a function and the x-axis over a defined interval. The integral is a key component in the problem being solved, as the derivative of the integral is being sought.
💡fundamental theorem of calculus
The fundamental theorem of calculus is a core result that connects the processes of differentiation and integration. It states that if a function is continuous over an interval, then the integral of its derivative over that interval is the function itself, up to a constant. In the video, this theorem is crucial for finding the derivative of the given integral, as it allows the transformation of the problem into a form that can be solved using the rules of calculus.
💡boundaries of integration
The boundaries of integration are the limits that define the interval over which an integral is calculated. In the video, the boundaries are crucial because they determine the portion of the function that contributes to the integral's value. The video's problem involves switching these boundaries, which affects how the derivative of the integral is computed.
💡antiderivative
An antiderivative, also known as an indefinite integral, is a function whose derivative is the given function. In the context of the video, the antiderivative is used to evaluate the definite integral, as the integral of a function is fundamentally the difference in the values of its antiderivative at the bounds of integration.
💡switching bounds
Switching bounds refers to the process of changing the order of the limits of integration. In the video, this concept is used to transform the problem of finding the derivative with respect to 'x' when 'x' is the lower bound of integration. By switching the bounds, the problem becomes one where 'x' is the upper bound, which simplifies the application of the fundamental theorem of calculus.
💡negative
The concept of a negative in the context of the video relates to the manipulation of integrals. When the negative sign is introduced, it effectively switches the order of the bounds of integration, as the negative of an integral from 'a' to 'b' is equal to the integral from 'b' to 'a'. This is crucial for the problem at hand, as it allows the application of the fundamental theorem of calculus in a more straightforward manner.
💡cosine function
The cosine function is a trigonometric function that describes periodic changes in a right-angled triangle or periodic waves. In the video, the cosine function appears within the integrand (the function being integrated) as part of the absolute value. The absolute value of the cosine is being integrated, which means the function's contribution to the area under the curve is always non-negative, regardless of the value of 't'.
💡absolute value
The absolute value of a number is its distance from zero on the number line, regardless of direction. In the video, the absolute value is applied to the cosine function to ensure that the integrand is non-negative, as the area under the curve is being calculated. The absolute value is integrated with respect to 't', and the resulting function after applying the fundamental theorem of calculus will involve the absolute value of the cosine of 'x', reflecting the transformation of the variable from 't' to 'x'.
💡root
A root, specifically the square root in this context, is a mathematical operation that undoes the squaring operation. In the video, the square root appears as part of the integrand, modifying the absolute value of the cosine function. The square root is applied to the absolute value of the cosine, and this expression is integrated with respect to 't'. After the integration and application of the fundamental theorem of calculus, the root operation remains, now applied to the absolute value of the cosine of 'x'.
💡evaluation
Evaluation in the context of the video refers to the process of determining the value of an expression or function. The video discusses the evaluation of definite integrals and their derivatives. The evaluation of the definite integral is key to finding the derivative of the function, as the fundamental theorem of calculus is used to transform the integral into a function of 'x'.
Highlights

The discussion revolves around finding the derivative with respect to x of a given function.

The function in question is a definite integral with x being one of the boundaries.

The fundamental theorem of calculus is mentioned as a potential tool to solve the problem.

A common confusion is addressed regarding the placement of x as the upper or lower bound of integration.

The key realization involves understanding the effect of switching bounds for a definite integral.

A review of the fundamental theorem of calculus is provided, explaining the evaluation of definite integrals.

The concept of negating a definite integral is explored, leading to a change in the boundaries.

The equivalence of negating the integral to switching the boundaries is established.

The original problem is reformulated to match the new understanding of switched boundaries.

The application of the fundamental theorem of calculus is now directly applicable to the reformulated problem.

The derivative with respect to x is expressed as the negative of the same integral with switched boundaries.

The final result is presented as the negative of a function involving the square root of the absolute value of cosine, now with respect to x.

The process demonstrates a method for dealing with the fundamental theorem of calculus when the bounds of integration are not in the expected order.

The explanation serves as a tutorial on the manipulation of definite integrals and their derivatives.

The transcript provides a clear and detailed walkthrough of the calculus concepts involved.

The problem-solving approach showcased is valuable for understanding the nuances of calculus applications.

The session concludes with a succinct summary of the solution, reinforcing the key takeaways.

Transcripts

Browse More Related Video

Finding derivative with fundamental theorem of calculus: x is on both bounds | Khan Academy

Integration by parts: definite integrals | AP Calculus BC | Khan Academy

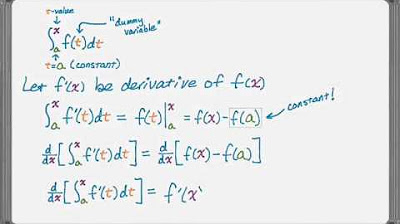
Second Fundamental Theorem of Calculus 2nd FTC

Definite integral of trig function | AP Calculus AB | Khan Academy

Derivatives of Integrals (w/ Chain Rule)

Definite integral of radical function | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus ConceptsDerivative ProblemsIntegration TechniquesBounds SwitchingMathematical AnalysisTheoretical ApproachEducational ContentAdvanced MathOnline TutorialMathematics Education