Chain Rule For Finding Derivatives
TLDRThis transcript delves into the concept of the chain rule in calculus, emphasizing its importance in differentiating composite functions. The chain rule is methodically explained with various examples, such as power functions, trigonometric functions, and complex expressions involving multiple composite functions. The process involves differentiating the outermost function, keeping the inner functions constant, and multiplying by the derivative of the inner function. The examples provided illustrate the application of the chain rule in diverse scenarios, highlighting its utility in simplifying and solving calculus problems.
Takeaways
- 📚 The chain rule is essential for differentiating composite functions, expressed as f(g(x)).
- 🔢 To apply the chain rule, differentiate the outer function with respect to x, keeping the inner function g(x) unchanged, and then multiply by the derivative of g(x).
- 💡 The general formula for the derivative of a power function using the chain rule is (n * u) * (u')^(n-1), where u is a function of x and n is the exponent.
- 🌟 When differentiating a constant raised to a power, separate the constant from the exponent and apply the chain rule.
- 📈 For example, the derivative of (5x + 3)^4 involves bringing down the exponent, applying the power rule, and multiplying by the derivative of (5x + 3).
- 🌐 The chain rule can be applied to a wide range of functions, including trigonometric functions like sine, cosine, and tangent.
- 🔍 When working with nested functions, differentiate the outermost function first, then proceed inward, differentiating each layer.
- 📊 For complex derivatives involving multiple composite functions, work from the outside to the inside, applying the chain rule at each step.
- 🧠 The process of differentiation can be simplified by recognizing patterns and applying the chain rule systematically.
- 📝 When differentiating complex expressions, sometimes it's necessary to rewrite or simplify the expression before applying the chain rule.
- 🔑 The quotient rule, which is (f'/g) - (f * g')/g^2, can be used in conjunction with the chain rule when differentiating a quotient of functions.
Q & A
What is the chain rule in calculus?
-The chain rule is a fundamental rule in calculus used to find the derivative of a composite function. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function.
How do you apply the chain rule to a function like f(g(x))?
-To apply the chain rule to a function like f(g(x)), you first differentiate the outer function f with respect to g, and then multiply that by the derivative of the inner function g with respect to x.
What is the general power rule formula combined with the chain rule?
-The general power rule formula combined with the chain rule is expressed as (d/dx)[u(x)^n] = n * u(x)^(n-1) * du/dx, where u(x) is the inner function and n is the exponent.
What is the derivative of (5x + 3)^4 using the chain rule?
-The derivative of (5x + 3)^4 using the chain rule is 4 * (5x + 3)^3 * (5), which simplifies to 20 * (5x + 3)^3.
How do you find the derivative of a function like x^2 - 3x raised to a power?
-To find the derivative of a function like x^2 - 3x raised to a power, you first bring down the exponent, then keep the inside function the same and subtract the exponent by 1. After that, you multiply by the derivative of the inside function.
What is the derivative of sin(6x)?
-The derivative of sin(6x) is 6 * cos(6x), as you use the chain rule to differentiate the sine function and keep the inside function (6x) the same.
How do you find the derivative of a function that involves a trigonometric function inside another trigonometric function?
-To find the derivative of a function with a trigonometric function inside another, you differentiate the outer function and then apply the chain rule to the inner function, continuing this process until you reach the innermost function.
What is the derivative of tan(x^3)?
-The derivative of tan(x^3) is 3x^2 * sec^2(x^3), which is found by differentiating the tangent function and then applying the chain rule to the inner function x^3 and its derivative, which is 3x^2.
How do you find the derivative of a complex composite function like (sin(tan(cos(x^3))))?
-To find the derivative of a complex composite function like (sin(tan(cos(x^3)))), you start by differentiating the outermost function (sin), then apply the chain rule to the next inner function (tan), and continue differentiating and applying the chain rule until you reach the innermost function (cos(x^3)).
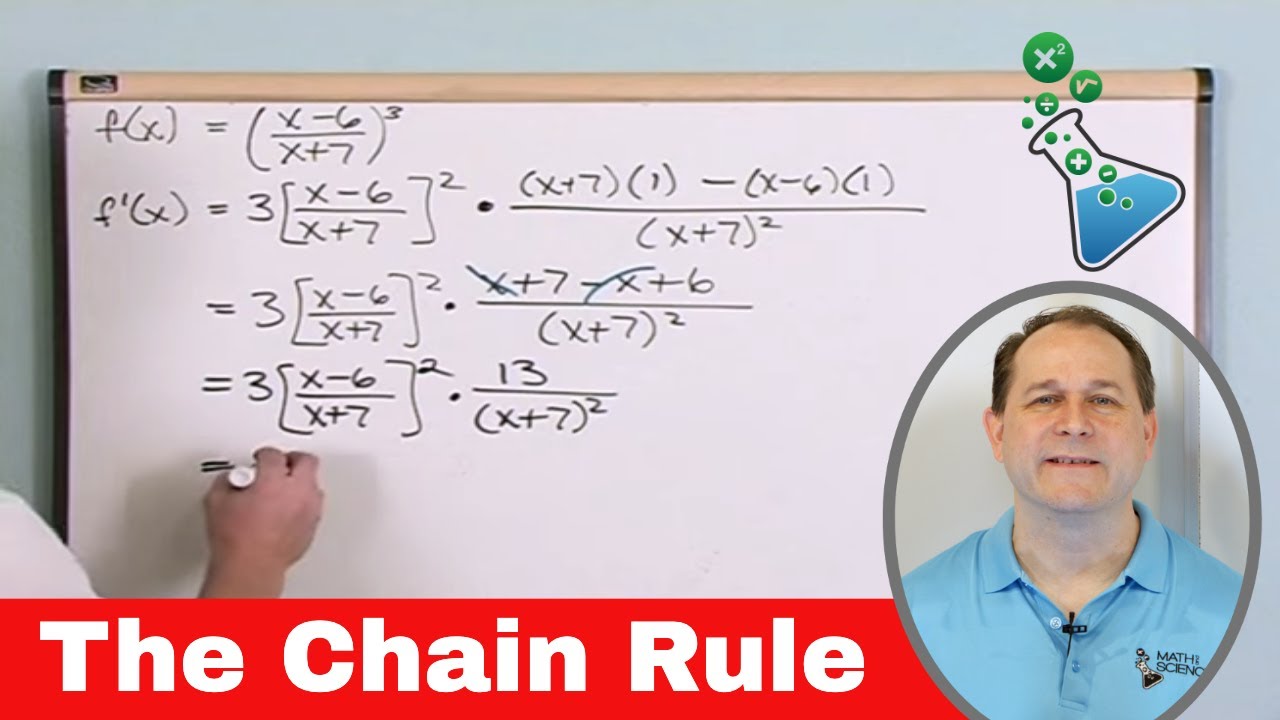
What is the derivative of a function involving a quotient, like (2x - 3) / (4 + 5x)^4?
-The derivative of a function involving a quotient, like (2x - 3) / (4 + 5x)^4, is found using the quotient rule. You first differentiate the numerator and the denominator separately, and then apply the formula (g * f' - f * g') / g^2, where f is the numerator and g is the denominator.
What is the product rule and how does it relate to the chain rule?
-The product rule is a calculus formula used to find the derivative of a product of two functions. It states that the derivative of f(x)g(x) is f(x)g'(x) + f'(x)g(x). The product rule is related to the chain rule in that both involve differentiating a function in terms of its components, but the product rule applies to the multiplication of functions, while the chain rule applies to the composition of functions.
Outlines
📚 Introduction to the Chain Rule
This paragraph introduces the concept of the chain rule in calculus, emphasizing its importance in differentiating composite functions. It explains that a composite function is one where a function is nested inside another, and the process involves differentiating the outer function while treating the inner function as a constant. The paragraph provides a step-by-step guide on how to apply the chain rule, using the power rule as an example. It also includes a practical example of finding the derivative of (5x + 3)^4, demonstrating the process of simplifying the expression using the chain rule.
🔢 Derivatives of Composite Functions with Various Examples
This paragraph delves into more complex examples of using the chain rule to find derivatives of composite functions. It covers a range of mathematical functions, including trigonometric and logarithmic functions, and explains how to differentiate them by applying the chain rule. The paragraph provides detailed examples, such as finding the derivative of x^2 - 3x raised to the fifth power, the derivative of sin(6x), and the derivative of more nested functions like secant(4x). The explanation is clear and methodical, guiding the learner through each step of the differentiation process.
🌟 Advanced Chain Rule Applications
This paragraph presents more advanced applications of the chain rule, focusing on cases where multiple functions are nested within each other. It explains how to work from the outside function towards the inside, differentiating each part and multiplying the results accordingly. The paragraph includes complex examples, such as finding the derivative of (cos(x^3))^7 * sin(sec(x^2)), and demonstrates the process of simplifying these expressions. It also touches on the product rule and how it can be used in conjunction with the chain rule, as seen in the example of x^3 * (4x + 5)^4.
📈 Solving Quotient Rule and Chain Rule Problems
The final paragraph tackles the quotient rule and its application in conjunction with the chain rule. It explains how to handle expressions where the numerator and denominator are both functions, and how to simplify the resulting derivatives. The paragraph provides a comprehensive example involving the derivative of (2x - 3) / (4 + 5x)^4, walking the learner through the process of applying both the quotient and chain rules. The explanation is detailed, ensuring a clear understanding of the differentiation process for more complex mathematical expressions.
Mindmap
Keywords
💡Chain Rule
💡Composite Function
💡Derivative
💡Power Rule
💡Differentiation
💡Exponent
💡Constant
💡Trigonometric Functions
💡Product Rule
💡Quotient Rule
Highlights
Introduction to the chain rule for derivatives.
Derivative of a composite function, f(g(x)).
Differentiate the outside function and multiply by the derivative of the inside function.
General power rule formula combined with the chain rule.
Example: Derivative of (5x + 3)^4 using the chain rule.
Derivative of x^2 - 3x raised to the fifth power.
Derivative of the sine function involving the chain rule.
Derivative of cosine(x^2) using the chain rule and power rule.
Derivative of the tangent function with a composite inside function.
Derivative of secant(4x) involving the chain rule.
Derivative of ln(x^7) using the power rule and chain rule.
Derivative of the square root of (x^3 - 7) using the chain rule.
Derivative of a complex expression involving multiple composite functions.
Derivative of a trigonometric function inside another trigonometric function.
Derivative of a compound trigonometric expression with multiple layers.
Example of a product rule and chain rule combined to find a derivative.
Derivative of a quotient involving a composite function inside.
Solving a complex derivative problem using the quotient and chain rules.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: