Reverse power rule | AP Calculus AB | Khan Academy

Khan Academy
25 Jan 201305:48
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of finding the antiderivative, or the reverse of the power rule, for a given function. It emphasizes that the rule applies to any exponent 'n' except negative one, to avoid division by zero. The script provides a step-by-step explanation of how to increase the exponent by one and divide by the new exponent to find the antiderivative. It also demonstrates this concept with examples, such as finding the antiderivative of x to the fifth power and 5 times x to the negative second power, highlighting the flexibility and power of this mathematical technique.

Takeaways
  • 📚 The problem involves finding the derivative and antiderivative of a function of the form x^(n+1)/(n+1) + 1 + c, with the condition that n ≠ -1 to avoid division by zero.
  • 🔄 The derivative of the given function is found using the power rule, which results in x^n, a general result applicable for any n ≠ -1.
  • 🔄 The antiderivative (or indefinite integral) of x^n is derived from understanding the reverse of the power rule, encapsulating a constant c to account for all possible antiderivatives.
  • 📈 The antiderivative of x^n is expressed as x^(n+1)/(n+1), showcasing the reverse power rule in action.
  • 🚫 The condition n ≠ -1 is emphasized as critical to avoid an undefined expression due to division by zero.
  • 🌟 The concept of the antiderivative is introduced as a powerful tool, applicable for any n as long as the condition is met, and it is noted that it represents a family of functions including a constant of integration.
  • 🧩 The script provides examples to illustrate the application of the reverse power rule, such as finding the antiderivative of x^5 and 5x^(-2)dx.
  • 📝 The example of x^5's antiderivative results in x^6/6 + c, demonstrating the increment of the exponent by 1 and division by the new exponent.
  • 📝 For the antiderivative of 5x^(-2)dx, the process involves multiplying the scalar (5) by the antiderivative of x^(-2), resulting in -5x^(-1) + c.
  • 🔧 The script clarifies that scalar multiplication can be applied to the antiderivative in a straightforward manner, as seen with the 5x^(-2)dx example.
  • 🔄 The verification process of antiderivatives is mentioned, where taking the derivative of the result should yield the original function.
Q & A

  • What is the main topic discussed in the transcript?

    -The main topic discussed in the transcript is the process of taking the derivative and antiderivative of a function, specifically focusing on the power rule and its reverse application.

  • Why is it assumed that n does not equal negative 1?

    -It is assumed that n does not equal negative 1 because if n were equal to negative 1, the expression would involve division by zero, which is undefined.

  • What is the power rule mentioned in the transcript?

    -The power rule mentioned in the transcript is a fundamental rule in calculus that states if you have a function of the form x^n, where n is any real number, the derivative of the function with respect to x is n*x^(n-1).

  • What is the expression for the derivative of x to the (n+1)th power over n plus 1?

    -The expression for the derivative is (n+1)*x^(n)/(n+1) + 0, which simplifies to x^n since the division by (n+1) cancels out.

  • What does the antiderivative of x to the n power represent?

    -The antiderivative of x to the n power represents the reverse process of taking the derivative, often referred to as the indefinite integral in calculus.

  • How is the antiderivative of x to the n power expressed in general terms?

    -The antiderivative of x to the n power is expressed in general terms as (x^(n+1))/(n+1) + C, where C is the constant of integration.

  • What is the antiderivative of x to the fifth power?

    -The antiderivative of x to the fifth power is x^(5+1)/(5+1) + C, which simplifies to x^6/6 + C.

  • How is the antiderivative of 5 times x to the negative 2 power dx evaluated?

    -The antiderivative of 5 times x to the negative 2 power dx is evaluated as 5*(x^(-2+1))/(-2+1) + C, which simplifies to -5x^(-1) + C or -5/x + C.

  • What is the significance of the constant term C in the antiderivative expression?

    -The constant term C in the antiderivative expression represents the family of functions that are antiderivative to the given function. It accounts for the arbitrary constant that is part of the indefinite integral.

  • How can the reverse power rule be verified?

    -The reverse power rule can be verified by taking the derivative of the antiderivative expression and checking if it yields the original function. For example, taking the derivative of x^6/6 + C results in x^5, confirming the rule's validity.

  • What is the importance of understanding the relationship between derivatives and antiderivatives?

    -Understanding the relationship between derivatives and antiderivatives is crucial in calculus as it forms the basis for many calculations, including solving integrals and analyzing the behavior of functions. It also helps in understanding the fundamental theorem of calculus, which connects differentiation and integration.

Outlines
00:00
📚 Understanding the Derivative and Antiderivative of x^n

This paragraph delves into the process of finding the derivative of a function, specifically focusing on the expression x^(n+1)/(n+1) + constant c, with the stipulation that n cannot be equal to -1 to avoid division by zero. The explanation uses the power rule to derive the function and emphasizes the generality of the rule by allowing any value for n except -1. The paragraph also introduces the concept of the antiderivative, or the reverse process of differentiation, and demonstrates how to apply the power rule in reverse to find the antiderivative of x^n. The explanation includes examples to illustrate the process and emphasizes the importance of the rule for solving definite integrals.

05:05
🔢 Applying the Antiderivative Rule with Specific Examples

The second paragraph continues the discussion on the antiderivative by providing specific examples of its application. It begins by calculating the antiderivative of x^5, illustrating the process of incrementing the exponent by 1 and dividing by the new exponent. The paragraph then moves on to a more complex example involving a scalar multiple (5 times x^(-2)) and explains how scalars interact with the antiderivative process. The explanation simplifies the expression and confirms the result by differentiating it to obtain the original function. The paragraph concludes by reinforcing the verification process for the antiderivative, encouraging the user to apply the derivative to the results and ensure they match the original function.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, the derivative is used to find the slope of the tangent line to a curve at a given point, which is essential for understanding how the function behaves locally. The script discusses taking the derivative of a function, x to the power of (n+1) divided by (n+1), plus a constant, and highlights that the derivative simplifies to x to the power of n, showcasing the reverse power rule.
💡power rule
The power rule is a basic rule in calculus that allows for the differentiation of functions that are raised to a power. According to the rule, the derivative of x to the n, denoted as x^n, is n*x^(n-1). In the video, the power rule is applied to find the derivative of a function, and it is also used to derive the antiderivative, which is the reverse process of finding the original function from its derivative. The script emphasizes the use of the power rule to simplify the expression and find the derivative of the given function.
💡antiderivative
An antiderivative, also known as an indefinite integral, is a function that represents the reverse process of differentiation. It is used to find the original function given its derivative. In the video, the antiderivative of x to the power of n is discussed, and it is shown that the antiderivative is x to the power of (n+1) divided by (n+1) plus a constant. This concept is crucial for understanding the relationship between differentiation and integration, which are the two fundamental operations in calculus.
💡constant
In the context of the video, a constant refers to a term that does not change with respect to the variable being differentiated or integrated. The derivative of a constant with respect to x is always zero, as it does not change when x changes. The antiderivative, on the other hand, includes an arbitrary constant, denoted as 'c', which accounts for the infinite number of functions that could have the same derivative. The presence of the constant 'c' in the antiderivative is a reflection of the fact that differentiation and integration are inverse operations, and integration is not a one-to-one process.
💡n plus 1
The phrase 'n plus 1' is used throughout the script to denote the exponent in the expressions being differentiated and integrated. It is a variable representation that helps in generalizing the process of finding derivatives and antiderivatives. The script emphasizes that the value of 'n' cannot be negative 1, as it would lead to division by zero, which is undefined. The term 'n plus 1' is crucial in applying the power rule and understanding the relationship between the exponents in the derivative and antiderivative.
💡x to the n
The term 'x to the n' represents the function where 'x' is raised to the power of 'n'. In the video, this term is central to the discussion of derivatives and antiderivatives. The derivative of this function with respect to 'x' is 'n times x to the power of (n-1)', and the antiderivative is 'x to the power of (n+1) divided by (n+1) plus a constant'. The script uses this term to illustrate the power rule and the process of finding antiderivatives, emphasizing the relationship between the exponents in the differentiation and integration processes.
💡arbitrary constant
An arbitrary constant, denoted as 'c' in the script, is a term that is added to an antiderivative to account for the infinite number of functions that can have the same derivative. When finding the antiderivative of a function, the constant 'c' represents the unknown constant that would make two otherwise identical functions have different values at a given point. The script emphasizes the inclusion of this arbitrary constant 'c' in the antiderivative to reflect the non-uniqueness of the integral, which is the reverse process of differentiation.
💡reverse power rule
The term 'reverse power rule' is used in the script to describe the process of finding the antiderivative of a function that is the derivative of a power function. It is the inverse operation of the power rule used in differentiation. The script demonstrates that the antiderivative of x to the power of n is x to the power of (n+1) divided by (n+1) plus a constant, effectively 'undoing' the differentiation process and finding the original function from its derivative.
💡integration
Integration is the process of finding the original function from its derivative, which is the antiderivative. It is one of the two fundamental operations in calculus, the other being differentiation. In the video, the process of integration is discussed in the context of finding the antiderivative of a given function. The script uses the term 'integration' to describe the process of applying the reverse power rule to find the antiderivative of x to the power of n.
💡differentiation
Differentiation is the process of finding the derivative of a function, which represents the rate of change of the function with respect to its variable. In the video, differentiation is used to find the derivative of the given function, x to the power of (n+1) over n plus 1, plus a constant. The script explains that the derivative simplifies to x to the power of n, and this process is essential for understanding how the function changes and for applying the reverse power rule to find the antiderivative.
💡undefined
In the context of the video, the term 'undefined' refers to the situation where the expression becomes mathematically invalid or does not have a meaningful value. Specifically, the script mentions that if 'n' were equal to negative 1, the expression would be undefined because it would involve division by zero. This highlights the importance of considering the domain of the variables when performing mathematical operations, especially in differentiation and integration.
Highlights

Derivative of x to the power of n plus 1 over n is discussed, with the assumption that n is not negative 1 to avoid division by zero.

The power rule is applied to find the derivative, which results in n times x to the power of n.

The derivative of a constant with respect to x is zero, simplifying the expression further.

The antiderivative of x to the n is x to the power of n plus 1 over n plus 1, encapsulating a constant term.

The concept of the antiderivative is introduced as the reverse of the power rule, applicable for any n not equal to negative 1.

An example is provided to find the antiderivative of x to the fifth power, resulting in x to the sixth power over 6 plus a constant.

The process of finding the antiderivative of a scalar multiple of a function is explained, showing that the scalar remains intact.

The antiderivative of 5 times x to the negative 2 power is calculated, demonstrating the rule's application to more complex functions.

The concept of an arbitrary constant in antiderivative is discussed, emphasizing that it can be represented differently but still represents an unknown value.

The derivative of the found antiderivative is taken to verify the rule, confirming that it indeed yields the original function.

The transcript provides a clear and detailed explanation of the power rule and its reverse in finding derivatives and antiderivatives.

The importance of avoiding division by zero when n equals negative 1 is emphasized to maintain the validity of the mathematical expressions.

The transcript uses a step-by-step approach to guide the reader through the process of finding derivatives and antiderivatives, making it accessible to learners.

The generality of the rule is highlighted, noting its applicability to any power as long as n is not negative 1.

The transcript provides a practical application of the power rule and its reverse in calculating derivatives and antiderivatives of polynomial functions.

The transcript's methodical explanation and use of examples make it a valuable resource for understanding the relationship between derivatives and antiderivatives.

The transcript emphasizes the encapsulation of multiple constants in the antiderivative, providing a comprehensive understanding of the concept.

The transcript's approach to explaining the power rule and its reverse is both theoretical and practical, offering insights into the mathematical principles and their applications.

Transcripts

Browse More Related Video

Definite integral of radical function | AP Calculus AB | Khan Academy

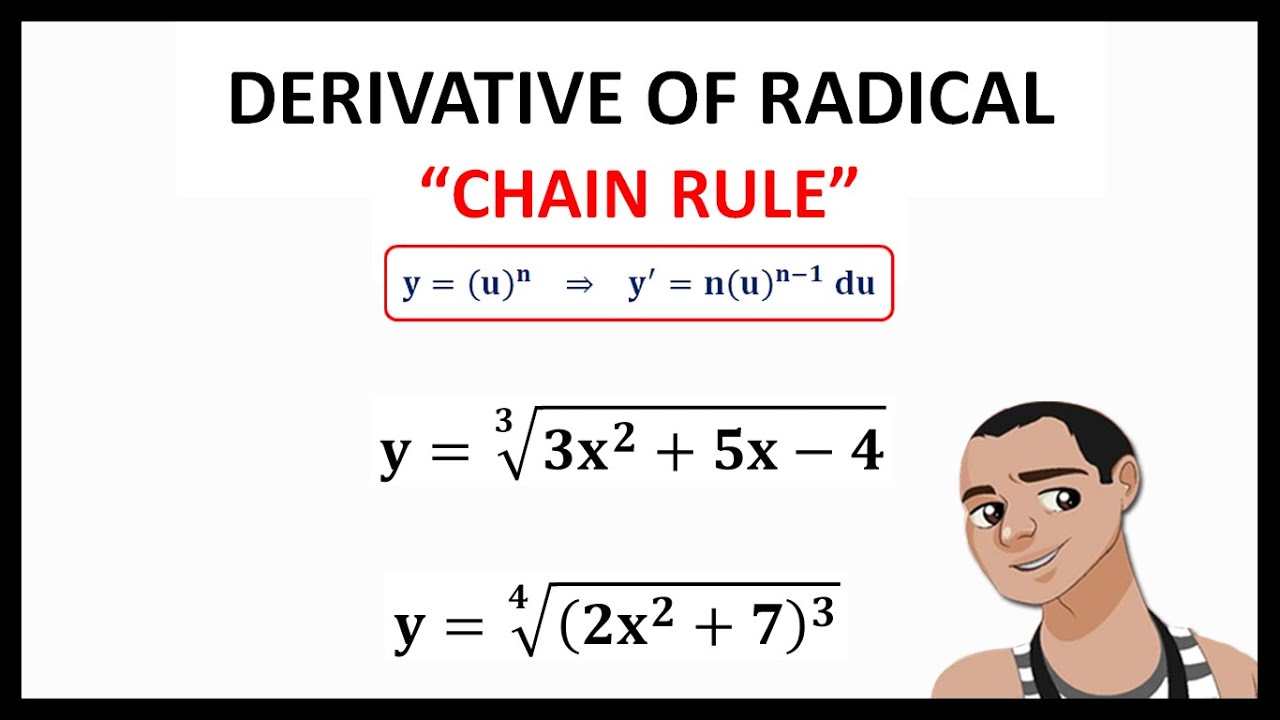
DERIVATIVE OF RADICAL: THE CHAIN RULE

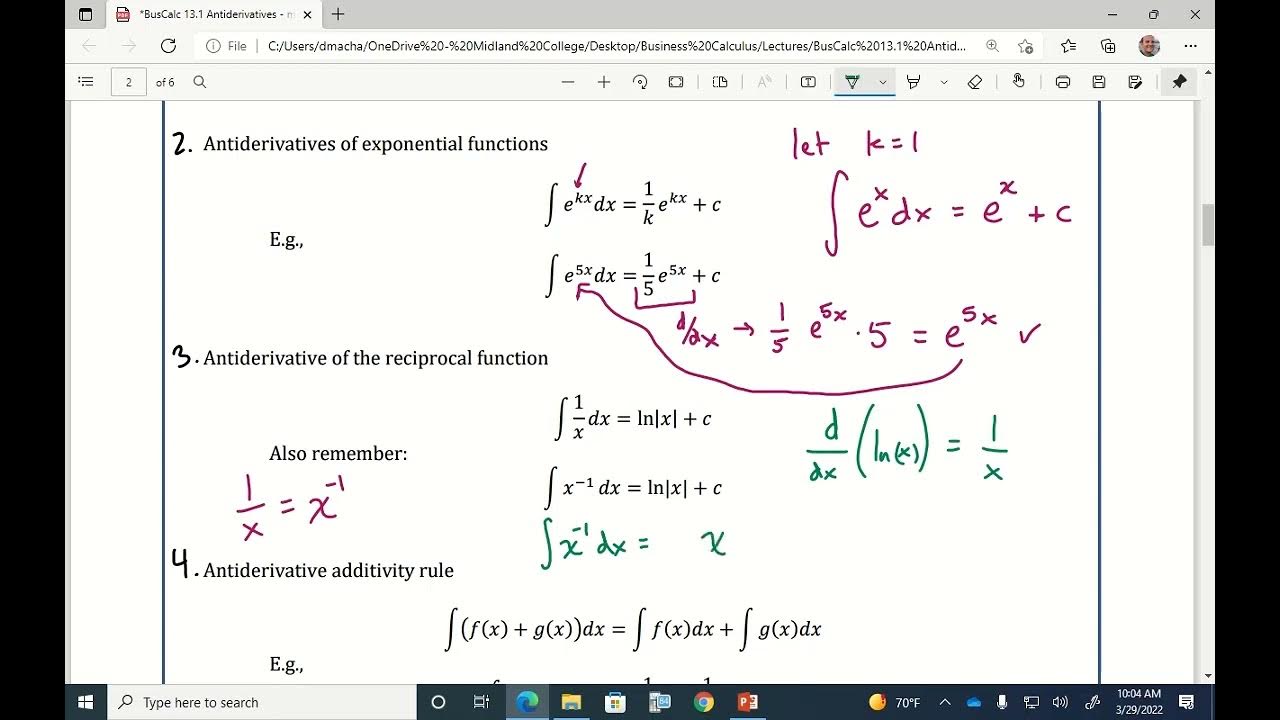
BusCalc 13.1 Antiderivatives

Calculus - How to find the derivative of a function using the power rule

The Integral Calculus Rules — Topic 86 of Machine Learning Foundations

The Indefinite Integral or Anti-derivative

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus ConceptsPower RuleDerivative AntiderivativeMathematical AnalysisEducational ContentAvoiding ErrorsGeneral ApplicationMathematics TutorialAlgebraic TechniquesInverse Processes