8.01x - Lect 2 - 1D Kinematics - Speed, Velocity, Acceleration
TLDRIn this engaging lecture, the concept of one-dimensional motion is explored through the analysis of velocity and acceleration. The discussion begins with the definition of average velocity, emphasizing its dependence on the choice of time intervals and the direction of motion. The lecture then delves into the concept of instantaneous velocity as the limiting case of average velocity as time intervals shrink. The critical distinction between speed and velocity is highlighted, with examples illustrating how average speed can differ from zero even when average velocity is zero. The lecture further introduces average acceleration, differentiating it from velocity and providing a clear explanation of its calculation. The significance of signs in physics and the impact of choosing different reference points are also discussed. Practical examples, such as measuring the speed of a bullet and the acceleration of a bouncing tennis ball, are used to demonstrate the application of these concepts. The lecture concludes with an exploration of instantaneous acceleration and its relationship with the second derivative of position with respect to time. The importance of understanding the underlying physics and being able to visualize and interpret the mathematical models is emphasized throughout.
Takeaways
- 📈 The concept of one-dimensional motion is introduced, focusing on the object's position along a straight line at different time intervals.
- 🏁 Average velocity is defined as the change in position (Δx) divided by the change in time (Δt) and is represented by a bar over the velocity symbol.
- 🔄 The average velocity for an object returning to its starting point is zero, emphasizing that average velocity is dependent on the choice of time intervals.
- 📊 The importance of direction in determining the sign of velocity and acceleration is highlighted, with the zero point on the x-axis being arbitrary.
- 🕒 The difference between speed and velocity is clarified, with average speed being the total distance traveled divided by the time taken, regardless of direction.
- 🎯 Instantaneous velocity is defined as the limit of the average velocity as the time interval approaches zero, represented by the first derivative of position with respect to time (v = dx/dt).
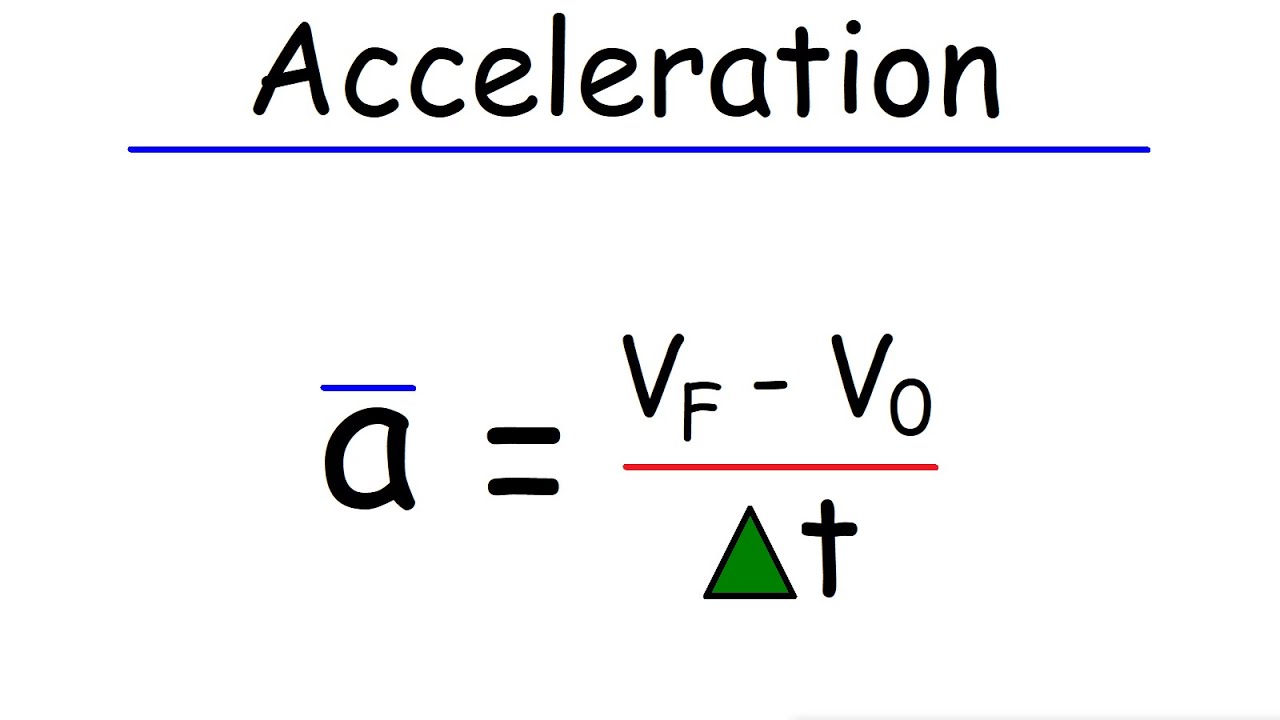
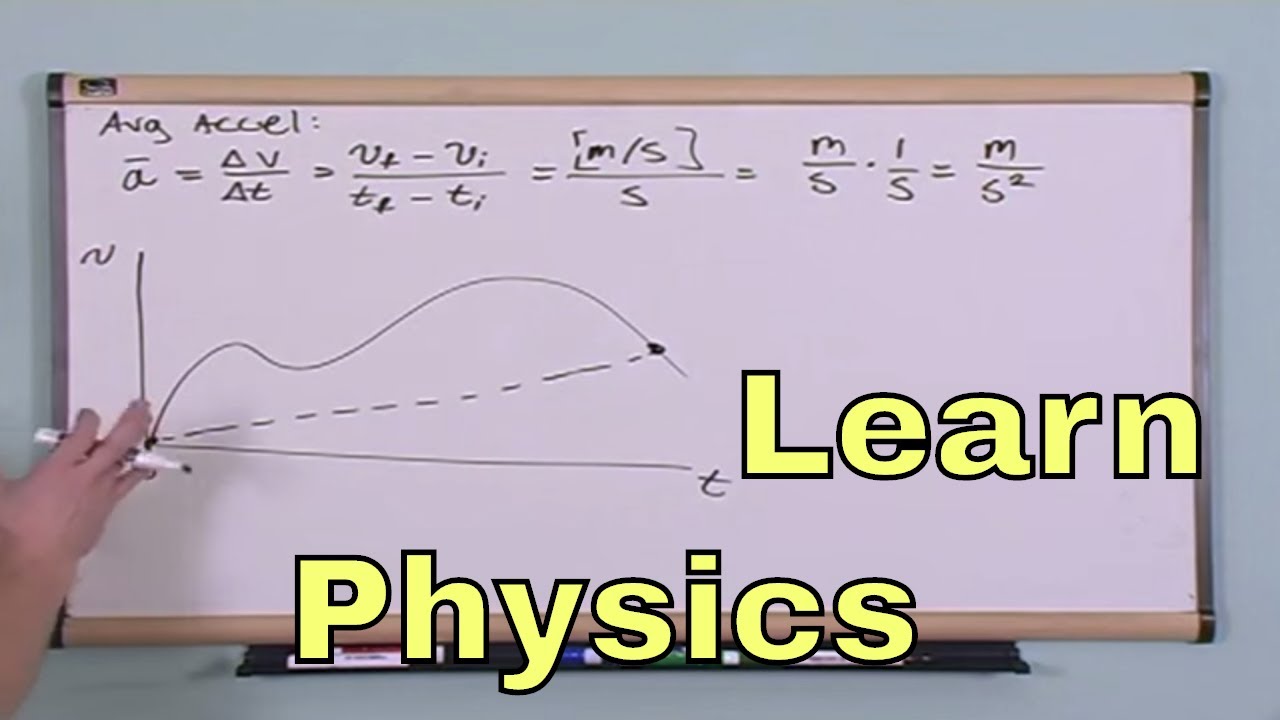
- 🚀 The concept of acceleration is introduced as the rate of change of velocity over time, with average acceleration calculated similarly to average velocity but considering changes in velocity (a = Δv/Δt).
- 🔽 The impact of gravitational acceleration (g) on free-falling objects is discussed, noting that it is constant and independent of the object's properties in a vacuum.
- 📐 The general equation for one-dimensional motion with constant acceleration is presented as x = C1 + C2t + C3t², where C1 represents the initial position, C2 the initial velocity, and C3 is related to the acceleration.
- 🕵️♂️ The demonstration of dropping an apple and using strobe light to observe its position at different instances during the fall visually illustrates the principles of acceleration and motion.
- 📸 The use of a strobe light to capture multiple instances of a falling object within a short time span demonstrates the increase in velocity over time due to gravity.
Q & A
What is the definition of average velocity?
-Average velocity is defined as the displacement (change in position) divided by the time interval during which the displacement occurred. In the context of the script, it is given by the formula x(t2) - x(t1) / (t2 - t1).
How does the direction of motion affect average velocity?
-The direction of motion affects average velocity by determining the sign of the displacement. If the object moves in the positive direction, the average velocity is positive; if it moves in the negative direction, the average velocity is negative. The choice of the positive direction is arbitrary but consistent within a given problem.
What is the difference between average velocity and average speed?
-Average velocity is the rate of change of position with respect to time and has direction, while average speed is the total distance traveled divided by the total time taken, without considering direction. Average speed is always non-negative, whereas average velocity can be positive, negative, or zero.
What is instantaneous velocity?
-Instantaneous velocity is the velocity of an object at a specific moment in time. It is the limiting value of the average velocity as the time interval approaches zero. Instantaneous velocity can be found by differentiating the position-time function with respect to time.
How can you calculate instantaneous speed?
-Instantaneous speed is the magnitude of the instantaneous velocity. It can be calculated by taking the square root of the sum of the squares of the instantaneous velocity components (if any), or by considering the rate at which an object covers distance at a particular moment, without regard to direction.
What is the relationship between velocity and acceleration?
-Acceleration is the rate of change of velocity with respect to time. It describes how quickly the velocity of an object is changing. If the acceleration is in the same direction as the velocity, the object is speeding up; if it is in the opposite direction, the object is slowing down.
What is the definition of average acceleration?
-Average acceleration is the change in velocity divided by the time interval over which the change occurs. It is a vector quantity that has both magnitude and direction, and can be calculated using the formula (v(t2) - v(t1)) / (t2 - t1).
How can you find the time when the velocity of an object is zero?
-To find the time when the velocity is zero, you set the instantaneous velocity equation to zero and solve for the time variable. This occurs at the points where the object changes direction or comes to a temporary stop.
What is the significance of the gravitational acceleration in the context of free-fall motion?
-Gravitational acceleration, denoted by 'g', is the constant acceleration due to gravity that all objects experience when they are in free fall, regardless of their mass or other physical properties (assuming no air resistance). It is a fundamental quantity in physics and has a standard value of approximately 9.81 m/s^2 on the surface of the Earth.
How can you determine the gravitational acceleration using free-fall experiments?
-By dropping an object and measuring the time it takes to fall a known distance, you can use the kinematic equations of motion to calculate the gravitational acceleration. Specifically, using the equation x = x0 + v0*t + 0.5*g*t^2, where x is the displacement, x0 is the initial position, v0 is the initial velocity (zero in the case of free fall), t is the time, and g is the gravitational acceleration, you can solve for 'g' once the time and displacement are known.
What is the significance of the experiment involving the strobe light and the falling apple?
-The strobe light experiment with the falling apple demonstrates the increasing velocity of the object in free fall. As the apple falls, it is illuminated by the strobe light at regular intervals, creating multiple images of the apple at different positions. The increasing number of images captured in a short duration indicates the acceleration of the apple due to gravity, as the velocity increases with time.
Outlines
📐 Introduction to Velocities and Accelerations
This paragraph introduces the concepts of velocities and accelerations in the context of one-dimensional motion. It begins with a simple example of an object moving along a straight line and defines the average velocity as the change in position divided by the change in time. The distinction between average velocity and instantaneous velocity is highlighted, with the latter being defined at a specific time. The concept of average speed is also introduced, which is the total distance traveled divided by the time taken, and is noted to be different from average velocity as it does not account for direction. The importance of sign conventions in physics is emphasized, and the impact of choosing different reference points for position and direction is discussed.
📉 Understanding Average Velocity and Speed
This section delves deeper into the concepts of average velocity and speed, emphasizing their differences and how they are calculated. It clarifies that while average velocity can be zero if an object returns to its starting point, average speed is not zero as it accounts for the total distance traveled. The paragraph also explains the relationship between instantaneous velocity and the first derivative of the position-time graph, and how to determine when the velocity is zero by looking for the derivative to be zero. The discussion includes the impact of the angle (alpha) on the sign of average velocity and the significance of sign conventions in physics calculations.
🏃♂️ Measuring Average Speed and Velocity
This paragraph discusses the practical aspects of measuring average speed and velocity, using the example of a bullet passing through two wires. It explains the importance of accounting for uncertainties in both distance and timing measurements. The text also explores the concept of instantaneous velocity and how it can be understood as the limiting case as time intervals approach zero. The significance of the first derivative of the position-time graph in determining instantaneous velocity is highlighted, and the equation v = dx/dt is introduced as a key formula to remember.
🚀 Introduction to Average Acceleration
This section introduces the concept of average acceleration, which is defined as the change in velocity over time. It explains how to calculate average acceleration and how it can be positive, negative, or zero depending on the change in velocity. The text also discusses the relationship between average acceleration and the direction of increasing x, and how reversing this direction can change the signs of both velocity and average acceleration. Practical examples involving a tennis ball and objects like a tomato or an egg are used to illustrate the concepts, emphasizing that the magnitude of acceleration, regardless of its sign, can cause objects to break upon impact.
📈 Instantaneous Acceleration and its Significance
This paragraph focuses on the concept of instantaneous acceleration, which is the acceleration at any given moment in time. It is described as the first derivative of the velocity-time graph and the second derivative of the position-time graph. The text explains how to determine where acceleration is zero, positive, or negative by analyzing the graph and understanding how the angle (alpha) changes. A straightforward example is provided, where the position of an object is given as a function of time, and the velocity and acceleration are derived from it. The example is used to illustrate how to find the times when the object's position, velocity, and acceleration are zero and how to plot the object's trajectory as a parabola.
🌍 Gravitational Acceleration and its Independence
This section discusses the concept of gravitational acceleration, which is constant and independent of various factors such as the mass, speed, chemical composition, size, and shape of the object, assuming no air drag and vacuum conditions. The general equations for one-dimensional motion under constant acceleration, such as gravity, are presented, and the terms C1, C2, and C3 are explained in relation to initial position, initial velocity, and half of the acceleration, respectively. The gravitational acceleration in Boston is given as 9.80 meters per second squared, and the text prompts the reader to calculate 'g' using two different experiments and to consider the uncertainties in these measurements.
🎥 Demonstration of Falling Objects and Alertness Test
This paragraph describes a demonstration involving the observation of falling objects to test alertness and understanding of the concepts of velocity and acceleration. The demonstration involves dropping an apple and using a strobe light to illuminate the apple at regular intervals, allowing the observer to see the apple's position at different moments during its fall. The observers are asked to estimate where the apple was during each flash and to count the number of flashes seen during a higher frequency strobe. The results of the demonstration are used to illustrate the increasing velocity of the falling object and to test the observers' ability to accurately perceive and count the number of flashes in a short period.
🤓 Conclusion and Weekend Advice
In this final paragraph, the speaker concludes the discussion by humorously advising those who only saw a certain number of flashes during the demonstration to rest over the weekend. The speaker also expresses the need for rest, indicating the end of the session and a light-hearted approach to the challenging material covered.
Mindmap
Keywords
💡Velocity
💡Acceleration
💡Position
💡Displacement
💡Speed
💡Derivative
💡Sign Convention
💡One-Dimensional Motion
💡Instantaneous
💡Gravitational Acceleration
💡Uncertainty
Highlights
Discussion on velocities and acceleration in one-dimensional motion.
Definition of average velocity and its calculation between two time points.
Explanation of how average velocity can be positive, negative, or zero depending on the position of the object at different times.
Introduction to the concept of average speed and how it differs from average velocity.
Illustration of the difference between speed and velocity through the example of an object's motion.
Derivation of instantaneous velocity as the limiting case of average velocity.
Explanation of how to calculate instantaneous velocity using the first derivative of position versus time.
Discussion on the significance of the direction of motion in determining the signs of velocity and acceleration.
Practical example of measuring the average speed of a bullet using wires and a timer.
Introduction to average acceleration and its calculation between two time points.
Explanation of how the signs in velocity and average acceleration depend on the chosen increasing value of x.
Demonstration of the impact of acceleration on objects like a tennis ball and a tomato.
Derivation of instantaneous acceleration as the first derivative of velocity versus time, and its relation to the second derivative of position versus time.
Example of finding the velocity and acceleration at any moment in time for an object in motion.
General equation for position and velocity as a function of time for one-dimensional motion with constant acceleration.
Discussion on the independence of gravitational acceleration from various physical properties of an object.
Experimental setup to measure gravitational acceleration by dropping an apple and timing its fall.
Illustration of the increasing velocity of a falling object with time through a strobe light experiment.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: