Sketching a curve | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRIn this lecture, the professor explains how to sketch the curve y = x / (1 + x^2) using knowledge of first and second derivatives. The process involves analyzing the function's domain, end behavior, and critical points. By calculating derivatives, identifying maximum and minimum values, and understanding concavity changes, a detailed graph of the function is constructed. The function is also noted to be odd, leading to symmetry about the origin. The lecture aims to reinforce concepts from calculus and precalculus in practical curve sketching.
Takeaways
- 📚 The lecture focuses on sketching the curve of the function y = x / (1 + x^2) using knowledge of first and second derivatives.
- 🔍 The function is defined for all x values because the denominator (1 + x^2) is always positive, so there are no vertical asymptotes.
- 🌐 The end behavior of the function is that as x approaches positive or negative infinity, the function approaches zero, indicating a horizontal asymptote at y = 0.
- ⚖️ The sign of the function is determined by the numerator (x), as the denominator is always positive.
- 📈 The first derivative, f'(x), is calculated to find critical points, which are potential maxima or minima.
- 📉 The second derivative, f''(x), is used to identify inflection points, where the concavity of the function changes.
- 🔢 The critical points are found where f'(x) = 0, which occurs at x = ±1, indicating potential maxima and minima.
- 🔍 The inflection points are found where f''(x) = 0, which occurs at x = 0 and x = ±√3, indicating changes in concavity.
- 📊 Evaluating the function at x = 0 gives the point (0, 0), which is on the graph and serves as a starting point for sketching.
- 📌 The function is symmetric about the origin because it is an odd function, with the numerator and denominator having opposite parity.
- 🎨 The final sketch of the curve should reflect the function's behavior: increasing and then decreasing around x = ±1, with inflection points at x = 0 and x = ±√3, and approaching the horizontal asymptote y = 0 as x goes to infinity.
Q & A
What is the main topic of the recitation?
-The main topic of the recitation is to sketch the curve of the function y = x / (1 + x^2) using first and second derivatives and understanding from algebra and precalculus.
Why is the function defined for all real numbers?
-The function is defined for all real numbers because the denominator, 1 + x^2, is always positive for any real number x, thus avoiding division by zero.
What is the end behavior of the function as x approaches positive or negative infinity?
-As x approaches positive or negative infinity, the function approaches 0 because the higher power in the denominator dominates the expression.
What is the horizontal asymptote of the function?
-The horizontal asymptote of the function is y = 0, as the function approaches this value as x goes to positive or negative infinity.
What does the sign of the function depend on?
-The sign of the function depends on the numerator, x, because the denominator, 1 + x^2, is always positive.
What is the first derivative of the function f(x) = x / (1 + x^2)?
-The first derivative, f'(x), is (1 - x^2) / (1 + x^2)^2, obtained by applying the quotient rule to the original function.
How can you determine the critical points of the function?
-Critical points can be determined by setting the first derivative equal to zero and solving for x, which gives the values where the function may have local maxima or minima.
What does the second derivative tell us about the function?
-The second derivative provides information about the concavity and points of inflection of the function, where the function changes from concave up to concave down or vice versa.
How many inflection points does the function have and where are they located?
-The function has three inflection points located at x = 0 and x = ±√3, determined by setting the second derivative equal to zero.
Why is the function y = x / (1 + x^2) considered an odd function?
-The function is considered odd because it satisfies the property f(-x) = -f(x), which is due to the numerator being an odd function and the denominator being an even function.
What symmetry does the function exhibit and why?
-The function exhibits rotational symmetry about the origin because it is an odd function, meaning that its graph is symmetric with respect to the origin.
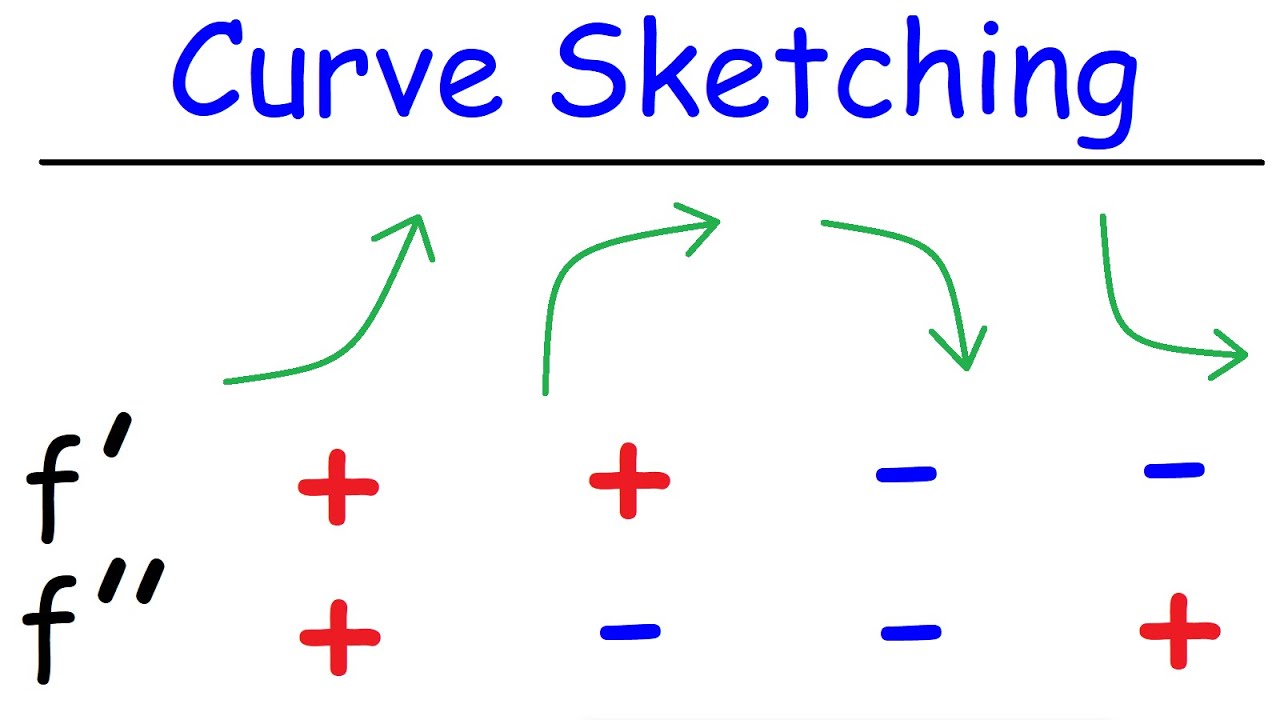
How can you sketch the curve of the function based on the information from the derivatives?
-You can sketch the curve by first plotting key points such as the intercepts and critical points, then using the first derivative to determine the slope of the tangent lines at those points, and the second derivative to determine the concavity and inflection points.
Outlines
📚 Introduction to Sketching a Curve with Derivatives
The professor begins the recitation by introducing the task of sketching the curve defined by the function y = x / (1 + x^2) using knowledge of first and second derivatives. The emphasis is on understanding the function's behavior through its derivatives without needing a perfect sketch. The professor plans to guide the students through the process after giving them time to attempt the sketch themselves. The initial step involves setting up an axis and considering the function's domain, which is all real numbers since the denominator never equals zero. The end behavior of the function is also discussed, noting that as x approaches infinity, the function approaches zero, indicating a horizontal asymptote.
🔍 Analyzing the Function's Derivatives and Critical Points
The professor continues by delving into the derivatives of the function. The first derivative is calculated to find critical points where the function may have local maxima or minima. The second derivative is then derived to identify inflection points, which indicate changes in concavity. The professor simplifies the expressions for both derivatives and sets them equal to zero to solve for x-values that correspond to these points of interest. The critical points found are x = ±1, and the inflection points are at x = 0 and approximately x = ±√3. The function's values at these points are evaluated, confirming that (0, 0), (1, 1/2), and (-1, -1/2) are on the graph, with the latter two indicating a maximum and minimum, respectively, based on the end behavior analysis.
📈 Sketching the Curve and Identifying Symmetry
In the final part of the script, the professor sketches the curve based on the information gathered from the derivatives and the function's values at critical points. The graph is expected to show a maximum at x = 1, a minimum at x = -1, and inflection points at x = 0 and x = ±√3. The professor also highlights the function's symmetry, pointing out that it is an odd function due to the numerator being odd and the denominator being even, which results in the graph being symmetric about the origin. The sketch is a rough representation of the curve's behavior, showing the ascent, descent, and changes in concavity, reflecting the calculated points and the function's inherent properties.
Mindmap
Keywords
💡First Derivative
💡Second Derivative
💡Critical Points
💡Inflection Points
💡End Behavior
💡Horizontal Asymptote
💡Concavity
💡Odd Function
💡Rational Function
💡Domain
Highlights
Introduction to using first and second derivatives to sketch the curve of the function y = x / (1 + x^2).
Emphasizing the importance of understanding the domain of the function, specifically checking for values that make the denominator zero.
Explanation of the end behavior of the function as x approaches positive and negative infinity, indicating a horizontal asymptote at y = 0.
Discussion on the sign of the graph, which is determined by the numerator since the denominator is always positive.
Derivation of the first derivative of the function using the quotient rule.
Derivation of the second derivative, highlighting its role in identifying changes in concavity.
Finding critical points by setting the first derivative equal to zero and solving for x.
Identifying potential maximum and minimum points at x = ±1 based on the first derivative.
Evaluating the function at x = 0 to establish the point (0, 0) on the graph.
Evaluating the function at x = 1 and x = -1 to find potential maximum and minimum values.
Using the second derivative to find inflection points and changes in concavity.
Identifying three inflection points at x = 0 and x = ±√3 based on the second derivative.
Highlighting the symmetry of the function due to its odd nature, with the graph being symmetric about the origin.
Rough sketching of the curve to visualize the behavior, including maximum, minimum, and inflection points.
Final remarks on the completed sketch of the curve, emphasizing the importance of using derivatives for curve analysis.
The practical application of the function's properties in sketching its curve, providing a comprehensive understanding of the function's behavior.
Encouragement for students to compare their sketches with the professor's to ensure a correct understanding of the curve's characteristics.
Transcripts
Browse More Related Video

Calculus 1 Lecture 3.4: The Second Derivative Test for Concavity of Functions

Lec 11 | MIT 18.01 Single Variable Calculus, Fall 2007
Curve Sketching - Graphing Functions Using Derivatives | Calculus

Concavity introduction | Using derivatives to analyze functions | AP Calculus AB | Khan Academy

Calculus I: Finding Intervals of Concavity and Inflection point

Curve Sketching with Asymptotes x/(x - 2)^2 and Derivatives of Rational Function
5.0 / 5 (0 votes)
Thanks for rating: