Curve Sketching with Asymptotes x/(x - 2)^2 and Derivatives of Rational Function

Anil Kumar
7 Apr 201617:55
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Anil Kumar guides viewers through the process of sketching the graph of the function f(x) = 2x / (x - 2) squared. He starts by identifying the y-intercept and vertical asymptote at x = 2, then discusses the horizontal asymptote at y = 0. Anil explains how to analyze the behavior of the function near these asymptotes and how it approaches infinity. He proceeds to find the local minimum at x = -2 and the point of inflection at x = -4 by calculating the first and second derivatives. The video concludes with a step-by-step guide on sketching the graph, taking into account intercepts, asymptotes, behavior, and concavity, providing viewers with a comprehensive understanding of graphing functions.

Takeaways
  • 📚 The video discusses the process of sketching the graph of the function f(x) = 2x / (x - 2)^2.
  • 📍 The y-intercept is found by setting x = 0, resulting in f(0) = 0, indicating the intercept is at the origin (0,0).
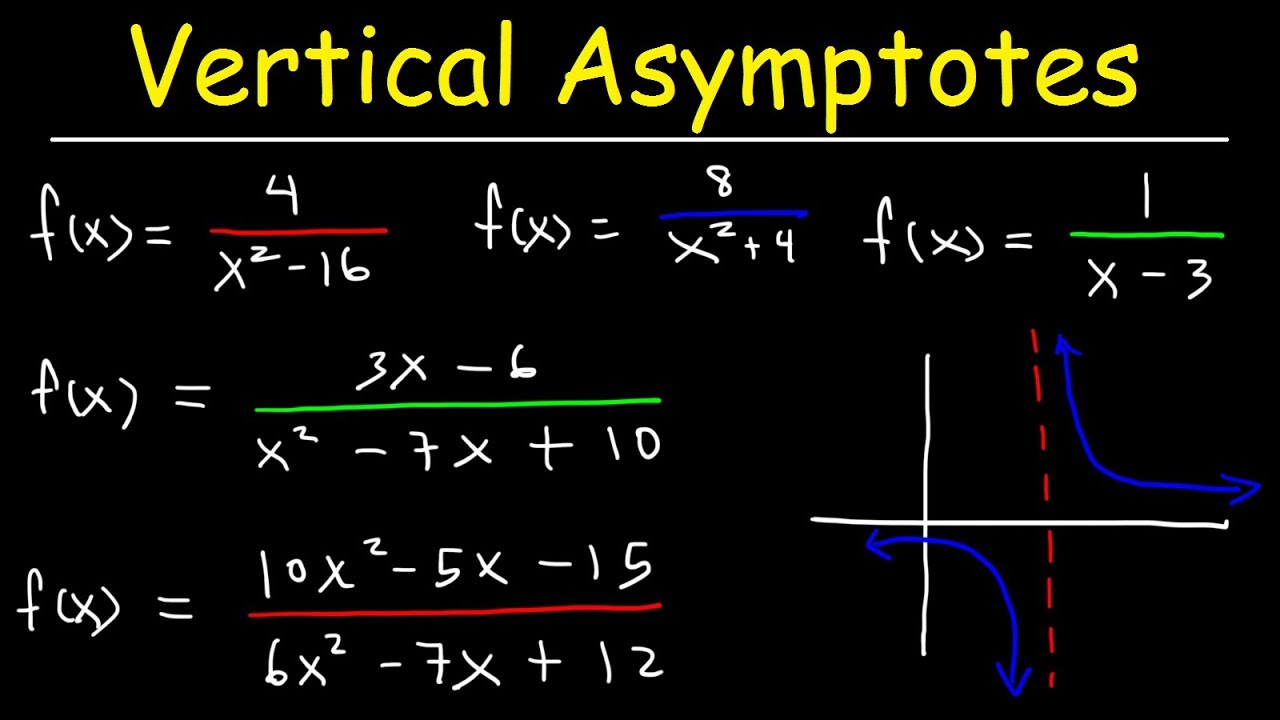
  • 🚫 The function has a vertical asymptote at x = 2, as the denominator becomes zero at this point, making the function undefined.
  • 📉 The horizontal asymptote is y = 0, as the degree of the denominator is higher than the numerator, causing the function to approach zero as x approaches infinity.
  • 🔍 Behavior near the vertical asymptote is analyzed by substituting values close to x = 2, showing the function approaches positive infinity from both sides.
  • 📈 Behavior near the horizontal asymptote is examined by substituting large negative and positive values for x, indicating the function approaches zero from both the negative and positive sides.
  • 🔢 The first derivative of the function is calculated using the quotient rule, leading to critical points at x = 2 ± 2.
  • 📉 Analysis of the first derivative reveals a local minimum at x = -2, with the function value f(-2) = -1/8.
  • 🔄 The second derivative is calculated to determine concavity, with a change in concavity indicating a point of inflection at x = -4.
  • 📊 The point of inflection is found to be at x = -4 with a function value f(-4) = -1/9, where the concavity changes from down to up.
  • 🎨 The final graph is sketched considering all the points, asymptotes, and behavior, providing a comprehensive visual representation of the function.
Q & A

  • What is the function being discussed in the video?

    -The function being discussed is f(x) = 2x / (x - 2)^2.

  • What is the y-intercept of the function f(x) = 2x / (x - 2)^2?

    -The y-intercept is at the point (0,0) because when x = 0, f(0) = 0 / (0 - 2)^2, which is indeed 0.

  • What is the x-intercept of the function?

    -The x-intercept is also at the origin (0,0), as the video script does not mention any other x-intercepts.

  • What is the equation for the vertical asymptote of the function?

    -The vertical asymptote is at x = 2, because the denominator of the function becomes zero at this point, making the function undefined.

  • What is the horizontal asymptote of the function, and why?

    -The horizontal asymptote is y = 0. As x approaches positive or negative infinity, the function approaches zero because the degree of the denominator is higher than the numerator.

  • How does the function behave near the vertical asymptote x = 2?

    -The function approaches positive infinity as x approaches 2 from both the left and right sides.

  • What is the first derivative of the function, and how is it found?

    -The first derivative, f'(x), is found using the quotient rule. It simplifies to -(x + 2) / (x - 2)^3, which helps in finding critical points and analyzing the function's increasing/decreasing behavior.

  • What are the critical points of the function, and how are they determined?

    -The critical points are x = 2 + 2 and x = -2, determined by setting the first derivative equal to zero and solving for x, which gives the points where the function could have local maxima or minima.

  • What is the local minimum value of the function, and at what x-value does it occur?

    -The local minimum occurs at x = -2, and the value of the function at this point is -1/8.

  • How is the point of inflection found, and what is its x-coordinate?

    -The point of inflection is found by analyzing the second derivative of the function. The x-coordinate of the point of inflection is -4.

  • What is the overall process for sketching the graph of the function as described in the video?

    -The process involves finding x and y intercepts, determining asymptotes and their behavior, identifying local maxima and minima, analyzing concavity and points of inflection, and then sketching the graph based on this information.

Outlines
00:00
📚 Introduction to Sketching the Graph of a Function

Anil Kumar introduces the process of graph sketching for the function f(x) = 2x / (x - 2)^2. He begins by identifying the y-intercept, which is found by setting x to 0, resulting in f(0) = 0, indicating the y-intercept at the origin (0,0). The video then proceeds to discuss the concept of vertical asymptotes, identifying x = 2 as the vertical asymptote due to the denominator becoming zero at this point. Horizontal asymptote is determined by the function approaching zero as x approaches infinity, indicating a horizontal asymptote at y = 0. Anil emphasizes the importance of plotting points and analyzing the behavior of the function near these asymptotes, which will help in developing a clear picture of the graph.

05:01
📉 Analyzing the Behavior Near Asymptotes and End Behavior

The script discusses the behavior of the function near the vertical asymptote at x = 2 by evaluating the function at points close to but not equal to 2, such as 1.9 and 2.1. It is observed that the function approaches positive infinity from both sides of the vertical asymptote. The end behavior of the function is also analyzed by substituting large negative and positive values for x, revealing that the function approaches zero from the negative side for large negative x and from the positive side for large positive x. This information helps in understanding how the graph will behave as it extends towards infinity.

10:03
🔍 Derivatives and Critical Points for Graph Sketching

Anil Kumar explains the process of finding the first derivative of the function using the quotient rule, which simplifies to f'(x) = -(x + 2) / (x - 2)^3. He identifies the critical points by setting the first derivative equal to zero, resulting in x = -2 and x = 2. The latter is discarded due to the vertical asymptote at x = 2. The behavior of the function is analyzed around the critical points, revealing a local minimum at x = -2. The value of the function at this point is calculated to be -1/8, providing the coordinates for the local minimum. The absence of a local maximum due to the vertical asymptote is also noted.

15:05
📈 Inflection Points and Concavity Analysis

The script moves on to the second derivative to analyze concavity and find inflection points. The second derivative is computed and simplified to reveal the concavity of the function. By testing points around the critical numbers x = -4 and x = 2, it is determined that the function changes concavity at x = -4, indicating an inflection point. The value of the function at x = -4 is calculated to be -1/9, providing the coordinates for the inflection point. The overall concavity of the function is described, with the graph showing concave down behavior for x < -4 and concave up behavior for x > -4.

🎨 Final Sketching of the Graph and Summary

With all the information gathered from intercepts, asymptotes, behavior near asymptotes, local extrema, and concavity, Anil Kumar provides a comprehensive sketch of the graph. The graph is shown with a local minimum at (-2, -1/8), a point of inflection at (-4, -1/9), and the correct behavior near the vertical and horizontal asymptotes. He emphasizes the importance of these elements in accurately representing the graph of the given function. The video concludes with a summary of the steps taken to sketch the graph, reinforcing the methodical approach to graph sketching.

Mindmap
Keywords
💡Graph Sketching
Graph sketching is the process of visually representing a mathematical function or relation on a coordinate plane. In the video, graph sketching is the central theme as the instructor discusses how to plot the graph of a given function, f(x) = 2x / (x - 2)^2. The process involves identifying key features such as intercepts and asymptotes, which are essential for accurately depicting the function's behavior.
💡Function
A function, in mathematics, is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. In the script, the function f(x) = 2x / (x - 2)^2 is the focus, and understanding its properties is crucial for sketching its graph correctly.
💡x-intercept
The x-intercept of a graph is the point where the graph crosses or touches the x-axis. It occurs when the function's value is zero. In the video, the x-intercept is found by setting f(0) to zero, which is a key step in determining the graph's position relative to the coordinate axes.
💡y-intercept
The y-intercept is the point where the graph of a function crosses the y-axis. It is found by evaluating the function at x = 0. In the script, the y-intercept is determined to be (0,0), which is a critical point in sketching the initial position of the graph.
💡Vertical Asymptote
A vertical asymptote is a vertical line that the graph of a function approaches but never crosses, occurring where the function's denominator is zero (and the numerator is not zero). In the video, the vertical asymptote is at x = 2, as the function becomes undefined at this point, and it is a key feature in sketching the graph's behavior near this value.
💡Horizontal Asymptote
A horizontal asymptote is a horizontal line that the graph of a function approaches as the values of the variable approach infinity or negative infinity. In the script, the horizontal asymptote is y = 0, indicating that the function's value approaches zero as x becomes very large in magnitude, which is important for understanding the long-term behavior of the graph.
💡Behavior Near Asymptotes
Behavior near asymptotes refers to how a function approaches its asymptotes. In the video, the instructor discusses the behavior of the function as it approaches both the vertical and horizontal asymptotes, which is crucial for sketching the graph accurately and understanding its limits.
💡First Derivative
The first derivative of a function measures its rate of change at a given point and can be used to find critical points, such as local maxima and minima. In the script, the first derivative of the function is calculated using the quotient rule, and it is used to analyze the function's increasing and decreasing intervals.
💡Local Minimum
A local minimum is a point on the graph of a function where the function changes from decreasing to increasing. In the video, the local minimum is found at x = -2, and its value is calculated to be -1/8, which helps in determining the shape of the graph in that region.
💡Concavity
Concavity refers to the curvature of a function's graph. A function is concave up where its second derivative is positive and concave down where its second derivative is negative. In the script, the second derivative is used to analyze the concavity of the function, which is essential for sketching the graph's curvature.
💡Point of Inflection
A point of inflection is a point on the graph of a function where the concavity changes. In the video, the point of inflection is found at x = -4, and it is where the function transitions from concave down to concave up, which is a critical feature in the graph's overall shape.
Highlights

Introduction to the process of sketching the graph of a function f(x) = (2x) / (x^2 - 4).

Finding the y-intercept by setting x to 0, resulting in f(0) = 0.

Identifying the origin (0,0) as the y-intercept point on the graph.

Determining the presence of a vertical asymptote at x = 2 due to the denominator becoming zero.

Plotting the vertical asymptote as a dotted line at x = 2.

Predicting a horizontal asymptote at y = 0 due to the degree of the denominator being higher than the numerator.

Sketching the horizontal asymptote as a dotted line along the x-axis.

Analyzing the behavior of the function near the vertical asymptote by testing points on either side of x = 2.

Observing the function approaches positive infinity as x approaches 2 from both sides.

Exploring the function's behavior towards the horizontal asymptote using values at negative and positive infinity.

Concluding the function approaches zero from the negative side at negative infinity and from the positive side at positive infinity.

Calculating the first derivative using the quotient rule to find critical points.

Identifying critical points at x = 2 ± 2 by analyzing the first derivative.

Determining a local minimum at x = -2 with a value of f(-2) = -1/8.

Finding the second derivative to analyze concavity and inflection points.

Identifying a point of inflection at x = -4 with a function value of f(-4) = -1/9.

Summarizing the process of sketching the graph by incorporating intercepts, asymptotes, behavior near asymptotes, local extrema, and points of inflection.

Transcripts

Browse More Related Video

How to graph a rational function using 6 steps

Q Center: a Math tutoring session

Infinite Limit vs Limits at Infinity of a Composite Function

How To Find The Vertical Asymptote of a Function

Graphing Rational Functions and Their Asymptotes

How to Graph Cosecant and Secant (Precalculus - Trigonometry 15)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Graph SketchingFunction AnalysisAsymptotesVertical AsymptoteHorizontal AsymptoteX and Y InterceptsBehavior Near AsymptotesLocal MinimumPoint of InflectionConcavity Changes