Fundamental Theorem of Calculus
TLDRThe video script explains how to find the area under a curve using the Fundamental Theorem of Calculus. It demonstrates the process through examples, starting with finding anti-derivatives and evaluating definite integrals from given limits. The script also covers handling functions with absolute values and fractional exponents, emphasizing the importance of understanding the graph's behavior to determine the correct area.
Takeaways
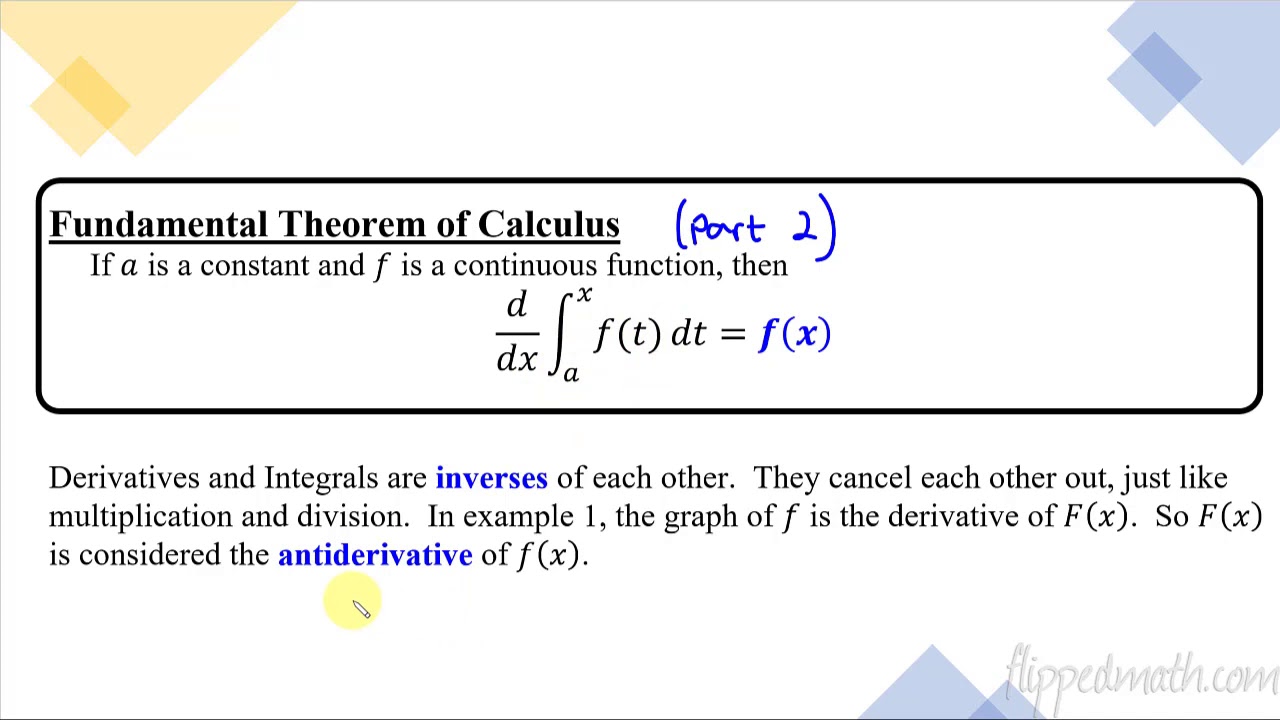
- 📚 The fundamental theorem of calculus is introduced, focusing on the first part which relates to finding the area under a curve.
- 📈 To find the area under a curve, treat the curve as if its derivative has been taken, and then find an anti-derivative.
- 🔍 The process involves integrating the function and then evaluating the anti-derivative at the limits of integration (A to B), subtracting the results.
- 🌐 An example is given with the function \( x^2 + 3 \), where the anti-derivative is found and evaluated from 1 to 2, resulting in an area of \( \frac{16}{3} \).
- 📉 The concept of net signed area is discussed, where areas below the x-axis are considered negative, affecting the total area calculation.
- 📚 The process is demonstrated with another example involving a quotient, showing how to separate the integral into parts and find the anti-derivatives.
- 📐 Fractional exponents are explained, emphasizing the method of taking the root first and then raising it to the power for easier calculation.
- 📌 The script covers the evaluation of the tangent function from 0 to \( \frac{\pi}{4} \), showing how to find the area under the curve using the anti-derivative.
- 🔢 Absolute values are addressed, explaining the need to split the integral into two parts when dealing with functions that include absolute values.
- 📉 The final example involves finding the area bounded by a graph, the x-axis, and vertical lines, demonstrating the process of finding an anti-derivative and evaluating it over a specified range.
Q & A
What is the main topic of the video script?
-The main topic of the video script is finding the area under a curve using the first part of the Fundamental Theorem of Calculus.
What does the first part of the Fundamental Theorem of Calculus state?
-The first part of the Fundamental Theorem of Calculus states that to find the area under a curve, treat it like it was the derivative of a function and find the antiderivative.
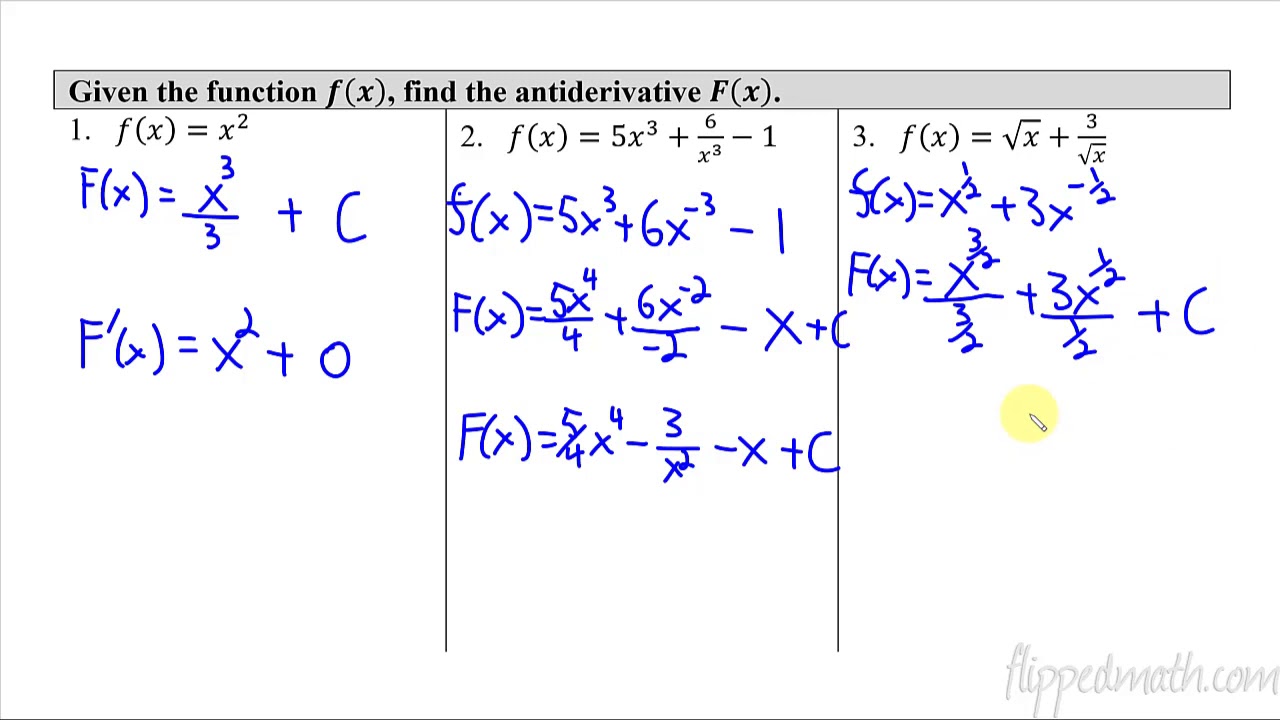
What is the process to find the area under the curve of x^2 + 3?
-To find the area under the curve of x^2 + 3, first find the antiderivative, which is x^3/3 + 3x. Then evaluate it from the lower limit to the upper limit and subtract these values.
What is the antiderivative of x^2 + 3?
-The antiderivative of x^2 + 3 is x^3/3 + 3x.
How do you handle the area calculation when the graph drops below the x-axis?
-When the graph drops below the x-axis, the area below the x-axis counts as a negative value in the net signed area calculation.
What is the exact area under the curve of x^2 + 3 from x = 1 to x = 2?
-The exact area under the curve of x^2 + 3 from x = 1 to x = 2 is 16/3.
How do you simplify the integral of a quotient, such as x/(x^1/2) - 4/x^2?
-To simplify the integral of x/(x^1/2) - 4/x^2, separate it into simpler terms: x^1/2 - 4x^(-2). Then find the antiderivative of each term individually.
What is the approach to evaluating integrals with fractional exponents?
-When evaluating integrals with fractional exponents, it's easier to take the root first and then raise it to the power to keep the numbers smaller.
How do you find the area under a curve involving absolute value, such as 2x - 1?
-To find the area under a curve involving absolute value, separate it into two intervals where the behavior of the function changes (at x = 1/2 for 2x - 1). Integrate each part separately, flipping the sign as necessary for the negative side.
What common mistake should be avoided when finding antiderivatives?
-A common mistake to avoid is incorrectly multiplying terms when finding antiderivatives, as seen in the error with 3x^2 over 2 being mistakenly simplified incorrectly.
Outlines
📚 Introduction to Area Under a Curve Using Fundamental Theorem of Calculus
This paragraph introduces the concept of finding the area under a curve when it's not a simple geometric shape like a triangle, trapezoid, or rectangle. It presents the first part of the fundamental theorem of calculus, which states that if you want to find the area under a curve, you should treat the function as if its derivative has already been taken. The method involves finding an anti-derivative (also known as the integral of the function), and then calculating the difference between the function's values at points A and B. The example given is finding the area under the curve of the function x^2 + 3, where the anti-derivative is found, and the process of plugging in the limits of integration and subtracting the results is demonstrated. The result is simplified to show the exact area under the curve, which is 16/3. The paragraph also includes a brief mention of graphing the function to visually confirm the positive area found.
🔍 Detailed Process of Calculating Area Under a Curve with Examples
The second paragraph continues the discussion on calculating the area under a curve, emphasizing the process of finding an anti-derivative as the first step. It provides an example involving a quotient that is separated into two parts, X^(3/2) and -4/X^2, and then simplified. The anti-derivatives for these parts are calculated, and the process of evaluating the definite integral from 1 to 9 is explained, including handling fractional exponents and simplifying the results. The paragraph also touches on the tangent function and its derivative, the secant, and how to evaluate the integral of the tangent function from 0 to π/4. Additionally, it discusses handling absolute values by breaking the integral into two intervals and finding the anti-derivatives for each interval, then evaluating and subtracting the results to find the area under the curve.
📉 Correcting Errors and Continuing the Calculation of Area Under a Curve
The third paragraph acknowledges a mistake made in the previous calculation and corrects it, emphasizing the importance of accuracy in mathematical processes. It then proceeds with another example of finding the area under the curve defined by the function 2x^2 - 3x + 2, bounded by the x-axis and the vertical lines x=0 and x=2. The anti-derivative of the function is calculated, and the process of evaluating the definite integral from 0 to 2 is described. The paragraph highlights the need to ensure that the graph does not dip below the x-axis, which would require breaking the integral into pieces. The final calculation is presented, with an apology for the earlier mistake, and the paragraph ends with a light-hearted note about making fun of the error the next day.
Mindmap
Keywords
💡Area under a curve
💡Fundamental Theorem of Calculus
💡Anti-derivative
💡Integration
💡Net signed area
💡Definite integral
💡Fractional exponents
💡Piwise function
💡Tangent function
💡Rookie mistake
Highlights
Introduction to finding the area under a curve using calculus without simple geometric shapes.
Explanation of the Fundamental Theorem of Calculus, focusing on the first part relevant to area calculation.
Process of finding the area under a curve by treating it like a derivative and using anti-derivatives.
Demonstration of calculating the area under the curve of the function x^2 + 3 by hand.
Integration of x^2 + 3 to find an anti-derivative without the constant C.
Using the anti-derivative to find the area by plugging in values from A to B and subtracting.
Calculation of the area under the curve x^2 + 3 resulting in a positive value of 16/3.
Graphing the function x^2 + 3 to visually confirm the positive area found.
Further examples of finding areas under curves with different functions.
Handling fractional exponents by taking the root first and then raising it to the power.
Process of finding the area under the curve for the function involving secant squared.
Dealing with absolute value functions by separating them into two intervals.
Explanation of how to find the area bounded by a graph, the x-axis, and vertical lines.
Error made during the calculation process and the correction of it.
Final calculation of the area under the curve for the function 2x^2 - 3x + 2.
Encouragement for students to engage with the material and learn from mistakes.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: