Infinite series as limit of partial sums | Series | AP Calculus BC | Khan Academy
TLDRThis script explores the concept of convergence or divergence in infinite series. It introduces a series S with a general formula for its nth partial sum, \( S_n = \frac{2n^3}{n+1} \cdot (n+2) \). The presenter explains that to determine if the series converges to a finite value or diverges, one should consider the limit of the partial sums as n approaches infinity. By simplifying the formula and analyzing the behavior of the limit, it's concluded that the series diverges because the limit of the partial sums tends to infinity, indicating the series does not converge to a finite value.
Takeaways
- 📚 The script discusses an infinite series S, which is the sum of a sequence from n=1 to infinity.
- 🔍 It provides a general formula for the partial sums of the series, where S sub n equals 2n cubed over (n+1) times (n+2).
- 🤔 The main question posed is whether the series converges to a finite value or diverges.
- 📉 The script suggests that the series diverges because the numerator grows faster than the denominator.
- 🧐 The concept of the limit as n approaches infinity of the partial sums is used to analyze the series' behavior.
- 🔢 By performing algebraic manipulation, the script simplifies the expression to see the behavior as n grows large.
- 📌 The script divides both the numerator and the denominator by n squared to isolate the leading terms.
- 📈 It is shown that as n approaches infinity, the leading term of the numerator (2n) grows without bound, while the denominator approaches 1.
- 🚫 The conclusion is that since the limit of the partial sums goes to infinity, the series diverges and does not converge to a finite value.
- 📝 The script emphasizes that for a series to converge, the limit of its partial sums must be a finite value, which is not the case here.
- 💡 The takeaway is that understanding the behavior of partial sums and their limits is crucial for determining the convergence of an infinite series.
Q & A
What is an infinite series S as described in the transcript?
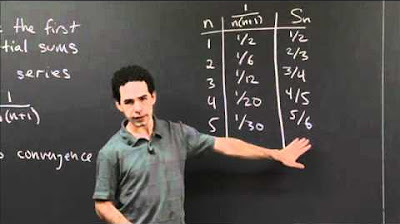
-An infinite series S is the sum from n = 1 to infinity of a sequence of terms a_n. It can be written as a_1 + a_2 + a_3 + ... continuing indefinitely.
How is the partial sum of the series S represented in the transcript?
-The partial sum of the series S is given by the formula S_n = 2n^3 / [(n + 1)(n + 2)].
What is the question posed about the series S in the transcript?
-The question posed is whether the infinite series S converges to a finite value or diverges and is unbounded.
What is the key idea used to determine if the series S converges or diverges?
-The key idea is to analyze the limit of the partial sums S_n as n approaches infinity. If the limit is finite, the series converges; if the limit is infinite, the series diverges.
How is the limit of the partial sums S_n calculated in the transcript?
-The limit is calculated by simplifying the expression for S_n. The numerator is a third-degree polynomial (2n^3) and the denominator is a second-degree polynomial (n^2 + 3n + 2).
What conclusion is drawn from the comparison of the numerator and the denominator in the limit calculation?
-The conclusion is that the numerator grows faster than the denominator, indicating that the expression is unbounded and approaches infinity.
What algebraic steps are taken to further clarify the limit as n approaches infinity?
-Both the numerator and the denominator are divided by n^2. This results in the expression 2n / (1 + 3/n + 2/n^2), which simplifies the analysis of the limit.
What happens to the terms 3/n and 2/n^2 as n approaches infinity?
-As n approaches infinity, both 3/n and 2/n^2 approach 0, making the denominator approach 1.
What final conclusion is reached about the series S based on the limit calculation?
-The final conclusion is that the limit of the partial sums S_n approaches infinity, meaning the series S diverges.
What condition would be necessary for the series S to converge?
-For the series S to converge, the limit of the partial sums S_n as n approaches infinity would need to be a finite value.
Outlines
📚 Understanding Infinite Series Convergence
This paragraph introduces the concept of an infinite series S, defined as the sum from n=1 to infinity of a sub n. The speaker explains the general formula for the partial sums of S, given as S sub n equals 2n cubed over (n+1) times (n+2). The main question posed is whether the series converges to a finite value or diverges. The paragraph sets the stage for an exploration of the series' behavior by examining the limit of partial sums as n approaches infinity.
Mindmap
Keywords
💡Infinite series
💡Partial sums
💡Convergence
💡Divergence
💡Limit
💡Polynomial
💡Numerator
💡Denominator
💡Algebra
💡Divide by n squared
💡Limit as n approaches infinity
Highlights
Infinite series S is defined as the sum from n=1 to infinity of a sub n.
Partial sums of S are given by the formula S sub n = 2n^3 / (n+1)(n+2).
The infinite series S is the limit as n approaches infinity of the partial sums.
The numerator is a third degree polynomial while the denominator is second degree, indicating the series will diverge.
Dividing the numerator and denominator by n squared simplifies the limit calculation.
As n approaches infinity, the limit of the partial sums goes to infinity.
The series diverges because the limit of the partial sums does not converge to a finite value.
For a series to converge, the limit of the partial sums should be a finite value.
The approach of taking the limit of partial sums to determine convergence is a fundamental concept in series analysis.
The video provides a clear algebraic method to evaluate the limit of the series.
Understanding the behavior of the numerator and denominator as n grows is key to determining convergence.
The video demonstrates that higher degree terms in the numerator dominate the limit.
Dividing by n squared helps isolate the leading terms for the limit evaluation.
The approach shows that the series does not have a finite sum, contrary to convergent series.
The video provides a step-by-step explanation of why the series diverges.
The concept of divergence is important in understanding the limits of series.
The video concludes that the series diverges, providing a clear conclusion to the analysis.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: