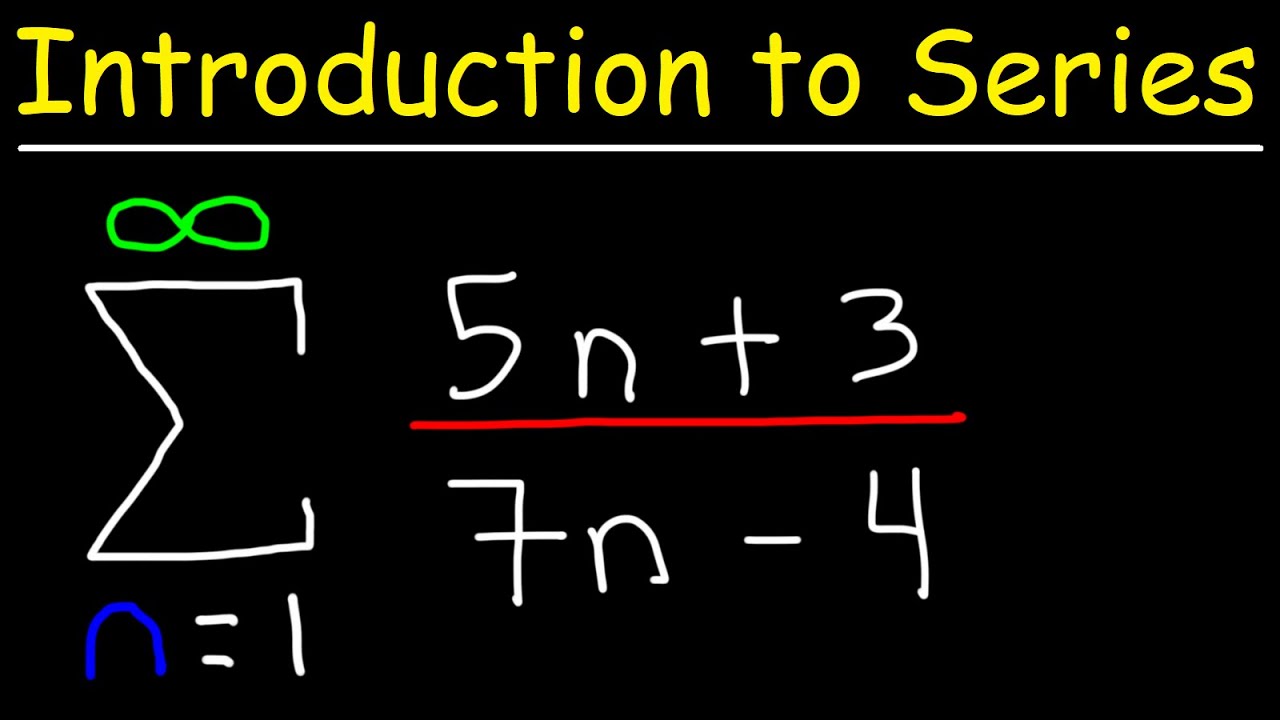
AP Calculus BC Lesson 10.3
TLDRThe video script discusses the nth term test for Divergence, a method to determine if a series diverges. It explains that if the limit of the sequence's terms as n approaches infinity is not zero, the series diverges. However, if the limit is zero, the test is inconclusive for convergence. The script provides examples to illustrate how to apply the test and clarify common mistakes, emphasizing that the test only confirms divergence, not convergence.
Takeaways
- 📌 The nth term test for Divergence states that if the limit as n approaches Infinity of a sub n is not equal to zero, the series diverges.
- 📈 The nth term test can only be used to determine divergence, not convergence of a series.
- 🚫 A common mistake is to assume that a series converges if the limit as n approaches Infinity of a sub n equals zero; the test is inconclusive in this case.
- 🌟 If a series converges and the limit as n approaches Infinity of a sub n is equal to zero, then the series converges by the nth term test.
- 🔢 Example analysis: A series with a general term of 4N^2 - 3n - 9/(-N^2 + n) diverges since the limit as n approaches Infinity is -4, which is not zero.
- 🔍 For the series 1/n^3, the nth term test is inconclusive as the limit as n approaches Infinity is zero.
- 📊 The nth term test is inconclusive for a series with a general term of -5 * (2/3)^n, as the limit as n approaches Infinity is zero.
- 📝 When the limit as n approaches Infinity of a sub n equals a non-zero value, the series diverges according to the nth term test.
- 🧠 For series like 3^n + 1 / (3^n + 1), the limit as n approaches Infinity is 3, indicating divergence by the nth term test.
- 🔄 The nth term test can be applied to series that do not fit the typical geometric series form, but other tests may be more appropriate for determining convergence or divergence.
- 📐 The secant function series diverges by the nth term test because the limit as n approaches Infinity does not exist, implying it is not equal to zero.
Q & A
What is the nth term test for divergence?
-The nth term test for divergence states that if the limit as n approaches infinity of a sub n is not equal to zero, then the series from n equals 1 to infinity of a sub n diverges.
What is the limitation of the nth term test in determining the convergence of a series?
-The nth term test can only be used to determine if a series diverges. It cannot be used to prove that a series converges, as it is inconclusive when the limit as n approaches infinity of a sub n is equal to zero.
What happens when the limit as n approaches infinity of a sub n equals zero?
-If the limit as n approaches infinity of a sub n equals zero, the nth term test is inconclusive. It does not provide any information about whether the series converges or diverges.
How does the nth term test apply to the series from n equals 1 to infinity of 4N squared minus 3n minus 9 over negative N squared plus n?
-The series diverges by the nth term test because the limit as n approaches infinity of the given expression is not equal to zero. The dominant term 4N squared in the numerator ensures that the limit is not zero, indicating divergence.
What is the significance of the series from n equals 1 to infinity of 1 over n cubed in the context of the nth term test?
-The limit as n approaches infinity of 1 over n cubed equals zero, which makes the nth term test inconclusive. This series is actually convergent, but the nth term test does not provide enough information to confirm this.
How does the nth term test help with the series from n equals 1 to infinity of negative five times two-thirds to the power of n?
-The nth term test is inconclusive for this series because the limit as n approaches infinity equals zero. However, using the properties of geometric series, we can determine that the series converges since the absolute value of the common ratio (two-thirds) is less than one.
What is the role of the nth term test in determining the convergence or divergence of the series from n equals 1 to infinity of 3 to the power of n plus 1 over 3 to the power of n plus one?
-The nth term test shows that the series diverges because the limit as n approaches infinity of the given expression is equal to 3, which is not zero.
How does the nth term test apply to the series from n equals 1 to infinity of 2 to the power of n over e to the power of n plus three?
-The nth term test is inconclusive for this series because the limit as n approaches infinity equals zero. However, by recognizing the series as a form of geometric series with a common ratio (2/e) less than one, we can conclude that the series converges.
What happens when applying the nth term test to the series from n equals 1 to infinity of the secant of n?
-The nth term test concludes that the series diverges because the limit as n approaches infinity does not exist, as the secant function oscillates indefinitely and does not approach zero.
Which series can the nth term test be used to determine divergence for, among the given options?
-The nth term test can be used to determine divergence for series 2 and 4 in the given options, as their limits as n approaches infinity are not equal to zero.
Outlines
📚 Introduction to the nth Term Test for Divergence
This paragraph introduces the concept of the nth term test for Divergence, explaining that if the limit as n approaches Infinity of a sub n is not equal to zero, then the series diverges. It clarifies that this test only indicates divergence, not convergence, and highlights a common misconception about the test's applicability. The explanation is supported by examples that demonstrate how to apply the test and interpret its results.
🧐 Analyzing Series with the nth Term Test
The paragraph delves into the application of the nth term test on various series, using examples to illustrate the process. It explains how to find the limit as n approaches Infinity for different functions and how this relates to the series' convergence or divergence. The paragraph also addresses the limitations of the nth term test, noting that it cannot be used to prove convergence and that other methods are needed for a definitive conclusion on series that do not diverge according to the test.
🔢 Evaluating Convergence and Divergence with the nth Term Test
This section continues the exploration of the nth term test by evaluating more series and determining their convergence or divergence. It explains the use of l'Hopital's rule in certain cases and the importance of recognizing when the test is inconclusive. The paragraph also introduces the concept of geometric series and how it can be used to determine the convergence of a series when the nth term test is inconclusive.
📈 Applying the nth Term Test to Specific Functions
The final paragraph focuses on applying the nth term test to specific functions, highlighting the conditions under which the test can confirm divergence. It examines a series of mathematical expressions, using both analytical and computational methods to determine if the limit as n approaches Infinity of a sub n is not equal to zero, which would indicate divergence. The paragraph concludes by emphasizing that while the nth term test can identify divergent series, other tests are necessary to confirm convergence.
Mindmap
Keywords
💡nth term test for Divergence
💡convergence
💡divergence
💡limit
💡series
💡geometric series
💡common ratio
💡l'Hopital's rule
💡horizontal asymptote
💡secant function
💡oscillating function
Highlights
The nth term test for Divergence is discussed, which states that if the limit as n approaches Infinity of a sub n is not equal to zero, then the series diverges.
The nth term test only tests for Divergence, not for convergence.
A common mistake is to think that if the limit as n approaches Infinity of a sub n equals zero, the series converges, which is not true.
If a series converges, then the limit as n approaches Infinity of a sub n must equal zero.
The series from n equals 1 to Infinity of 4N squared minus 3n minus 9 over negative N squared plus n diverges, as the limit as n approaches Infinity of a sub n is not zero.
The nth term test is inconclusive for the series from n equals 1 to Infinity of 1 over n cubed, as the limit as n approaches Infinity equals zero.
The series from n equals 1 to Infinity of negative five times two-thirds n to the power of n is shown to be inconclusive by the nth term test, but converges by geometric series test.
The series from n equals 1 to Infinity of 3 to the power of n plus 1 over 3 to the power of n plus one diverges, as the limit as n approaches Infinity is 3, not zero.
The series from n equals 1 to Infinity of 4N over the square root of N squared plus 4 diverges, as the limit as n approaches Infinity equals four, not zero.
The series from n equals 1 to Infinity of 2 to the power of n over e to the power of n plus three is shown to converge by geometric series test, not by the nth term test.
The series from n equals 1 to Infinity of the secant of n diverges by the nth term test, as the limit does not exist and is not equal to zero.
The series from n equals 1 to Infinity of e to the power of n plus 2 over 2 N cubed diverges, as the limit as n approaches Infinity is infinity, not zero.
The nth term test can be used to determine Divergence for series where the limit as n approaches Infinity of a sub n is not equal to zero.
Series 2 (e to the power of n plus 3 over 8N) and series 4 (cosine Pi n over 4E) can be determined to diverge using the nth term test.
Series 1 (2N to the fifth minus three n cubed over N to the sixth) and series 3 (2 times 1/3 to the power of n) cannot be determined to diverge using the nth term test, as their limits approach zero.
The nth term test is a valuable tool for quickly identifying if a series diverges, but other methods are needed for a definitive conclusion on convergence.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: