Calc 2, Exam 1 walkthrough (Fall 2023)
TLDRThis video script offers a detailed walkthrough of a Calculus 2 exam, focusing on the application of integration. It covers various problems, including finding the length of a parametric curve, the surface area of a curve revolved around an axis, and the work done in lifting a steel beam with a pulley system. The script also explores the area enclosed by polar coordinates, volume of revolution using both the shell and washer methods, finding the equation of a tangent line to a polar curve, and calculating the center of mass of a plate with variable density. The instructor emphasizes the importance of understanding formulas, simplifying expressions, and carefully setting up integrals to solve these problems.
Takeaways
- 📚 The script is a walkthrough of a Calculus 2 exam, focusing on applications of integration, particularly for finding lengths of curves, areas, and volumes.
- 🔍 It emphasizes the importance of correctly identifying the type of problem being addressed, such as length of curve, surface area, or work done in a physical system.
- 📝 The script provides a detailed explanation of how to set up integrals for different scenarios, including parametric equations, polar coordinates, and volume of revolution.
- 📈 The importance of algebraic simplification is highlighted, especially when dealing with the integrands that can become complex.
- 📐 The script explains the concept of symmetry in problems and how it can be used to simplify calculations by breaking down the problem into more manageable parts.
- 📉 The process of finding the center of mass for a two-dimensional shape with variable density is discussed, involving the calculation of mass and moments.
- 📘 The script includes a step-by-step guide for setting up and solving a problem involving a pulley system, illustrating the concept of work done in lifting an object.
- 📌 Attention to detail is stressed, particularly in distinguishing between different components of a problem, such as the radius of rotation in volume of revolution problems.
- 📑 The script provides a methodical approach to solving complex integrals, including the use of trigonometric identities and power reduction formulas.
- 🔑 The concept of 'moments' is introduced in the context of finding the center of mass, which is a key component in understanding the balance of an object.
- 🎯 The final takeaway is the encouragement to practice and study, highlighting the importance of patience and careful step-by-step problem-solving in calculus.
Q & A
What is the main topic of the video script?
-The main topic of the video script is a comprehensive review of a Calculus 2 exam, focusing on applications of integration, including finding the length of curves, surface area, volume of solids of revolution, and center of mass.
What are the three scenarios for finding the length of a curve as described in the script?
-The three scenarios for finding the length of a curve are: 1) when the curve is given in the form y = f(x), 2) when the curve is given parametrically as x = x(t) and y = y(t), and 3) when the curve is given in polar coordinates as r = f(θ).
How is the length of a parametric curve calculated?
-The length of a parametric curve is calculated by integrating the square root of the derivative of x with respect to t squared plus the derivative of y with respect to t squared, from t=a to t=b.
What is the formula for finding the surface area of a curve revolved around the x-axis?
-The formula for finding the surface area of a curve revolved around the x-axis is 2π times the integral from a to b of the absolute value of y times the square root of 1 plus the derivative of y with respect to x squared, dx.
What is the concept of work in the context of lifting a steel beam with a pulley system?
-In the context of lifting a steel beam with a pulley system, work is the product of the force required to lift the beam and the distance the beam is moved. This includes the weight of the beam and the variable weight of the cable as it is lifted.
How is the work done in lifting a beam with a cable calculated in the script?
-The work done is calculated by integrating from the ground to the top floor the force (which varies due to the changing weight of the cable) times the small increment in height dy.
What is the significance of symmetry in solving problems related to areas and volumes in polar coordinates?
-Symmetry in problems related to areas and volumes in polar coordinates allows for simplification of the calculations by breaking down the problem into smaller, more manageable parts, such as calculating the area of one 'petal' of a four-leaf clover shape and then multiplying by four.
What is the method used to find the volume of a solid formed by revolving a region around a line using horizontal slices?
-The method used to find the volume of a solid formed by revolving a region around a line using horizontal slices is the washer method, where the volume is found by integrating the difference of the squares of the outer and inner radii, multiplied by the thickness dy, from the bottom to the top of the region.
How is the center of mass of a two-dimensional object calculated?
-The center of mass of a two-dimensional object is calculated by finding the average location of the mass, which involves integrating the product of the density function and the distance from a reference point (like the x-axis) over the area, and then dividing by the total mass.
What is the key to solving the problem of finding the Cartesian equation of the tangent line to a polar curve at a specific point?
-The key to solving the problem of finding the Cartesian equation of the tangent line to a polar curve at a specific point is to find the point of tangency in Cartesian coordinates, calculate the slope of the tangent line using derivatives in parametric form, and then use the point-slope form to write the equation of the line.
What is the importance of understanding the density function in center of mass problems?
-Understanding the density function is crucial in center of mass problems because it determines how mass is distributed across the object. This distribution affects the calculation of both the total mass and the moments, which are necessary to find the center of mass.
Outlines
📚 Cal 2 Exam Review: Applications of Integration
This paragraph introduces a Cal 2 exam from Fall 2023, focusing on the application of integration. The speaker reminisces about the structure of older exams and emphasizes the importance of writing one's name on the exam. The first problem discussed is finding the length of a parametric curve, with a detailed explanation of the three scenarios for calculating the length of a curve. The process involves understanding the context of the problem, identifying the correct formula, and applying calculus to find the solution.
🔍 Detailed Analysis of a Cal 2 Exam Problem
The speaker provides a step-by-step solution to a problem from the Cal 2 exam about finding the length of a parametric curve. The explanation includes taking derivatives, setting up the integral, and simplifying the expression to find the length. The speaker also discusses common mistakes to avoid and emphasizes the importance of careful algebraic simplification and checking each step for accuracy.
📐 Surface Area Calculations in Cal 2
The paragraph delves into a two-part problem involving the calculation of surface area when a curve is revolved around an axis. The first part involves setting up an integral to find the surface area generated when the curve y = 2 * x^3 is revolved around the x-axis from x = 1 to x = 4. The second part is similar but involves revolving around the y-axis. The speaker explains the concept of surface area integrals, the importance of understanding radius and length in the context of revolving a curve, and how to set up the integral for both scenarios.
🏗️ Work Done in Lifting a Steel Beam Using a Pulley System
This paragraph presents a word problem involving a construction crew using a pulley system to lift a 500lb steel beam 30ft to the third floor of a building. The cable's weight density is 3lb per foot. The speaker explains how to calculate the work done in lifting the beam by considering the varying weight due to the cable's length as the beam is raised. The problem involves integrating the force over the distance to find the total work, taking into account the changing weight of the cable as the beam is lifted.
📉 Work-Energy Principle in a Pulley System
Continuing from the previous paragraph, the speaker calculates the total work done in lifting the steel beam using a pulley system. The calculation accounts for the decreasing weight of the cable as the beam is raised. The integral from 0 to 30 feet is evaluated, considering the force due to the beam's weight and the cable's weight density. The final answer is given in foot-pounds, representing the work done in lifting the beam.
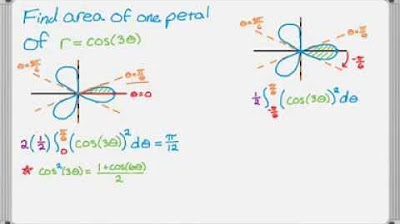
🌀 Area Calculation in Polar Coordinates
The speaker discusses how to find the area enclosed by a curve given in polar coordinates, specifically the curve 4 cosine 2 Theta, from 0 to 2 pi. The problem is approached by considering symmetry and focusing on a quarter of the curve, then multiplying by 8 to account for the full shape. The formula for area in polar coordinates is explained, and the integration is set up accordingly. The process involves using the power reduction formula for cosine squared and evaluating the integral to find the area.
📐 Volume of Revolution Using Shells Method
The paragraph explains how to set up an integral to find the volume of a shape formed by rotating the region between two curves around a given line, using the shells method. The curves are y = 9 - x^2 and y = x + 3, and the rotation is around the line x = 2. The speaker outlines the process of setting up the integral for the volume, emphasizing the importance of identifying the radius and height for the shells and the correct limits of integration. The formula for the volume using the shells method is provided, and the integral is set up without evaluation.
🛠 Volume of Revolution Using Washers Method
This paragraph continues the discussion of the volume of revolution problem, but now the focus is on using the washers method. The speaker explains the need to integrate with respect to y and to express everything in terms of y. The setup involves splitting the integral at the point where the curves intersect and considering the two parts separately. The washers method formula is introduced, and the integral is set up for both parts of the region, taking care to identify the outer and inner radii correctly.
📉 Calculating the Equation of a Tangent Line in Polar Coordinates
The speaker describes the process of finding the Cartesian equation of a tangent line to a polar curve at a specific point. The polar curve is r = 2 + cosine Theta, and the point of tangency is at Theta = pi. The process involves finding the point on the curve, calculating the slope of the tangent line, and converting the polar coordinates to Cartesian coordinates. The slope is found by differentiating the polar equations with respect to Theta and evaluating at the given point. The final equation of the tangent line is given in slope-intercept form.
🏗️ Center of Mass Calculation for a Plate with Variable Density
The final paragraph discusses a center of mass problem for a plate with a variable density function. The plate is bounded by two functions and has a density that increases with x. The speaker explains the concept of mass and moment in the context of center of mass calculations and outlines the process of finding the y-coordinate of the center of mass. The mass is calculated by integrating the density function over the area, and the moment is calculated by considering the distance of each element from the x-axis. The final step is to divide the moment by the mass to find the y-coordinate of the center of mass.
Mindmap
Keywords
💡Cal 2 Exam
💡Integration
💡Parametric Curve
💡Surface Area
💡Work
💡Polar Coordinates
💡Volume of Revolution
💡Center of Mass
💡Density Function
💡Moment
Highlights
Introduction to a Cal 2 exam focusing on applications of integration.
Emphasis on the importance of writing names on exams for proper credit.
Explanation of finding the length of a parametric curve using integration.
Recap of different scenarios for length of curve problems and their respective formulas.
Detailed walkthrough of calculating the length of a specific parametric curve.
Discussion on setting up integrals for surface area of a curve revolved around an axis.
Clarification on the difference between revolving around the x-axis and y-axis and its impact on formulas.
Introduction of a word problem involving work done in lifting a steel beam using a pulley system.
Calculation of work accounting for the varying weight of the cable as the beam is lifted.
Approach to finding the area enclosed by a curve given in polar coordinates.
Use of symmetry to simplify the calculation of the area for a polar curve.
Problem setup for finding the volume of a region rotated around a line using the shell method.
Clarification on the difference between the shell and washer methods for volume of revolution.
Setup for finding the volume of a region using the washer method, with attention to changing functions.
Process of finding the Cartesian equation of a tangent line to a polar curve at a specific point.
Conversion from polar to Cartesian coordinates to find the slope of a tangent line.
Final problem involving the calculation of the center of mass for a plate with variable density.
Explanation of mass and moment calculations for determining the center of mass.
Conclusion summarizing the process and encouraging exam preparation and practice.
Transcripts
Browse More Related Video

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 4)

AP Calculus BC Lesson 9.8

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 2)

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 1)
Calculus Polar Area Rose Curve Example
Calc 3, Exam 1 walkthrough (Spring 2023)
5.0 / 5 (0 votes)
Thanks for rating: